Molar Conductivity - Definition, Formula, Variations, Specific Conductivity

The discovery and understanding of molar conductivity have profound implications across various fields of science and industry. Molar conductivity, a key concept in electrochemistry, was developed over time through the work of various scientists. The concept of molar conductivity is closely related to the work of the Swedish chemist *Svante Arrhenius*. Arrhenius is best known for his contributions to the theory of electrolytic dissociation. The concept of molar conductivity was formally introduced in the late 19th and early 20th centuries. Svante Arrhenius presented his theory of electrolytic dissociation in 1887, which laid the foundation for understanding molar conductivity.

JEE Main 2025: Chemistry Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics |  PYQs

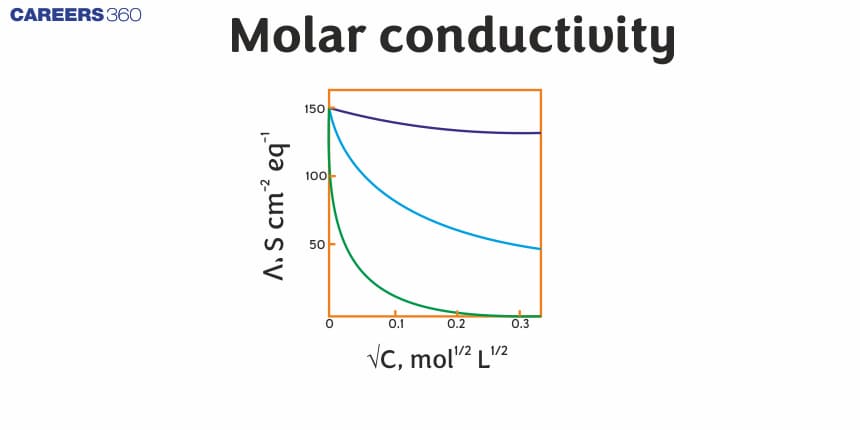
Before the discovery of molar conductivity, it was very difficult to determine the behavior of electrolytes. Molar conductivity is very helpful in predicting that the electrolyte can dissociate into the ions and these conduct electricity. Arrhenius discovered this concept, and it greatly contributes to the modern theory of electrolytes. And this modern theory become a very important part of physical chemistry and electrochemistry as well. Before this concept, it was very challenging to express the movement of ions or electrons that conduct electricity in the solution. Molar conductivity provides a way to predict and measure this behavior of electrolytes.

Molar And Equivalent Conductance

Molar Conductance

The molar conductance is defined as the conductance of all the ions produced by the ionization of 1 mole of an electrolyte when present in V ml of solution. It is denoted by Λm.
Molar conductance $\left(\wedge_{\mathrm{m}}\right)=\kappa \times \mathrm{V}$
where V is the volume in ml containing 1 gm mole of the electrolyte.
If c is the concentration of the solution in mole per liter, then:

$\Lambda_{\mathrm{m}}=\kappa \times \frac{1000}{\mathrm{c}}$
where c is the concentration of the solution in M, the units of $\Lambda_m$ are ohm^-1 cm² mol^-1 or S cm² mol^-1 When the units of $\kappa$ are S cm^-1.

It is to be noted that changing the units of the quantities involved will lead to a change in the formula. For the sake of homogeneity, $\Lambda_m=\frac{\kappa}{C}$ when all the quantities are expressed in their SI unit.

Also, if A_xB_y is an electrolyte dissociating as:
$\begin{aligned} & \mathrm{A}_{\mathrm{x}} \mathrm{B}_{\mathrm{y}} \rightleftharpoons \mathrm{xA}^{\mathrm{y}+}+\mathrm{yB} \mathrm{B}^{\mathrm{x}-} \\ & \text { Thus, } \wedge_{\mathrm{m}} \mathrm{A}_x \mathrm{~B}_{\mathrm{y}}=x \cdot \wedge_{\mathrm{m}}\left(\mathrm{A}^{\mathrm{y}+}\right)+\mathrm{y} \cdot \wedge_{\mathrm{m}}\left(\mathrm{B}^{x-}\right)\end{aligned}$

Equivalent Conductance

One of the factors on which the conductance of an electrolytic solution depends is the concentration of the solution. In order to obtain comparable results for different electrolytes, it is necessary to take equivalent conductance.
It is defined as the conductance of all the ions produced by one gram equivalent of an electrolyte in a given solution. It is denoted by Λ_eq.
$\wedge_{\text {eq }}=\frac{1000 \times \kappa}{\mathrm{N}}$
If ‘V’ is the volume in ml containing 1 gm equivalent of the electrolyte, the above equation can be written as:
$\wedge_{\mathrm{eq}}=\kappa \times \mathrm{V}$
Its units are ohm^-1 cm² equiv^-1 or S cm² equiv^-1. A similar constraint of units exists in the formula as that in molar conductance.
Equivalent conductance is also given as follows:$\begin{aligned} & \text { Equivalent conductance }=\frac{\text { Molar conductance }}{x}, \\ & \text { where } x=\frac{\text { Molecular mass }}{\text { Equivalent mass }}=\mathrm{n}-\text { factor }\end{aligned}$

Recommended topic video on(Molar conductivity)

Some Solved Examples

Example.1

1. Resistance of a conductivity cell filled with a solution of an electrolyte of concentration 0.1 M is 100 $\Omega$ .The conductivity of this solution is 1.29 Sm^-1. The resistance of the same cell, when filled with 0.2 M of the same solution, is 520 $\Omega$ . The molar conductivity (in Sm²mol^-1) of 0.2 M solution of the electrolyte will be :

1)0.0124

2)0.124

3)0.000124

4) (correct)0.00124

Solution

The formula of molar conductivity -

$\Lambda m\left(S^2 \mathrm{~cm}^2 \mathrm{~mol}^{-1}\right)=\frac{\kappa(S \mathrm{~cm})}{1000 \mathrm{Lm}^{-3} \times \text { molarity }\left(\mathrm{mol} \mathrm{L}^{-1}\right)}$

From the first data set, $\mathrm{C}=0.1 \mathrm{M}, \mathrm{R}=100 \Omega, \kappa=1.29 \mathrm{Sm}^{-1}$

$
\begin{aligned}
& K=\frac{1}{R} \times \frac{l}{A} \\
\Rightarrow & 1.29=\frac{1}{100} \times \frac{l}{A}
\end{aligned}
$

Cell constant $=129 \mathrm{~m}^{-1}$
Now, for the second data set.

$\begin{aligned} & \Lambda_m=K \times \frac{100}{M}=\frac{1}{R} \times \frac{l}{A} \times \frac{1000}{M} \\ & \Lambda_m=\frac{1}{520} \Omega^{-1} \times 129 \mathrm{~m}^{-1} \times \frac{1000}{0.2} \times 10^{-6} \\ & \Lambda_m=12.4 \times 10^{-4} \mathrm{Sm}^2 \mathrm{~mol}^{-1} \\ & \Lambda_{\mathrm{m}}=0.000129 \mathrm{Sm}^2 \mathrm{~mol}^{-1}\end{aligned}$

Hence, the answer is the option (4).

Example.2

2. Which of the following graphs is correct between molar conductivity $\left(\wedge_m\right)$ versus $\sqrt{C}$ correct?

1) (correct)

2)

3)

4)

Solution

molar conductivity increases with a decrease in concentration.

$\left(\Lambda_m^0\right)_{K^{+}}>\left(\Lambda_m^0\right)_{N a^{+}}$

Both NaCl and KCl are strong electrolytes but Na⁺ has less conductance than K⁺ due to more hydration therefore graph (1) shows the correct trend.

Therefore, Option(1) is correct.

Example.3

3.If $C=0.000521 \mathrm{molL}^{-1}$and $\Lambda_m=147.81 \mathrm{Scm}^2 \mathrm{~mol}^{-1}$, then what
would be the value of $\Lambda_m^o$ :if $A=87.46 \frac{\mathrm{Scm}^2 \mathrm{~mol}^{-1}}{\left(\mathrm{~mol} L^{-1}\right)^{\frac{1}{2}}}$

1) (correct)147.856

2)147.854

3)147.888

4)147.899

Solution

As we have learned,

Variation of Molar Conductivity in Strong Electrolyte -

$\Lambda m=\Lambda^0 m-A C^{\frac{1}{2}}$

- wherein

For strong electrolytes, $\Lambda m$ increases slowly with dilution.

$\begin{aligned} & \Lambda_m=\Lambda_m^o-A C^{\frac{1}{2}} \\ \Rightarrow & \Lambda_m^o=\Lambda_m+A C^{\frac{1}{2}}=147.81+87.46 \times 0.000521=147.8555 \approx 147.856\end{aligned}$

Hence, the answer is the option (1).

Example.4

4.The limiting molar conductivities $\Lambda^0$ for $\mathrm{NaCl}, \mathrm{KBr}$ and KCl are 126,152 and $150 \mathrm{Scm}^{-2} \mathrm{~mol}^{-1}$ respectively. The value of $\Lambda^0$ for NaBr is $\left(\right.$ in $\left.\mathrm{Scm}^2 \mathrm{~mol}^{-1}\right)$

1) (correct)128

2)176

3)278

4)302

Solution

$\begin{aligned} & \Lambda_{\mathrm{NaCl}}^{\circ}=\Lambda_{\mathrm{Na}^{+}}^{\circ}+\Lambda_{\mathrm{Cl}^{-}}^{\circ} \\ & \Lambda_{\mathrm{KBr}}^{\circ}=\Lambda_{\mathrm{K}^{+}}^{\circ}+\Lambda_{\mathrm{Br}^{-}}^{\circ} \\ & \Lambda_{\mathrm{KCl}}^{\circ}=\Lambda_{\mathrm{K}^{+}}^{\circ}+\Lambda_{\mathrm{Cl}^{-}}^{\circ}\end{aligned}$

Equation (i) + (ii) – (iii)

$\Lambda_{\mathrm{NaBr}}^{\circ}=\Lambda_{\mathrm{Na}^{+}}^{\circ}+\Lambda_{\mathrm{Br}^{-}}^{\circ}$

$=126+152-150=128 \mathrm{~S} \mathrm{~cm}^2 \mathrm{~mol}^{-1}$

Hence, the answer is the option (1).

Example.5

Electrolyte & KCl & $\mathrm{KNO}_3$ & HCl & NaOAc & NaCl
$\left(\mathrm{S} \mathrm{cm}^2 \mathrm{~mol}^{-1}\right)$ & 149.9 & 145.0 & 426.2 & 91.0 & 126.5

5. Calculate the molar conductance of acetic acid using appropriate molar conductances of the electrolytes listed above at infinite dilution in $\mathrm{H}_2 \mathrm{O}$ at $25^{\circ} \mathrm{C}$

1)517.2

2)552.7

3) (correct)390.7

4)217.5

Solution

From Kohlrausch's Law of Infinite Dilution,

$\begin{aligned} \Lambda_{\mathrm{AcOH}}^{\infty} & =\Lambda_{\mathrm{AcONa}}^{\infty}+\Lambda_{\mathrm{HCl}}^{\infty}-\Lambda_{\mathrm{NaCl}}^{\infty} \\ & =91.0+426.2-126.5 \\ & =390.7 \mathrm{Scm}^2 \mathrm{~mol}^{-1}\end{aligned}$

Hence, the answer is 390.7

Example.6

6. The equivalent conductances of Sodium Chloride, Hydrochloric Acid, and Sodium Acetate at infinite dilution are $126.45,426.16$, and $91 \mathrm{ohm}^{-1} \mathrm{~cm}^2 \mathrm{eq}^{-1}$, respectively at $25^{\circ} \mathrm{C}$.. Calculate the equivalent conductance $\left(\right.$ in ohm $\left.{ }^{-1} \mathrm{~cm}^2 \mathrm{eq}^{-1}\right)$of acetic acid at infinite dilution.

1)370.8

2) (correct)390.7

3)377.7

4)407.7

Solution

From Kohlrausch's Law of Infinite Dilution,

$\Lambda_{\mathrm{CH}_3 \mathrm{COONa}}^{\infty}=\lambda_{\mathrm{CH}_3 \mathrm{COO}}{ }^{-}+\lambda_{\mathrm{Na}^{+}}=91$

$\Lambda_{\mathrm{HCl}}^{\infty}=\lambda_{\mathrm{H}^{+}}+\lambda_{\mathrm{Cl}^{-}}=426.15$

$\Lambda_{\mathrm{NaCl}}^{\infty}=\lambda_{\mathrm{Na}^{+}}+\lambda_{\mathrm{Cl}^{-}}=126.45$

Adding (1) and (2) and subtracting (3), we get

$\Lambda_{\mathrm{CH}_3 \mathrm{COOH}}^{\infty}=\lambda_{\mathrm{CH}_3 \mathrm{COO}-}+\lambda_{\mathrm{H}^{+}}=390.7 \mathrm{ohm}^{-1} \mathrm{~cm}^2 \mathrm{eq}^{-1}$

Hence, the answer is 390.7

Hence, the answer is the option (2).

Example.7

7. The molar conductivities of KCl, NaCl, and KNO₃ are 152, 128, and 111 S cm² mol^-1 respectively. What is the molar conductivity (in S cm² mol^-1 ) of NaNO₃?

1)101

2) (correct)87

3)-101

4)-391

Solution

As we have learned,

The formula for limiting molar conductivity for electrolyte -

$\Lambda_m^0=\lambda_{+}^0 \cdot \nu_{+}+\nu_{-} \cdot \lambda^0$

$\lambda_{+}^0$ and $\lambda_{-}^0$

are limiting molar conductivities of cation and anion respectively.

if an electrolyte on dissociation gives $\nu_{+}$cation and $\nu_{-}$ anions.

$\Lambda_{N a N O_3}^o=\Lambda_{\mathrm{NaCl}}^0+\Lambda_{K N O_3}^0-\Lambda_{K C l}^0=128+111-152=87$

Hence, the answer is the option (2).

Summary

Molar conductivity is the quantitative ability of any electrolyte to conduct electricity. With the help of molar conductivity, we can predict the strength of any electrolytes and their nature at the different different concentrations. In electrochemistry, the Molar conductivity is part of deep and broader studies. This discovery became important for the advancement of electrochemistry. We understand here how the ions carry the electrical charges in solutions, which are the center of various physical and chemical processes. As electrolyte applications become widespread in the lab and the chemical industries it is very important to express the behavior and efficiency of electrolytes. Molar conductivity has many applications in the theoretical and practical fields. There are various theoretical models That are influenced by the molar conductivity Debye-Hückel theory, which describes the interaction of ions in any solution. This knowledge is very important in controlling the behavior of electrolytes. Electrochemical processes rely on efficient ion transport. Understanding molar conductivity helped in designing better electrochemical cells and systems by optimizing electrolyte solutions Molar conductance is used to determine the electrolytic strength of solution Molar conductivity helps in assessing how well different electrolytes dissociate into ions in solution, which is crucial for applications like batteries, fuel cells, and electroplating. In Characterizing Electrolyte Solutions to understand how electrolytes behave in different concentrations. It allows for the characterization of weak and strong electrolytes by studying how molar conductivity varies with concentration, providing insights into the degree of dissociation and ion mobility. We are also used for Chemical Analysis to analyze and quantify ionic species in solutions, used in titrations, and other analytical techniques to measure the concentration of ions in solution.