Radial Nodes And Planar Nodes

In the realm of chemistry, many experiments were conducted to understand the structure of atoms and also the subatomic particles. After the discovery of the subatomic particles scientist were keen to know their position inside the atoms. How electrons, protons, and neutrons are arranged, and how does their specific position and arrangement make the atom stable? Are there specific points inside the atom where their probability of finding them is maximum, and where is the minimum or zero? To find out such answers, areas were found inside the atomic structure at the radial and angular plane, where the probability of electrons is zero.

JEE Main 2025: Chemistry Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics |  PYQs

This Story also Contains

1. Radial Node and Angular Node- Fundamental to Atomic Structure:
2. Nodes:
3. Some Solved Examples
4. Conclusion

In this article, we will cover the concept of Electromagnetic Waves and several related parameters. This concept falls under the broader category of Atomic structure, which is a crucial chapter in Class 11 chemistry. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), National Eligibility Entrance Test (NEET), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

Also read -

• NCERT Solutions for Class 11 Chemistry
• NCERT Solutions for Class 12 Chemistry
• NCERT Solutions for All Subjects

Radial Node and Angular Node- Fundamental to Atomic Structure:

In Context with atomic structure, nodes are the specific location, point, and area where finding the probability of an electron is zero. Nodes can be either Radial or Angular.

It has been discovered that generally speaking, ns-orbitals have (n – 1) nodes, meaning that the number of nodes rises as the principal quantum number, n, grows.
Charge cloud diagrams are one way to depict these variations in probability densities.

Nodes:

Total number of nodes = (n - 1)

A radial node is a spherical surface where the probability of finding an electron is zero. The number of radial nodes increases with the principle quantum number (n).

No. of radial nodes = (n- l- 1)

where n is the principal quantum number, l is the azimuthal quantum number.

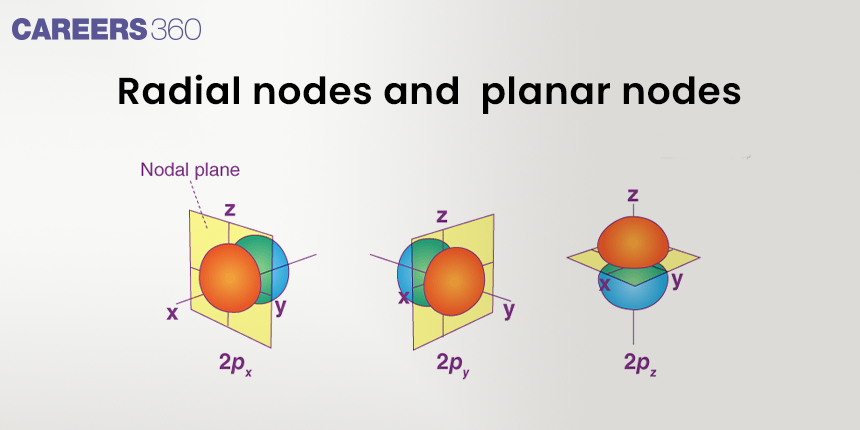
The angular node is also called the nodal plane. An angular node is a plane that passes through the nucleus. The angular node is equal to the azimuthal quantum number (l).

No. of planar nodes = l

where l is the azimuthal quantum number.

Recommended topic video on(Radial Nodes And Planar Nodes)

Some Solved Examples

Example1:The number of radial nodes of 3s and 2p orbital are respectively

1) (correct) 2, 0

2) 0, 2

3) 1, 2

4) 2, 11

Solution

As we learn

For a given orbital, the number of radial nodes =n−l−1

For 3s orbital

n=3, l=0

Number of radial nodes = 3-0-1= 2

For 2p orbital

n = 2, l = 1

Number of radial nodes = 2-1-1 =0

Hence, the answer is the option (1).

Example 2: The number of planar nodes in d_x-y is

1) 0

2) 1

3) (correct) 2

4) 3

Solution

As we learn

No. of planar nodes= l where l is the azimuthal quantum number.

Number of planar nodes = l

For d_xy, l = 2

Number of planar nodes = 2

Hence, the answer is the option (3).

Example 3: The number of radial nodes and 2p orbitals are respectively

1) (correct) 2,0

2) 0,2

3) 1,2

4) 2,1

Solution

We know,

The number of Radial nodes for any electron in any orbital is given by the value of (n−ℓ−1)
For 3 s electrons,
n=3,ℓ=0⇒(n−ℓ−1)=3−0−1=2

And for 2p electron,
n=2,ℓ=1⇒(n−ℓ−1)=2−1−1=0

Hence, the answer is the option (1).

Example 4: A certain orbital has no angular nodes and two radial nodes. The orbital is :

1) (correct) 3s

2) 3p

3) 2s

4) 2p

Solution

the number of angular nodes is given by ‘l’, i.e., one angular node for p orbitals, two angular nodes for ‘d’ orbitals, and so on.

Radial modes = n-l-1

The total number of nodes is given by (n–1), i.e., the sum of l angular nodes and (n – l – 1) radial nodes.

Given

A certain orbital has no angular nodes and two radial nodes,

So, l=0,

It will be s orbital, it does not have angular nodes.

And

Radial nodes = 2

n – l –1 = 2

n – 0 – 1 = 2

n= 3

So, The orbital will be 3s.

Hence, the answer is the option (1).

Example 5: A certain orbital has no angular nodes and two radial nodes. The orbital is :

1) (correct)3s

2) 2p

3) 2s

4) 2p

Solution

the number of angular nodes is given by ‘l’, i.e., one angular node for p orbitals, two angular nodes for ‘d’ orbitals, and so on.

Radial nodes = n-l-1

The total number of nodes is given by (n–1), i.e., the sum of l angular nodes and (n – l – 1) radial nodes.

Given

A certain orbital has no angular nodes and two radial nodes,

So, l=0,

It will be s orbital, it does not have angular nodes.

And

Radial nodes = 2

n – l –1 = 2

n – 0 – 1 = 2

n= 3

So, The orbital will be 3s.

Hence, the answer is the option (1).

Also check-

• NCERT Exemplar Class 11th Chemistry Solutions
• NCERT Exemplar Class 12th Chemistry Solutions
• NCERT Exemplar Solutions for All Subjects

NCERT Chemistry Notes:

• NCERT Notes Class 11th Chemistry
• NCERT Notes Class 12th Chemistry
• NCERT Notes For All Subjects

Conclusion

So, it can noted that radial and angular nodes are quite significant, and it is concepts that are required to explain atomic structure as well as electron properties. Radial nodes describe volumes within the electron cloud inside an atom where the electron density is most likely going to be at its low and is involved in the determination of both the size of the atomic orbitals and the shapes. Angular nodes on the other hand are points on the surfaces of the atoms where the Electron Density Distribution preference along the certain axes is also not present and thus influences the orientation or even the symmetry of the orbitals. Taken together, all of these nodes provide the base from which one can conceptualize how electrons are divided within an atom and therefore, predict chemical bonding or reactivity. Once again, an understanding of the meaning of radial and angular nodes adds more clarity to different happenings within atomic structures and the factors involved in different forms of science.