Speed of electromagnetic radiation and EM radiation

Speed of Electromagnetic Radiation And EM Radiation

Edited By Shivani Poonia | Updated on Sep 16, 2024 03:04 PM IST

Scientists were always curious to understand the relationship between electricity and the magnetic effect. Whenever a current is passed through a current-carrying conductor, it experiences a magnetic effect too. We found that an electric current generates a magnetic field and that two wires carrying currents impose a magnetic force on one another. Moreover, a magnetic field that varies over time produces an electric field. Is the converse also true? Does a fluctuating electric field serve as the source of a magnetic field? It is true.

JEE Main 2025: Chemistry Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

Electromagnetic Radiation - From Spark to Spectrum:
Parameters to Define Wave
Electromagnetic Spectrum :
Solved Examples-
Conclusion

Speed of Electromagnetic Radiation And EM Radiation

Several experiments were conducted to understand the relationship between electric current and magnetic field. According to James Clerk Maxwell (1831–1879), an electric current and a time-varying electric field can both produce a magnetic field. While applying Ampere's circuital equation to calculate the magnetic field at a location outside of a capacitor coupled to a time-varying current, Maxwell found an anomaly in it.

In this article, we will cover the concept of Electromagnetic Waves and several related parameters. This concept falls under the broader category of Atomic structure, which is a crucial chapter in Class 11 chemistry. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), National Eligibility Entrance Test (NEET), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

Let us discuss Electromagnetic waves and several parameters related to the wave such as Wavelength, period, frequency, and speed as the related formula.

Electromagnetic Radiation - From Spark to Spectrum:

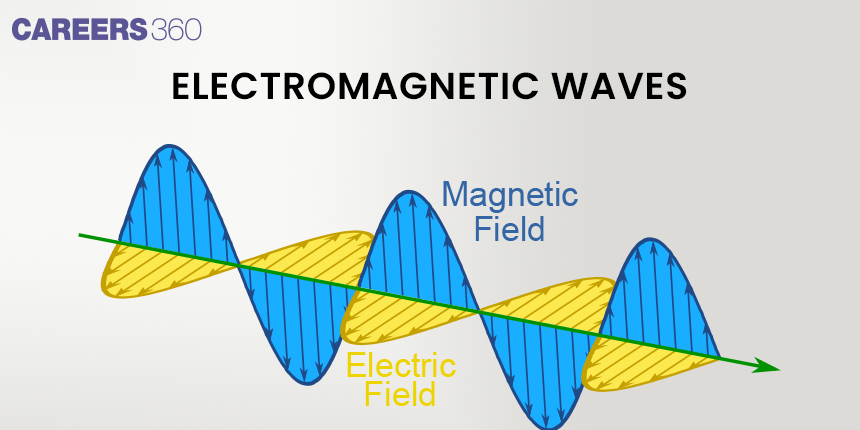
According to electromagnetic wave theory, energy is emitted continuously from a source in the form of radiation (or waves), known as electromagnetic radiation. Electromagnetic radiations have both magnetic field as well as electric field components which oscillate in the phase perpendicular to each other as well as perpendicular to the direction of wave propagation. These waves do not require any medium for propagation and can propagate through a vacuum. Many types of electromagnetic radiation constitute what is known as the electromagnetic spectrum.

Parameters to Define Wave

There are several parameters required to characterize or define a wave. These parameters are defined below:

1. Wavelength $ \text { }(\lambda) $: It is the distance travelled by the wave during one complete oscillation.

The maxima are called crests and the minima are called Troughs. Alternatively, the distance between two consecutive crests or two consecutive troughs is also called the wavelength.

2. Period (T): It is the time required for one complete oscillation or one complete cycle by a wave.

3. Frequency $ (\nu) $ : It is the number of waves produced by the source in one second

It is the inverse of the period. Its SI unit is Hertz (Hz).

$ \nu=\frac{1}{\mathrm{~T}} $

4. Speed (c): It is the distance travelled by the wave in one second.
In one time period, the wave travels a distance equal to its wavelength.

$ \begin{aligned}
& \mathrm{c}=\frac{\text { distance }}{\text { time }}=\frac{\text { Wavelength }}{\text { Time Period }}=\frac{\lambda}{\mathrm{T}} \\
& \because \nu=\frac{1}{\mathrm{~T}} \\
& \therefore \mathrm{c}=\nu \times \lambda
\end{aligned} $

The speed of all the different components of light is the same i.e. they travel with the speed of $ 3 \times 10^8 \mathrm{~m} / \mathrm{s} $. Their frequency and wavelength are different
5. Wave number $ (\bar{\nu}) $: It is the inverse of the wavelength. It can also be defined as the number of wavelengths present in unit length.

$ \bar{\nu}=\frac{1}{\lambda} $

Also check-

Electromagnetic Spectrum :

The rays present on the left extreme of the spectrum have the greatest frequency, least wavelength, and the greatest energy, As the frequency increases, wavelength decreases, and energy increases.

Solved Examples-

Example 1: Which of the following EM radiation lies in the highest energy region?

1) UV rays
2) X-rays
3) (correct) $ \gamma- rays $
4) Radio waves

Solution:
Wavelength of electromagnetic radiation and EM radiation -Radio wave - $ 3 \times 10^{14} $ to $ 3 \times 10^7 $ Angstrom
Microwave -$ 3 \times 10^9 $ to $ 3 \times 10^6 $ Angstrom
Infrared - $ 6 \times 6^6 $ to 7600 Angstrom
Ultraviolet -3800 to 150 Angstrom
X-rays -150 to 0.1 Angstrom
Gamma rays -0.1 to 0.01 Angstrom

Now, The Relation between Energy and Wavelength of EM Waves,
$ \begin{aligned}
& \mathrm{E}=\mathrm{h} \nu=\frac{\mathrm{hc}}{\lambda} \\
& \mathrm{E} \propto \frac{1}{\lambda}
\end{aligned} $

The energy of the EM wave is in the following order $ \gamma $ rays > $ \mathrm{X} $ - rays > UV rays> visible rays> Infrared rays> microwave > radio wave

Hence, the answer is the option(3).

Example 2: The value of Planck's constant is $ 6.63 \times 10^{-34} \mathrm{Js} $. The velocity of light is $ 3.0 \times 10^8 \mathrm{~ms}^{-1}$. Which value is closest to the wavelength in nanometers of a quantum of light with a frequency of $ 8 \times 10^{15} s^{-1} $
1) $ 3 \times 10^7 $
2) $ 2 \times 10^{-25} $
3) $ 5 \times 10^{-18} $
4) (correct) 37.5

Solution:
We know that,

$ \begin{aligned}
& \nu=\frac{c}{\lambda} \\
& \Rightarrow \lambda=\frac{c}{\nu}
\end{aligned} $

$ \Rightarrow \lambda=\frac{c}{\nu}=\frac{3 \times 10^8}{8 \times 10^{15}}=3.75 \times 10^{-8} \mathrm{~m} $

Hence, the answer is the option (4).

Example 3: Assertion: Electromagnetic waves can be polarised.

Reasoning: The direction of the electric field vector in an electromagnetic wave determines its polarization.

1) (correct) Both assertion and reasoning are true, and the reasoning is the correct explanation of the assertion.

2) Both assertion and reasoning are true, but the reasoning is not the correct explanation of the assertion.

3) The assertion is true, but the reasoning is false.

4)Both assertion and reasoning are false.

Solution:

An electromagnetic wave consists of oscillating electric and magnetic fields that are perpendicular to each other and the direction of propagation of the wave. The direction of the electric field vector in an electromagnetic wave determines its polarization. If the electric field vector oscillates in a single plane, the wave is said to be polarised. Electromagnetic waves can be polarised by using a polarising filter, which transmits only the waves that have their electric field vectors oriented in a particular direction. Therefore, both the assertion and reasoning are true, and the reasoning is the correct explanation of the assertion.

Hence, the answer is the option (1).

Conclusion

We discussed the electromagnetic wave theory, in which we understand the relationship between electricity and magnetism. The electromagnetic wave emits energy and is hence also called electromagnetic radiation. Understanding electromagnetic waves allows us to predict other phenomena related to electricity and other electrical transformations. The Generators, Transformers, and other wireless devices principles are directly or indirectly based on electromagnetic wave theory.

Also check-

Frequently Asked Questions (FAQs)

1. Name two waves from the visible spectrum.

Red light and Blue light.

2. How are wavelength and Time period related to the speed of light?

$ \mathrm{c}=\frac{\text { distance }}{\text { time }}=\frac{\text { Wavelength }}{\text { Time Period }}=\frac{\lambda}{\mathrm{T}} $

3. What is an Electromagnetic wave?

Electromagnetic waves or EM waves are the source of energy that travels in free space and does not require a medium to propagate. it has a dual nature one is wave nature and another particle nature which is called photons.

4. What is the range of EM waves for human audible range?

The audible spectrum for the human ear is 20 to 20,000 Hz

5. What are the different uses of X-rays?

X-rays play very crucial roles in different fields such as security scanning, dental imaging, in medical imaging 1. Radiography, 2. CT scan or computed Tomography.