Addition and Subtraction of Fractions

In our daily life we deal with numbers all around us in some form or the other. We perform the elementary arithmetic operations on them like addition, subtraction, multiplication, division, etc. and find it easy to work with whole and natural numbers. However, when it comes to dealing with parts or fractions and perform addition or subtraction with them, we find it a bit difficult.

This Story also Contains

1. Addition of Fractions
2. Subtraction of Fractions
3. Addition of Fractions Examples with Answers

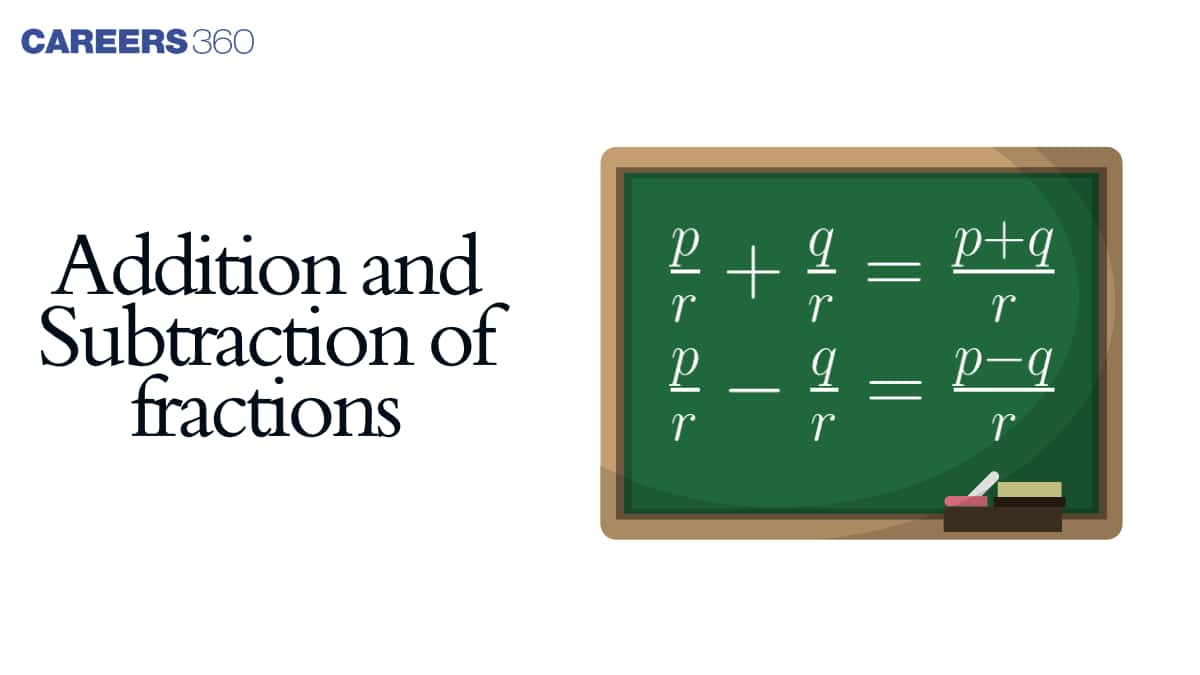
In this article we will discuss about the concept of addition of fractions in detail. We will also look into addition of fractions with different denominators, addition of fractions questions, addition of fractions with answers and much more.

Addition of Fractions

Fractions are defined as a number in the form $\frac{p}{q}$. Addition of fractions helps us to deal with fractions which have same or different denominators and the process of adding them. For example, here we perform the addition of two fractions and that gives us a specific result.

If the denominator comes out to be same, we add them directly and call them like fractions. While if not same, they are unlike fractions.

Addition of Fractions with Same Denominators

If the above condition is met, we simply add the numerator and keep denominator same. In the example below, we add 2 fractions namely $\frac{p}{q}$ and $\frac{r}{q}$. Since their denominator is same, we directly add them to get the answer as $\frac{\mathrm{p}+\mathrm{r}}{ \mathrm{q}}$ fraction.

For example: We add the fractions: $\frac{3}{6}$ and $\frac{4}{6}$.
Since the denominators are same, hence we can add the numerators directly.

$
\frac{3}{6}+\frac{4}{6}=\frac{3+4}{6}=\frac{7}{6}
$

Addition of Fractions with Different Denominators

If we face such condition, then we cannot add the numerators directly. Hence, we make the denominator same by taking LCM and then proceed to add the numerators.

For example: Add $\frac{3}{12} + \frac{4}{8}$
Solution: Both the fractions $\frac{3}{12}$ and $\frac{4}{8}$ have different denominators.
We can write $\frac{3}{12}=\frac{1}{4}$, in a simplified fraction and $\frac{4}{8}$ as $\frac{1}{2}$.
Now, $\frac{1}{4}$ and $\frac{1}{2}$ are two fractions.
LCM of $2$ and $4=4$
Now we multiply $\frac{1}{2}$ by $\frac{2}{2}$.

$
\frac{1}{2} \times \frac{2}{2}=\frac{2}{4}
$

Now add $\frac{1}{4}$ and $\frac{1}{2}$

$
\frac{1}{4}+\frac{1}{2}=\frac{3}{4}
$

Hence, the sum of $\frac{3}{12}$ and $\frac{4}{8}$ is $\frac{3}{4}$.

Adding fractions with whole numbers

First we write given whole number in the form of a fraction (for example, $\frac{3}{1}$ ), then make denominator same and add fractions. At last we simplify them.

For example: Add $\frac{9}{2}+4$
Here, $\frac{9}{2}$ is a fraction and $4$ is a whole number.
We can write $4$ as $\frac{4}{1}$.
Now making the denominators same, we get;
$\frac{9}{26}$ and $\frac{4}{1} \times(\frac{2}{2})=\frac{8}{2}$
Add $\frac{9}{2}$ and $\frac{8}{2}$

$
\frac{9}{2}+\frac{8}{2}=\frac{17}{2}
$

Hence, the sum of $\frac{9}{2}$ and $4$ is $\frac{17}{2}$.

Adding Fractions with Co-prime Denominators

Co-prime denominators are those which do not have common factors, other than 1. Hence, we follow the below steps for adding fractions with co prime denominators.

1. First we check the denominators whether they are co-prime or not.

2. Then we multiply the first fraction (numerator and denominator) with the denominator of the other fraction and the second fraction (numerator and denominator) with the denominator of the first fraction.

3. At last we add the resulting fractions and simplify.

For example, we add the fractions $\frac{2}{5}$ and $\frac{3}{4}$.
The denominators 5 and 4 are coprime since they have only one highest common factor 1.

So, $(\frac{2}{5})+(\frac{3}{4})=\frac{2}{5} \times \frac{3}{6} = \frac{(4 \times 2)+(3 \times 5)}{4 \times 5}=\frac{23}{20}$

Adding Mixed Fractions

A mixed fraction is a combination of a whole number and a fraction. So we follow the steps as :

1. We convert the given mixed fraction into improper fraction.

2. Next we check if denominators are same or not. If different, then we rationalize them.

3. Next we add the fractions and simplify.

For example: $1\frac{1}{5} + 2\frac{4}{3}$
Solution:
Step 1: We convert the given mixed fractions to improper fractions.

$1\frac{1}{5} = \frac{(1 \times 5)+1}{5} = \frac{6}{5}$

$ 2\frac{3}{4} = \frac{(2 \times 3)+4}{3} = \frac{10}{3}$

Step 2: Next we make the denominators same by taking the LCM and multiplying the suitables fractions for both.

LCM of 3 and 5 is 15.
So, $\frac{6}{5}=\frac{6}{5} \times \frac{3}{3}=\frac{18}{15}$

$
\frac{10}{3}=\frac{10}{3} \times \frac{5}{5}= \frac{50}{15}
$

Step 3: Next we take the denominator as common and add numerators.

$
\frac{18}{15}+\frac{50}{15}=\frac{18+50}{15}=\frac{68}{15}
$

Subtraction of Fractions

If the denominators are same, we subtract directly and if different, we need to rationalise them first and then do subtraction.

Example 1: Subtract $\frac{5}{3}$ from $\frac{8}{3}$.
Solution: Since the denominator of two fractions $\frac{5}{3}$ and $\frac{8}{3}$ is common, therefore, we can directly subtract them:

$
\frac{8}{3} - \frac{5}{3}=\frac{8-5}{3}=\frac{3}{3}=1
$

Addition of Fractions Examples with Answers

Example 1: Add $\frac{2}{10}$ and $\frac{7}{10}$.
Solution: Given fractions: $\frac{2}{10}$ and $\frac{7}{10}$
Since the denominators are same, we add directly.

$
\begin{aligned}
& \frac{2}{10}+\frac{7}{10} \\
& =\frac{2+7}{10} \\
& =\frac{9}{10}
\end{aligned}
$

Example 2: Add $\frac{13}{5}$ and $4$.
Solution: We can write $4$ as $\frac{4}{1}$
Now, $\frac{13}{5}$ and $\frac{4}{1}$ are the two fractions to be added. We need to simplify the denominators first here as they are not same.

$
\begin{aligned}
& \frac{13}{5}+\frac{4}{1} \\
& \operatorname{LCM}(5,1)=5
\end{aligned}
$

Multiplying the second fraction, $\frac{4}{1}$ by $5$ both in numerator and denominator, $\frac{(4 \times 5)}{(1 \times 5)}=\frac{20}{5}$

Now $\frac{13}{5}$ and $\frac{20}{5}$ have a common denominator $5$ and we add them.

$
\begin{aligned}
&\frac{13}{5} +\frac{20}{5} \\
& =\frac{33}{5}
\end{aligned}
$

Example 3: Add the following fractions: $\frac{11}{7}$ and $\frac{3}{7}$
Solution:
The given fractions are like fractions. Hence, $\frac{11}{7}+\frac{3}{7}=\frac{11+3}{7}=\frac{14}{7}=2$

Example 4: Add the following fractions: $\frac{2}{5}$ and $\frac{4}{3}$
Solution:
The given fractions are unlike fractions. Hence, LCM of $3$ and $5$ is $15$.

$
\begin{aligned}
& \frac{2}{5}+\frac{4}{3}=(\frac{2}{5} \times \frac{3}{3})+(\frac{4}{3} \times \frac{5}{5}) \\
& =\frac{6}{15}+\frac{20}{15} \\
& =\frac{6+20}{5}=\frac{26}{15}
\end{aligned}
$

Example 5: How to add a whole number and a fraction: $9+\frac{1}{3}$?
Solution: The whole number $9$ can be written in the form of a fraction as $\frac{9}{1}$. Now,

$
\begin{aligned}
& 9+\frac{1}{3}=\frac{9}{1}+\frac{1}{3} \\
& =(\frac{9}{1} \times \frac{3}{3})+\frac{1}{3} \\
& =\frac{27}{3}+\frac{1}{3} \\
& =\frac{27+1}{3} \\
& =\frac{28}{3}
\end{aligned}
$

For more such questions refer to addition of fractions worksheets with answers.

List of Topics Related to Additive Inverse