What is Altitude of a Triangle? Definition, Formulas and Examples

The Altitude of a Triangle

Edited By Team Careers360 | Updated on Jan 29, 2024 10:38 AM IST

The altitude of a triangle is the perpendicular drawn from the vertex of a triangle to the opposite side. As there are only three sides in a triangle, therefore only three perpendiculars can be drawn in it on a side from opposite vertices. Different triangles have different kinds of altitudes. The height of a triangle is used in calculating the area of a triangle. This height or length of perpendicular is generally denoted by the letter' h'.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics

This Story also Contains

Properties of Altitude of a Triangle
The altitude of a Triangle Formula
Height of a Scalene Triangle
Altitudes of Different Triangles
The altitude of an Equilateral Triangle
The altitude of an Isosceles Triangle
The altitude of a right-angled triangle-
The formulas for the altitude are summed up in the following table.
Difference Between Median and Altitude of Triangle

Properties of Altitude of a Triangle

• A triangle can have three altitudes.

• The altitudes can be within or outside the triangle which is depended upon the type of triangle.

• The altitude makes an angle of 90 ° to the opposite side of the triangle to it.

• The point of intersection of the three altitudes of a triangle is said to be the orthocenter of the triangle.

The altitude of a Triangle Formula

The formula we use to find the area of a triangle is given below-

A=\frac{1}{2}*B*H (here the H represents the altitude and B represents the base).

Using this formula, we can calculate the height( altitude) of a triangle

Altitude=\frac{\lgroup 2*Area\rgroup}{Base}

Height of a Scalene Triangle

In the scalene triangle, all three sides are of different lengths. therefore, we get three different heights or lengths of altitudes corresponding to each side.

To find the altitude or height of a scalene triangle, we use Heron's formula which is given below-

h=2\sqrt{s}[s-a][s-b][s-c]bh=2s[s-a][s-b][s-c]

Here , h is the height or altitude of the triangle,

s is the semi- perimeter;

a, b, and c are the sides of the triangle.

The path to deciding the formula for the altitude of a scalene triangle is as follows

• Using Heron's formula the area of a triangle will be equal to

A=2\sqrt{s}[s-a][s-b][s-c]

A={s}[s-a][s-b][s-c]

• The formula to find the area of a triangle with respect to its base' B ' and altitude' H ' is

A=\frac{1}{2}*B*H

• If we place both the area formulas the same, so, we get,

\frac{1}{2}*b*h=\sqrt{s. \left ( s-a \right ) .\left ( s-b \right ).\left ( s-c \right )}\\

• Hence, the altitude of a scalene triangle is

h=2\sqrt{s. \left ( s-a \right ) .\left ( s-b \right ).\left ( s-c \right )}/\:b

Altitudes of Different Triangles

Regarding altitude, in different triangles, there will be different types of altitude.

For an obtuse-angled triangle, two altitudes are outside and one inside the triangle.

For an acute angled triangle, all three altitudes are inside the triangle.

For a right-angled triangle, the perpendicular sides are altitudes for each other, while the altitude on the hypotenuse is inside the triangle.

The altitude of an Equilateral Triangle

A triangle in which all three sides are equal is said to be an equilateral triangle.

As all three sides and angles are equal in an equilateral triangle, all the altitudes of an equilateral triangle are also equal.
In an equilateral triangle, altitude, median, and angle bisector are all given by the same line.
The length of altitude of an equilateral triangle with side ‘a’ is given by (√3/2)a.

The altitude of an Isosceles Triangle

An isosceles triangle is a triangle in which two sides are equal. The altitude of an isosceles triangle is vertical to its base.

In an isosceles triangle, the altitudes on the equal sides are equal in length.
In an isosceles triangle, the altitude, median, and angle bisector from the common vertex of equal sides are represented by same line.
The length of this altitude is given by h = √(a2 - (b2/4)), where a is the length of equal sides and b is the length of third side, and h is the length of altitude corresponding to the third (unequal) side.

The altitude of a right-angled triangle-

It divides the triangle into two same triangles.

From the right triangle altitude theorem, the altitude on the hypotenuse is always equal to the geometric mean of line segments formed by altitude on the hypotenuse

In a right triangle, when a perpendicular is drawn from the vertex to the hypotenuse, two similar right triangles are formed. This is known as the right triangle altitude theorem.

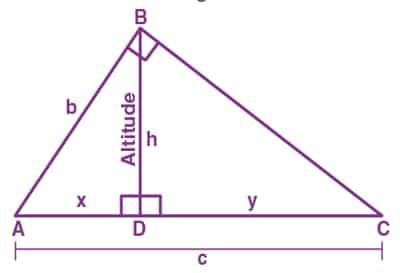
1706452282543

In the above triangle,

△ADB ∼ △BDC

Therefore

\frac{AD}{BD}=\frac{BD}{DC}

BD^{2}=AD*DC

h^{2}=x*y

h=\sqrt{xy}

Hence, this shows the altitude of a right triangle.

The formulas for the altitude are summed up in the following table.

Scalene Triangle	h=2\sqrt{s. \left ( s-a \right ) .\left ( s-b \right ).\left ( s-c \right )}/\:b
Isosceles Triangle	h=\sqrt{\:a^{2}-\frac{b^{2}}{4}}
Equilateral Triangle	h=\frac{\sqrt{3}}{2}a
Right Triangle	h=\sqrt{xy}

Difference Between Median and Altitude of Triangle

The median and the altitude of a triangle are the types of triangles whose line segments join the vertex to the opposite side of a triangle. They differ from each other

Let’s understand the difference table which is given below

Median of a Triangle	The altitude of a Triangle
The median of a triangle is the line segment which is drawn from the vertex to the opposite side of the triangle.	The altitude of a triangle is the perpendicular distance from the base to the opposite vertex of the triangle.
It always lies inside the triangle.	The altitude of a triangle can be both outside or inside of the triangle which depends upon the type of triangle.
Median of the triangle divides a triangle into two equal parts.	On the other hand, the altitude of a triangle may or may not divide any triangle into two equal parts.
The median of the triangle always bisects the base of the triangle in two equal parts.	The altitude of the triangle may or may not bisect the base of the triangle.
The centroid of the triangle is known as the point where all three medians of a triangle meet at a point.	The orthocenter of that triangle is defined as the point where all the three altitudes of the triangle meet each other.