Angle of Intersection between Two Curves: Formula, Examples

The angle of Intersection is an important concept in calculus. It is used to find out the angle between the curves. The tangent line to the curve is a straight line that touches a curve at a single point without crossing it at that point. These concepts of Angle of Intersection between two curves have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

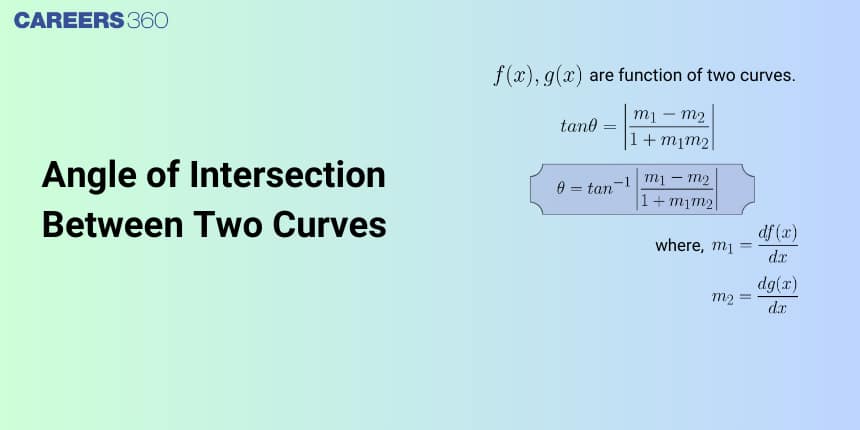
In this article, we will cover the concept of the Angle of Intersection of two Curves. This topic falls under the broader category of Calculus, which is a crucial chapter in Class 11 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of six questions have been asked on this topic in JEE Main from 2013 to 2023, including one in 2013, one in 2018, one in 2019, and three in 2021.

What is the Angle of Intersection?

The angle of intersection of two curves is defined as the angle between the tangents to the two curves at their point of intersection
Let $C_1$ and $C_2$ be two curves having equations $y=f(x)$ and $y=g(x)$, respectively.
Let $P T_1$ and $P T_2$ be two tangents to the curves $C_1$ and $C_2$ at their point of intersection.
Let $\theta$ be the angle between the two tangents $P T_1$ and $P T_2$ and $\theta_1$ and do tangents make the angles with the positive direction of the X -axis in the anti-clockwise sense.

Then

$
m_2=\tan \theta_2=\left(\frac{\mathrm{d} y}{\mathrm{~d} x}\right)_{C_2}
$

From the figure it follows, $\theta=\theta_2-\theta_1$

$
\begin{aligned}
& \Rightarrow \tan \theta=\tan \left(\theta_2-\theta_1\right)=\frac{\tan \theta_2-\tan \theta_2}{1+\tan \theta_2 \tan \theta_1} \\
& \Rightarrow=\tan \theta\left|\frac{\left(\frac{\mathrm{d} y}{\mathrm{~d} x}\right)_{C_1}-\left(\frac{\mathrm{d} y}{\mathrm{~d} x}\right)_{C_2}}{1+\left(\frac{\mathrm{d} y}{\mathrm{~d} x}\right)_{C_1}\left(\frac{\mathrm{d} y}{\mathrm{~d} x}\right)_{C_2}}\right|
\end{aligned}
$

The intersection of these curves is defined as the acute angle between the tangents.

The angle of Intersection of Two Curves

Let y = f (x) and y = g (x) be two curves intersecting at a point P(x₀, y₀) . Then the angle of intersection of two curves is defined as the angle between the tangent to the two curves at the point of intersection.

Let $P T_1$ and $P T_2$ be tangents to the curve $y=f(x)$ and $y=g(x)$ at their point of intersection.
Let $\Theta$ be the angle between two tangents $P T_1$ and $P T_2, \Theta_1$ and $\Theta_2$ are angles made by tangents $P T_1$ and $P T_2$ with the positive direction of $x$-axis, then

$
\begin{aligned}
& m_1=\tan \theta_1=\left(\frac{d}{d x}(f(x))\right)_{\left(x 0, y_0\right)} \\
& m_2=\tan \theta_2=\left(\frac{d}{d x}(g(x))\right)_{\left(x_0, y_0\right)}
\end{aligned}
$

from the figure $\theta=\theta_1-\theta_2$

$
\begin{aligned}
& \Rightarrow \tan \theta=\tan \left(\theta_1-\theta_2\right)=\frac{\tan \theta_1-\tan \theta_2}{1+\tan \theta_1 \cdot \tan \theta_2} \\
& \Rightarrow \tan \theta=\left|\frac{\left(\frac{\mathbf{d}}{\mathrm{dx}}(\mathbf{f}(\mathbf{x}))\right)_{\left(\mathbf{x}_0, \mathbf{y}_{\mathbf{o}}\right)}-\left(\frac{\mathrm{d}}{\mathrm{dx}}(\mathbf{g}(\mathbf{x}))\right)_{\left(\mathbf{x}_0, \mathbf{y}_{\mathbf{o}}\right)}}{1+\left(\frac{\mathrm{d}}{\mathrm{dx}}(\mathbf{f}(\mathbf{x}))\right)_{\left(\mathbf{x}_{\mathbf{0}}, \mathbf{y}_{\mathbf{o}}\right)} \cdot\left(\frac{\mathrm{d}}{\mathbf{d x}}(\mathbf{g}(\mathbf{x}))\right)_{\left(\mathbf{x}_0, \mathbf{y}_0\right)}}\right|
\end{aligned}
$

Orthogonal Curves

If the angle of the intersection of two curves is a right angle then two curves are called orthogonal curves.
In this case, $\tan \theta=90^{\circ}$

$
\Rightarrow\left(\frac{d}{d x}(f(x))\right)_{\left(x_0, y_0\right)} \cdot\left(\frac{d}{d x}(g(x))\right)_{\left(x_0, y_0\right)}=-1
$

this is also the condition for two curves to be orthogonal.

Condition for two curves to touch each other

$
\left(\frac{d}{d x}(f(x))\right)_{\left(x_0, y_0\right)}=\left(\frac{d}{d x}(g(x))\right)_{\left(x_0, y_0\right)}
$

Recommended Video Based on Angle of Intersection of Two Curves:

Solved Examples Based On Angle of Intersection of Two Curves:

Example 1: If the curves $y^2=6 x, 9 x^2+b y^2=16$ intersect each other at right angles, then the value of b is :
[JEE Main 2028]
1) $9 / 2$
2) 6
3) $7 / 2$
4) 4

Solution
As we have learned
Condition of Orthogonality -
Two curves intersect each other orthogonally if the tangents to each of them subtend a right angle at the point of intersection of two curves:

$
m_1 \times m_2=-1
$

$
\begin{aligned}
& y^2=6 x \ldots \ldots \ldots \\
& 9 x^2+6 b x=16
\end{aligned}
$

$\qquad$ $\qquad$
Slope of tangent of first curve

$
\begin{aligned}
& 2 y \frac{d y}{d x}=6 \Rightarrow \frac{d y}{d x}=\frac{6}{2 y} \\
& m_1=\frac{6}{2 y}
\end{aligned}
$

Slope of tangent of second curve

$
\begin{aligned}
& 18 x+2 b y \frac{d y}{d x}=0 \\
& \Rightarrow \frac{d y}{d x}=\frac{-9 x}{b y} \\
& \frac{-9 x}{b y}=m_2
\end{aligned}
$

So $m_1 \times m_2=-1$

$
\begin{aligned}
& m_1 m_2=-1 \\
& \left(\frac{6}{2 y}\right)\left(\frac{-b x}{9 y}\right)=1 \\
& -27 x=-b y^2 \\
& -27 x=-b(6 x) \\
& b=\frac{27}{6}=\frac{9}{2}
\end{aligned}
$

$\lim _{y \rightarrow 0} \frac{\sqrt{1+\sqrt{1+y^4}}-\sqrt{2}}{y^4}$
[JEE Main 2019]
1) exists and equals $\frac{1}{4 \sqrt{2}}$
2) exists and equals $\frac{1}{2 \sqrt{2}(\sqrt{2}+1)}$
3) exists and equals $\frac{1}{2 \sqrt{2}}$
4) does not exist|

Solution
Angle of intersection of two curves -
The angle of intersection of two curves is the angle subtended between the tangents at their point of intersection Let $m_1$ \& $m_2$ are two slope of tangents at intersection point of two curves then

$
\tan \theta=\frac{\left[m_1-m_2\right]}{1+m_1 m_2}
$

where $\theta$ is angle between two curves tangents.

$
\lim _{y \rightarrow 0} \frac{\sqrt{1+\sqrt{1+r^4}}-\sqrt{2}}{y^4}
$

$
\begin{aligned}
& \Rightarrow \lim _{y \rightarrow 0} \frac{\sqrt{1+\sqrt{1+y^4}}-\sqrt{2}}{y^4} \times \frac{\sqrt{1+\sqrt{1+y^4}}+\sqrt{2}}{\sqrt{1+\sqrt{1+y^4}}+\sqrt{2}} \\
& \Rightarrow \lim _{y \rightarrow 0} \frac{1+\sqrt{1+y^4}-2}{y^4\left(\sqrt{1+\sqrt{1+y^4}}\right)+\sqrt{2}}
\end{aligned}
$

again factorize

$
\begin{aligned}
& \Rightarrow \lim _{y \rightarrow 0} \frac{\left(\sqrt{1+y^4}\right)-1}{y^4\left(\sqrt{1+\sqrt{1+y^4}}\right)+\sqrt{2}} \times \frac{\sqrt{1+y^4}+1}{\sqrt{1+y^4}+1} \\
& \Rightarrow \lim _{y \rightarrow 0} \frac{1+y^4-1}{y^4\left(\sqrt{1+\sqrt{1+y^4}+\sqrt{2}}\right)\left(\sqrt{1+y^4}+1\right)} \\
& \Rightarrow \lim _{y \rightarrow 0} \frac{1}{\left(\sqrt{1+\sqrt{1+y^4}+\sqrt{2}}\right)\left(\sqrt{1+y^4}+1\right)}=\frac{1}{4 \sqrt{2}}
\end{aligned}
$

ellipse $\frac{x^2}{9}+\frac{y^2}{1}=1$ and the circle $x^2+y^2=3$

Example 3: Let $\theta$ be the acute angle between the tangents to the at their point of intersection in the first quadrant. Then $\tan \Theta$ is equal to:
[JEE Main 2021]
1) $\frac{5}{2 \sqrt{3}}$
2) $\frac{4}{\sqrt{3}}$
3) $\frac{2}{\sqrt{3}}$
4) 2

Solution

$
\begin{aligned}
& x^2=3-y^2, \frac{x^2}{9}+y^2=1 \\
& \Rightarrow 3-y^2+3 y^2=9 \Rightarrow 8 y^2=6 \\
& \Rightarrow x^2=3-\frac{3}{4}=\frac{9}{4} \Rightarrow x= \pm \frac{3}{2}
\end{aligned}
$

So, the point of intersection in the first quadrant is

$
\left(\frac{3}{2}, \frac{\sqrt{3}}{2}\right)
$
Slope of Tangent to ellipse

$
=m_1: \frac{-1}{9} \frac{x_1}{y_1}=\frac{-\sqrt{3}}{9} \text { slope of Tangent to circle }=m_2: \frac{-x_1}{y_1}=-\sqrt{3}
$

$\tan \theta=\left|\frac{m_1-m_2}{1+m_1 m_2}\right|=\left|\frac{\sqrt{3}-\frac{\sqrt{3}}{9}}{1+\frac{3}{9}}\right|$

$
=\frac{8 \sqrt{3}}{12}=\frac{2 \sqrt{3}}{3}=\frac{2}{\sqrt{3}}
$
Hence, the answer is the option (3).

Example 4: An angle of intersection of the curves $\frac{x^2}{\mathrm{a}^2}+\frac{y^2}{\mathrm{~b}^2}=1$ and $x^2+y^2=\mathrm{ab}, \mathrm{a}>\mathrm{b}$ is :
[JEE Main 2021]

$
\begin{aligned}
& \text { 1) } \tan ^{-1}(2 \sqrt{a b}) \\
& \tan ^{-1}\left(\frac{a+b}{\sqrt{a b}}\right) \\
& \text { 2) } \tan ^{-1}\left(\frac{a-b}{\sqrt{a b}}\right) \\
& \text { 4) } \tan ^{-1}\left(\frac{a-b}{2 \sqrt{a b}}\right)
\end{aligned}
$

Solution

Find a point of intersection of the curves

$
\begin{aligned}
& x^2=a b-y^2 \\
& \Rightarrow \frac{a b-y^2}{a^2}+\frac{y^2}{b^2}=1 \\
& \Rightarrow y^2\left(\frac{1}{b^2-\frac{1}{a^2}}\right)=1-\frac{a b}{a^2}=1-\frac{b}{a} \\
& \Rightarrow y^2 \frac{\left(a^2-b^2\right)}{a^2 b^2}=\frac{a-b}{a} \\
& \Rightarrow y^2=\frac{a b^2}{a+b} \Rightarrow y=b \sqrt{\frac{a}{a+b}} \\
& x^2=a b-\frac{a b^2}{a+b}=\frac{a^2 b}{a+b} \Rightarrow x=a \sqrt{\frac{b}{a+b}} \\
& \text { So, }\left(x_1, y_1\right)=\left(a \sqrt{\frac{b}{a+b}}, b \sqrt{\frac{a}{a+b}}\right) \\
& C_1: \frac{x^2}{a^2}+\frac{y^2}{b^2}=1
\end{aligned}
$

Slope of tangent at $\left(x_1, y_1\right)$

$
\begin{aligned}
& m_1=\frac{-x_1 b^2}{y_1 a^2}=\frac{-a \sqrt{b}}{b \sqrt{a}} \times \frac{b^2}{a^2}=-\left(\frac{b}{a}\right)^{3 / 2} \\
& C_2: x^2-y^2=a b
\end{aligned}
$

Slope of tangent at $\left(x_1, y_1\right)$

$
\begin{aligned}
& m_2=\frac{-x_1}{y_1}=\frac{-a \sqrt{b}}{b \sqrt{a}}=-\left(\frac{a}{b}^{1 / 2}\right) \\
& \tan \theta=\left|\frac{m_1-m_2}{1+m_1 m_2}\right|:\left|\frac{-\frac{b \sqrt{b}}{a \sqrt{a}}+\frac{\sqrt{a}}{\sqrt{b}}}{1+\frac{b \sqrt{b}}{a \sqrt{a}} \times \frac{\sqrt{a}}{\sqrt{b}}}\right|=\left|\frac{\frac{a^2-b^2}{a \sqrt{a b}}}{\frac{a+b}{a}}\right| \\
& \Rightarrow \theta=\tan ^{-1}\left(\frac{a-b}{\sqrt{a b}}\right)
\end{aligned}
$

Hence, the answer is the option (2).

$\frac{x^2}{\alpha}+\frac{y^2}{4}=1$ and $y^3=16 x$ intersect at right angles, then a value of $\alpha$ is:
[JEE Main 2013]
1) 2
2) $\frac{4}{3}$
3) $\frac{1}{2}$
4) $\frac{3}{4}$

Solution

$
\begin{aligned}
& \frac{x^2}{\alpha}+\frac{y^2}{4}=1 \Rightarrow \frac{2 x}{\alpha}+\frac{2 y}{4} \cdot \frac{d y}{d x}=0 \\
& \Rightarrow \frac{d y}{d x}=\frac{-4 x}{\alpha y} \\
& y^3=16 x \\
& \Rightarrow 3 y^2 \frac{d y}{d x}=16 \\
& \Rightarrow \frac{d y}{d x}=\frac{16}{3 y^2}
\end{aligned}
$

Since, the curves intersect at right angles, then

$
\begin{aligned}
& \therefore \frac{-4 x}{\alpha y} \times \frac{16}{3 y^2}=-1 \Rightarrow 3 \alpha y^3=64 x \\
& \Rightarrow \alpha=\frac{64 x}{3 \times 16 x}=\frac{4}{3}
\end{aligned}
$

Hence, the answer is the option (2).

Summary

Angle of intersection is an important concept in mathematics that measures the deviation of the curve at a point. The angle measures can be acute, obtuse, or orthogonal. It is applied in most of the concepts of maths, physics, and chemistry.