Angles in a Pentagon (Interior, Exterior and Central Angle)

We can define a pentagon as a two-dimensional figure that has 5 sides and 5 angles, in which an angle is formed when the two sides of the pentagon share a common point. We can also observe that the number or vertices and angles in a pentagon is 5. In this article we will learn about the concepts of angles in a pentagon, how to find the sum of angles in a pentagon, measure of angles in a pentagon, internal angles in a pentagon, sum of exterior angles in a pentagon, etc.

This Story also Contains

1. Pentagon and its Types
2. Sum of Angles in a Pentagon
3. Angles in a Pentagon Examples

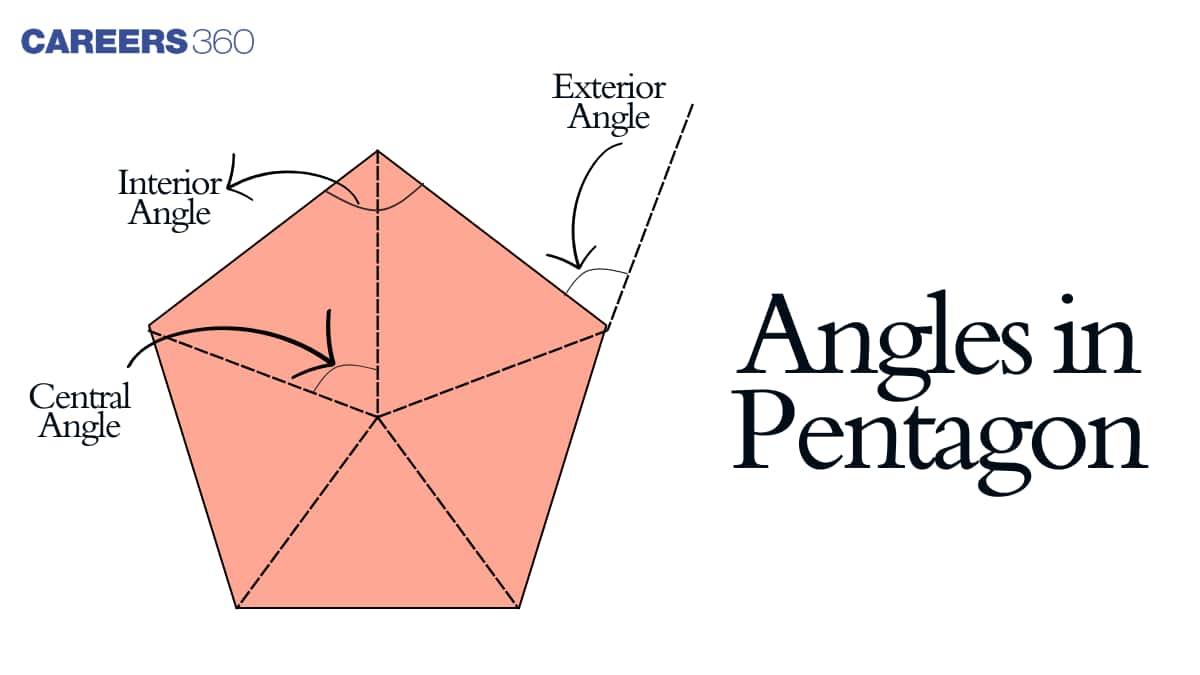
Pentagon and its Types

A pentagon is a five sided two dimensional shape. It has 5 angles.

Now we look at various types of pentagon.

Regular Pentagon: It is a pentagon in which all sides are equal to its interior angles.

Irregular Pentagon: It is the opposite of regular pentagon where the sides and angles are not same.

Convex Pentagon: A pentagon with the vertices pointing always outwards with all interior angles measuring less than 180°.

Concave Pentagon: Concave pentagon is formed when atleast one of the interior angles is greater than 180° with atleast one of the vertices points inward.

Sum of Angles in a Pentagon

Now we will talk about sum of all angles in a pentagon. We will also know how to calculate angles in a pentagon, and number of angles in a pentagon.

Sum of angles in a pentagon formula

We know that a pentagon is formed from three triangles. So, the measure of all angles in a pentagon will be 3 times of measure of angles of a triangle. The measure of angles of a triangle is $180^{\circ}$, hence, the sum of angles in a pentagon $=3 \times 180^{\circ}=540^{\circ}$. Here we have used the angle sum property of a triangle which says that the sum of all interior angles of a triangle is always equal to 180 degrees.

Sum of Interior Angles in a Pentagon

Internal angles in a pentagon can be described as those angles that lie inside the boundary of a pentagon. Now, let us see what is the sum of interior angles in a pentagon?

The measure of each interior angle is given by $=\frac{\left[(n-2) \times 180^{\circ}\right]}{n}=\frac{540^{\circ}}{5}=108^{\circ}(n=$ number of sides)

Sum of Exterior Angles in a Pentagon

We know from our prior knowledge that the formula which helps us to calculate the sum of interior angles of a polygon is $(n-2) \times 180^{\circ}$.

Therefore, we divide the above expression by n(number of interior angles) and so each interior angle $=\frac{\left[(n-2) \times 180^{\circ}\right]}{ n}$.

Hence, we derive each exterior angle $=[180^{\circ} \mathrm{n}-180^{\circ} \mathrm{n}+\frac{360^{\circ}}{\mathrm{n}}=\frac{360^{\circ}}{\mathrm{n}}.$
The sum of exterior angles of a polygon $=\frac{360^{\circ}}{\mathrm{n}}$.
Since we know that the number of sides in a pentagon is 5 , therefore $n=5$.
Hence, the sum of exterior angles of a pentagon $=5\frac{360^{\circ}}{\mathrm{5}}=360^{\circ}$.

Measure of each exterior angle of a pentagon $=\frac{360^{\circ}}{\mathrm{n}}=\frac{360^{\circ}}{\mathrm{5}}=72^{\circ}$. $(n=$ number of sides)

Central Angle of a Pentagon

We know that the measure of the central angle of a regular pentagon makes a circle. When we divide the pentagon into five congruent triangles, then the angle at one vertex of them will be $72^{\circ}(\frac{360^{\circ}}{5} =72^{\circ})$.

Angles in a Pentagon Examples

Example 1: Three angles of a pentagon are $40^{\circ}, 20^{\circ}$ and $120^{\circ}$, now find the other two angles.

Solution: Given three angles are $40^{\circ}, 20^{\circ}$ and $120^{\circ}$.
Sum of three angles $=180^{\circ}$
Sum of the other two angles $=540^{\circ}-180^{\circ}=360^{\circ}$
Now, $180^{\circ}+180^{\circ}=360^{\circ}$
Hence, the other two angles of pentagon are $180^{\circ}$ and $180^{\circ}$.

Example 2: What is the measure of fifth angle of pentagon if 4 of them are 60 degree, 45 degree, 100 degree and 30 degree ?

Solution: Let the unknowm angle be a.
By angle sum property of pentagon, $60+45+100+30+a=540$

$
a=540-235=305
$

Example 3: Find the exterior angle of the following regular pentagon.

Solution: Let that angle by y.

Exterior angle of pentagon is given by $\frac{360}{ n}=\frac{360}{5}=72$ degrees.

Example 4: Angles in a pentagon are as 200 degree, 30 degree, 10 degree, 40 degree. What is the measure of fifth angle ?

Solution: By angle sum property of pentagon, the last angle is given by $540-280=260$ degrees.

Example 5: Are all the angles in a pentagon equal?
Ans: Yes, in a regular pentagon all the angles are equal.

List of Topics Related to Angles in a Pentagon