Area of Hexagon

Edited By Team Careers360 | Updated on Jul 02, 2025 05:19 PM IST

The portion or the region inside the six sides of the hexagon is called the area of the hexagon. A polygon can be defined as the closed 2-D shape with six sides and six angles. The Hexagon word comes from Greek words "Hexa," which means "six," and "Gona," which means "corner,".

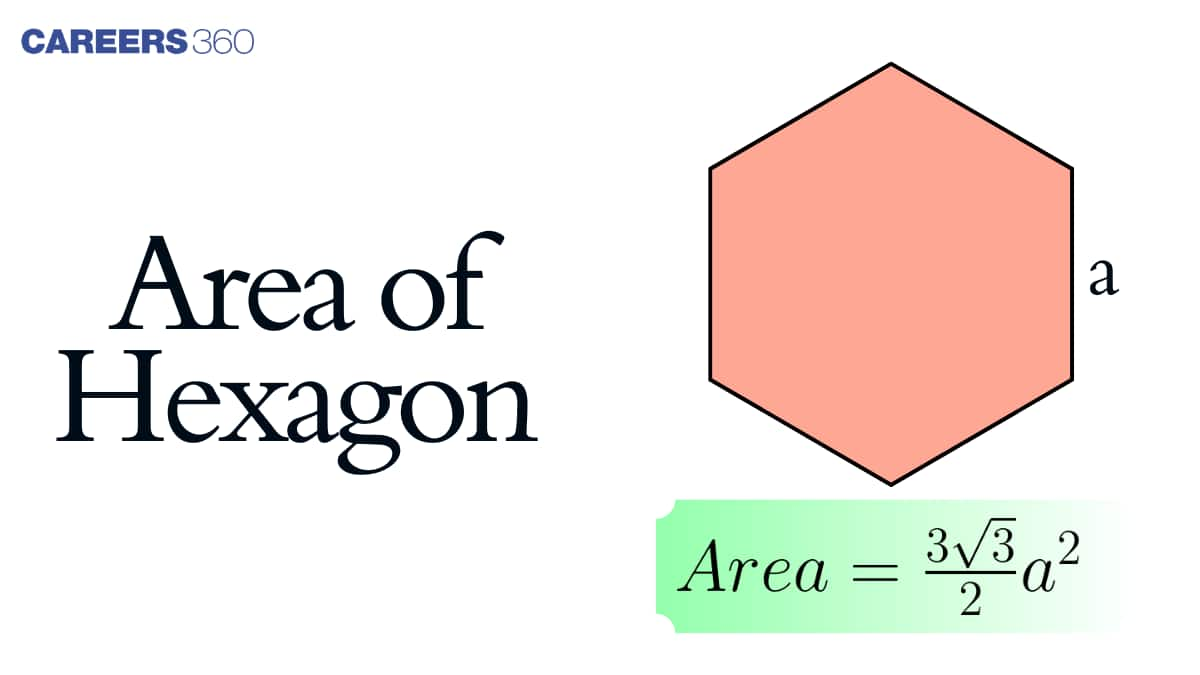
Hexagon

Hexagons are six-sided polygonal shapes. A hexagon is referred to as a regular hexagon if all of its sides are equal and all of its angles are the same. There are a total of 9 diagonals in a hexagon. A normal hexagon has 720 degrees total internal angles. The inner angles are all 120 degrees, as well. The outside angle of a regular hexagon is 60 degrees, while the total exterior angle is 360 degrees.

This Story also Contains

Hexagon
Area Of Hexagon
Area Of Hexagon With Apothem

Area of Hexagon - Definition, Formula, Derivation and Examples

Six equilateral triangles make up a regular hexagon, which has six equal sides and six angles. Whether you're dealing with an irregular hexagon or a regular hexagon, there are numerous ways to figure out the area of a hexagon. The area of the hexagon formula can be calculated in a number of ways. There are several ways to compute a hexagon's area, which is stated in square units like $m^{2}, cm^{2}, in^{2}, ft^{2}$, and so on.

The area of a regular hexagon is calculated using the formula $\frac{3\sqrt{3} a^2}{2}$, where a is the length of a hexagonal side. The formula Area of the Hexagon = $\frac{3\sqrt{3} a^2}{2}$, which is the length of the hexagonal side, can be used to determine the area of a regular hexagon when one of its sides is known.

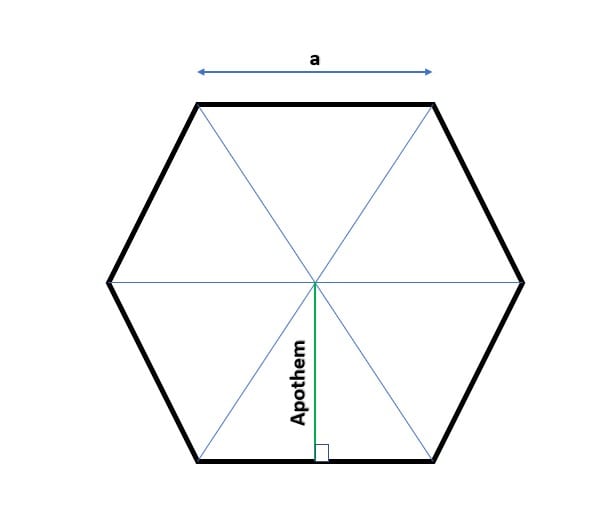
Area Of Hexagon With Apothem

When the side length and the apothem are known, the area of a regular hexagon can be determined. Apothem is the section of line traced from the hexagon's center that is parallel to each hexagonal side.

Area of hexagon = (1/2) × apothem × Perimeter of hexagon.

1706507423206

Frequently Asked Questions (FAQs)

1. What is the Area of Hexagon?

The area of the hexagon is defined as the area enclosed within the sides of the hexagon. The area of a hexagon is expressed in square units like $m^{2}, cm^{2}, in^{2}, ft^{2}$, and so on.

2. What will be the area of a regular hexagon if it has a side length of 8 units?

Given, the length of the side of the regular hexagon = 8 units.

We know that, The formula Area of the Hexagon =$\frac{3\sqrt{3} s^2}{2}$, here s is the side of the hexagon

Area of the Hexagon = $\frac{3\sqrt{3} \times 8^2}{2} =166.27$ sq. units

3. How Can We Find the Irregular Hexagon's Area?

The area of an asymmetric hexagon cannot be calculated using a precise formula. Triangles and rectangles can be used to partition a hexagon into multiple forms. The area of the hexagon can then be computed by adding the areas of these other forms.

4. What is the definition of Polygon?

A polygon is a straightforward closed curve. A polygon is a closed, two-dimensional shape that is circumscribed by three or more straight lines. Polygons include shapes like triangles, squares, rectangles, pentagons, and hexagons.

The sides of the polygon are the individual segments. Vertices are the places where two segments converge. Adjacent sides refer to segments that have the same vertex. A diagonal is a segment whose endpoints are non-adjacent vertices.

5. What is a hexagon and why is its area important in calculus?

A hexagon is a six-sided polygon. Its area is important in calculus, particularly in the application of integrals, because it demonstrates how complex shapes can be broken down into simpler components for calculation. This concept is crucial in understanding how integrals can be used to find areas of irregular shapes.

6. How can you derive the area formula for a regular hexagon using trigonometry?

To derive the area formula, divide the hexagon into six equilateral triangles. The area of each triangle is (√3 * s^2) / 4, where s is the side length. Multiplying this by 6 gives the total hexagon area: 6 * (√3 * s^2) / 4 = (3√3 * s^2) / 2.

7. Can you use calculus to find the area of an irregular hexagon?

Yes, calculus can be used to find the area of an irregular hexagon. You would need to set up an integral that describes the boundary of the hexagon, possibly using piecewise functions for each side, and then integrate to find the enclosed area.

8. What is the difference between inscribed and circumscribed hexagons in relation to a circle?

An inscribed hexagon fits inside a circle with its vertices touching the circle's circumference, while a circumscribed hexagon encloses a circle with its sides tangent to the circle. The areas of these hexagons provide upper and lower bounds for the area of the circle.

9. What is the significance of the hexagon's center in calculating its area?

The center of a regular hexagon is significant because it allows you to divide the hexagon into six congruent equilateral triangles. This division simplifies the area calculation and is the basis for deriving the area formula using trigonometry.

10. How does the area of a hexagon relate to the concept of integration?

The area of a hexagon relates to integration as it can be calculated by dividing the hexagon into triangles and summing their areas. This process mirrors the fundamental concept of integration, where we sum infinitesimal areas to find the total area under a curve.

11. What is the formula for the area of a regular hexagon?

The formula for the area of a regular hexagon is A = (3√3 * s^2) / 2, where s is the length of one side. This formula is derived from the relationship between the side length and the hexagon's dimensions.

12. Why does the hexagon area formula include √3?

The √3 in the formula comes from the trigonometric relationships in an equilateral triangle. Specifically, it arises from the sine of 60°, which is √3/2. This value is crucial in calculating the height of the equilateral triangles that make up the hexagon.

13. How does the area of a hexagon change if you double its side length?

If you double the side length of a hexagon, its area increases by a factor of 4. This is because the area is proportional to the square of the side length (s^2 in the formula). So, doubling s results in 2^2 = 4 times the original area.

14. How does the area of a hexagon compare to the area of a circle with the same perimeter?

A regular hexagon has a larger area than a circle with the same perimeter. This is because the hexagon is the polygon with the largest area for a given perimeter, approaching the area of a circle as the number of sides increases.

15. How can you use integration to find the area of a hexagon rotated around an axis?

To find the area of a hexagon rotated around an axis, you would use the washer or shell method of integration. This involves setting up an integral that represents the volume of the solid formed by the rotation, which is related to the area of the original hexagon.

16. What is the relationship between the area of a hexagon and its inradius?

The area of a regular hexagon can be expressed in terms of its inradius (r) as A = 2√3 * r^2. The inradius is the radius of the inscribed circle, and this relationship demonstrates how the hexagon's area is directly proportional to the square of its inradius.

17. How does the concept of differential forms relate to calculating the area of a hexagon?

Differential forms provide a coordinate-independent approach to integration. When applied to a hexagon, a 2-form can be integrated over the hexagon's surface to calculate its area. This method is particularly useful for hexagons defined on curved surfaces or in higher-dimensional spaces.

18. How does the concept of conformal mapping apply to the area of a hexagon?

Conformal mapping, which preserves angles but not necessarily areas, can be applied to transform a hexagon into other shapes. While the area may change under such transformations, studying how it changes can provide insights into complex analysis and its applications in geometry and physics.

19. What is the significance of the hexagon's dual polyhedron in relation to its area?

The dual polyhedron of a hexagon is a cube. While this relationship is more relevant in 3D geometry, it demonstrates how the hexagon's properties, including its area, relate to higher-dimensional concepts. The surface area of the cube is proportional to the square of the hexagon's side length.

20. How does the concept of differential geometry apply to calculating the area of a hexagon on a curved surface?

In differential geometry, calculating the area of a hexagon on a curved surface involves integrating the area element over the surface. This requires considering the metric tensor of the surface and can lead to results different from those in flat Euclidean space, demonstrating the impact of curvature on area calculations.

21. What is the relationship between the area of a hexagon and its polar moment of inertia?

The polar moment of inertia of a regular hexagon about its center is related to its area and side length. It can be expressed as J = (5/8) * M * s^2, where M is the mass and s is the side length. This relationship shows how area calculations are fundamental to understanding rotational dynamics of shapes.

22. How does the concept of fractals relate to the area of a hexagon?

While a regular hexagon is not a fractal, fractal concepts can be applied by creating hexagonal patterns that repeat at different scales. The area of such a fractal pattern would depend on the iteration level, demonstrating how simple shapes can lead to complex area calculations in fractal geometry.

23. How can you use the method of cylindrical shells to find the volume of a solid formed by rotating a hexagon around an axis?

The method of cylindrical shells can be used to find the volume of a solid formed by rotating a hexagon around an axis by setting up an integral that sums the volumes of thin cylindrical shells. This method demonstrates how 2D area concepts extend to 3D volume calculations in calculus.

24. What is the relationship between the area of a hexagon and its second moment of area?

The second moment of area (also known as the moment of inertia of plane area) for a regular hexagon about an axis through its center is related to its area and side length. It's given by I = (5/144) * s^4, where s is the side length. This relationship is crucial in structural engineering and demonstrates how area concepts extend to more complex geometric properties.

25. What is the relationship between the area of a hexagon and its apothem?

The area of a regular hexagon can be expressed in terms of its apothem (a) and side length (s) as A = 3as. The apothem is the distance from the center to the midpoint of any side, and it forms the height of each of the six triangles that make up the hexagon.

26. How can you use the concept of limits to understand the area of a hexagon?

The concept of limits can be applied to understand the hexagon's area by considering a sequence of regular polygons with increasing numbers of sides. As the number of sides approaches infinity, the polygon's area approaches that of a circle, demonstrating the connection between polygonal and circular areas.

27. How does the area of a hexagon change if you increase the number of sides while keeping the perimeter constant?

As you increase the number of sides while keeping the perimeter constant, the area of the polygon increases. It approaches the area of a circle with that perimeter as the number of sides approaches infinity. This demonstrates the principle of optimization in calculus.

28. Can you use Green's theorem to calculate the area of a hexagon?

Yes, Green's theorem can be used to calculate the area of a hexagon. It relates a line integral around the hexagon's boundary to a double integral over its interior. This method is particularly useful for irregular hexagons or when the hexagon is defined by a set of parametric equations.

29. How does the concept of symmetry simplify the calculation of a hexagon's area?

The symmetry of a regular hexagon allows us to calculate the area of just one-sixth of the hexagon (an equilateral triangle) and multiply by 6. This significantly simplifies the calculation and is why the area formula is derived from the area of an equilateral triangle.

30. What is the relationship between the area of a hexagon and its inscribed circle?

The area of a regular hexagon is always larger than the area of its inscribed circle. The ratio of these areas is constant and equal to 2√3/π, or approximately 1.1027. This relationship demonstrates how polygons approximate circles as the number of sides increases.

31. How can you use the concept of definite integrals to find the area between a hexagon and an inscribed circle?

To find the area between a hexagon and its inscribed circle, you would set up a definite integral that represents the difference between the hexagon's boundary and the circle's equation. This would involve integrating the difference of their radii over the appropriate angular interval.

32. What is the significance of the hexagon in the study of tessellations, and how does this relate to area?

Hexagons are significant in tessellations because they can completely cover a plane without gaps or overlaps. This property is related to their area efficiency – hexagons have the largest area-to-perimeter ratio of any regular polygon that can tessellate. This concept connects geometry, area calculation, and optimization.

33. How does the area of a hexagon change if you increase its internal angles while keeping one side fixed?

As you increase the internal angles of a hexagon while keeping one side fixed, the hexagon becomes more "spread out," and its area increases. This change can be quantified using trigonometric relationships and demonstrates how angle measures affect area in polygons.

34. What is the connection between the area of a hexagon and the concept of packing density?

The area of a hexagon is crucial in understanding packing density because hexagons have the highest packing density of any regular polygon. This means they can cover the largest area with the least amount of perimeter, which is why hexagonal patterns are often found in nature and engineering.

35. How can you use partial derivatives to optimize the area of a hexagon with respect to its dimensions?

Partial derivatives can be used to optimize the area of a hexagon by expressing the area in terms of multiple variables (like side length and angle) and then finding the values of these variables that maximize the area. This process involves setting the partial derivatives to zero and solving the resulting system of equations.

36. What is the relationship between the area of a hexagon and its circumradius?

The area of a regular hexagon can be expressed in terms of its circumradius (R) as A = (3√3 * R^2) / 2. This relationship is derived from the fact that the circumradius is equal to the side length in a regular hexagon, demonstrating the connection between circular and polygonal measurements.

37. How does the concept of limits apply when approximating the area of a circle using inscribed hexagons?

When approximating the area of a circle using inscribed hexagons, we can consider a sequence of hexagons with an increasing number of sides. As the number of sides approaches infinity, the limit of the areas of these hexagons converges to the area of the circle, demonstrating a fundamental concept in calculus.

38. What is the significance of the golden ratio in relation to the area of a hexagon?

While the golden ratio is more commonly associated with pentagons, it has an interesting relationship with hexagons. The ratio of the area of a regular hexagon to the area of an equilateral triangle formed by alternate vertices of the hexagon is equal to the golden ratio. This showcases the interconnectedness of geometric concepts.

39. How can you use the method of exhaustion to approximate the area of an irregular hexagon?

The method of exhaustion, a precursor to modern integration, can be used to approximate the area of an irregular hexagon by inscribing and circumscribing regular polygons with an increasing number of sides. The areas of these polygons provide upper and lower bounds for the hexagon's area, converging to the true value as the number of sides increases.

40. What is the significance of Heron's formula in calculating the area of an irregular hexagon?

Heron's formula, typically used for triangles, can be applied to calculate the area of an irregular hexagon by dividing it into four triangles. The areas of these triangles can be calculated using Heron's formula and then summed to find the total hexagon area. This method is useful when only the side lengths of the hexagon are known.

41. How can you use the concept of vector cross products to find the area of a hexagon in 3D space?

In 3D space, the area of a hexagon can be calculated using vector cross products. By treating the hexagon's sides as vectors, you can compute the cross product of adjacent sides to find the area of each triangular component. The sum of these areas gives the total hexagon area, demonstrating the application of vector calculus in geometry.

42. What is the relationship between the area of a hexagon and its moment of inertia?

The moment of inertia of a hexagon is directly related to its area. For a regular hexagon rotating about its center, the moment of inertia I = (5/12) * M * s^2, where M is the mass and s is the side length. This relationship showcases how area calculations are fundamental to understanding the physical properties of shapes.

43. How can you use the method of finite differences to approximate the area of a hexagon?

The method of finite differences can be used to approximate the area of a hexagon by dividing it into a grid of smaller squares. By counting the squares fully inside the hexagon and estimating partial squares at the boundary, an approximation of the area can be obtained. This method demonstrates numerical techniques in geometry.

44. What is the relationship between the area of a hexagon and its circumference?

For a regular hexagon, the relationship between area (A) and circumference (C) is A = (√3 * C^2) / 24. This relationship demonstrates how the area scales with the square of the circumference, a property shared by all similar shapes.

45. What is the significance of the hexagon's Schläfli symbol in relation to its area?

The Schläfli symbol for a regular hexagon is {6}, indicating a polygon with 6 sides meeting at equal angles. This symbol encapsulates the hexagon's symmetry, which is crucial in deriving its area formula. It also relates the hexagon to other regular polygons and higher-dimensional polytopes.

46. How can you use the concept of Riemann sums to approximate the area of an irregular hexagon?

Riemann sums can be used to approximate the area of an irregular hexagon by dividing it into smaller rectangles or trapezoids. The sum of these areas approximates the hexagon's area, with the approximation improving as the number of divisions increases. This method demonstrates the fundamental concept behind integration.

47. What is the significance of the hexagon's dihedral group in relation to its area?

The dihedral group D6 describes the symmetries of a regular hexagon. These symmetries (6 rotations and 6 reflections) are crucial in simplifying area calculations. Understanding these symmetries allows us to compute the area of just one-twelfth of the hexagon and extend it to the whole, demonstrating the power of group theory in geometry.

48. How does the concept of isoperimetric inequality apply to hexagons?

The isoperimetric inequality states that among all closed curves with a given perimeter, the circle encloses the maximum area. For hexagons, this means that a regular hexagon has the largest area for a given perimeter compared to any other hexagon. This concept demonstrates the optimization principles inherent in geometric shapes.

49. What is the significance of the hexagon's Wythoff symbol in relation to its area?

The Wythoff symbol for a regular hexagon is 3|2 3, which describes how it can be constructed from an equilateral triangle. This construction method is directly related to the hexagon's area calculation, as it shows how the hexagon can be divided into six equilateral triangles, each contributing one-sixth of the total area.