Circumcenter of a Triangle and Its Formula
Finding the circumcenter of a triangle is essential for solving many geometry problems, including locating the center of a circle that passes through all three vertices. Using the circumcenter formula, you can easily calculate its coordinates and apply them in construction, distance measurement, and circle-related questions in mathematics. This article explains the circumcenter of a triangle and its formula in a straightforward, easy-to-follow way.
Circumcentre: Definition
The circumcentre ($O$) of a triangle is the point of intersection of the perpendicular bisectors of the triangle’s sides. A perpendicular bisector of a side is a line that passes through the midpoint of the side and is perpendicular to it.
The circumcentre is also defined as the centre of the circle that passes through all three vertices of the triangle. This circle is called the circumcircle, and the circumcentre is its centre.
Key properties of the circumcentre based on triangle type:
In an acute-angled triangle, the circumcentre lies inside the triangle.
In an obtuse-angled triangle, the circumcentre lies outside the triangle.
In a right-angled triangle, the circumcentre is located at the midpoint of the hypotenuse.
Thus, the circumcentre is the point where all perpendicular bisectors of a triangle are concurrent and serves as the centre of the circumcircle.
Circumcentre of a Triangle: Formula
Let $A$, $B$, and $C$ be the vertices of triangle $ABC$ with coordinates $A(x_1, y_1)$, $B(x_2, y_2)$, and $C(x_3, y_3)$.
Then, the coordinates of the circumcentre ($O$) are given by: $(x_c, y_c) = \left( \frac{x_1 \sin 2A + x_2 \sin 2B + x_3 \sin 2C}{\sin 2A + \sin 2B + \sin 2C}, \frac{y_1 \sin 2A + y_2 \sin 2B + y_3 \sin 2C}{\sin 2A + \sin 2B + \sin 2C} \right)$
Note: For a right-angled triangle, the circumcentre is the midpoint of the hypotenuse, and the coordinates simplify to:
$(x_c, y_c) = \left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2} \right)$
Properties of Circumcircle and Circumcentre
The perpendicular from the center of a circle to any chord bisects the chord. Therefore, the circumcentre of a triangle is the point of concurrency of the perpendicular bisectors of its sides.
The distance of the circumcentre from all three vertices of the triangle is the same. This distance is called the circumradius ($R$):
$OA = OB = OC = R$The angle subtended by a chord at the center of the circumcircle is twice the angle subtended by the same chord at any point on the circumference:
$\angle AOB = 2 \times \angle ACB$The distance of the circumcentre from the sides of the triangle can be expressed using the circumradius. For example, if $O$ is the circumcentre and $E$ and $F$ are points on sides of the triangle:
$OE = R \cos B, \quad OF = R \cos C$In an acute-angled triangle, the circumcentre lies inside the triangle.
In an obtuse-angled triangle, the circumcentre lies outside the triangle.
In a right-angled triangle, the circumcentre is located at the midpoint of the hypotenuse.
Circumcenter in Special Triangles
The circumcenter’s location changes based on the type of triangle, which affects how we calculate it and its applications in geometry. Knowing its position helps in constructions, finding circumcircle radius, and solving coordinate geometry problems.
Circumcenter of an Equilateral Triangle
In an equilateral triangle, all sides are equal, and all angles are $60^\circ$. The circumcenter coincides with the centroid and incenter. It lies exactly at the center of the triangle, making construction and distance calculations straightforward.
Coordinates of the circumcenter in an equilateral triangle with vertices $A(x_1, y_1)$, $B(x_2, y_2)$, $C(x_3, y_3)$ are:
$(x_c, y_c) = \left( \frac{x_1 + x_2 + x_3}{3}, \frac{y_1 + y_2 + y_3}{3} \right)$
The circumradius $R$ is calculated as: $R = \frac{a}{\sqrt{3}}$
where $a$ is the length of a side.
This makes equilateral triangles simple to work with in geometry problems and competitive exam questions.
Circumcenter of a Right-Angled Triangle
For a right-angled triangle, the circumcenter always lies at the midpoint of the hypotenuse. This is because the hypotenuse acts as the diameter of the circumcircle.
If the hypotenuse has endpoints $A(x_1, y_1)$ and $B(x_2, y_2)$, the circumcenter coordinates are:
$(x_c, y_c) = \left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2} \right)$
The circumradius is: $R = \frac{\text{Hypotenuse}}{2}$
This property simplifies calculations and is particularly useful for geometry problems involving right triangles and circle constructions.
Circumcenter of an Isosceles and Scalene Triangle
In an isosceles triangle, the circumcenter lies on the axis of symmetry. In scalene triangles, it can be inside or outside the triangle depending on the angles. For any triangle with vertices $A(x_1, y_1)$, $B(x_2, y_2)$, $C(x_3, y_3)$, the coordinates of the circumcenter are given by:
$x_c = \frac{(x_1^2 + y_1^2)(y_2 - y_3) + (x_2^2 + y_2^2)(y_3 - y_1) + (x_3^2 + y_3^2)(y_1 - y_2)}{2 \left( x_1(y_2 - y_3) - y_1(x_2 - x_3) + x_2 y_3 - x_3 y_2 \right)}$
$y_c = \frac{(x_1^2 + y_1^2)(x_3 - x_2) + (x_2^2 + y_2^2)(x_1 - x_3) + (x_3^2 + y_3^2)(x_2 - x_1)}{2 \left( x_1(y_2 - y_3) - y_1(x_2 - x_3) + x_2 y_3 - x_3 y_2 \right)}$
This formula works for both isosceles and scalene triangles and is essential in coordinate geometry and competitive exams for accurately finding the circumcenter.
How to Construct a Circumcenter Geometrically
Constructing the circumcenter accurately is important for geometric problems, triangle constructions, and creating circumcircles in real-life applications.
Using Compass and Straightedge
Draw the perpendicular bisector of at least two sides of the triangle using a compass and straightedge.
The intersection of these perpendicular bisectors is the circumcenter:
(xc,yc)(x_c, y_c)(xc,yc)
This method is fundamental in manual geometry constructions and helps visualize the circumcenter in any triangle.
Perpendicular Bisector Method
For a triangle with vertices $A(x_1, y_1)$, $B(x_2, y_2)$, $C(x_3, y_3)$:
Find the midpoints of sides:
$M_{AB} = \left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2} \right), \quad M_{BC} = \left( \frac{x_2 + x_3}{2}, \frac{y_2 + y_3}{2} \right)$
Draw perpendicular lines at these midpoints.
Solve for their intersection to get the circumcenter coordinates: $(x_c, y_c)$
This method is widely used in coordinate geometry and ensures accurate circumcircle construction for any triangle type.
Solved Examples Based on Circumcentre
Example 1: Let $\mathrm{C}(\alpha, \beta)$ be the circumcenter of the triangle formed by the lines
$\begin{aligned} & 4 x+3 y=69 \\ & 4 y-3 x=17 \text { and } \\ & x+7 y=61\end{aligned}$
Then $(\alpha-\beta)^2+\alpha+\beta$ is equal to [JEE MAINS 2023]
Solution:
$25y = 175$
$y = 7, \quad x = 12$
$A(12, 7)$
$-3x + 4y = 17$
$3x + 21y = 183$
$25y = 200$
$y = 8, \quad x = 5$
$B(5, 8)$
$\therefore \text{Circumcenter}$
$\alpha = \frac{17}{2}, \quad \beta = \frac{15}{2}$
$\left(\frac{17}{2}, \frac{15}{2}\right)$
$(\alpha - \beta)^2 + \alpha + \beta$
$1 + 16 = 17$
Hence, the answer is $17$.
Example 2: If the points $P$ and $Q$ are respectively the circumcenter and the orthocentre of a $\triangle \mathrm{ABC}$, the $\overrightarrow{\mathrm{PA}}+\overrightarrow{\mathrm{PB}}+\overrightarrow{\mathrm{PC}}$ is equal to [JEE MAINS 2023]
Solution:
$\begin{aligned} & \overrightarrow{\mathrm{PA}}+\overrightarrow{\mathrm{PB}}+\overrightarrow{\mathrm{PC}}=\vec{a}+\vec{b}+\vec{c} \\ & \overrightarrow{\mathrm{PG}}=\frac{\overrightarrow{\mathrm{a}}+\overrightarrow{\mathrm{b}}+\vec{c}}{3} \\ & \Rightarrow \overrightarrow{\mathrm{a}}+\overrightarrow{\mathrm{b}}+\overrightarrow{\mathrm{c}}=3 \overrightarrow{\mathrm{PG}}=\overrightarrow{\mathrm{PQ}}\end{aligned}$
Hence, the answer is $\overrightarrow{P Q}$.
Solution:
Trigonometric Ratios of Functions -
$\sin \Theta = \frac{\text{Opp}}{\text{Hyp}}$
$\cos \Theta = \frac{\text{Base}}{\text{Hyp}}$
$\tan \Theta = \frac{\text{Opp}}{\text{Base}}$
wherein
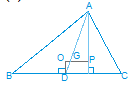
From figure, we can observe that $\triangle O G D$ is directly similar to $\triangle P G A$.
List of Topics Related to the Circumcenter of a triangle
Explore key topics connected to the circumcenter of a triangle, including formulas, properties, solved examples, and important concepts that help in mastering coordinate geometry and competitive exam preparation.
NCERT Resources
Access comprehensive NCERT resources for Chapter 10 – Straight Lines, including Class 11 Maths notes, solutions, and exemplar problems. These materials help students understand concepts, practice effectively, and excel in exams.
NCERT Class 11 Maths Notes for Chapter 10 - Straight Lines
NCERT Class 11 Maths Solutions for Chapter 10 - Straight Lines
NCERT Class 11 Maths Exemplar Solutions for Chapter 10 - Straight Lines
Practice Questions based on the Circumcenter of a triangle
Solve these carefully designed practice questions on the circumcenter of a triangle to strengthen your understanding of formulas, properties, and construction methods. These circumcenter problems will help you master geometry concepts and improve exam preparation.
Circumcircle Of A Triangle - Practice Question MCQ
You can practice the related questions from the links shared below:
Frequently Asked Questions (FAQs)
The intersection of the perpendicular bisectors of the three sides is called circumcentre. The distance of the circumcentre from all the vertices of the triangle is the same which is equal to the circumradius of the triangle.
The circle passing through the vertices of a triangle is called a circumcircle. The circumcircle of triangle ABC is the unique circle passing through the three vertices A, B, and C.
In a triangle other than the equilateral triangle, the orthocentre (H), centroid (G), and circumcentre (0) are collinear with a ratio HG: G O = 2: 1
The circle passing through the vertices of a triangle is called a circumcircle. The circumcentre of the obtuse-angled triangle lies outside the triangle.