Derivatives of Inverse Function

Differentiation is one of the important parts of Calculus, which applies to measuring the change in the function at a certain point. Mathematically, it forms a powerful tool by which slopes of functions are determined, the maximum and minimum of functions found, and problems on motion, growth, and decay, to name a few. These concepts of differentiation have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Inverse Function
2. Differentiation of Inverse Function
3. Solved Examples Based on the Derivative of Inverse Functions
4. Summary

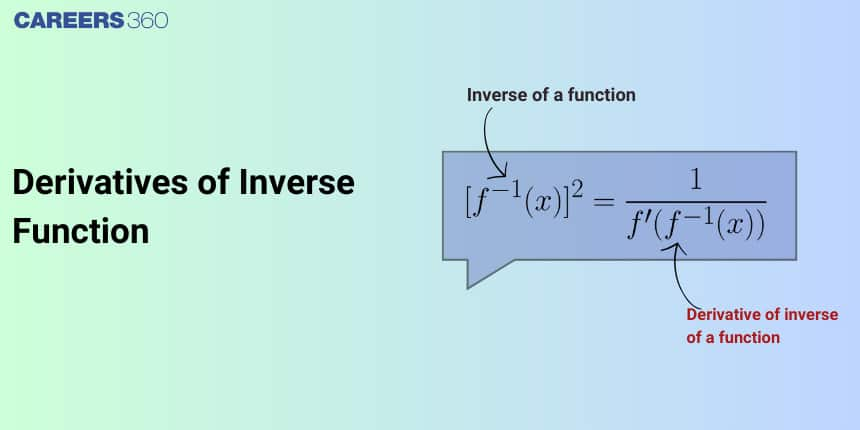
In this article, we will cover the concepts of the inverse of a function. This concept falls under the broader category of sets relation and function, a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of five questions have been asked on this concept, including one in 2013, two in 2014, one in 2019, and one in 2022.

Inverse Function

What is an Inverse Function: An inverse function or an anti-function is defined as a function, which can reverse into another function. The inverse of a function reverses the operation of the function, mapping outputs back to their corresponding inputs. If a function $f$ maps $x$ to $y$, the inverse function, denoted $f^{-1}$, maps $y$ back to $x$. We know that the inverse of a function is defined when the function is one-one and onto.

Function $f: X \rightarrow Y$ is an invertible function if it is one-one and onto
Also, its inverse g is defined in the following way
$g: Y \rightarrow X$ such that if $f(a)=b$, then $g(b)=a$
The function $g$ is called the inverse of $f$ and is denoted by $f^{-1}$.
Let us consider a one-one and onto function f with domain A and co-domain B . Where, $\mathrm{A}=$ $\{1,2,3,4\}$ and $B=\{2,4,6,8\}$ and $f: A \rightarrow B$ is given $f(x)=2 x$, then write $f$ and $f^{-1}$ as a set of ordered pairs.

So, $f=\{(1,2)(2,4)(3,6)(4,8)\}$
And $f^{-1}=\{(2,1)(4,2)(6,3)(8,4)\}$

In above definition domain of $f=\{1,2,3,4\}=$ range of $f^{-1}$
Range of $f=\{2,4,6,8\}=$ domain of $f^{-1}$.
Steps to find the inverse of a function:
i) First we write $f(x)$ as $y$ and equate $y=f(x)$, where $f(x)$ is a function in $x$
ii) Then we separate the variable $x$ as the dependent variable and express it in terms of $y$ by assuming $y$ as the independent variable
iii) Then we write $g(y)=x$ where $g(y)$ is a function in $y$
iv) And finally, we replace every $y$ with $x$.

Properties of an inverse function
i) The inverse of a bijection is unique.
ii) if $\mathrm{f}: \mathrm{A} \rightarrow \mathrm{B}$ is a bijection and $\mathrm{g}: \mathrm{B} \rightarrow \mathrm{A}$ is the inverse of f , then $f \circ g=I_B$ and $g \circ f=I_A$, where $\mathrm{I}_A$ and $\mathrm{I}_B$ are identity functions on the sets $A$ and $B$, respectively.
iii) The inverse of a bijection is also a bijection.
iv) If $\mathrm{A} \rightarrow \mathrm{B}$ and $\mathrm{g}: \mathrm{B} \rightarrow \mathrm{C}$ are two bijections, then (got) ${ }^{-1}=\mathrm{f}^{-1} \mathrm{og}^{-1}$
v) The graphs of $f$ and its inverse function, are mirror images of each other in the line $y=x$.

Differentiation of Inverse Function

Let $f(x)$ be a function that is both invertible and differentiable. Let $y=g(x)$ be the inverse of $f(x)$. Then,

$
f(g(x))=x \quad \text { (Property of inverse function) }
$
Differentiating both sides

$
f^{\prime}(g(x)) \cdot g^{\prime}(x)=1
$

(Chain Rule)

$
g^{\prime}(x)=\frac{1}{f^{\prime}(g(x))}
$

Illustration

If $f(x)=x^3+x^5$, and $g(x)$ is the inverse of $f(x)$, then find $g^{\prime}(2)$
Solution
Using $g^{\prime}(x)=\frac{1}{f^{\prime}(g(x))}$, put $\mathrm{x}=2$

$
g^{\prime}(2)=\frac{1}{f^{\prime}(g(2))}
$
Now we need to get the value of $g(2)$
As we know for inverse functions if $f(a)=b$, then $g(b)=a$. So let $g(2)=p$, then $f(p)=2$

$
\begin{aligned}
& p^3+p^5=2 \\
& p=1=g(2)
\end{aligned}
$
Putting this in (i)

$
g^{\prime}(2)=\frac{1}{f^{\prime}(1)}=\frac{1}{f^{\prime}(1)}=\frac{1}{3 x^2+5 x^4}(\text { at } x=1)=\frac{1}{8}
$

Recommended Video Based on the Derivative of Inverse Functions

Solved Examples Based on the Derivative of Inverse Functions

Example 1: If $f(x)=x^2-x+5, x>\frac{1}{2}$ and $g(x)$ is its inverse function,then $g^{\prime}(7)$ equals:
1) $-\frac{1}{3}$
2) $\frac{1}{13}$
3) $\frac{1}{3}$
4) $-\frac{1}{13}$

Solution:
Given that, $g(x)$ is inverse of $f(x)$

$
g(f(x))=x
$

differentiate both side

$
g^{\prime}(f(x)) \cdot f^{\prime}(x)=1
$

we need to find $g^{\prime}(7)$, so $f(x)=7$
now,

$
f(x)=x^2-x+5=7
$

$x=-1$ or $x=2$
since, $x>\frac{1}{2}$, so, $x=2$

$
\begin{aligned}
& f^{\prime}(x)=2 x-1 \\
& g^{\prime}(7) \cdot f^{\prime}(x)=1 \\
& g^{\prime}(7)=\frac{1}{2 x-1}
\end{aligned}
$

put $x=2$

$
g^{\prime}(7)=\frac{1}{3}
$

Hence, the answer is the option 3.

Example 2: Let $f(x)=\frac{x^2-x}{x^2+2 x}, x \neq 0,-2$. Then $\frac{d}{d x}\left[f^{-1}(x)\right]_{\text {(wherever it is defined ) }}$ is equal to :
1) $\frac{-1}{(1-x)^2}$
2) $\frac{3}{(1-x)^2}$
3) $\frac{1}{(1-x)^2}$
4) $\frac{-3}{(1-x)^2}$

Solution:

$\begin{aligned} & \text { Let } y=\frac{x^2-x}{x^2+2 x} \\ & \Rightarrow \quad\left(x^2+2 x\right) y=x^2-x \\ & \Rightarrow \quad x(x+2) y=x(x-1) \\ & \Rightarrow x[(x+2) y-(x-1)]=0 \\ & \because \quad x \neq 0, \therefore \quad \therefore(x+2) y-(x-1)=0 \\ & \Rightarrow x y+2 y-x+1=0 \\ & \Rightarrow x(y-1)=-(2 y+1) \\ & \therefore \quad x=\frac{2 y+1}{1-y} \Rightarrow f^{-1}(x)=\frac{2 x+1}{1-x} \\ & \frac{d}{d x}\left(f^{-1}(x)\right)=\frac{2(1-x)-(2 x+1)(-1)}{(1-x)^2} \\ & =\frac{2-2 x+2 x+1}{(1-x)^2}=\frac{3}{(1-x)^2}\end{aligned}$

Hence, the answer is option 2.

Example 3: Let $f(x)=\log _e(\sin x),(0<x<\pi)$ and $g(x)=\sin ^{-1}\left(e^{-x}\right),(x \geq 0)$. If $\alpha$ is a positive real number such that $a=(f \circ g)^{\prime}(\alpha)$ and $b=(f \circ g)(\alpha)$, then:
1) $a \alpha^2+b \alpha+a=0$
2) $a \alpha^2-b \alpha-a=1$
3) $a \alpha^2-b \alpha-a=0$
4) $a \alpha^2+b \alpha-a=-2 \alpha^2$

Solution:

$
\begin{aligned}
& \frac{d}{d x}\left(x^n\right)=n x^{n-1} \\
& \frac{d}{d x}\left(\frac{1}{f(x)}\right)^n=\frac{-n}{(f(x))^{n+1}} \cdot \frac{d}{d x} f(x) \ldots . . \text { and so on } \\
& f(x)=\log _{\mathrm{e}}(\sin x),(0<x<\pi) \text { and } \\
& g(x)=\sin ^{-1}\left(e^{-x}\right),(x \geq 0) . \\
& f(g(x))=f(g(x))=\log _e\left(\sin \left(\sin ^{-1}\left(e^{-x}\right)\right)\right) \\
& =\log _e\left(e^{-x}\right) \\
& =(-x)
\end{aligned}
$
$
f(g(x))^{\prime}=-1
$
Now,

$
f(g(\alpha))=-\alpha=b
$

and $f(g(x))^{\prime}$ at $x=\alpha$ is $-1=a$

Now, let's check every option
(1) $a \alpha^2+b \alpha+a=0$
put the value in LHS

$
(-1) \cdot(-b)^2+b \cdot(-b)+(-1)=-b^2-b^2-1 \neq 0
$

(2) $a \alpha^2-b \alpha-a=1$
put the value in LHS

$
(-1) \cdot(-b)^2-b \cdot(-b)-(-1)=-b^2+b^2+1
$

(3) $a \alpha^2-b \alpha-a=0$
put the value in LHS

$
(-1) \cdot(-b)^2-b \cdot(-b)-(-1)=-b^2+b^2+1=1 \neq 0
$

(4) $a \alpha^2+b \alpha-a=-2 \alpha^2$
put the value in LHS

$
(-1) \cdot(-b)^2+b \cdot(-b)-(-1)=-2 b^2+1 \neq-2 \alpha^2
$
So, option (2) is correct as $a \alpha^2-b \alpha-a=1$ is true

Hence, the answer is the option (2).

Example 4: Let $f: \mathbf{R} \rightarrow \mathbf{R}_{\text {be defined as }} f(x)=x^3+x-5$ If $g(x)$ is a function such that $f(g(x))=x, \quad \forall^{\prime} x^{\prime} \in \mathbf{R}$, then $g^{\prime}(63)$ is equal to $\qquad$ .
1) $\frac{1}{49}$
2) $\frac{3}{49}$
3) $\frac{43}{49}$
4) $\frac{91}{49}$

Solution:

Here $g(f(x))=x$ means $f(x)$ and $g(x)$ are inverse of each other.
Now, $g^{\prime}(f(x)) f^{\prime}(x)=1$.

$
\Rightarrow g^{\prime}(f(x))=\frac{1}{f^{\prime}(x)}----(1)
$
Now $f(x)=63 \Rightarrow x^3+x-5=63$.

$
\Rightarrow x^3+x-68=0
$
So $\mathrm{x}=4$ Satisfies the above eqn

$
\begin{aligned}
g^{\prime}(63) & =\frac{1}{f^{\prime}(4)} \text { from eq }{ }^n-(1) \\
& =\frac{1}{3(4)^2+1}=\frac{1}{49}
\end{aligned}
$

Hence, the answer is the option 1.

Example 5: If $f(x)=x+\tan x$ and $g(x)$ is the inverse of $f(x)$, then $g^{\prime}(x)$ is equal to
1) $\frac{1}{1+(g(x)-x)^2}$
2) $\frac{1}{2+(g(x)-x)^2}$
3) $\frac{1}{2+(g(x)-x)^2}$
4) None of these

Solution:

INVERTIBLE FUNCTION -
A function $\mathrm{f}: \mathrm{X} \rightarrow \mathrm{Y}$ is defined to be invertible if there exists a function $\mathrm{g}: \mathrm{Y} \rightarrow \mathrm{X}$ such that $g \circ f=I_x$ and $f \circ g=I_y$
wherein
The function $g$ is called the inverse of $f$ and is denoted by $f^{-1}$.
A function $f: X \rightarrow Y$ is invertible if and only if $f$ is a bijective function.

$
\begin{aligned}
& f(x)=x+\tan x \\
& f\left(f^{-1}(x)\right)=f^{-1}(x)+\tan \left(f^{-1}(x)\right) \\
& x=g(x)+\tan (g(x)) \\
& 1=g^{\prime}(x)+\sec ^2(g(x)) g^{\prime}(x) \\
& g^{\prime}(x)=\frac{1}{2+\tan ^2(g(x))} \\
& g^{\prime}(x)=\frac{1}{2+(x-g(x))^2}
\end{aligned}
$

Hence, the answer is the option 3.

Summary

The differentiated part of the function is called the derivative of the function. The inverse of a function reverses the mapping of the original function, provided the function is bijective. Finding the inverse involves expressing the function, solving for the input variable, and interchanging variables. Inverse functions are essential for solving equations, modeling, and many areas of mathematics and applied sciences. Verifying the correctness of inverse functions ensures accurate reversibility of operations.