Double Angle Formulas

The double angle formula is used to convert the trigonometric ratios of double angles into the trigonometric ratios of single angles. The double angle formula can be derived using the Trigonometric ratios formula of compound angles( Putting A=B). The double-angle formulas can be used to derive the reduction formulas, which are formulas we can use to reduce the power of a given expression involving even powers of sine or cosine In real life, we use the double-angle formula to simplify the trigonometric expressions by converting double angle to single angle.

JEE Main: Study Materials | High Scoring Topics | Preparation Guide

JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs

In this article, we will cover the concept of Double Angle Formula. This category falls under the broader category of Trigonometry, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

Double Angle Formula

The double angle formula is used to transform the trigonometric ratios of double angles into the trigonometric ratios of single angles. The double angle formulas can be derived from the sum formulas where both angles are equal.

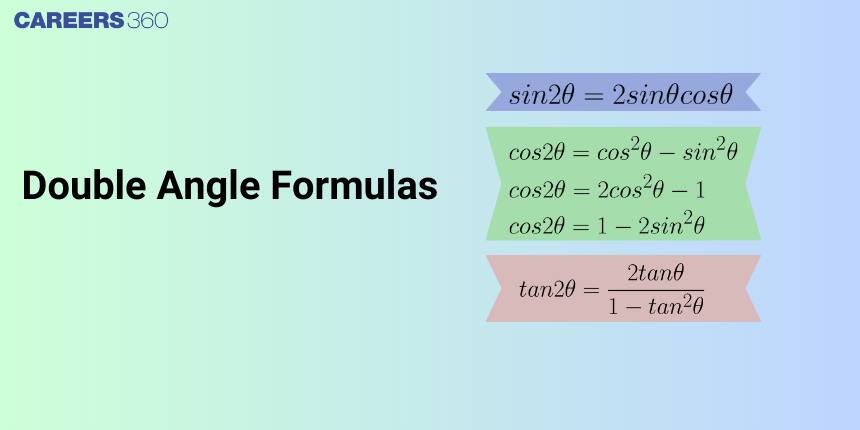
The formulas

$\begin{aligned} 1. \sin (2 \theta) & =2 \sin \theta \cos \theta \\ & =\frac{2 \tan \theta}{1+\tan ^2 \theta} \\ 2. \cos (2 \theta) & =\cos ^2 \theta-\sin ^2 \theta \\ & =1-2 \sin ^2 \theta \\ & =2 \cos ^2 \theta-1 \\ & =\frac{1-\tan ^2 \theta}{1+\tan ^2 \theta} \\ 3. \tan (2 \theta) & =\frac{2 \tan \theta}{1-\tan ^2 \theta}\end{aligned}$

Proof of Double Angle Formula

The double-angle formulas are a special case of the sum formulas, where α = β.

Double Angle Formula For sine

For deriving the double angle formula of sine we use the sum formula of sine functions i.e,

$
\sin (\alpha+\beta)=\sin \alpha \cos \beta+\cos \alpha \sin \beta
$
If we let $\alpha=\beta=\theta$, then we have

$
\begin{aligned}
& \sin (\theta+\theta)=\sin \theta \cos \theta+\cos \theta \sin \theta \\
& \sin (2 \theta)=2 \sin \theta \cos \theta
\end{aligned}
$

Double Angle Formula For cosine

For deriving the double angle formula of cosine we use the sum formula of cosine functions i.e,

$
\cos (\alpha+\beta)=\cos \alpha \cos \beta-\sin \alpha \sin \beta
$
Letting $\alpha=\beta=\theta$, we have

$
\begin{aligned}
& \cos (\theta+\theta)=\cos \theta \cos \theta-\sin \theta \sin \theta \\
& \cos (2 \theta)=\cos ^2 \theta-\sin ^2 \theta
\end{aligned}
$

We can write this formula in different forms as per the requirement of the question,

$
\begin{aligned}
\cos (2 \theta) & =\cos ^2 \theta-\sin ^2 \theta \\
& =\left(1-\sin ^2 \theta\right)-\sin ^2 \theta \\
& =1-2 \sin ^2 \theta
\end{aligned}
$
The second variation is:

$
\begin{aligned}
\cos (2 \theta) & =\cos ^2 \theta-\sin ^2 \theta \\
& =\cos ^2 \theta-\left(1-\cos ^2 \theta\right) \\
& =2 \cos ^2 \theta-1
\end{aligned}
$

Double Angle Formula For tan

For deriving the double angle formula of $\tan$ we use the sum formula of $\tan$ functions i.e,
Replacing $\alpha=\beta=\theta$ in the sum formula gives

$
\begin{aligned}
\tan (\alpha+\beta) & =\frac{\tan \alpha+\tan \beta}{1-\tan \alpha \tan \beta} \\
\tan (\theta+\theta) & =\frac{\tan \theta+\tan \theta}{1-\tan \theta \tan \theta} \\
\tan (2 \theta) & =\frac{2 \tan \theta}{1-\tan ^2 \theta}
\end{aligned}
$

Reduction Formula

The double-angle formulas can be used to derive the reduction formulas, which are formulas we can use to reduce the power of a given expression involving even powers of sine or cosine.

$\begin{aligned} & \sin ^2 \theta=\frac{1-\cos (2 \theta)}{2} \\ & \cos ^2 \theta=\frac{1+\cos (2 \theta)}{2} \\ & \tan ^2 \theta=\frac{1-\cos (2 \theta)}{1+\cos (2 \theta)}\end{aligned}$

Recommended Video Based on Double Angle Formulas

Solved Example Based on Double Angle Formulas

Example 1:
$2 \sin \left(\frac{\pi}{22}\right) \sin \left(\frac{3 \pi}{22}\right) \sin \left(\frac{5 \pi}{22}\right) \sin \left(\frac{7 \pi}{22}\right) \sin \left(\frac{9 \pi}{22}\right)$ is equal to: [JEE MAINS 2022]
Solution: Given expression can be converted to cosine terms:

$
\begin{aligned}
& =2 \cos \left(\frac{10 \pi}{22}\right) \cos \left(\frac{8 \pi}{22}\right) \cos \left(\frac{6 \pi}{22}\right) \cos \left(\frac{4 \pi}{22}\right) \cdot \cos \left(\frac{2 \pi}{22}\right) \\
& =2 \cos \left(\frac{\pi}{11}\right) \cdot \cos \left(\frac{2 \pi}{11}\right) \cdot \cos \left(\frac{3 \pi}{11}\right) \cdot \cos \left(\frac{4 \pi}{11}\right) \cdot \cos \left(\frac{5 \pi}{11}\right) \\
& =2 \cos \left(\frac{\pi}{11}\right) \cdot \cos \left(\frac{2 \pi}{11}\right) \cdot \cos \left(\frac{4 \pi}{11}\right) \cdot\left[-\cos \left(\pi-\frac{3 \pi}{11}\right)\right] \cdot\left[-\cos \left(\pi+\frac{5 \pi}{11}\right)\right] \\
& =2 \cos \left(\frac{\pi}{11}\right) \cdot \cos \left(\frac{2 \pi}{11}\right) \cdot \cos \left(\frac{4 \pi}{11}\right) \cdot \cos \left(\frac{8 \pi}{11}\right) \cdot \cos \left(\frac{16 \pi}{11}\right) \\
& =\frac{2 \cdot \sin \left(\frac{32 \pi}{11}\right)}{2^5 \cdot \sin \left(\frac{32 \pi}{11}\right)} \\
& =\frac{1}{16}
\end{aligned}
$

Hence, the answer is $1 / 16$

Example 2: If $\frac{\sin ^{-1} x}{a}=\frac{\cos ^{-1} x}{b}=\frac{\tan ^{-1} y}{c} ; 0<x<1$, then the value of $\cos \left(\frac{\pi c}{a+b}\right)$ is :
[JEE MAINS 2021]
Solution
Let $\frac{\sin ^{-1} x}{a}=\frac{\cos ^{-1} x}{b}=\frac{\tan ^{-1} y}{c}=r$
So,
$\frac{\sin ^{-1} x}{r}=a, \frac{\cos ^{-1} x}{r}=b, \frac{\tan ^{-1} y}{r}=c$
$a+b=\frac{1}{r}\left(\sin ^{-1}+\cos ^{-1} x\right)=\frac{\pi}{2 r}$
$\cos \left(\frac{\pi \mathrm{c}}{\mathrm{a}+\mathrm{b}}\right)=\cos \left(\frac{\pi \tan ^{-1} \mathrm{y}}{\frac{\pi}{2 \mathrm{r}} \mathrm{r}}\right)$
$=\cos \left(2 \tan ^{-1} \mathrm{y}\right)$
let $\tan ^{-1} y=\theta$

$
\begin{aligned}
& =\cos (2 \theta) \\
& =\frac{1-\tan ^2 \theta}{1+\tan ^2 \theta}=\frac{1-\mathrm{y}^2}{1+\mathrm{y}^2}
\end{aligned}
$
Hence, the answer is $\frac{1-y^2}{1+y^2}$

Example 3: If $\sin \theta+\cos \theta=\frac{1}{2}$, then $16(\sin (2 \theta)+\cos (4 \theta)+\sin (6 \theta))$ is equal to: [JEE MAINS 2021]

Solution: $\sin \theta+\cos \theta=\frac{1}{2}$.
Squaring both the sides, we get:

$
\begin{aligned}
& \sin ^2 \theta+\cos ^2 \theta+2 \sin \theta \cos \theta=\frac{1}{4} \\
& \Rightarrow \sin 2 \theta=-\frac{3}{4}
\end{aligned}
$
Now $\quad \cos 4 \theta=1-2 \sin ^2 2 \theta$.

$
\begin{aligned}
& \Rightarrow \cos 4 \theta=1-2\left(\frac{9}{16}\right)=-\frac{1}{8} \\
& \text { and } \begin{aligned}
\sin 6 \theta & =3 \sin 2 \theta-4 \sin ^3(2 \theta) \\
& =3 \cdot\left(-\frac{3}{4}\right)-4\left(\frac{-3}{4}\right)^3 \\
& =\left(\frac{-3}{4}\right)\left[3-\frac{4 \cdot 9}{16}\right] \\
& =-\frac{9}{16}
\end{aligned}
\end{aligned}
$
$
\begin{aligned}
& \therefore \quad 16(\sin 2 \theta+\cos 4 \theta+\sin 6 \theta) \\
& =16\left(\frac{-3}{4}-\frac{1}{8}-\frac{9}{16}\right)=-23
\end{aligned}
$

Hence, the answer is -23

Example 4: If $
L=\sin ^2\left(\frac{\pi}{16}\right)-\sin ^2\left(\frac{\pi}{8}\right)
$ and $\qquad$, then: [JEE MAINS 2020]
Solution

$
\begin{aligned}
L & =\sin ^2\left(\frac{\pi}{16}\right)-\sin ^2\left(\frac{\pi}{8}\right) \\
& =\frac{1-\cos (x / 8)}{2}-\left(\frac{1-\cos (\pi / 4)}{2}\right) \\
& =\frac{\cos \pi / 4-\cos (x / 8)}{2}-\frac{1}{2} \\
& =\frac{1}{26}-\frac{1}{3} \cos (x / 8)
\end{aligned}
$
$
\begin{aligned}
M & =\cos ^2(\pi / 16)-\sin ^2(\pi / 8) \\
& =\left(\frac{\cos (x / 8)+1)}{2}\right)-\left(\frac{1-\cos (\pi / 4)}{2}\right) \\
& =\frac{1}{2} \cos (\pi / 8)+\frac{1}{2 \sqrt{2}}
\end{aligned}
$

Hence, the answer is $
M=\frac{1}{2 \sqrt{2}}+\frac{1}{2} \cos \left(\frac{\pi}{8}\right)
$

Example 5: The number of distinct solutions of the equation, $\log _{\frac{1}{2}}|\sin x|=2-\log _{\frac{1}{2}}|\cos x|$ in the interval $[0,2 \pi]$, is
[JEE MAINS 2020]
Solution

$
\begin{aligned}
& \log _{\frac{1}{2}}(|\sin (x)|)+\log _{\frac{1}{2}}(|\cos (x)|)=2 \\
& \log _{\frac{1}{2}}(|\sin (x)||\cos (x)|)=2 \\
& \log _{\frac{1}{2}}(|\sin (x)||\cos (x)|)=\log _{\frac{1}{2}}\left(\frac{1}{4}\right) \\
& |\sin x||\cos x|=\frac{1}{4} \\
& \sin 2 \theta=\frac{1}{2} \\
& x=\frac{\pi}{12}+\pi n, x=\frac{5 \pi}{12}+\pi n
\end{aligned}
$
The total number of solutions is 8
Hence, the answer is 8