Equal and Equivalent Sets

Sets are a foundational concept in mathematics, central to various fields such as statistics, geometry, and algebra. Roster and Set Builder sets are the basic elemental concepts in mathematics that one could use to explain real-life examples of the said topic. In Mathematics, two of the most basic topics are equality and equivalence of sets having instances that help in analyzing the relation between two or more sets or groups of objects. Equal represents exact value while equivalent represents equal characteristics. So now, what are equal and equivalent sets?

In this article, we will cover the concept of equal and equivalent sets. This concept falls under the broader category of sets relation and function, a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

Set

It is simply a collection of distinct objects, considered as a whole. These objects, called elements or members of the set, can be anything: numbers, people, letters, etc. Sets are particularly useful in defining and working with groups of objects that share common properties.

It is a well-defined collection of distinct objects and it is usually denoted by capital letters A, B, $\mathrm{C}, \mathrm{S}, \mathrm{U}, \mathrm{V} \ldots \ldots$.

What are Equal Sets?

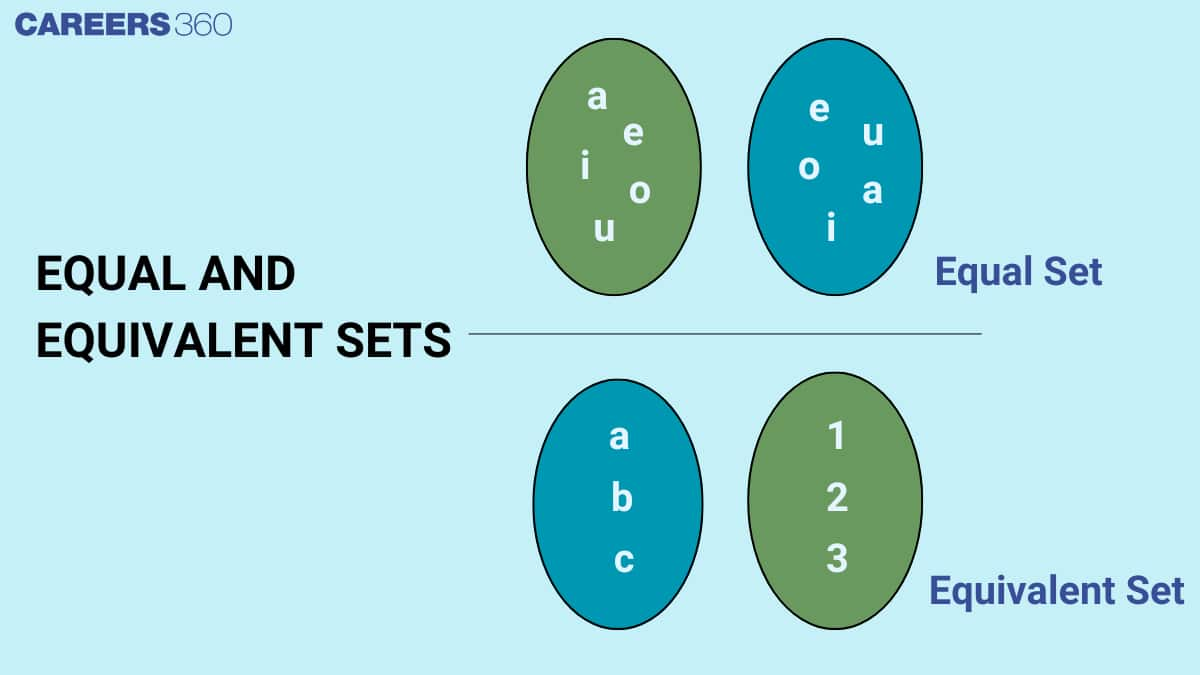
Equal sets are ones that contain the same or equal elements as the other and they suggest that there is a one-to-one relationship in which each member of one of the sets is also a member of the other without duplication or omission of any element.

Two sets $A$ and $B$ are said to be equal if they have exactly the same elements and we write $A=$ B.

Otherwise, the sets are said to be unequal and we write $\mathrm{A} \neq \mathrm{B}$.
Example If $A=\{3,2,1,4\}$ and $B=\{2,3,4,1\}$, then both have exactly the same elements, and hence $\mathrm{A}=\mathrm{B}$.

Properties of Equal Sets

1. The equality of two sets remains unchanged by the order of their elements.
2. Equal sets contain the same number of elements.
3. In equal sets, all elements are equal.
4. The power sets of equal sets also have the same cardinality.
5. Equal and equivalent sets both contain the same number of elements.
6. When two sets are subsets of each other, denoted by $A \subseteq B$ and $B \subseteq A$, the two sets are considered equal.
7. All equal sets are also equivalent, not all equivalent sets are equal.

What are Equivalent Sets?

Two sets having the same number of elements are called equivalent sets.

Form 1: If two sets $A$ and $B$ have the same cardinality if there exists an objective function from set $A$ to $B$.

Form 2: Two sets $A$ and $B$ are equivalent if they have the same cardinality i.e. $n(A)=n(B)$.
Example: $A=\{H, T, P, V\}$ and $B=\{1,2,3,4\}$, they both are equivalent as several elements in both are the same.

Properties of Equivalent Sets

1. The equality of the two sets may or may not be affected by the order of the elements.

2. If the number of elements in two or more sets is the same, then they are equal.

3. The cardinality of equivalent sets is the same.

4. Equivalent sets have the same cardinality.

5. The symbol for similar sets is ~ or ≡.

6. Equivalent sets can be equal or not.

7. Equivalent sets may or may not be equivalent.

Difference between equal and equivalent sets

Cardinal Number

The number of elements in a set is called its cardinal number or cardinality of a set. It is denoted by $n(A)$. If $\mathrm{A}=\{\mathrm{a}, \mathrm{s}, \mathrm{d}\}$, then $n(A)=3$
and if $B=\left\{x: x^2=1\right\}$, then $B=\{1,-1\}$, and hence $n(B)=2$

Note:

• Equivalent sets have the same cardinal number
• Two equivalent sets may or may not be equal, but equal sets are always equivalent.

Recommended Video on Equal and Equivalence Sets

Solved Examples Based on Equal and Equivalent Sets

Example 1: Which of the following are equal sets?
1) $A=\{1,2,3,4\}$ and $B=$ collection of natural numbers less than 6
2) $A=\{$ prime numbers less than 6$\}$ and $B=\{$ prime factors of 30$\}$
3) $A=\{0\}$ and $B=\{x: x>15$ and $x<5\}$

Solution

In option (1), $A=\{1,2,3,4\}$ but $B=\{1,2,3,4,5\}$, hence not equal.

In option (2), $A=\{2,3,5\}$ but $B=\{2,3,5\}$, hence equal.
In option (3), $A=\{0\}$ but $B=$ Null set, hence not equal.
Hence, the answer is the option (2).

Example 2: Which of the following is NOT true?
1) Equivalent sets can be equal.
2) Equal sets are equivalent.
3) Equivalent sets are equal.
4) None of these

Solution
As we learned
In this Question,
Equivalent sets may or may not be equal sets but equal sets always have the same number of elements and hence equal sets are always equivalent.

Hence, the answer is the option 3.

Example 3: Which of the following sets is an empty set?
11) $A=\{x: x$ is an even prime number $\}$
2) $B=\{x: x$ is an even number divisible by 3$\}$
3) $\mathrm{C}=\{\mathrm{x}: \mathrm{x}$ is an odd integer divisible by 6$\}$
4) $\mathrm{D}=\{\mathrm{x}: \mathrm{x}$ is an odd prime number $\}$

Solution

In this Question,

Option 1 = ${2}$, Option 2 = ${6,12,18,....}$, Option 4 = ${3,5,7,...}$

For option 3, as there are no odd numbers divisible by $6$, so it is an empty set

Hence, the answer is the option 3.

Example 4: Which of the following is not an empty set?
1) Real roots of $x^2+2 x+3=0$
2) imaginary roots of $x^2+3 x+2=0$
3) Real roots of $x^2+x+1=0$
4) Real roots of $x^4-1=0$

Solution
Option (1) has imaginary roots as Discriminant $<0$. So, there is no real root, and the set is empty.

Option (2) has real roots as Discriminant $>0$. So, there is no imaginary root, and the set is empty.

Option (3) has imaginary roots as Discriminant $<0$. So, there is no real root, and the set is empty.

Option (4): $x^4-1=0 \Rightarrow\left(x^2-1\right)\left(x^2+1\right)=0$, which gives $x= \pm 1$
Hence, it has 2 real roots. So, it is not empty.
Hence, the answer is the option 4.

Example 5: Which of the following are NOT equivalent sets?
1) $P=\{A, B, C, D, E\}$ and $Q=\{$ Jan,Feb,Mar,April,May $\}$
2) $P=\{x: x$ is a prime number on dice $\}$ and $Q=\{x: x$ is an even number on dice $\}$
3) $P=\left\{x: x \in R\right.$ and $\left.x^2-5 x+6=0\right\}$ and $Q=\left\{x: x \in R\right.$ and $\left.x^2-4 x+5=0\right\}$
4) $A=\{x: x$ is a vowel $\}$ and $B=\{x: x$ is a natural number less than 6$\}$

Solution
In (A), both sets have $5$ elements, hence they are equivalent.
In (B), $P=\{2,3,5\}$ and $Q=\{2,4,6\}$, hence equivalent.
In (C), $P$ has 2 elements as there are 2 roots of $x^2-5 x+6=0$ (as discriminant $>0$ ) but $x^2-4 x+5=0$ has no real roots (as discriminant $<0$ ), so $Q$ is empty, hence they are not equivalent.

In (D), both sets have $5$ elements, hence they are equivalent.
Hence, the answer is the option 3.

Summary

This difference is crucial when comparing sets with the use of equals sign and equivalent sets to address any issues of relations aspects in a Maths class to students. Absolute synonyms in mathematics and science relate to two quantitative sets where the magnitude and quality of corresponding elements are proportional in that an equated element can match every element of the set in its corresponding set. On the other hand, all are equal numbers of elements or sets with cardinality that could be different though the elements could be different.