Even and Odd Function: Definition, Graph, Properties and Examples

In maths, there are various types of functions that we study. The process of determining whether a function is even or odd can be done by graphical method. They can be checked by plugging in the negative inputs (-x) in place of x into the function f(x) and considering the corresponding output values.These functions are crucial for numerous mathematical domains, such as algebra, calculus, and Fourier analysis.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. What is Even and Odd function?
2. Difference Between Even and Odd Function
3. Properties of Even and Odd Functions
4. Mixed type of functions
5. Even and Odd Functions in Trigonometry
6. Integral Properties of Even and Odd Functions
7. Fourier Series of Even and Odd Function
8. Solved Examples based on even and odd functions

In this article, we will cover the concepts of the even and odd functions. This concept falls under the broader category of sets relation and function, a crucial chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of one question has been asked on this concept, including one in 2019.

What is Even and Odd function?

Even and odd functions are functions which are classified based on the symmetry in functions.

Even Function

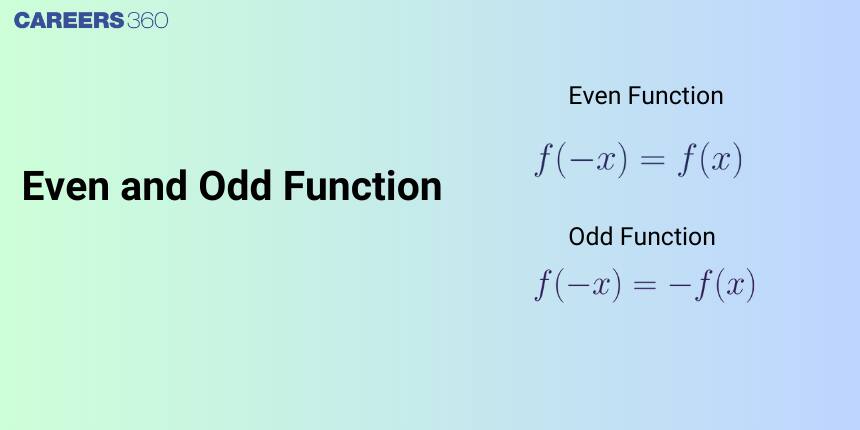
For a real-valued function $f(x)$, when the output value of $f(-x)$ is the same as $f(x)$, for all values of $x$ in the domain of $f$, the function is said to be an even function which means that the negative sign does not affect the value of the function.

If for a function $f(x)$, $f(-x)=f(x)$ always hold true, then the function is known as an even function. Even functions are symmetric about the $y$-axis.

$y = x^2$

$ y = |x| $

$y = cos(x)$

Odd Function

When all values of $x$ in the domain of $f$ for a real-valued function $f(x)$ have an output value of $f(-x)$ equal to the negative of $f(x)$, the function is said to be odd. Which means that the negative sign does affect the value of the function.

If for a function $f(x) and f(-x)=-f(x)$ then the function is known as an odd function. Odd functions are symmetric about the origin.

$y = sin(x)$

$y = x^3$

Condition for Even and Odd Functions

Even Function: An even function satisfies equation $f(-x)=f(x)$, for all values of $x$ in $D(f)$, where $D(f)$ denotes the domain of the function $f$.

In other words, we can say that the equation $f(-x)-f(x)=0$ holds for an even function, for all $x$.

Odd Function:

The following equation is necessary to hold for an odd function. For all values of $x$ in $D(f)$, where $D(f)$ represents the function $f$'s domain, $f(-x)$ equals $-f(x)$.We can state that for each $x$, the equation $f(-x)+f(x)=0$ holds true for an odd function.

Examples of Even and Odd Functions

Even Function: $f(x)=x^2$. $f(-x)=(-x)^2=x^2$ for all values of $x$, as the square of a negative number is the same as the square of the positive value of the number. This implies $f(-x)=f(x)$, for all $x$. Hence, $f(x)=x^2$ is an even function.

Similarly, functions like $x^4, x^6, x^8$, etc. are even functions.

Odd Function: $f(x)=x^3$. $f(-x)=(-x)^3=-\left(x^3\right)$ for all values of $x$, as the cube of a negative number is the same as the negative of the cube of the positive value of the number. This means that $f(-x)=-$ $f(x)$, for all $x$. Hence, $f(x)=x^3$ is an odd function.

Similarly, functions like $x^5, x^7$, $x^9$, etc. are odd functions.

How to Check Even and Odd Functions?

To know how to check even and odd functions, the following methods can be used.

1. Algebraic Method:

   • Substitute $−x$ into the function that is given to us.
   • Simplify the expression as much as we can
   • At the end we check if the resulting expression is equal to $ f(x)$ (even) or $−f(x)$ (odd).

2. Graphical Method:

   • We plot the function on graph or a sheet of paper.
   • Then we check for symmetry about the y-axis(if there then it is an even function) or the origin (if there then it is an odd function).

NOTE: We can have functions that are neither even nor odd. Eg, $ y = x+1$

Graph of Even and Odd Functions

Even Function: The graph of an even function remains unchanged when reflected across the $y$-axis. For example, $y=x^2$

Odd Function: The graph of an odd function remains unchanged when rotated $180$ degrees around the origin. For example, $y=x^3$

Difference Between Even and Odd Function

Properties of Even and Odd Functions

Both types of functions exhibit some properties as discussed below:

1. Addition and Subtraction:

   • The sum of two even functions is always even.
   • The sum of two odd functions is always odd.
   • The sum of an even and odd function is always an odd function.

2. Multiplication:

   • The product of two even functions is always even.
   • The product of two odd functions is always even.
   • The product of an even function and an odd function is always odd.

3. Multiplication:

   • The integral of an even function over a symmetric interval $[-3$, a $]$ is twice the integral from 0 to a: $\int_{-a}^a f(x) d x=2 \int_0^a f(x) d x$
   • The integral of an odd function over a symmetric interval $[-a, a]$ is zero:$\int_{-a}^a f(x) d x=0$

Mixed type of functions

• Both Even and Odd Functions- A real-valued function $f(x)$ is said to be both even and odd function if it satisfies the condition $f(-x)=f(x)$ and $f(-x)=-f(x)$ for all values of $x$ in the domain of $f(x)$. In mathematics, there is only one function which is both even and odd and that is the zero function for all $x$. We know that for zero function, $f(-x)=-f(x)=f(x)=0$, for all values of $x$. Hence, it can be said that $f(x)=0$ is both an even and odd function.
• Neither Even Nor Odd Function - A real-valued function $f(x)$ is said to be neither even nor odd if it does not satisfy $f(-x)=f(x)$ and $f(-x)=-f(x)$ for atleast one value of $x$ in the domain of the function $f(x)$. Let us consider an example to understand the definition better. Consider $\mathrm{f}(\mathrm{x})=8 \mathrm{x}^5+5 \mathrm{x}^2+1, \mathrm{f}(-\mathrm{x})=8(-\mathrm{x})^5+5(-x)^2+1=-8 x^5+5 x^2+1$ which is neither equal to $f(x)$ nor $-\mathrm{f}(\mathrm{x})$. Hence, can be concluded that $\mathrm{f}(\mathrm{x})=8 \mathrm{x}^5+$ $5 x^2+1$ is neither even nor odd function.

Even and Odd Functions in Trigonometry

Even in the field of trigonometry, we have the concept of even and odd functions as very useful. The various quadrants in the 2-D plane carry different signs of trigonometric ratios. Their nature changes with sign as the quadrant changes.In the first quadrant , all six trigonometric ratios have positive values. In the second quadrant, only sine and cosecant are positive. In the third quadrant, only tangent and cotangent are positive. In the fourth quadrant, only cosine and secant are positive.

Now to check whether a function is even or odd, we take the help of a unit circle. A unit circle can be used to check if a trigonometric ratio is even or odd in nature. An angle measured in anticlockwise direction is a positive angle whereas the angle measured in the clockwise direction is a negative angle.

• $\sin \theta=y, \sin (-\theta)=-y ;$ Therefore, $\sin (-\theta)=-\sin \theta .($ odd function).
• $\cos \theta=y, \cos (-\theta)=y ;$ Therefore, $\cos (-\theta)=\cos \theta .($ even function).
• $\tan \theta=y, \tan (-\theta)=-y$; Therefore, $\tan (-\theta)=-\tan \theta$. (odd function).
• $\operatorname{cosec} \theta=y, \operatorname{cosec}(-\theta)=-y ;$ Therefore, $\operatorname{cosec}(-\theta)=-$ $\operatorname{cosec} \theta$. (odd function.)
• $\sec \theta=y, \sec (-\theta)=y ;$ Therefore, $\sec (-\theta)=\sec \theta$. (even function).
• $\cot \theta=y, \cot (-\theta)=-y ;$ Therefore, $\cot (-\theta)=-\cot \theta$. (odd function).

Integral Properties of Even and Odd Functions

We know that the integral of a function always gives the area below the curve.If the function is even or odd, and provided that the interval is [-a, a], we can apply the following two rules:

• When $f(x)$ is even, $\int^{-a}_ a \int^{a}_{-a} f(x) d x=2 \int^{0}_ a \int^{0}_ a f(x) d x$
• When $f(x)$ is odd, $\int^{-a}_ a \int^{a}_ {-a} f(x) d x=0$

Fourier Series of Even and Odd Function

Fourier series is the representation of periodic function as the sum of sine and cosines. The fourier series of a periodic function with period $2L$ is $f(x)=a_0+\sum_{n=1}^{\infty}\left[a_n \cos \left(\frac{n \pi x}{L}\right)+b_n \sin \left(\frac{n \pi x}{L}\right)\right]$ where $a_0=\frac{1}{2 L} \int_{-L}^L f(x) d x$, $a_n=\frac{1}{L} \int_{-L}^L f(x) \cos \left(\frac{n \pi x}{L}\right) d x$ and .$b_n=\frac{1}{L} \int_{-L}^L f(x) \sin \left(\frac{n \pi x}{L}\right) d x$.

Fourier series of even function: The sine terms $\left(b_n\right)$ disappears because $\sin (-x)=-\sin (x)$. Integrating an odd function over a symmetric interval results in zero. Only the sine terms will remain in the fourier series.

Fourier series of odd function: The cosine terms $\left(a_n\right)$ vanish because $\cos (-x)=\cos (x)$. Integrating an odd function multiplied by an even function over a symmetric interval results in zero. Only the cosine terms will remain in the fourier series.

How to Determine Even and Odd Function in Fourier Series?

• Analyze if $f(-x)=f(x)$ or $f(-x)=-f(x)$.
• Apply the formulas for $a_n$ and $b_n$ to identify non-zero coefficients.
• If $a_n$ is $0$ then it is a odd function and if $b_n$ is $0$ then it is a even function.

Fourier Series Even and Odd Function Formula

Now let us look into the fourier series formula for even and odd functions.

Even function: Fourier series formula for even functions is $f(x)=a_0+\sum_{n=1}^{\infty} a_n \cos \left(\frac{n \pi x}{L}\right)$ where $a_n=\frac{2}{L} \int_0^L f(x) \cos \left(\frac{n \pi x}{L}\right) d x$.

Odd function: Fourier series formula for odd functions is $f(x)=\sum_{n=1}^{\infty} b_n \sin \left(\frac{n \pi x}{L}\right)$ where $b_n=\frac{2}{L} \int_0^L f(x) \sin \left(\frac{n \pi x}{L}\right) d x$.

Important Notes on Even and Odd Functions

• - A function $f(x)$ is even if $f(-x)=f(x)$, for all values of $x$ in $D(f)$ and it is odd if $f(-x)=-f(x)$, for all values of $x$.
• In trigonometry, $\cos \theta$ and $\sec \theta$ are even functions whereas $\sin \theta, \operatorname{cosec} \theta, \tan \theta, \cot \theta$ are odd functions.
• The graph even function is symmetric with respect to the $y$-axis and the graph of an odd function is symmetric about the origin.
• $f(x)=0$ is the only function that is an even and odd function both.

Recommended Video Based on Even and Odd Functions

Solved Examples based on even and odd functions

Example 1: The graph of the function $y=f(x)$ is symmetrical about the line $x=2$, then
1) $f(x)=f(-x)$
2) $f(2+x)=f(2-x)$
3) $f(x+2)=f(x-2)$
4) $f(x)=-f(-x)$

Solution:

Since a graph is symmetric about the $y$-axis means $x=0$ then it is even function and $f(-x)=f(x)$
$\therefore \quad \mathrm{f}(0-\mathrm{x})=\mathrm{f}(0+\mathrm{x}) \quad(\mathrm{b}<\mathrm{z}$ it is symmetric about $\mathrm{v}=0)$
But in question, it is symmetric about $x=2$ then $f(2-x)=f(2+x)$

Hence, the answer is option 2 .

Example 2: Which of the following functions is an even function?

1) $x^6+3 x^3-5$
2) $x^6+3 x^4-5$
3) $x^6+3 x^3-5 x$
4) $x^6+3 x^4-5 x$

Solution:
|f $f(x)=x^6+3 x^4-5$

$f(-x)=x^6+3 x^4-5=f(x)$
Hence, it is an even function

Hence, the answer is option 2.

Example 3: Which of the following functions is an even function?

1) $\sin x$
2) $\cos x$
3) $\tan x$
4) $\csc x$

Solution:
Even Function -

$f(-x)=f(x)$

Symmetric about $\gamma_{\text {-axis }}$
If $f(x)=\cos x$
then $f(-x)=\cos (-x)=\cos x$
Hence, the answer is option 2 .

Example 4: A function $f$ from the set of natural numbers to integers defined by
$f(n)=\left\{\begin{array}{l}\frac{n-1}{2}, \text { when } n \text { is odd } \\ -\frac{n}{2}, \text { when } n \text { is even is }\end{array}\right.$

1) onto but not one­-one

2) one­-one and onto both

3) neither one-­one nor onto

4) one­-one but not onto.

Solution:

$f(x)= \begin{cases}\frac{n-1}{2} & \text { when } n \text { is odd } \\ -\frac{n}{2} & \text { when } n \text { is even }\end{cases}$

$f(1) = 0$

$f(2) = -1$

$f(3) = 1$

$f(4) = 2$

$f(5) = 2$

Clearly, this pattern will cover all the integers, hence onto

Also, it is a one-one function

Hence, the answer is option 2

Example 5: The function $f(x)=\log \left(x+\sqrt{x^2+1}\right)$
1) an odd function
2) a periodic function
3) neither an even nor an odd function
4) an even function.

Solution:
As we learned in
Odd Function -

$f(-x)=-f(x)$

- wherein

Symmetric about origin

$\begin{aligned}
& f(x)=\log \left(x+\sqrt{1+x^2}\right) \\
& f(-x)=\log \left(-x+\sqrt{1+x^2}\right) \\
& f(x)+f(-x)=\log \left(\sqrt{1+x^2}+x\right)+\log \left(\sqrt{1+x^2}-x\right) \\
& =\log \left(1+x^2-x^2\right)=\log 1=0
\end{aligned}$
It is an odd function.

Hence, the answer is the option 1.

List of Topics Related to Even and Odd Function