Finite and Infinite sets

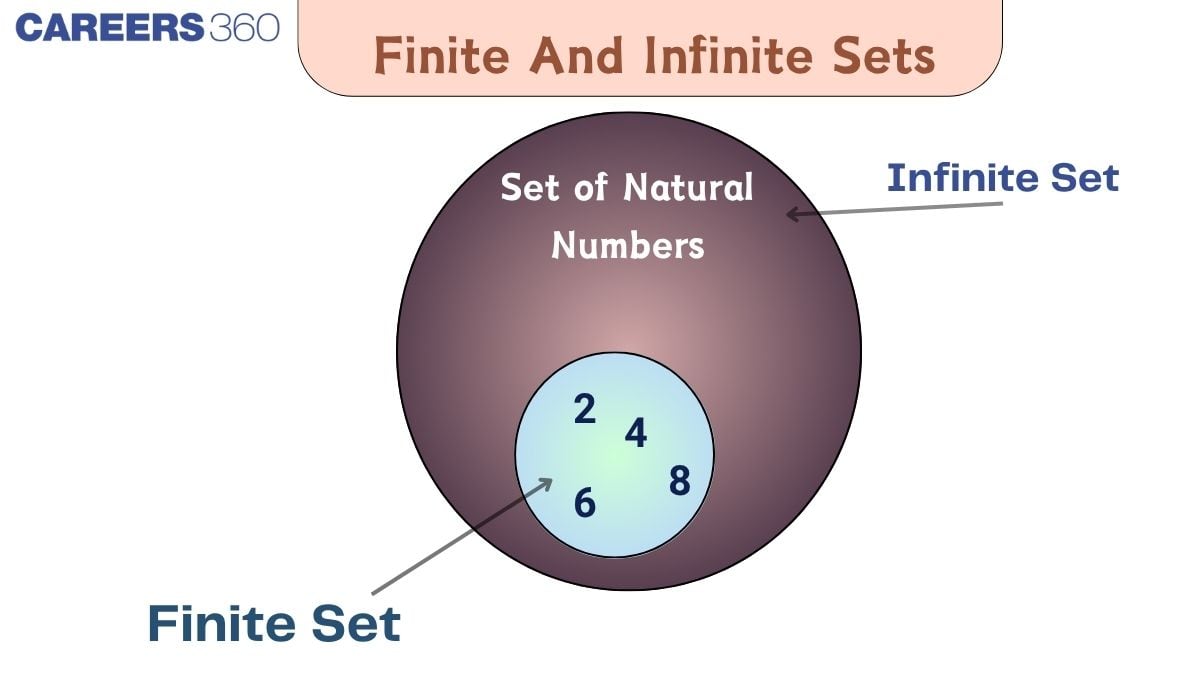
What are Finite and Infinite Sets?

Set is simply a collection of distinct objects, considered as a whole. These objects, called elements or members of the set, can be anything: numbers, people, letters, etc. Sets are particularly useful in defining and working with groups of objects that share common properties.

It is a well-defined collection of distinct objects, and it is usually denoted by capital letters A, B, C, S, U, V…...

Consider the set of all even numbers less than 100; the elements in this set are countable. Now consider the set of all natural numbers; the elements in this set are not countable, as the natural numbers go on till infinity. A set with countable elements is a finite set, whereas a set in which the number of elements is uncountable is an infinite set.

Finite set

A set that is empty or consists of a finite number of elements is called a finite set.

Examples: $\varphi,\{\mathrm{a}\},\{1,2,5,9\},\{\mathrm{x}: \mathrm{x}$ is a person of age more than 18$\}$

Properties of Finite Sets

• A subset of a Finite set is finite
• The union of two or more finite sets is finite
• The power set of a finite set is countable

Cardinality of a Finite Set

If '$a$' represents the number of elements of set $A$, then the cardinality of a finite set is $n(A)=a$. The cardinality of a finite set is a natural number or possibly $0$; it can be either.

So, the Cardinality of the set $A$ of all English alphabet is 26 because the number of elements (alphabets) is $26$.

Hence, $n(A)=26$.

Infinite Set

A set which has infinite elements is called an infinite set.

Examples: A set of all the lines passing through a point, a set of all circles in a plane, a set of all points in a plane, $N, Z, Q, Q^{\prime}, R$,

$\{x: 2<x<2.1\}$

Properties of Infinite Sets

• The union of two or more infinite sets is infinite
• The power set of an uncountable infinite set is infinite
• The superset of an infinite set yields an infinite set

Cardinality of Infinite Sets

The cardinality of a set is $n(A)=x$, where $x$ is the number of elements of a set $A$. The cardinality of an infinite set is $n(A)=\infty$ as the number of elements is unlimited in it.

Countable Finite and Infinite Sets

A set is said to be countable if its elements can be matched one-to-one with the set of natural numbers. All finite sets are countable by definition. Interestingly, some infinite sets, like the set of all integers, are also countable because we can still arrange them in a sequence. However, some sets, like the set of real numbers between 0 and 1, are uncountably infinite.

Whether you are exploring examples for finite and infinite sets or learning about finite and infinite sets in discrete mathematics, understanding the concept of countability is key.

Difference between Finite and Infinite Sets

Understanding the difference between finite and infinite sets is essential in set theory. This section highlights the key distinctions based on the number of elements, representation, and practical examples to help you grasp the concept clearly.

Note: Empty and singleton sets are finite sets.

Solved Examples Based on the Finite and Infinite Sets

Example 1: Which of the following sets is a finite set?
1) $P=\{$ natural numbers greater than $50\}$
2) $Q=\{$ integers less than $5\}$
3) $R=\{$ whole numbers more than $10\}$
4) $\mathrm{S}=\{$ natural numbers less than $5\}$

Solution:
There are only $4$ natural numbers less than $5$. So it is a finite set.
Hence, the answer is option 4.

Example 2: Which of the following is an example of an infinite set?
1) A set of all the persons living in India.
2) A set of all the human beings living on Mars.
3) Set of all the stars in the Universe.
4) The set of satellites of Earth.

Solution:
As there are infinite stars in the Universe, so is an infinite set.
Note that the population of India is finite.
Hence, the answer is option 3.

Example 3: Which of the following is not an infinite set?
1) Set of all real numbers.
2) Set of all perfect squares.
3) Set of all the divisors of $x$, where $x \in N$.
4) Set of all prime numbers.

Solution:
The number of divisors of a number is finite.
Hence, the answer is option 3.

Example 4: Which of the following sets is infinite?
1) A set of all the lines passing through a point.
2) A set of lines passing through two distinct points.
3) Divisors of 11.
4) Months of a year.

Solution:
In this Question,
Infinite lines are passing through a point, so it is an infinite set.
For the second option, there is only one such line, so it is a finite set
For option C, divisors of 11 are 1 and 11, so it is a finite set.
For option $D$, the set has 12 elements, so it is a finite set.
Hence, the answer is option 1.

Example 5: Which of the following sets is a finite set?
1) A set of all points in a plane.
2) A set of all points on a line segment.
3) Set of all lines in a plane.
4) Set of all circles passing through three non-collinear points.

Solution

In this question,
The number of geometrical points and lines in a plane is infinite. Also, the number of points in a line segment is infinite.
However, there is only one circle passing through three non-collinear points, so it is a finite set (as it has one element).
Hence, the answer is option 4.

List of Topics Related to Finite and Infinite Sets

To fully understand finite and infinite sets, it's important to explore several related topics in set theory. Concepts like roster and set builder form, universal set, subsets, complement of a set, and De Morgan's laws provide essential context and connections. This list will help you build a well-rounded understanding of how sets behave and interact.

NCERT Useful Resources

This section features a curated set of useful NCERT study resources for Class 11 Mathematics Chapter 1: Sets, aimed at supporting thorough concept clarity and exam readiness. It includes detailed chapter notes, step-by-step solutions to NCERT textbook exercises, and a wide range of exemplar problems, making it a complete toolkit for mastering the topic of Sets.

NCERT Maths Class 11 Chapter 1 Sets Notes

NCERT Maths Class 11 Chapter 1 Sets Solutions

NCERT Maths Exemplar Problems Class 11 Chapter 1 Sets

Practice Questions based on Finite and Infinite Sets

This section presents a thoughtfully curated set of practice questions on Finite and Infinite Sets, designed to strengthen your conceptual understanding through application. The questions include multiple-choice formats that also touch upon related topics such as Singleton Sets, Power Sets, and set operations like Union, Intersection, and Difference, helping you prepare effectively for exams.

Finite Set, Infinite Set, Singleton Set - Practice Question MCQ

Practice questions on the next topics covering various concepts based on sets, properties of sets such as union, intersection, difference, etc.