Functions, Image and Pre-image: Definition and Examples

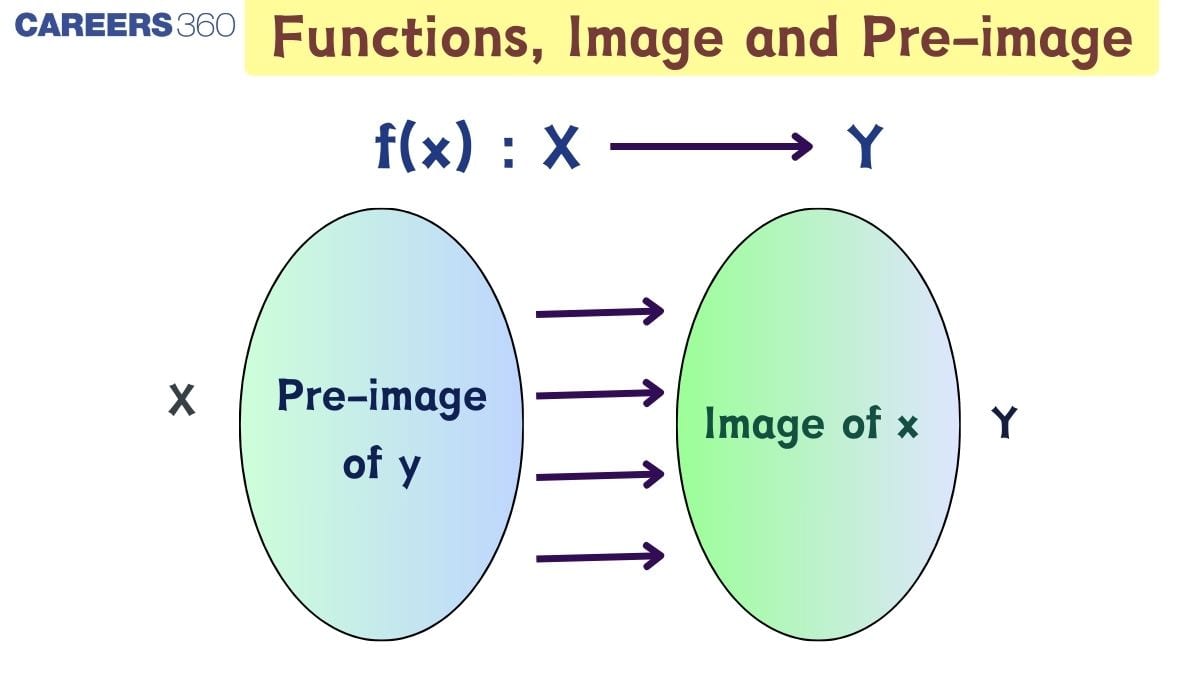
Introduction to Functions, Image, and Pre-image

Functions, image, and pre-image are fundamental ideas in mathematics that describe how inputs are connected to outputs. The image of a function is the set of resulting values after applying the function rule, while the pre-image is the set of original inputs that produce a given output. These concepts are essential for understanding domain, range, and mapping in algebra and set theory.

What is a Function in Mathematics?

A function is a special type of relation where every element of the input set (domain) is paired with exactly one element of the output set (codomain).

Definition:
A relation from a set $A$ to a set $B$ is said to be a function from $A$ to $B$ if every element of set $A$ has one and only one image in set $B$.

Alternate form:
If $A$ and $B$ are two non-empty sets, then a relation from $A$ to $B$ is said to be a function if each element $x$ in $A$ is assigned a unique element $f(x)$ in $B$, and it is written as:
$f: A \rightarrow B$
This is read as "$f$ is a mapping from $A$ to $B$".

Example:
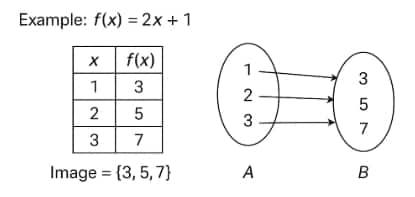
If $f(x) = 2x + 1$ and $A = {1, 2, 3}$, then the mapping is:
$f(1) = 3,\ f(2) = 5,\ f(3) = 7$

Understanding Domain, Range, and Mapping

• Domain: The set of all possible input values (pre-images). For $f(x) = x^2$, the domain can be all real numbers $\mathbb{R}$.

• Range: The set of all possible output values (images). For $f(x) = x^2$, the range is $[0, \infty)$.

• Mapping: The pairing between each domain element and its range element. This can be shown with an arrow diagram:
  $1 \rightarrow 3,\ 2 \rightarrow 5,\ 3 \rightarrow 7$

Mapping diagrams make it clear how pre-images from the domain lead to images in the range.

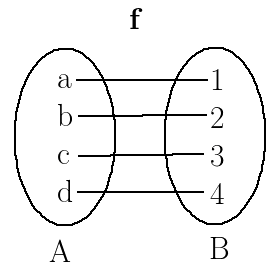
Function

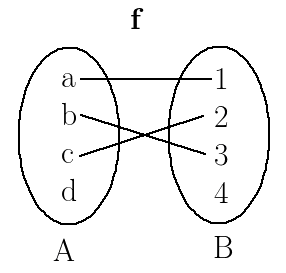
Function

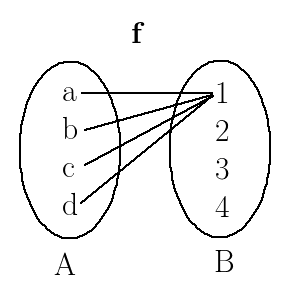
Not a function

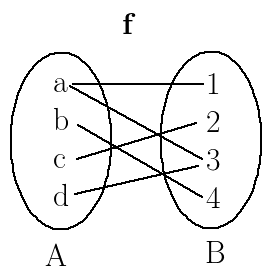
Not a function

The third one is not a function because $d$ is not related(mapped) to any element in $B$.

Fourth is not a function as element a in $A$ is mapped to more than one element in $B$.

Image of a Function

The image of a function refers to the set of all output values that the function produces from its domain.

Definition:

Given a function $f: A \rightarrow B$ and a subset $X \subseteq A$, the image of $X$ under $f$ is:
$f(X) = \{ f(x) \mid x \in X \}$

If we take the entire domain $A$, the image of the function $f$ (also called the range) is:
$\text{Image}(f) = f(A) = \{ f(a) \mid a \in A \}$

Important Notes:

1. Always specify whether you are talking about the image of an element or of a subset.

2. $f(x)$ is an element of the codomain, whereas $f(C)$ is a subset of the codomain.

3. If $y \in f(C)$, then $y \in B$ and there exists an $x \in C$ such that $f(x) = y$.

How to Find the Image of a Function

1. Start with the function rule $f(x)$.

2. Substitute each input from the domain into $f(x)$.

3. Collect all distinct outputs — these form the image.

Example: For $f(x) = 2x + 1$ and $A = {1, 2, 3}$, the image is:
$f(1) = 3,\ f(2) = 5,\ f(3) = 7$
So, $\text{Image}(f) = {3, 5, 7}$.

Example : If $f(x) = x^2$ and $A = {-2, -1, 0, 1, 2}$,
$f(-2) = 4,\ f(-1) = 1,\ f(0) = 0,\ f(1) = 1,\ f(2) = 4$
Thus, $\text{Image}(f) = {0, 1, 4}$.

Image in Set Theory and Real-Life Applications

• Set Theory: Images are used to define mappings and to check properties like injectivity and surjectivity.

• Real-Life: For example, if a function converts Celsius to Fahrenheit, the set of temperatures in Fahrenheit you get is the image of the Celsius inputs.

Pre-image of a Function

The pre-image of a function is the set of all input values that map to a specific output value or set of output values.

Definition:

Given a function $f: A \rightarrow B$ and a subset $Y \subseteq B$, the pre-image of $Y$ under $f$ is:
$f^{-1}(Y) = \{ x \in A \mid f(x) \in Y \}$

If $y \in B$, the pre-image of ${y}$ is:
$f^{-1}(\{y\}) = \{ x \in A \mid f(x) = y \}$

Steps to Find the Pre-image of a Function

1. Identify the output value(s) in $B$.

2. Solve the equation $f(x) = y$ for $x$.

3. Collect all solutions — these form the pre-image.

Example: If $f(x) = x^2$ and $y = 4$,
$x^2 = 4 \quad \Rightarrow \quad x = -2,\ 2$
So, $f^{-1}({4}) = {-2, 2}$.

Pre-image of a Set Under a Function

If $Y = {1, 4}$ and $f(x) = x^2$, then:
$f^{-1}(Y) = \{ x \in \mathbb{R} \mid x^2 = 1 \ \text{or} \ x^2 = 4 \}$
Thus, $f^{-1}(Y) = {-2, -1, 1, 2}$.

Pre-image Examples in Algebra and Set Theory

• Algebra: In $f(x) = 3x - 1$, the pre-image of $5$ is found by solving $3x - 1 = 5 \ \Rightarrow \ x = 2$.

• Set Theory: If $B = {b_1, b_2}$ and $f$ maps multiple $a_i$ to the same $b_j$, then all those $a_i$ are part of the pre-image of ${b_j}$.

Image vs Pre-image in Functions

In mathematics, image and pre-image describe the two sides of a function’s mapping process. The image focuses on the output side (results), while the pre-image focuses on the input side (origins). Understanding both helps in analysing the domain, range, and the overall behaviour of a function.

Difference Between Image and Pre-image

Relation Between Image, Pre-image, Domain, and Range

1. Domain ($A$) – The set of all possible input values. Every pre-image belongs to the domain.

2. Range (Image of Entire Domain) – The set $f(A)$ consisting of all actual outputs of $f$.

3. Image of a Subset ($f(X)$) – Outputs corresponding to inputs in subset $X \subseteq A$.

4. Pre-image of a Subset ($f^{-1}(Y)$) – Inputs that produce outputs in subset $Y \subseteq B$.

Key Relation:

• If $y \in f(X)$, then $\exists, x \in X$ such that $f(x) = y$.

• If $x \in f^{-1}(Y)$, then $f(x) \in Y$.

Example:

Let $f: \mathbb{R} \rightarrow \mathbb{R}$ be $f(x) = x^2$.

• Domain: $\mathbb{R}$

• Range: $[0, \infty)$

• Image of ${-2, 2, 3}$: ${4, 9}$

• Pre-image of ${4, 9}$: ${-2, 2, -3, 3}$

Solved Examples Based On the Image and Pre-image of Functions

Example 1: A real-valued function $f(x)$ satisfies the functional equation

$f(x-y) = f(x) f(y) - f(a-x) f(a+y)$ where $a$ is a given constant and $f(0) = 1$.

Find $f(2a - x)$.

1) $f(x)$

2) $-f(x)$

3) $f(-x)$

4) $f(a) + f(a-x)$

Solution:

$f(x-y) = f(x) f(y) - f(a-x) f(a+y)$

$f(0) = 1,\quad f(2a-x) = ?$

Put $x = 0,\ y = 0$:

$f(0) = f(0) \times f(0) - f(a) \times f(a)$

$1 = 1 - f^2(a)$

$f^2(a) = 0$

$f(a) = 0$

Now, $f(2a - x) = f(a + a - x) = f(a - (x-a))$

Let $x \rightarrow a$ and $y \rightarrow x-a$:

$f(a) f(x-a) - f(a-a) f(a + x-a) = 0 - 1 \times f(x) = -f(x)$

Hence, the answer is option 2.

Example 2: If $f(x) + 2f\left(\frac{1}{x}\right) = 3x,\ x \neq 0$, and

$S = \{x \in \mathbb{R} : f(x) = f(-x)\}$, then $S$:

1) is an empty set

2) contains exactly one element

3) contains exactly two elements

4) contains more than two elements

Solution:

$f(x) + 2f\left(\frac{1}{x}\right) = 3x$

Interchanging $x$ with $\frac{1}{x}$:

$f\left(\frac{1}{x}\right) + 2f(x) = \frac{3}{x}$

Multiply the first equation by $2$:

$2f(x) + 4f\left(\frac{1}{x}\right) = 6x$

Compare with the second equation:

$2f\left(\frac{1}{x}\right) + f(x) = 3x$

Subtract:

$3f(x) = \frac{6}{x} - 3x$

$f(x) = \frac{2}{x} - x$

Now, $f(-x) = \frac{2}{-x} + x$

Set $f(x) = f(-x)$:

$\frac{2}{x} - x = -\frac{2}{x} + x$

$\frac{4}{x} - 2x = 0$

$\frac{4 - 2x^2}{x} = 0$

$4 = 2x^2$

$x^2 = 2$

$x = \pm\sqrt{2}$, $x \neq 0$

Hence, the answer is option 3.

Example 3: Which of the following relations is not a function?

1) $\{(1,2), (1,3), (2,3), (1,4)\}$

2) $\{(1,2), (2,2), (3,2), (5,4)\}$

3) $\{(1,2), (3,4), (5,6)\}$

4) $\{(1,2), (3,4)\}$

Solution:

In option 1, the element $1$ has two images, $2$ and $3$. Hence it is not a function.

Hence, the answer is option 1.

Example 4: If $n(A) = 4$ and $n(B) = 3$, then the total number of functions from $A$ to $B$ is:

Solution:

As we learned, the number of functions:

$f: A \rightarrow B$

$n(A) = m$

$n(B) = n$

Total number of functions $= n^m$

Number of functions $= (n(B))^{n(A)}$

$= 3^4 = 81$

Hence, the answer is $81$.

Example 5: If $n(A) = n(B)$ and total functions from $A$ to $B$ are $3125$, then what is the value of $n(A) + n(B)$?

Solution:

As we learned, the number of functions:

$f: A \rightarrow B$

$n(A) = m$

$n(B) = n$

Total number of functions $= n^m$

Since $3125 = 5^5$

Thus, $n(A) = n(B) = 5$

Thus, $n(A) + n(B) = 10$

Hence, the answer is $10$.

List of Topics related to Functions, Image and Pre-Image

Understanding functions, image, and pre-image opens the door to many important topics in mathematics. From algebraic functions to domain, co-domain, and range, each concept helps us explore how inputs map to outputs. Learning about piecewise functions, transformations of functions, and the Cartesian product with ordered pairs gives a complete picture of how relationships between sets work. These topics build a strong foundation for solving problems in algebra, set theory, and beyond.

Algebraic Functions

Domain, Co-Domain and Range of a Function

Piecewise Function

Domain, Range of Relation

Transformation of functions

Cartesian Product and Ordered Pairs

NCERT Resources

For mastering functions, image, and pre-image, NCERT resources offer clear explanations and practice. The NCERT notes for Class 12 Maths Chapter 1: Relations and Functions give a quick revision of key concepts, while the NCERT solutions help in step-by-step problem solving. The NCERT exemplar solutions go deeper with advanced questions, strengthening understanding and application skills.

NCERT Notes for Class 12 Maths Chapter 1 - Relations and Functions

NCERT Solutions for Class 12 Maths Chapter 1 - Relations and Functions

NCERT Exemplar Solutions for Class 12 Maths Chapter 1 - Relations and Functions

Practice Questions based on Functions, Image and Pre-Image

Strengthen your understanding of functions, image, and pre-image with targeted practice questions and MCQs. Test your knowledge on important topics like domain, co-domain, and range of a function, one-to-one and many-to-one functions, onto and into functions, and bijective functions. You can also practice composition of functions, their conditions and properties, as well as finding the inverse of a function. These questions are designed to improve both conceptual clarity and problem-solving speed.

Functions Image And Pre Image Practice Question MCQ

You can practice the following topics: