General Solution of Trigonometric Equations

Edited By Komal Miglani | Updated on Jul 02, 2025 07:40 PM IST

A trigonometric equation is any equation that contains trigonometric functions. We have learned about trigonometric identities, which are satisfied for every value of the involved angles whereas, trigonometric equations are satisfied only for some values (finite or infinite in number) of the angles. In real life, we use trigonometric equations for making roof inclination, installing ceramic tiles, and building and navigating directions.

This Story also Contains

Trigonometric Equations
Solution of Trigonometric Equation
General Solution of some Standard Equations
Important Points to remember while solving trigonometric equations
Summary
Solved Examples Based on Solution of Trigonometric Equations

General Solution of Trigonometric Equations

In this article, we will cover the concept of Solution of Trigonometric Equations. This category falls under the broader category of Trigonometry, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of thirty-one questions have been asked on this concept, including one in 2016, two in 2017, one in 2019, one in 2020, seven in 2021, nine in 2022 and eight in 2023.

Trigonometric Equations

Trigonometric equations are, as the name implies, equations that involve trigonometric functions. Trigonometric equations are satisfied only for some values (finite or infinite in number) of the angles. A value of the unknown angle that satisfies the given trigonometric equation is called a solution or a root of the equation. For example, equation 2 sin x = 1 is satisfied by x = π/6 is the solution of the equation between o and π. The solutions of a trigonometric equation lying in the interval [0,π ) are called principal solutions.

We know that trigonometric ratios are periodic functions. Functions sin x, cos x, sec x, and cosec x are periodic with period 2π and functions tan x and cot x are periodic functions with period π. Therefore, solutions of trigonometric equations can be generalized with the help of the period of trigonometric functions.

NEET Highest Scoring Chapters & Topics

This ebook serves as a valuable study guide for NEET exams, specifically designed to assist students in light of recent changes and the removal of certain topics from the NEET exam.

Download E-book

What are Trigonometric Equations?

The linear equation $a x+b=0$ can be written as a trigonometric equation as

$
a \sin \theta+b=0
$

which is also sometimes written as

$
\sin \theta=\sin \alpha
$

The quadratic equation

$
a x^2+b x+c=0
$

as an example of a trigonometric equation is written as

$
a \cos ^2 \theta+b \cos \theta+c=0
$

But unlike normal solutions of equations, where the number of solutions is based on the degree of the variable, in trigonometric equations, the same value of the solution exists for different values of $\theta$.

For example,
$\sin \theta=\frac{1}{2}=\sin \frac{\pi}{6}=\sin \frac{5 \pi}{6}=\sin \frac{13 \pi}{6}$, and so on, as the values of the sine function repeat after every $2 \pi$ radians.
Examples of Trigonometric Equations

$
\begin{gathered}
\sin 2 x-\sin 4 x+\sin 6 x=0 \\
2 \cos ^2 x+3 \sin x=0 \\
\cos 4 x=\cos 2 x \\
\sin 2 x+\cos x=0 \\
\sec ^2 2 x=1-\tan 2 x
\end{gathered}
$

Solution of Trigonometric Equation

The value of an unknown angle that satisfies the given trigonometric equation is called a solution or root of the equation. For example, $2 \sin \theta=1$, clearly $\theta=30^{\circ}$ satisfies the equation; therefore, $30^{\circ}$ is a solution of the equation. Now trigonometric equation usually has infinite solutions due to the periodic nature of trigonometric functions. So this equation also has (360+30)^o,(720+30)^o,(-360+30)^o, and so on, as its solutions.

Some important proofs.

1. Prove that for any real numbers x and y, sin x = sin y implies x = nπ + (-1)ⁿy, where n ∈ Z

Proof: If $\sin x=\sin y$, then

$
\begin{gathered}
\sin x-\sin y=0 \\
\Rightarrow 2 \cos \left(\frac{x+y}{2}\right) \sin \left(\frac{x-y}{2}\right)=0 \\
\Rightarrow \cos \left(\frac{x+y}{2}\right)=0 \quad \text { or } \quad \sin \left(\frac{x-y}{2}\right)=0 \\
\Rightarrow \frac{x+y}{2}=\frac{(2 n+1) \pi}{2} \quad \text { or } \quad \frac{x-y}{2}=n \pi, \quad \text { where } n \in \mathbb{Z}
\end{gathered}
$

[Because $\sin A=0$ implies $A=n \pi$ and $\cos A=0$ implies $A=\frac{(2 n+1) \pi}{2}$, where $n \in \mathbb{Z}$ ]

$
\begin{gathered}
\Rightarrow x=(2 n+1) \pi-y \quad \text { or } \quad x=2 n \pi+y, \quad \text { where } n \in \mathbb{Z} \\
\Rightarrow x=(2 n+1) \pi+(-1)^{2 n+1} y \quad \text { or } \quad x=2 n \pi+(-1)^{2 n} y, \quad \text { where } n \in \mathbb{Z}
\end{gathered}
$

Combining these two results, we get:

$
x=n \pi+(-1)^n y, \quad \text { where } n \in \mathbb{Z}
$

2. Prove that for any real numbers x and y, cos x = cos y implies x = 2nπ ± y, where n ∈ Z.

Proof: If $\cos x=\cos y$, then

$
\begin{gathered}
\cos x-\cos y=0 \\
\Rightarrow-2 \sin \left(\frac{x+y}{2}\right) \sin \left(\frac{x-y}{2}\right)=0 \\
\Rightarrow \sin \left(\frac{x+y}{2}\right)=0 \quad \text { or } \quad \sin \left(\frac{x-y}{2}\right)=0 \\
\Rightarrow \frac{x+y}{2}=n \pi \quad \text { or } \quad \frac{x-y}{2}=n \pi, \quad \text { where } n \in \mathbb{Z}
\end{gathered}
$

(Because $\sin A=0$ implies $A=n \pi$, where $n \in \mathbb{Z}$ )

$
\Rightarrow x=2 n \pi-y \quad \text { or } \quad x=2 n \pi+y, \quad \text { where } n \in \mathbb{Z}
$

Hence,

$
x=2 n \pi \pm y, \quad \text { where } n \in \mathbb{Z}
$

3. Prove that if x and y are not odd multiples of π/2, then tan x = tan y implies x = nπ + y, where n ∈ Z.

Proof: If $\tan x=\tan y$, then

$
\begin{gathered}
\tan x-\tan y=0 \\
\Rightarrow \frac{\sin x}{\cos x}-\frac{\sin y}{\cos y}=0 \\
\Rightarrow \frac{\sin x \cos y-\cos x \sin y}{\cos x \cos y}=0 \\
\Rightarrow \sin (x-y)=0
\end{gathered}
$

$\Rightarrow x-y=n \pi, \quad$ where $n \in \mathbb{Z} \quad[$ Because $\sin A=0$ implies $A=n \pi$, where $n \in \mathbb{Z}]$

$
\Rightarrow x=n \pi+y, \quad \text { where } n \in \mathbb{Z}
$

Principal Solution

The solutions of a trigonometric equation that lie in the interval [0, 2π). For example, if $2 \sin \theta=1$, then the two values of sinӨ between 0 and 2π are π/6 and 5π/6. Thus, π/6 and 5π/6 are the principal solutions of equation $2 \sin \theta=1$.

General Solution

As trigonometric functions are periodic, solutions are repeated within each period, so, trigonometric equations may have an infinite number of solutions. The solution consisting of all possible solutions of a trigonometric equation is called its general solution.

We have the following trigonometric equations whose solutions are quadrantile angles.

Equation	Solution
$\sin \theta=0$	$\theta=n \pi, \quad n \in \mathbb{I}$
$\cos \theta=0$	$\theta=(2 n+1) \frac{\pi}{2}, \quad n \in \mathbb{I}$
$\tan \theta=0$	$\theta=n \pi, \quad n \in \mathbb{I}$
$\sin \theta=1$	$\theta=(4 n+1) \frac{n}{2}, \quad n \in \mathbb{I}$
$\cos \theta=1$	$\theta=2 n \pi, \quad n \in \mathbb{I}$
$\sin \theta=-1$	$\theta=(4 n-1) \frac{\pi}{2}, \quad n \in \mathbb{I}$
$\cos \theta=-1$	$\theta=(2 n+1) \pi, \quad n \in \mathbb{I}$
$\cot \theta=0$	$\theta=(2 n+1) \frac{n}{2}, \quad n \in \mathbb{I}$

Steps to Solve Trigonometric Equations

Transform the given trigonometric equation into an equation with a single trigonometric ratio (sin, cos, tan).
Change the equation with the trigonometric equation, having multiple angles, or submultiple angles into a simple angle.
Now represent the equation as a polynomial equation, quadratic equation, or linear equation.
Solve the trigonometric equation similar to normal equations, and find the value of the trigonometric ratio.
The angle of the trigonometric ratio or the value of the trigonometric ratio represents the solution of the trigonometric equation.

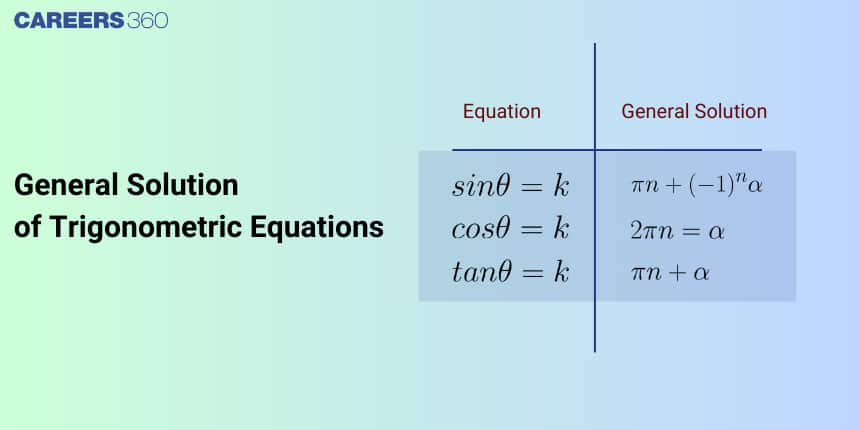
General Solution of some Standard Equations

1. $\sin \theta=\sin \alpha$

$
\begin{aligned}
& \text { Given, } \sin \theta=\sin \alpha \Rightarrow \sin \theta-\sin \alpha=0 \\
& \Rightarrow 2 \cos \frac{\theta+\alpha}{2} \sin \frac{\theta-\alpha}{2}=0 \\
& \Rightarrow \cos \frac{\theta+\alpha}{2}=0 \quad \text { or } \quad \sin \frac{\theta-\alpha}{2}=0 \\
& \Rightarrow \frac{\theta+\alpha}{2}=(2 \mathrm{n}+1) \frac{\pi}{2} \quad \text { or } \quad \frac{\theta-\alpha}{2}=\mathrm{n} \pi, \quad \mathrm{n} \in \mathbb{I} \\
& \Rightarrow \theta=(2 \mathrm{n}+1) \pi-\alpha \quad \text { or } \theta=2 \mathrm{n} \pi+\alpha, \quad \mathrm{n} \in \mathbb{I} \\
& \Rightarrow \theta=(\text { any odd multiple of } \pi)-\alpha \space \space ..... (i)\\
& \Rightarrow \theta=(\text { any even multiple of } \pi)+\alpha \space .....(ii)\\
& \text { from (i) and (ii) } \\
& \theta=\mathrm{n} \pi+(-1)^{\mathrm{n}} \alpha, \mathrm{n} \in \mathbb{I}
\end{aligned}
$

2. $\cos \theta=\cos \alpha$

$\begin{aligned} & \Rightarrow \cos \alpha-\cos \theta=0 \\ & \Rightarrow 2 \sin \frac{\alpha+\theta}{2} \sin \frac{\theta-\alpha}{2}=0 \\ & \Rightarrow \sin \frac{\alpha+\theta}{2}=0 \text { or } \sin \frac{\theta-\alpha}{2}=0 \\ & \Rightarrow \frac{\alpha+\theta}{2}=\mathrm{n} \pi \text { or } \frac{\theta-\alpha}{2}=\mathrm{n} \pi, \mathrm{n} \in \mathbb{I} \\ & \Rightarrow \theta=2 \mathrm{n} \pi-\alpha \text { or } \theta=2 \mathrm{n} \pi+\alpha, \mathrm{n} \in \mathrm{Z} \\ & \Rightarrow \theta=2 \mathrm{n} \pi \pm \alpha, \mathrm{n} \in \mathbb{I}\end{aligned}$

3. $\tan \theta=\tan \alpha$

$
\begin{aligned}
& \text { Given, } \tan \theta=\tan \alpha \\
& \Rightarrow \frac{\sin \theta}{\cos \theta}=\frac{\sin \alpha}{\cos \alpha} \\
& \Rightarrow \sin \theta \cos \alpha-\cos \theta \sin \alpha=0 \\
& \Rightarrow \sin (\theta-\alpha)=0 \\
& \Rightarrow \theta-\alpha=\mathrm{n} \pi \\
& \Rightarrow \theta=\mathrm{n} \pi+\alpha, \text { where } \mathrm{n} \in \mathbb{I}
\end{aligned}
$

Trigonometric Equation $f^2(x)=f^2(\alpha)$, where $\mathrm{f}(\mathrm{x})$ is trigonometric function

4. $\sin ^2 \theta=\sin ^2 \alpha$

$
\begin{aligned}
& \Rightarrow \sin ^2 \theta=\sin ^2 \alpha \\
& \Rightarrow \sin (\theta+\alpha) \sin (\theta-\alpha)=0
\end{aligned}
$
$\because$ we are using the identity, $\sin (A+B) \sin (A-B)=\sin ^2 A-\sin ^2 B$
$
\begin{aligned}
& \Rightarrow \sin (\theta+\alpha)=0 \text { or } \sin (\theta-\alpha)=0 \\
& \Rightarrow \theta+\alpha=n \pi \text { or } \theta-\alpha=n \pi, n \in \mathbb{I} \\
& \Rightarrow \theta=n \pi \pm \alpha \in \mathbb{I}
\end{aligned}
$

Note:

The general solution of the equation $\cos ^2 \theta=\cos ^2 \alpha$ and $\tan ^2 \theta=\tan ^2 \alpha$ is also $\theta=\mathrm{n} \pi \pm \alpha \in \mathbb{I}$.

Important Points to remember while solving trigonometric equations

While solving a trigonometric equation, squaring the equation at any step should be avoided as much as possible. If squaring is necessary, check the solution for values that do not satisfy the original equation.
Never cancel terms containing unknown terms on the two sides that are in the product. It may cause the loss of a genuine solution.
The answer should not contain such values of angles that make any of the terms undefined or infinite.
Domain should not change while simplifying the equation. If it changes, necessary corrections must be made.
Check that the denominator is not zero at any stage while solving the equations.

Summary

Solving a triangle entails utilizing geometric and trigonometric principles to determine the lengths of sides and measures of angles based on given information. By applying the Law of Sines, the Law of Cosines, and other relevant formulas, one can systematically find all unknown elements of the triangle, ensuring accuracy through the verification of angles and side lengths.

Solved Examples Based on Solution of Trigonometric Equations

Example 1: $
\text { Let } S=\left\{x \in\left(-\frac{\pi}{2}, \frac{\pi}{2}\right): 9^{1-\tan ^2 x}+9^{\tan ^2 x}=10\right\}\space {\text {and }} \mathrm{b}=\displaystyle\sum_{x \in \mathrm{S}} \tan ^2\left(\frac{\mathrm{x}}{3}\right) \text {, then } \frac{1}{6}(\beta-14)^2 \text { is }
$ is equal to: [JEE MAINS 2023]

1) 16

2) 32

3) 8

4) 64

Solution:

$\begin{aligned} & \text { Let } 9^{\tan ^2 x}=P \\ & \frac{9}{P}+P=10 \\ & P^2-10 P+9=0 \\ & (P-9)(P-1)=0 \\ & P=1,9 \\ & 9^{\tan ^2 x}=1,9^{\tan ^2 x}=9 \\ & \tan ^2 x=0, \tan ^2 x=1 \\ & x=0, \pm \frac{\pi}{4} \quad \therefore x \in\left(-\frac{\pi}{2}, \frac{p}{2}\right) \\ & \beta=\tan ^2(0)+\tan ^2\left(+\frac{\pi}{12}\right)+\tan ^2\left(-\frac{\pi}{12}\right) \\ & =0+2(\tan 15)^2 \\ & 2(2-\sqrt{3})^2 \\ & 2(7-4 \sqrt{3}) \\ & \text { Then } \frac{1}{6}(14-8 \sqrt{3}-14)^2=32\end{aligned}$

Hence, the answer is option 2.

Example 2: The set of all values $\lambda$ of for which the equation $\cos ^2 2 x-2 \sin ^4 x-2 \cos ^2 x=\lambda$ has a real solution $x$, is [JEE MAINS 2023]

1) $[-2,-1]$
2) $\left[-1,-\frac{1}{2}\right]$
3) $\left[-\frac{3}{2},-1\right]$
4) $\left[-2,-\frac{3}{2}\right]$

Solution:

$\begin{aligned} & \cos ^2 2 x-2\left(\frac{1-\cos 2 x}{2}\right)^2-(1+\cos 2 x)=\lambda \\ & \Rightarrow \quad \cos ^2 2 x-2\left(\frac{1-\cos ^2 2 x-2 \cos 2 x}{4}\right)-1-\cos 2 x=\lambda\end{aligned}$

Let $\cos 2 \mathrm{x}=\mathrm{t}$
$
\begin{aligned}
& \Rightarrow \quad 2 t^2-1-t^2+2 t-2-2 t=2 \lambda \\
& \Rightarrow \quad \mathrm{t}^2-3=2 \lambda \quad \because 0 \leq \mathrm{t}^2 \leq 1 \\
& \Rightarrow \quad \mathrm{t}^2=2 \lambda+3 \\
& 0 \leq 2 \lambda+3 \leq 1 \\
& -3 \leq 2 \lambda \leq-2 \\
& \frac{-3}{2} \leq \lambda \leq-1
\end{aligned}
$

Hence, the answer is the option 3.

Example 3: If $m$ and $n$ respectively are the numbers of positive and negative values of $q$ in the interval $[-p, p] \space {\text {that satisfy the equation }}$ $\cos 2 \theta \cos \frac{\theta}{2}=\cos 3 \theta \cos \frac{9 \theta}{2}$, then mn is equal to [JEE MAINS 2023]

1) 25

2) 20

3) 16

4) 27

Solution:

$\begin{aligned} & 2 \cos 2 \theta \cos \frac{\theta}{2}=2 \cos 3 \theta \cos \frac{9 \theta}{2} \\ & \cos \frac{5 \theta}{2}+\cos \frac{3 \theta}{2}=\cos \frac{15 \theta}{2}+\cos \frac{3 \theta}{2} \\ & \cos \frac{5 \theta}{2}-\cos \frac{15 \theta}{2}=0 \\ & \sin 5 \theta=0 \text { or } \sin \frac{5 \theta}{2}=0 \\ & \theta=\frac{\mathrm{n} \pi}{5} \text { or } \frac{2 \mathrm{n} \pi}{5} \\ & \theta=0, \pm \frac{\pi}{5}, \pm \frac{2 \pi}{5}, \pm \frac{3 \pi}{5}, \pm \frac{4 \pi}{5}, \pm \pi \\ & \mathrm{m}=\mathrm{n}=5 \\ & \mathrm{mn}=25\end{aligned}$

Hence, the answer is 25.

Example 4: The number of values of $\alpha$ in $[0,2 \pi]$ for which $2 \sin ^3 \alpha-7 \sin ^2 \alpha+7 \sin \alpha=2$, is :

1) 6
2) 4
3) 3
4) 1

Solution:

As we learned in

Trigonometric Equations -
The equations involving trigonometric functions of unknown angles are known as trigonometric equations.
wherein-
e.g. $\cos ^2 \theta-4 \cos \theta=1$
$2 \sin ^3 \alpha-7 \sin ^2 \alpha+7 \sin \alpha=2$
$\Rightarrow\left(2 \sin ^2 \alpha-5 \sin \alpha+2\right)(\sin \alpha-1)=0$
$\Rightarrow \sin \alpha=1$ or $\sin \alpha=\frac{5 \pm \sqrt{25-16}}{4}=\frac{8}{4}$
Hence, possible solutions are $\sin \alpha=1$ or $\frac{1}{2}$
Hence solutions are $\frac{\pi}{6}, \frac{\pi}{2}$ and $\frac{3 \pi}{6}$
Hence, the answer is the option 3.

Example 5: The number of elements in the set $S=\left\{\theta \in[0,2 \pi]: 3 \cos ^4 \theta-5 \cos ^2 \theta-2 \sin ^6 \theta+2=0\right\}\space {\text {is: }}$ [JEE MAINS 2023]

1) 10

2) 9

3) 8

4) 12

Solution:

$\begin{aligned} & 3 \cos ^4 \theta-5 \cos ^2 \theta-2 \sin ^6 \theta+2=0 \\ & \Rightarrow 3 \cos ^4 \theta-3 \cos ^2 \theta-2 \cos ^2 \theta-2 \sin ^6 \theta+2=0 \\ & \Rightarrow 3 \cos ^4 \theta-3 \cos ^2 \theta+2 \sin ^2 \theta-2 \sin ^6 \theta=0 \\ & \Rightarrow 3 \cos ^2 \theta\left(\cos ^2 \theta-1\right)+2 \sin ^2 \theta\left(\sin ^4 \theta-1\right)=0 \\ & \Rightarrow-3 \cos ^2 \theta \sin ^2 \theta+2 \sin ^2 \theta\left(1+\sin ^2 \theta\right) \cos ^2 \theta-1 \\ & \Rightarrow \sin ^2 \theta \cos ^2 \theta\left(2+2 \sin ^2 \theta-3\right)=0 \\ & \Rightarrow \sin ^2 \theta \cos ^2 \theta\left(2 \sin ^2 \theta-1\right)=0\end{aligned}$

Case 1: $\sin ^2 \theta=0 \rightarrow 3$ solution; $\theta=\{0, \pi, 2 \pi\}$

Case 2: $\cos ^2 \theta=0 \rightarrow 2$ solution $; \theta=$ $\left\{\frac{\pi}{2}, \frac{3 \pi}{2}\right\}$

Case 3: $\sin ^2 \theta=\frac{1}{2} \rightarrow 4$ solution $; \theta=\left\{\frac{\pi}{4}, \frac{3 \pi}{4}, \frac{5 \pi}{4}, \frac{7 \pi}{4}\right\}$

Hence, the answer is the option (2).

Frequently Asked Questions (FAQs)

1. What is the general solution of a trigonometric equation?

The general solution of a trigonometric equation is an expression that represents all possible solutions to the equation. It typically includes a variable (usually n) that represents any integer, allowing the solution to repeat infinitely due to the periodic nature of trigonometric functions.

2. How does the period of a trigonometric function affect its general solution?

The period of a trigonometric function determines the interval at which solutions repeat in the general solution. For example, sine and cosine have a period of 2π, so their general solutions often include terms like "2πn" where n is an integer.

3. What is the significance of the term "2πn" in many general solutions?

The term "2πn" (where n is an integer) represents complete rotations around the unit circle. Adding 2πn to any solution gives another valid solution because it completes a full rotation, bringing the function back to its starting value.

4. How do you find the general solution for sin(x) = 0?

The general solution for sin(x) = 0 is x = πn, where n is any integer. This is because sine equals zero at 0°, 180°, 360°, etc., which correspond to 0, π, 2π, etc. in radians.

5. What's the difference between a particular solution and the general solution?

A particular solution is a specific value that satisfies the equation, while the general solution represents all possible solutions. The general solution typically includes a variable (like n) that, when given specific integer values, generates all particular solutions.

6. Why do trigonometric equations often have infinite solutions?

Trigonometric equations often have infinite solutions because trigonometric functions are periodic. This means they repeat their values at regular intervals, causing the equation to be satisfied multiple times as the angle changes by multiples of 2π (or 360°).

7. Why is it important to consider the domain when solving trigonometric equations?

Considering the domain is crucial because trigonometric functions have restricted ranges. For example, sine and cosine are limited to [-1, 1]. Solutions outside this range are not valid, so the domain helps identify meaningful solutions.

8. How does the concept of reference angles relate to general solutions?

Reference angles help identify all angles that have the same trigonometric value. In general solutions, they're often represented by terms like "±arcsin(k)" or "π ± arccos(k)", which account for symmetrical angles in different quadrants.

9. What role does the CAST rule play in finding general solutions?

The CAST rule (Cosine, All, Sine, Tangent) helps determine in which quadrants a trigonometric function is positive. This is useful when expanding particular solutions into general solutions, ensuring all valid quadrants are included.

10. How do you approach solving equations involving multiple trigonometric functions?

To solve equations with multiple trigonometric functions, try to isolate one function using trigonometric identities. Then solve for that function and use inverse trigonometric functions to find the angle. Finally, consider all possible solutions to form the general solution.

11. How do you determine if a trigonometric equation has no solution?

A trigonometric equation has no solution if it requires the function to take on a value outside its range. For example, sin(x) = 2 has no solution because sine is always between -1 and 1.

12. How do you interpret the general solution x = arcsin(k) + 2πn in practical terms?

This general solution means that x can be any angle that has a sine value of k, plus any number of full rotations (2πn). The arcsin(k) gives the initial angle in the first quadrant, and adding 2πn accounts for all equivalent angles in subsequent rotations.

13. What's the relationship between the general solution and the unit circle?

The general solution represents all points on the unit circle that satisfy the equation. Each term in the general solution corresponds to a set of points that repeat around the circle at regular intervals.

14. How does solving tan(x) = k differ from solving sin(x) = k or cos(x) = k?

Solving tan(x) = k is different because tangent has a period of π (not 2π like sine and cosine). Its general solution typically includes "πn" instead of "2πn". Also, tangent doesn't have a restricted range, so k can be any real number.

15. Why is it important to verify solutions when solving trigonometric equations?

Verification is crucial because the solving process can sometimes introduce extraneous solutions or miss valid ones. Checking ensures that all solutions satisfy the original equation and no incorrect solutions are included.

16. How do you approach trigonometric equations with variables in the argument, like sin(ax + b) = k?

First, isolate the argument (ax + b) using inverse trigonometric functions. Then, solve for x and adjust the general solution to account for the coefficient 'a' and the constant 'b'.

17. How do you solve trigonometric equations involving reciprocal functions (csc, sec, cot)?

Convert the equation to use primary functions (sin, cos, tan) by applying reciprocal relationships. Then solve the resulting equation and form the general solution, being careful to exclude values that make the original reciprocal function undefined.

18. How do you approach solving systems of trigonometric equations?

Solve systems by using substitution or elimination methods, similar to algebraic systems. Often, using trigonometric identities to simplify equations is helpful. The general solution should satisfy all equations in the system simultaneously.

19. What role do graphical methods play in understanding general solutions?

Graphical methods provide visual insight into the nature and number of solutions. They can help verify the general solution by showing the periodic nature of solutions and identifying where functions intersect or reach certain values.

20. How do you approach trigonometric equations where the variable appears both inside and outside the trigonometric function?

For equations like xsin(x) = 1, try to isolate the trigonometric term on one side. Then use graphical methods or numerical techniques to find particular solutions, before generalizing to form the complete solution set.

21. Why is it sometimes necessary to use the "plus-minus" (±) symbol in general solutions?

The ± symbol is used to account for symmetrical solutions. For example, in sin(x) = k, both arcsin(k) and π - arcsin(k) are valid solutions in the principal period. The ± allows us to represent both these solutions concisely.

22. How do you approach solving trigonometric equations with double angles?

For equations with double angles (e.g., sin(2x)), first use double angle formulas to express the equation in terms of single angles. Then solve the resulting equation and adjust the general solution to account for the halved angle.

23. What's the significance of the principal value when finding general solutions?

The principal value is the solution in the primary interval of the inverse trigonometric function. It serves as the starting point for generating the general solution, which extends this value to all possible angles.

24. How do you solve a trigonometric equation of the form asin(x) + bcos(x) = c?

This type of equation can be solved by converting it to a single trigonometric function using the substitution method (R-formula) or by squaring both sides and using the Pythagorean identity. The general solution is then derived from the resulting equation.

25. Why is factoring important in solving some trigonometric equations?

Factoring is crucial for equations that can be written as a product of trigonometric terms. It allows you to set each factor to zero and solve separately, combining the results to form the general solution.

26. What's the connection between the general solution and the concept of periodicity?

The general solution directly reflects the periodicity of trigonometric functions. The term involving 'n' (like 2πn) represents the function's period, showing how solutions repeat at regular intervals.

27. Why is it sometimes necessary to restrict the domain when giving the general solution?

Domain restrictions are necessary when the equation or context limits the possible values of the variable. For example, in physical applications, angles might be restricted to a certain range, affecting the valid solutions.

28. How does the presence of constants inside trigonometric functions (e.g., sin(x+π/4) = 1/2) affect the general solution?

Constants inside trigonometric functions shift the graph horizontally. In the general solution, this shift is accounted for by subtracting the constant from the usual form. For example, x = π/4 + 2πn becomes x = -π/4 + 2πn.

29. Why is it important to consider the quadrants when forming general solutions?

Considering quadrants ensures all valid solutions are included. Different quadrants may have solutions with different signs or forms, and overlooking a quadrant can lead to incomplete general solutions.

30. What's the significance of the Pythagorean identity in solving trigonometric equations?

The Pythagorean identity (sin²(x) + cos²(x) = 1) is crucial for solving equations involving both sine and cosine. It allows you to express one function in terms of the other, often simplifying the equation.

31. How do you solve trigonometric equations involving inverse functions?

For equations with inverse functions (e.g., arcsin(x) = π/4), apply the forward function to both sides. This often leads to an equation in a standard form, which can then be solved using usual methods.

32. Why is it important to consider the range of inverse trigonometric functions when solving equations?

The range of inverse trigonometric functions is restricted (e.g., arcsin(x) is [-π/2, π/2]). Ignoring this can lead to missing or incorrect solutions, especially when the equation involves compositions of trigonometric and inverse trigonometric functions.

33. How do you approach solving trigonometric equations with mixed units (degrees and radians)?

Convert all angle measures to the same unit (preferably radians) before solving. After finding the general solution, you may convert back to the original units if required.

34. What strategies can you use to simplify complex trigonometric equations before solving?

Strategies include using trigonometric identities to rewrite expressions, factoring common terms, and applying angle addition or double angle formulas. The goal is to transform the equation into a more standard or recognizable form.

35. How do you interpret solutions to trigonometric equations in the context of real-world problems?

In real-world contexts, consider the practical meaning of the variable (e.g., angle, time, position). Often, you'll need to restrict the general solution to a specific range that makes sense in the given scenario.

36. What's the relationship between the general solution and the concept of congruent angles?

The general solution essentially describes all congruent angles that satisfy the equation. The term involving 'n' (like 2πn) represents the addition of complete rotations, which leads to congruent angles with the same trigonometric values.

37. How do you approach trigonometric equations where the variable appears in the amplitude?

For equations like asin(x) = 1 where 'a' is the variable, isolate 'a' first. Then consider the restrictions on 'a' based on the range of sine. The general solution might involve describing the possible values of 'a' rather than 'x'.

38. Why is it sometimes necessary to use logarithms in solving trigonometric equations?

Logarithms are useful in equations involving exponentials and trigonometric functions, such as e^(sin(x)) = 2. By taking logarithms of both sides, you can often simplify the equation to a standard trigonometric form.

39. How do you solve trigonometric equations involving infinite series?

For equations with infinite series (e.g., sin(x) + sin(x/2) + sin(x/4) + ... = 1), try to recognize patterns or use sum formulas. Often, these equations require advanced techniques and may not have closed-form general solutions.

40. What role does complex number theory play in understanding general solutions to trigonometric equations?

Complex number theory provides a deeper understanding of trigonometric functions and their solutions. It reveals connections between exponential and trigonometric functions (Euler's formula) and can sometimes simplify the process of finding general solutions.

41. How do you approach trigonometric equations where the variable appears in multiple frequencies?

For equations like sin(x) + sin(2x) = 0, try to use trigonometric identities to express the equation in terms of a single frequency. If this isn't possible, consider using substitution methods or numerical techniques to find solutions.

42. Why is it important to consider the behavior of trigonometric functions as x approaches infinity when forming general solutions?

Understanding the behavior as x approaches infinity helps in recognizing patterns in the solutions. It reinforces the periodic nature of trigonometric functions and explains why general solutions often involve terms like 2πn that repeat infinitely.

43. How do you solve trigonometric equations involving products of three or more functions?

For equations like sin(x)cos(x)tan(x) = 1, try to simplify using trigonometric identities. Often, you can reduce the product to a single function or a simpler combination of functions. Then solve the resulting equation to find the general solution.

44. What's the significance of the unit circle in deriving general solutions?

The unit circle provides a geometric interpretation of trigonometric functions and their solutions. It helps visualize periodic behavior, symmetry, and relationships between different functions, all of which are crucial in forming general solutions.

45. How do you approach solving trigonometric equations that arise from real-world scenarios, like simple harmonic motion?

Start by identifying the trigonometric model that describes the scenario. Then solve the equation as usual, but pay special attention to the context when interpreting the general solution. Often, you'll need to restrict solutions to a meaningful range.

46. Why is it important to understand the concept of odd and even functions when solving trigonometric equations?

Understanding odd and even properties helps in recognizing symmetries in solutions. For example, if f(x) is odd and f(x) = 0, then both x and -x are solutions. This can simplify the process of forming the general solution.

47. How do you solve trigonometric equations involving composite functions, like sin(cos(x)) = 1/2?

For composite functions, work from the outside in. In this case, first solve sin(y) = 1/2, then solve cos(x) = y. Combine these steps to form the general solution, being careful to consider the domains at each stage.

48. What strategies can you use to check if your general solution is complete and correct?

To verify a general solution: 1) Substitute it back into the original equation to ensure it satisfies for all integer values of n. 2) Check if it covers all possible solutions by considering different periods and quadrants. 3) Graph both sides of the equation to visually confirm the solutions.

49. How does the concept of linear independence relate to solving systems of trigonometric equations?

Linear independence is crucial when dealing with systems of trigonometric equations. If the equations are linearly dependent, the system may have infinitely many solutions or no solutions. Understanding this helps in determining whether a unique general solution exists.

50. Why is it important to consider the possibility of extraneous solutions when solving trigonometric equations?

Extraneous solutions can be introduced during the solving process, especially when squaring both sides or using certain trigonometric identities. Always verify solutions in the original equation to ensure the general solution doesn't include any invalid answers.