Graphs of General Trigonometric Functions

Graph of trignometric ratio depicts the behavior of trignometric function. It tells about its amplitude and period. In real life, we use the Graph of Trigonometric Function in the study of engines, waves, and the growth of animals and plants.

In this article, we will cover the concept of Graph of Trigonometric Function. This category falls under the broader category of Trigonometry, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Questions based on this topic have been asked frequently in JEE Mains.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

Trigonometric ratios

The trigonometric ratios of a given angle are the ratios of a right-angled triangle's sides with regard to any one of its acute angles.The six trigonometric ratios are sine (sin) , cosine (cos) , tangent(tan), cotangent(cot) , secant(sec) , cosecant(cosec) .

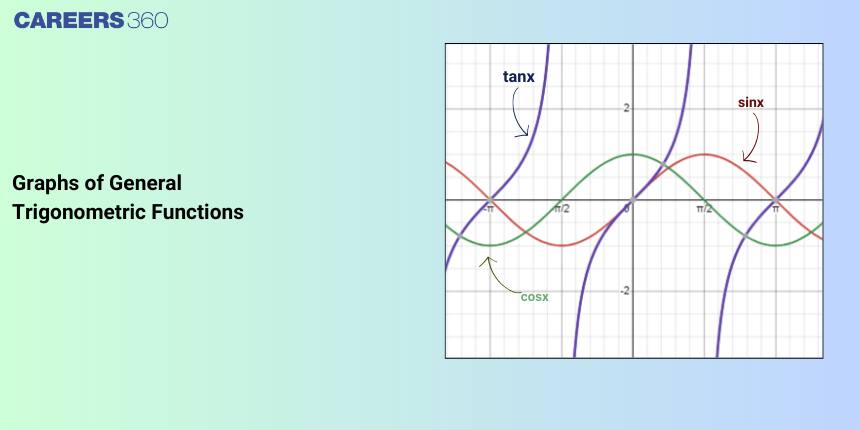
Graph of trignometric functions

The graph of trignometric functions is as follows,
Sine Function
Sine is the ratio of the length of the opposite side/perpendicular to the length of the hypotenuse to the given angle.

$
y=f(x)=\sin (x)
$

In the graph of sine functions the value of angles are taken on the $x$-axis while the values of $y=\sin x$ at each given angle are taken on the $Y$-axis.

Domain is R
Range is $[-1,1]$
We observe that $\sin x$ completes one full cycle of its possible values (from -1 to 1 ) in the interval of length $2 \pi$.
So, the period of $\sin x$ is $2 \pi$.
The graph drawn in the interval $[0,2 \pi]$ repeats to the right and the left.
$\operatorname{Sin} x$ passes the graph as $\sin x=0$
So, The root of $y=\sin x$ is $n \pi$ where $n=\ldots \ldots-3,-2,-1,0,1,2,3,4 \ldots \ldots$.
Min value of $\sin x=-1$ at $=3 \pi / 2$
The max value of $\sin x=1$ at $\pi / 2$
Amplitude $=1$

$
\sin (-x)=-\sin x
$

So, $\sin \mathrm{x}$ is an odd function.

Cosine Function

| Cosine is defined as the ratio of the length of the adjacent side/base to the length of the hypotenuse to the given angle.

$
y=f(x)=\cos (x)
$

In the graph of the cosine function, the values of angles (degrees) are taken on the $x$-axis while the values of $y=\cos x$ at each given angle are taken on the $Y$-axis.

$
\sin (x+90)=\cos x
$

So, the $\cos x$ graph is drawn by shifting the $\sin \mathrm{x}$ graph by 90 degrees.
Domain is $R$
Range is $[-1,1]$ $\cos x=\pi / 2$. So, The root of $y=\cos x$ is $n(\pi / 2)$ where $n=\ldots . .-3,-2,-1,1,2,3,4 \ldots \ldots$.

Min value of $\cos x=-1$ at $2 \pi$
The max value of $\cos x=1$ at 0 and $4 \pi$
Amplitude $=1$
Line of Symmetry $=$ Y- axis

$
\cos (-x)=\cos x
$

So, $\cos x$ is an even function

Tangent Function

The tangent function is the ratio of the length of the opposite side/perpendicular to the length of the adjacent side/base to the given angle.

$
y=f(x)=\tan (x)
$

In the graph of the tangent function, the values of angles (degrees) are taken on the $x$-axis while the values of $y=\tan x$ at each given angle are taken the $Y$-axis.

Domain is $\mathbb{R}-\left\{\frac{(2 \mathrm{n}+1) \pi}{2}, \mathrm{n} \in \mathbb{I}\right\}$
Range is $R$
We observe that $\tan x$ increase in each of the intervals ..... $(-3 \pi / 2,-\pi / 2),(-\pi / 2, \pi / 2),(\pi / 2,3 \pi / 2) \ldots \ldots$. The graph goes from negative to positive infinity. Period $=180$ degree $=\pi$. The root of the function $y=\tan x$ is $n \pi$ where $\mathrm{n}=\ldots . . .-3,-2,-1,1,2,3 \ldots \ldots$

The amplitude is undefined as the graph tends to infinity.
Line of symmetry $=$ origin

$
\tan (-x)=-\tan x
$

So, the tangent function is odd.

Cosecant Function

Cosecant is the ratio of the hypotenuse length to the length of the opposite side/perpendicular to the given angle.

$
y=f(x)=\operatorname{cosec}(x)
$

In the graph of the cosecant function, the values of angles (degrees) are taken on the X -axis while the values of $\mathrm{y}=\csc \mathrm{x}$ at each given angle are taken on the Y -axis.

Domain is $\mathrm{R}-\{\mathrm{n} \pi, \mathrm{n} \in \mathrm{I}$ (Integers) $\}$
Range is $R-(-1,1)$
As the curve repeats after an interval of $2 \pi$, the period of the cosecant function is $2 \pi$.
The amplitude of the graph of a cosecant function is undefined as the curve does not have a maximum or a minimum value and tends to infinity.
Line of symmetry= origin
Vertical asymptotes are $x=n \pi$

$
\operatorname{cosec}(-x)=-\operatorname{cosec} x
$

So, $\operatorname{cosec} x$ is an odd function.

Secant Function

Sec is the ratio of the length of the hypotenuse to the length of the adjacent side/base to the given angle.

$
y=f(x)=\sec (x)
$

In the graph of the secant function, the values of angles (degrees) are taken on the $x$-axis while the values of $y=\sec x$ at each given angle are taken on the $Y$-axis.

Domain is $\mathbb{R}-\left\{\frac{(2 \mathrm{n}+1) \pi}{2}, \mathrm{n} \in \mathbb{I}\right\}$
The range is $R-(-1,1)$
The amplitude of the graph of a secant function is undefined as the curve does not have a maximum or a minimum value and tends to infinity. As the curve repeats after an interval of $2 \pi$, the period of the sunction is $2 \pi$.
Line of Symmetry $=$ Y-axis
Vertical asymptotes $=\mathrm{x}=(2 \mathrm{n}+1) \pi / 2$
$\operatorname{Sec}(-x)=\sec x$
So, it is an even function

Cotangent Function

It is the ratio of the length of the adjacent side/base to the length of the opposite side/perpendicular to the given angle.

$
y=f(x)=\cot (x)
$

In the graph of the cotangent function, the values of angles (degrees) are taken on the $X$-axis while the values of $y=\cot \theta$ at each given angle are taken on the $Y$-axis.

Domain is $\mathrm{R}-\{\mathrm{n} \pi, \mathrm{n} \in \mathrm{I}$ (Integers) $\}$
Range is $R$
The graph goes from negative to positive infinity.nWe observe that $\cot x$ decreases in each of the intervals $\qquad$ $. .(-2 \pi /,-\pi),(-\pi, 0),(0, \pi)$ $\qquad$ The root of the equation $y=\cot x$ is $n \pi / 2$ where $n=$ $\qquad$ $-3,-2,-1,1,2,3$. $\qquad$ The amplitude is undefined as the graph tends to infinity.

As the curve repeats after an interval of $\pi$, the period of the cotangent function is $\pi$.
Line of symmetry $=$ origin
Vertical asymptotes are $x=n \pi$.
$\cot (-x)=-\cot x$
So it is an odd function.

Important Features of Graphs of Trigonometric Functions

Every trigonometric graph has important features: amplitude, vertical shift, period, phase, and phase shift.

Amplitude

Amplitude is half of the distance between the maximum value and the minimum value, or the height of the curve from the center line.

Vertical Shift

The displacement of the graph perpendicular to the x-axis is known as vertical shift.

Period

The period is the distance between the repetitions of any function.

Phase

The position of the waveform at a fraction of a period is referred to as its phase, and it is expressed in angles or radians.

Phase Shift

The displacement of the graph perpendicular to the y-axis is known as the phase shift

Graph of the General Form of Trigonometric Functions

The general form of a sine function is given as follows:

$
y=a \sin (b x+c)+d
$

Where,
|a| = Amplitude
$2 \pi /|b|=$ Period,
$\mathrm{c} / \mathrm{b}=$ Phase shift, and
$\mathrm{d}=$ Vertical shift.

How to Graph a Trigonometric Function?

To graph a trigonometric function, follow the steps mentioned below:
Step 1: To draw the graph of a trigonometric function, convert it into its general form, $y=a \sin (b x+c)+d$.
Step 2: Now, identify the different parameters such as amplitude, phase shift, vertical shift, and period.
Step 3: The value of the period is $2 \pi /|\mathrm{b}|$ for sine and cosine functions, whereas for tangent and cotangent functions it is $\pi /|\mathrm{b}|$. Phase shift $=-\mathrm{c} / \mathrm{b}$.
Step 4: Finally, plot the graph using the parameters calculated above.

Transformation of the graph of trignometric functions

1. The graph of $y=-f(x)$ from the graph of $y=f(x)$

Clearly, $\mathrm{f}(\mathrm{x})>0$ when $\mathrm{f}(\mathrm{x})<0$ and $-\mathrm{f}(\mathrm{x})<0$ when $\mathrm{f}(\mathrm{x})>0$.
So, graph $f y=-f(x)$ is obtained by flipping the graph of $y=f(x)$ upside down.
The period of function does not change in such transformation.
2. The graph of $f y=f(x)+$ a from the graph of $y=f(x)$

Graph $f y=f(x)+a$ is obtained by shifting the graph of $y=f(x)$, by a unit upward if $a>0$ and a unit downward if $a<0$.
The shape of the transformed graph remains the same.,
The shape of the transformed graph remains the same. So, the period does not change but the range changes.

Summary

Graphs of Trigonometric Functions enhance the understanding and application of trigonometry across various disciplines. They represent complex mathematical concepts, making them important in both theoretical studies and practical applications. Understanding graphs of trignometric functions helps us to solve various complex problems.

Recommended Video

Solved Examples Based on the Graph of Trigonometric Function

Example 1: If $\sqrt{3}\left(\cos ^2 x\right)=(\sqrt{3}-1) \cos x+1$, the number of solutions of the given equation when $x \in\left[0, \frac{\pi}{2}\right]$ is

Solution: In this question, we are going to relate the concept of trignometric function and quadratic equation,

$
\begin{aligned}
& \sqrt{3}(\cos x)^2-\sqrt{3} \cos x+\cos x-1=0 \\
& \Rightarrow(\sqrt{3} \cos x+1)(\cos x-1)=0 \\
& \left.\Rightarrow \cos x=1 \text { or } \cos x=-\frac{1}{\sqrt{3}} \text { ( reject }\right) \\
& \text { because } \cos x>0 \forall \mathrm{x} \in\left[0, \frac{\pi}{2}\right] \\
& \Rightarrow x=0 \text { only }
\end{aligned}
$

Hence, the answer is the number of solutions of the qiven equation is 1 .

Example 2: The number of solutions of the equation
$x+2 \tan x=\frac{\pi}{2}$ in the interval $[0,2 \pi]_{\text {is : }}$
[JEE MAINS 2021]
Solution

$
\begin{aligned}
& x+2 \tan x=\frac{\pi}{2} \\
& \Rightarrow 2 \tan x=\frac{\pi^2}{2}-x \\
& \Rightarrow \tan x=-\frac{1}{2} x+\frac{\pi}{4}
\end{aligned}
$

plot the graph

3 solution

Hence, the number of solutions in the equation is 3.

Exxample 3:The number of solutions of $\sin 3 x=\cos 2 x$, in the interval $\left(\frac{\pi}{2}, \pi\right)$ is :
[JEE MAINS 2018]

Solution: Graph of Trigonometric Ratios -

This is the graph of $y=\sin x$

and Graph of Trigonometric Ratios -

This is the graph of $y=\cos x$

Let’s join both the graph.

There is only one solution.

Hence the answer is 1.

Example 4: Find the number of points where $f(x)$ has its maximum value.

$
f(x)=|\sin (3 x)|+|\cos (3 x)| \quad x \epsilon[0, \pi]
$

Solution
Graph of Sine Function

$
y=f(x)=\sin (x)
$

Domain is $R$

Range is $[-1,1]$
Graph of Cosine Function

$
y=f(x)=\cos (x)
$

Domain is R

Range is $[-1,1]$
We know the maximum possible value of $f(x) \leq \sqrt{2}$ at $x=\frac{\pi}{12}$
We can see in the graph that there are 6 points where the graph of $|\sin (3 x)|$ and $|\cos (3 x)|$ intersects and has its maximum value.
Hence, the number of points where $f(x)$ has its maximum value is 6 .
Example 5: The number of roots of the equation, $(81)^{\sin ^2 x}+(81)^{\cos ^2 x}=30$ in the interval $[0, \pi]$ is equal to :
Solution

$
\begin{aligned}
& (81)^{\sin ^2 x}+(81)^{\cos ^2 x}=30 \\
& (81)^{\sin ^2 x}+\frac{(81)^1}{(18)^{\sin ^2 x}}=30 \\
& (81)^{\sin ^2 x}=t \\
& t+\frac{81}{t}=30 \\
& t^2-30 t+81=0 \\
& (t-3)(t-27)=0 \\
& (81)^{\sin ^2 x}=3^1 \quad \text { or } \quad(81)^{\sin ^2 x}=3^3 \\
& 3^{4 \sin ^2 x}=3^1 \quad \text { or } \quad 3^{4 \sin ^2 x}=3^3 \\
& \sin ^2 x=\frac{1}{4} \quad \text { or } \quad \sin ^2 x=\frac{3}{4}
\end{aligned}
$

Hence, The number of roots of the equation is 4 .