Greatest Binomial Coefficient

The Binomial Theorem is an important concept of algebra that helps to expand the expressions. A Binomial is an expression with two terms. It is difficult to solve the powers manually therefore this expression makes it simpler to solve. This theorem is widely used in real-life applications in mathematics including calculus etc.

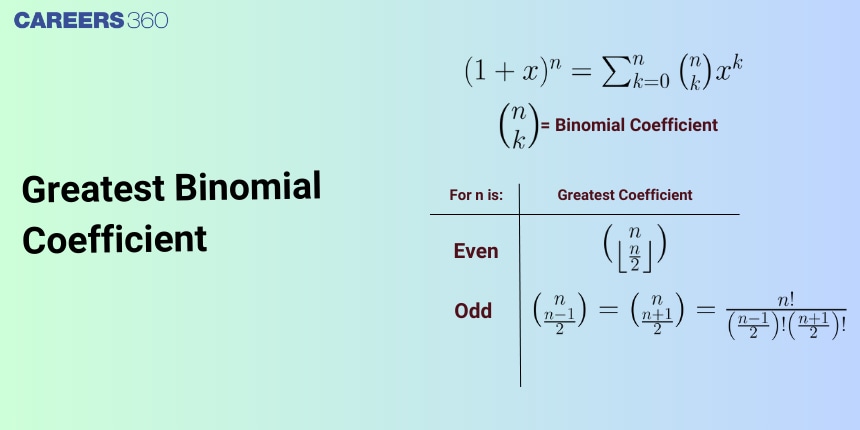
What is Binomial Expression?

An algebraic expression consisting of only two terms is called a Binomial Expression $e g \cdot(a+b)^2,\left(\sqrt{x}+\frac{k}{x^2}\right)^5,(x+9 y)^{-2 / 3}$

Binomial Theorem

If $n$ is any positive integer, then

$ (a + b)^n = \binom{n}{0} a^n + \binom{n}{1} a^{n - 1} b + \binom{n}{2} a^{n - 2} b^2 + \dots + \binom{n}{n - 1} a b^{n - 1} + \binom{n}{n} b^n $

Binomial Coefficient

The combination $\binom{n}{r}$ or ${ }^{\mathrm{n}} \mathrm{C}_{\mathrm{r}}$ occuring in the Binomial theorem is called a Binomial coefficient, where $\binom{n}{r}=C(n, r)={ }^n C_r=\frac{n!}{r!(n-r)!}$.

Numerically Greatest Value

The numerical value of each term of the binomial expansion is determined by the value of the Binomial coefficients. Numerically greatest value is defined as the largest term among the product of the variable coefficients(Binomial coefficients) in the Binomial expansion. In general, Numerically greatest value of the Binomial expansion of $(x+a)^n$ is the $r$th and $(r+1)$th term where $r=\frac{(n+1)}{1+|\frac{x}{a}|}$. It is represented as $T_{r}$ and $T_{r+1}$.

Method to find the Numerically Greatest Term of the expansion $(x+a)^n$

First, find the value of $r$ which is

$
\mathrm{r}=\frac{(n+1)}{1+|\frac{x}{a}|}
$

If $r$ is an integer, then $T_r$ and $T_{r+1}$ are numerically equal and both are greatest terms.

If $r$ is not an integer, then $T_{r+1}$ is the greatest term, where [r] is an integral part of r.

Recommended Video Based on Greatest Value:

Solved Examples Based on Greatest Value:

Example 1: The greatest value of the term independent of $x$ in the expansion of $\left(x \sin a+x^{-1} \cos a\right)^{10}$, is
1) $2^5$
2) $\frac{10!}{(5!)^2}$
3) $\frac{1}{2^5} \cdot \frac{10!}{(5!)^2}$
4) none of these

Solution

$
T_{r+1}={ }^{10} C_r(x \sin \alpha)^{10-r} \cdot\left(\frac{\cos \alpha}{x}\right)^r
$

It is independent of $x$ if $r=5$.
The term independent of $x={ }^{10} \mathrm{C}_5 \cdot \sin ^5 \mathrm{a} \cdot \cos ^5 \mathrm{a}$

$
={ }^{10} C_5 \cdot \frac{1}{2^5}(\sin 2 \alpha)^5 \leq{ }^{10} C_5 \cdot \frac{1}{2^5}
$

Hence, the answer is the option 3.

Example 2: $\ln n$ is an even positive integer, then the condition that the greatest term in the expansion of $(1+x)^n$ many have the greatest coefficient also, is ( x is positive)
1) $\frac{n}{n+2}<x<\frac{n+2}{n}$
2) $\frac{n+1}{n}<x<\frac{n}{n+1}$
3) $\frac{n}{n+4}<x<\frac{n+4}{4}$
4) none of these

Solution

Let $\mathrm{n}=2 \mathrm{~m}$
If $n$ is even then the greatest binomial coefficient $={ }^n C_{n / 2}={ }^{2 m} C_m$

$
=(m+1) \text { th term }=T_{m+1}
$
Now, since $T_{m+1}$ is the greatest term

$
m<\frac{(2 m+1)}{1+\left|\frac{1}{x}\right|}<(m+1)
$

Solving it and putting $m=n / 2$ we get

$
\frac{n}{n+2}<x<\frac{n+2}{n}
$
Hence, the answer is the option 1.

Example 3: If for some positive integer n , the coefficients of three consecutive terms in the binomial expansion of $(1+x)^{n+5}$ are in the ratio $5: 10: 14$, then the largest coefficient in this expansion is.
1) $462$
2) $330$
3) $792$
4) $252$

Solution

$
\begin{aligned}
& \text { Let } \mathrm{n}+5=\mathrm{N} \\
& { }^{\mathrm{N}} \mathrm{C}_{\mathrm{r}-1}:{ }^{\mathrm{N}} \mathrm{C}_{\mathrm{r}}:{ }^{\mathrm{N}} \mathrm{C}_{\mathrm{r}+1}=5: 10: 14 \\
& \Rightarrow \frac{{ }^{\mathrm{N}} \mathrm{C}_{\mathrm{r}}}{{ }^{\mathrm{N}_{\mathrm{C}}} \mathrm{C}_{\mathrm{r}-1}}=\frac{\mathrm{N}+1-\mathrm{r}}{\mathrm{r}}=2 \\
& \mathrm{~N}_{\mathrm{C}_{\mathrm{r}+1}}^{\mathrm{N}_{\mathrm{C}_{\mathrm{r}}}}=\frac{\mathrm{N}-\mathrm{r}}{\mathrm{r}+1}=\frac{7}{5} \\
& \Rightarrow \mathrm{r}=4, \mathrm{~N}=11 \\
& \Rightarrow(1+\mathrm{x})^{11}
\end{aligned}
$

Largest coefficient $={ }^{11} \mathrm{C}_6=462$
Hence, the answer is option (1).

Example 4: Find the numerically greatest term in the expansion of $(2+3 x)^9$, when $x=\frac{2}{3}$
1) $6^{\text {th }}$ term
2) $5^{\text {th }}$ term
3) $5^{\text {th }}$ term and $6^{\text {th }}$ term
4) $8^{\text {th }}$ term

Solution
Here $a=2$ and $b=3 x=2($ As $x=2 / 3)$

So,

$
m=\frac{n+1}{1+|a / b|}=\frac{10}{1+1}=5
$

As $m$ is an integer, so there are two numerically greatest terms
$T_m$ and $T_{m+1}: T_5$ and $T_6$
Hence, the answer is the option 3.
Example 5: Let for the $9^{\text {th }}$ term in the binomial expansion of $(3+6 x)^{\mathrm{n}}$, in the increasing powers of 6 x , $x=\frac{3}{2}$
$k+n_0$ is equal to :
1) $24$
2) $15$
3) $17$
4) $20$

Solution

$
\frac{\mathrm{n}+1}{1+\left|\frac{\mathrm{a}}{\mathrm{b}}\right|}=\frac{\mathrm{n}+1}{1+\left|\frac{3}{6 \times \frac{3}{2}}\right|}=\frac{3(\mathrm{n}+1)}{4}
$
As $g^{\text {th }}$ term is greatest,

$
\begin{aligned}
& \therefore 8<\frac{3(\mathrm{n}+1)}{4}<9 \\
& 32<3(\mathrm{n}+1)<36 \\
& 10.66<\mathrm{n}+1<12 \\
& \quad 9.66<\mathrm{n}<11 \\
& \therefore \mathrm{n}_0=10 \\
& \mathrm{k}=\frac{{ }^{10} \mathrm{C}_6 \cdot(3)^4(6)^6}{{ }^{10} \mathrm{C}_3 \cdot 3^7(6)^3}=14 \\
& \therefore \quad \mathrm{k}+\mathrm{n}_0=24
\end{aligned}
$
Hence, the answer is $24$ .