Half Angle Formula

The half-angle formula is used to find the value of the trigonometric ratios like 22.5°, 15°. half-angle of trigonometric functions with the help of an angle. These formulae can be derived from the reduction formulas and we can use them when we have an angle that is half the size of a special angle. It is used to find the exact value of the trigonometric ratios of 15 (half of 30 degrees), 22.5( half of 45 degrees), and so on.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. What are Half Angle Formula ?
2. Derivation of Half Angle Formulas
3. Solved Example Based on Half Angle Formula

In this article, we will cover the concept of the half-angle formula. This concept falls under the broader category of Trigonometry, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

What are Half Angle Formula ?

The half-angle formula is used to find the value of the trigonometric ratios of the angles like 22.5° (which is half of the angle 45°), 15° (which is half of the angle 30°), etc.

Half Angle Formulas

The half-angle formula can be derived with the help of the reduction formula.
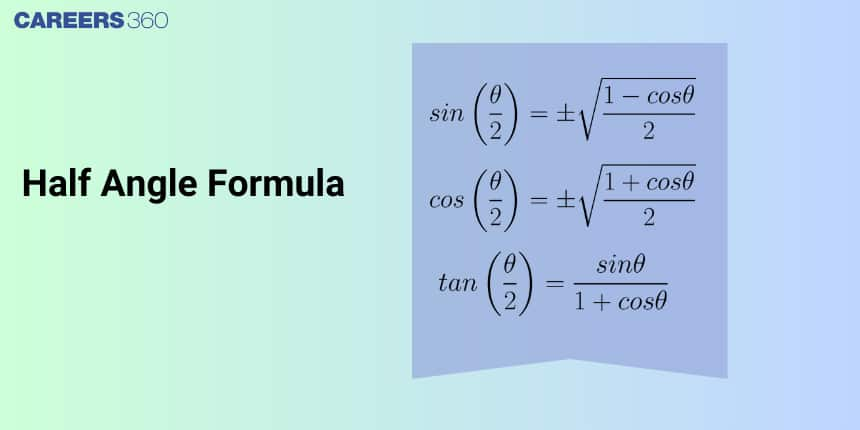
1. $\sin \left(\frac{\alpha}{2}\right)= \pm \sqrt{\frac{1-\cos \alpha}{2}}$
2. $\cos \left(\frac{\alpha}{2}\right)= \pm \sqrt{\frac{1+\cos \alpha}{2}}$
3. $\tan \left(\frac{\alpha}{2}\right)= \pm \sqrt{\frac{1-\cos \alpha}{1+\cos \alpha}}$

Note that the half-angle formulas are preceded by a $\pm$ sign. This does not mean that both positive and negative expressions are valid. Rather, only one sign needs to be taken and that depends on the quadrant in which $\alpha / 2$ lies.

Derivation of Half Angle Formulas

The half-angle formula can be derived with the help of the reduction formula.
Reduction formulas are:
$\sin ^2 \theta=\frac{1-\cos (2 \theta)}{2}$
$\cos ^2 \theta=\frac{1+\cos (2 \theta)}{2}$
$\tan ^2 \theta=\frac{1-\cos (2 \theta)}{1+\cos (2 \theta)}$

Derivation of Half Angle Formula for Sine

The half-angle formula for sine is derived as follows:

$
\begin{aligned}
\sin ^2 \theta & =\frac{1-\cos (2 \theta)}{2} \\
\sin ^2\left(\frac{\alpha}{2}\right) & =\frac{1-\cos \left(2 \cdot \frac{\alpha}{2}\right)}{2} \\
& =\frac{1-\cos \alpha}{2} \\
\sin \left(\frac{\alpha}{2}\right) & = \pm \sqrt{\frac{1-\cos \alpha}{2}}
\end{aligned}
$

Derivation of Half Angle Formula for Cosine

To derive the half-angle formula for cosine, we have

$
\begin{aligned}
\cos ^2 \theta & =\frac{1+\cos (2 \theta)}{2} \\
\cos ^2\left(\frac{\alpha}{2}\right) & =\frac{1+\cos \left(2 \cdot \frac{\alpha}{2}\right)}{2} \\
& =\frac{1+\cos \alpha}{2} \\
\cos \left(\frac{\alpha}{2}\right) & = \pm \sqrt{\frac{1+\cos \alpha}{2}}
\end{aligned}
$

Derivation of Half Angle Formula for Tangent

For the tangent identity, we have

$
\begin{aligned}
\tan ^2 \theta & =\frac{1-\cos (2 \theta)}{1+\cos (2 \theta)} \\
\tan ^2\left(\frac{\alpha}{2}\right) & =\frac{1-\cos \left(2 \cdot \frac{\alpha}{2}\right)}{1+\cos \left(2 \cdot \frac{\alpha}{2}\right)} \\
& =\frac{1-\cos \alpha}{1+\cos \alpha} \\
\tan \left(\frac{\alpha}{2}\right) & = \pm \sqrt{\frac{1-\cos \alpha}{1+\cos \alpha}}
\end{aligned}
$

Derivation of Half Angle Formula Using Semiperimeter

We can also represent the half-angle formula using the side of the triangle.

Derivation of Half-Angle Formula for Sine

$
\begin{aligned}
& \sin \frac{A}{2}=\sqrt{\frac{(s-b)(s-c)}{b c}} \\
& \sin \frac{B}{2}=\sqrt{\frac{(s-a)(s-c)}{a c}} \\
& \sin \frac{C}{2}=\sqrt{\frac{(s-a)(s-b)}{a b}}
\end{aligned}
$
We know that,

$
\cos \mathrm{A}=1-2 \sin ^2 \frac{\mathrm{A}}{2} \Rightarrow \sin ^2 \frac{\mathrm{A}}{2}=\frac{1-\cos \mathrm{A}}{2}
$
Now, for any $\triangle A B C, \cos A=\frac{b^2+c^2-a^2}{2 b c}$

Using the above two formulas

In a similar way, we can derive other formulas.

$
\begin{aligned}
& \sin ^2 \frac{A}{2}=\frac{1}{2}\left[1-\frac{b^2+c^2-a^2}{2 b c}\right] \\
& =\frac{1}{2}\left[\frac{2 b c-b^2-c^2+a^2}{2 b c}\right] \\
& =\frac{1}{2}\left[\frac{a^2-(b-c)^2}{2 b c}\right] \\
& =\frac{(\mathrm{a}-\mathrm{b}+\mathrm{c})(\mathrm{a}+\mathrm{b}-\mathrm{c})}{4 \mathrm{bc}} \\
& =\frac{(2 \mathrm{~s}-2 \mathrm{~b})(2 \mathrm{~s}-2 \mathrm{c})}{4 \mathrm{bc}} \quad[\because \mathrm{a}+\mathrm{b}+\mathrm{c}=2 \mathrm{~s}] \\
& \Rightarrow \sin ^2 \frac{\mathrm{A}}{2}=\frac{(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}{\mathrm{bc}} \\
& \text { As } 0<\frac{\mathrm{A}}{2}<\frac{\pi}{2} \text {, so } \sin \frac{\mathrm{A}}{2}>0 \\
& \sin \frac{\mathrm{A}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}{\mathrm{bc}}}
\end{aligned}
$

Derivation of Half-Angle Formula for Cosine

$
\begin{aligned}
& \cos \frac{\mathrm{A}}{2}=\sqrt{\frac{(\mathrm{s})(\mathrm{s}-\mathrm{a})}{\mathrm{bc}}} \\
& \cos \frac{\mathrm{B}}{2}=\sqrt{\frac{(\mathrm{s})(\mathrm{s}-\mathrm{b})}{\mathrm{ac}}} \\
& \cos \frac{\mathrm{C}}{2}=\sqrt{\frac{(\mathrm{s})(\mathrm{s}-\mathrm{c})}{\mathrm{ab}}}
\end{aligned}
$
We know that

$
\begin{aligned}
& \cos \mathrm{A}=2 \cos ^2 \frac{\mathrm{A}}{2}-1 \Rightarrow \cos ^2 \frac{\mathrm{A}}{2}=\frac{1+\cos \mathrm{A}}{2} \\
& \text { And for any } \triangle \mathrm{ABC}, \cos \mathrm{A}=\frac{\mathrm{b}^2+\mathrm{c}^2-\mathrm{a}^2}{2 \mathrm{bc}}
\end{aligned}
$

Using the above two formulas

$
\begin{aligned}
& \cos ^2 \frac{A}{2}=\frac{1}{2}\left[1+\frac{b^2+c^2-a^2}{2 b c}\right] \\
&=\frac{1}{2}\left[\frac{2 \mathrm{bc}+\mathrm{b}^2+\mathrm{c}^2-\mathrm{a}^2}{2 \mathrm{bc}}\right] \\
&=\frac{1}{2}\left[\frac{\left.(\mathrm{b}+\mathrm{c})^2-\mathrm{a}^2\right]}{2 \mathrm{bc}}\right] \\
&=\frac{(\mathrm{b}+\mathrm{c}+\mathrm{a})(\mathrm{b}+\mathrm{c}-\mathrm{a})}{4 \mathrm{bc}} \\
&=\frac{(\mathrm{b}+\mathrm{c}+\mathrm{a})(\mathrm{a}+\mathrm{b}+\mathrm{c}-2 \mathrm{a})}{4 \mathrm{bc}} \\
&=\frac{(2 \mathrm{~s})(2 \mathrm{~s}-2 \mathrm{a})}{4 \mathrm{bc}} \quad[\because \mathrm{a}+\mathrm{b}+\mathrm{c}=2 \mathrm{~s}] \\
& \Rightarrow \cos ^2 \frac{\mathrm{A}}{2}=\frac{(\mathrm{s})(\mathrm{s}-\mathrm{a})}{\mathrm{bc}}
\end{aligned}
$

As $0<\frac{\mathrm{A}}{2}<\frac{\pi}{2}$, so $\cos \frac{\mathrm{A}}{2}>0$

$
\cos \frac{\mathrm{A}}{2}=\sqrt{\frac{(\mathrm{s})(\mathrm{s}-\mathrm{a})}{\mathrm{bc}}}
$

In a similar way, we can derive other formulas

Derivation of Half Angle Formula for tan

This half-angle formula can be proved using tan x = sin x/cos x, and using the half-angle formula of sine and cosine.

$
\begin{aligned}
& \tan \frac{A}{2}=\sqrt{\frac{(s-b)(s-c)}{s(s-a)}} \\
& \tan \frac{B}{2}=\sqrt{\frac{(s-a)(s-c)}{s(s-b)}} \\
& \tan \frac{C}{2}=\sqrt{\frac{(s-a)(s-b)}{s(s-c)}}
\end{aligned}
$

This half-angle formula can be proved using $\tan x= \frac{\sin x}{\cos x}$ and using the half-angle formula of sine and cosine.

Recommended Video Based on Half Angle Formula

Solved Example Based on Half Angle Formula

Example 1: If $\tan \left(\frac{\pi}{9}\right), x, \tan \left(\frac{7 \pi}{18}\right)$ are in arithmetic progression and $\tan \left(\frac{\pi}{9}\right), \mathrm{y}, \tan \left(\frac{5 \pi}{18}\right)$ are also in arithmetic progression, then $|x-2 y|$ is equal to [JEE MAINS 2021]
Solution

$
\begin{aligned}
& x=\frac{\tan \left(\frac{\pi}{9}\right)+\tan \left(\frac{7 \pi}{18}\right)}{2} \text { and } \\
& y=\frac{\tan \left(\frac{\pi}{9}\right)+\tan \left(\frac{5 \pi}{18}\right)}{2}
\end{aligned}
$
$
\begin{aligned}
& \therefore x-2 y=\frac{1}{2}\left[\tan \left(\frac{\pi}{9}\right)+\tan \left(\frac{7 \pi}{18}\right)-2 \tan \left(\frac{\pi}{9}\right)-2 \tan \left(\frac{5 \pi}{18}\right)\right] \\
& =\frac{1}{2}\left[\tan \left(\frac{7 \pi}{18}\right)-\tan \left(\frac{\pi}{9}\right)-2 \tan \left(\frac{5 \pi}{18}\right)\right] \\
& =\frac{1}{2}\left[\tan \left(\frac{\pi}{2}-\frac{\pi}{9}\right)-\tan \left(\frac{\pi}{9}\right)-2 \tan \left(\frac{5 \pi}{18}\right)\right] \\
& =\frac{1}{2}\left[\cot \left(\frac{\pi}{9}\right)-\tan \left(\frac{\pi}{9}\right)-2 \tan \left(\frac{\pi}{2}-\frac{2 \pi}{9}\right)\right] \\
& =\frac{1}{2}\left[\frac{\cos \left(\frac{\pi}{9}\right)}{\sin \left(\frac{\pi}{9}\right)}-\frac{\sin \left(\frac{\pi}{9}\right)}{\cos \left(\frac{\pi}{9}\right)}-2 \cot \left(\frac{2 \pi}{9}\right)\right] \\
& =\frac{1}{2}\left[\frac{2 \cos (2 \pi / 9)}{\sin \left(\frac{2 \pi}{9}\right)}-2 \cot \left(\frac{2 \pi}{9}\right)\right] \\
& =0
\end{aligned}
$

Hence, the answer is 0

Example 2: The value of $2 \sin \left(\frac{\pi}{8}\right) \sin \left(\frac{2 \pi}{8}\right) \sin \left(\frac{3 \pi}{8}\right) \sin \left(\frac{5 \pi}{8}\right) \sin \left(\frac{6 \pi}{8}\right) \sin \left(\frac{7 \pi}{8}\right)$ is :
[JEE MAINS 2021]
Solution:

$
\begin{aligned}
& \text { As } \sin \left(\frac{5 \pi}{8}\right)=\sin \left(\pi-\frac{3 \pi}{8}\right)=\sin \left(\frac{3 \pi}{8}\right) \\
& \text { similarly } \sin \left(\frac{6 \pi}{8}\right)=\sin \left(\frac{2 \pi}{8}\right) \text { and } \sin \left(\frac{7 \pi}{8}\right)=\sin \left(\frac{\pi}{8}\right)
\end{aligned}
$

$\therefore$ Required value

$
\begin{aligned}
& =2 \cdot \sin ^2\left(\frac{\pi}{8}\right) \cdot \sin ^2\left(\frac{2 \pi}{8}\right) \cdot \sin ^2\left(\frac{3 \pi}{8}\right) \\
& =2 \cdot\left(\frac{1}{\sqrt{2}}\right)^2 \cdot \sin ^2\left(\frac{\pi}{8}\right) \cdot \sin ^2\left(\frac{3 \pi}{8}\right) \\
& =\sin ^2\left(\frac{\pi}{8}\right) \cdot \cos ^2\left(\frac{\pi}{8}\right) \\
& =\frac{4}{4} \sin ^2\left(\frac{\pi}{8}\right) \cdot \cos ^2\left(\frac{\pi}{8}\right) \\
& =\frac{1}{4}\left(\sin \left(\frac{\pi}{4}\right)\right)^2=\frac{1}{4} \cdot \frac{1}{2}=\frac{1}{8}
\end{aligned}
$
Hence, the answer is $1 / 8$

Example 3: If $\cos \alpha+\cos \beta=\frac{3}{2}$ and $\sin \alpha+\sin \beta=\frac{1}{2}$ and $\theta \quad$ is the arithmetic mean of $\alpha$ and $\beta$, then $\sin 2 \theta+\cos 2 \theta$ is equal to : [JEE MAINS 2015]
Solution:

Trigonometric Ratios of Submultiples of an Angle -

$
\begin{gathered}
\tan 2 \theta=\frac{2 \tan \theta}{1-\tan ^2 \theta} \\
\sin C+\sin D=2 \cdot \sin \left(\frac{C+D}{2}\right) \cos \left(\frac{C-D}{2}\right) \\
\cos C+\cos D=2 \cdot \cos \left(\frac{C+D}{2}\right) \cos \left(\frac{C-D}{2}\right)
\end{gathered}
$
This shows the transformation formulae and double angle formulae.
Therefore,

$
\begin{aligned}
& \cos \alpha+\cos \beta=\frac{3}{2} \Rightarrow 2 \cos \frac{\alpha+\beta}{2} \cos \frac{\alpha-\beta}{2}=\frac{3}{2} \\
& \sin \alpha+\sin \beta=\frac{1}{2} \Rightarrow 2 \sin \frac{\alpha+\beta}{2} \cos \frac{\alpha-\beta}{2}=\frac{1}{2}
\end{aligned}
$

dividing (2) by (1), we get

$
\tan \frac{(\alpha+\beta)}{2}=\frac{1}{3} \Rightarrow \tan \theta=\frac{1}{3}
$

$
\begin{aligned}
& \Rightarrow \tan 2 \theta=\frac{2 \times \frac{1}{3}}{1-\frac{1}{9}}=\frac{3}{4} \\
& \Rightarrow \sin 2 \theta=\frac{3}{5}, \quad \cos 2 \theta=\frac{4}{5}
\end{aligned}
$

[By making a triangle]
Thus,

$
\Rightarrow \sin 2 \theta+\cos 2 \theta=\frac{7}{5}
$
Hence, the answer is $7 / 5$

Example 4: Let $\alpha, \beta$ be such that $\pi<\alpha-\beta<3 \pi$. If $\sin \alpha+\sin \beta=-21 / 65$, and
$\cos \alpha+\cos \beta=-27 / 65$ then the value of $\cos \frac{\alpha-\beta}{2}$ is :

Solution:

$\sin \alpha+\sin \beta=\frac{-21}{65} \Rightarrow 2 \sin \frac{(\alpha+\beta)}{2} \cos \frac{(\alpha-\beta)}{2}=\frac{-21}{65}$ $\qquad$

$
\cos \alpha+\cos \beta=\frac{-27}{65} \Rightarrow 2 \cos \frac{(\alpha+\beta)}{2} \cos \frac{(\alpha-\beta)}{2}=\frac{-21}{65}
$
Squaring and adding,

$
\begin{aligned}
& 4 \cos ^2 \frac{(\alpha-\beta)}{2}\left[\sin ^2 \frac{\alpha+\beta}{2}+\cos ^2 \frac{\alpha+\beta}{2}\right]=\frac{21^2+27^2}{65^2} \\
& \Rightarrow \cos ^2 \frac{(\alpha-\beta)}{2}=\frac{1170}{4 \times 4225} \Rightarrow \cos ^2 \frac{(\alpha-\beta)}{2}=\frac{1170}{4 \times 4225}=\frac{9}{130} \\
& \Rightarrow \cos \frac{(\alpha-\beta)}{2}=\frac{-3}{\sqrt{130}} \\
& {\left[\because \pi<\alpha-\beta<3 \pi \Rightarrow \frac{\pi}{2} \leq \frac{\alpha-\beta}{2} \leq \frac{3 \pi}{2} \Rightarrow \cos \left(\frac{\alpha-\beta}{2}\right)<0\right]} \\
& \text { Hence, the answer is }-\frac{3}{\sqrt{130}}
\end{aligned}
$

Example 5: Find the range of functions $\tan \frac{\alpha}{2} \cdot \tan \alpha$
Solution:

$
\begin{aligned}
& \quad \tan \frac{\alpha}{2} \cdot \tan \alpha=\left(\frac{1-\cos \alpha}{\sin \alpha}\right) \cdot \frac{\sin \alpha}{\cos \alpha} \\
& =\sec \alpha-1 \\
& \text { Range of } \sec \alpha(-\infty,-1] U [ 1, \infty) \\
& \text { Range of } f(\alpha) \text { is }(-\infty,-2] U [0, \infty)
\end{aligned}
$

Hence, the answer is $(-\infty,-2] U [0, \infty)$