Indeterminate Forms of Limits

Limits are one of the most basic ideas in calculus, where one can learn how functions behave as they approach particular points. Of interest, though, is that some limits tend not to be as straightforward as finding the others, such that they could evaluate indeterminate forms. An indeterminate form in mathematics means that we cannot be able to determine the original value even after the substitution of the limits.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Limit of Indeterminate Form and Algebraic limit
2. Indeterminate List:
3. Solved Examples Based On Limits on Indeterminate Forms:
4. Summary

In this article, we will cover the concept of the Limit of Indeterminate Form and Algebraic limit. This topic falls under the broader category of Calculus, which is a crucial chapter in Class 11 Mathematics.This is very important not only for board exams but also for competitive exams, which even include Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of six questions have been asked on this topic in JEE Main from 2013 to 2023, one question in 2013, one question in 2015, two questions in 2019, one in 2020, and one in 2023.

Limit of Indeterminate Form and Algebraic limit

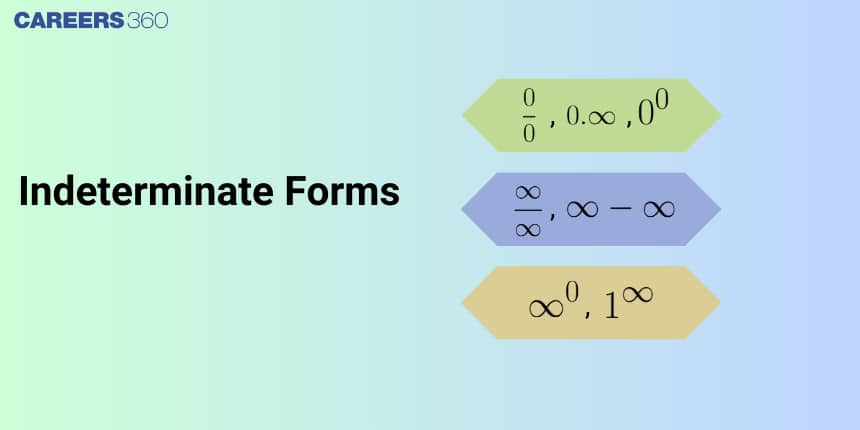
Indeterminate forms arise in limits when the standard limit rules yield expressions that do not directly lead to a specific value. If we directly substitute x = a in f(x) while evaluating $\lim\limits _{x \rightarrow a} f(x)$ and will get one of the seven following forms $\frac{0}{0}, \frac{\infty}{\infty}, \infty-\infty, 1^{\infty}, 0^0, \infty^0, \infty \times 0$ then it is called indeterminate form.

For example.
(i) $\lim\limits _{x \rightarrow 2} \frac{x^2-4}{x-2}=\frac{0}{0}$ indeterminate form.
(ii) $\lim\limits _{x \rightarrow 0} \frac{\sin x}{x}=\frac{0}{0}$ indeterminate form.
(iii) $\lim\limits _{x \rightarrow \pi / 2}(\tan x)^{\cos x}=\infty^0$ indeterminate form.

Note:

1. $\frac{0}{0}$ form means the numerator and denominator are both tending to $0$ (AND NOT exactly $0$ )
$\lim\limits _{ x \rightarrow 0^{+}} \frac{[x]}{x}$, when we input the values of $x$ close to $0($ and $x>0)$,then denominator is tending to $0$ , but numerator values are not tending to 0 BUT numerator is exactly $0$. So this is not $0$ form.

2. We can convert one indeterminate form into another and vice verse.

L’Hospital’s Rule

L'Hospital's Rule states that, if $\lim\limits _{x \rightarrow a} \frac{f(x)}{g(x)}$ is of $\frac{0}{0}$ or $\frac{\infty}{\infty}$ form , then differentiate numerator and denominator till this intermediate form is removed. $\lim\limits _{x \rightarrow a} \frac{f(x)}{g(x)}=\lim\limits _{x \rightarrow a} \frac{f^{\prime}(x)}{g^{\prime}(x)}$

But, if we again get the indeterminate form $\frac{0}{0}$ or $\frac{\infty}{\infty} \lim\limits _{x \rightarrow a} \frac{f(x)}{g(x)}=\lim\limits _{x \rightarrow a} \frac{f^{\prime}(x)}{g^{\prime}(x)}=\lim\limits _{x \rightarrow a} \frac{f^{\prime \prime}(x)}{g^{\prime \prime}(x)}$ (so we differentiate numerator and denominator again)
This process is continued till the indeterminate form $\frac{0}{0}$ or $\frac{\infty}{\infty}$ is removed.
Some Application of L'Hospital's Rule.
(i) $\lim\limits _{x \rightarrow 0} \frac{\sin x}{x}=\lim\limits _{x \rightarrow 0} \frac{\cos x}{1}=1$
(ii) $\lim\limits _{x \rightarrow \infty} \frac{\log _e x}{x}=\lim\limits _{x \rightarrow \infty} \frac{1 / x}{1}=0$
(iii) $\lim\limits _{x \rightarrow 0} \frac{\log _e(1+x)}{x}=\lim\limits _{x \rightarrow 0} \frac{\frac{1}{1+x}}{1}=1$

Indeterminate List:

1. $\frac{0}{0}$

Condition: Occurs when both the numerator and the denominator of a fraction approach zero as the independent variable approaches a certain value ccc.
Transformation: To resolve $\frac{0}{0}$, various techniques can be employed:
- Factorization: Factor the numerator and/or the denominator to cancel common factors.
- Rationalization: Multiply by a suitable form of 1 to simplify the expression.
- L'Hôpital's Rule: Differentiate the numerator and denominator separately if the limit exists.

Example: Evaluate $\lim\limits _{x \rightarrow 0} \frac{\sin x}{x}$

$
\lim\limits _{x \rightarrow 0} \frac{\sin x}{x}= \frac{0}{0}
$

But by L'Hospital rule, $\lim\limits _{x \rightarrow 0} \frac{\sin x}{x}=\lim\limits _{x \rightarrow 0} \frac{\cos x}{1}=1$

2. $\frac{\infty}{\infty}$

Condition: Occurs when both the numerator and the denominator of a fraction approach infinity as the independent variable approaches a certain value c .
Transformation: To resolve $\frac{\infty}{\infty}$, techniques include:
- L'Hôpital's Rule: Useful when both the numerator and denominator are differentiable functions near c.
- Rewriting: Factor out or manipulate terms to express the fraction in a more manageable form.

3. $0 \cdot \infty$

Condition: Occurs when a limit involves the product of a term approaching zero and another term approaching infinity.

Transformation: To resolve , convert it into a form amenable to limit laws:

- Rewrite: Express the limit in a way that allows separation or manipulation.

- Algebraic manipulation: Factor and simplify the expression.

Example: Evaluate

$
\lim\limits _{x \rightarrow 0} x \cdot \frac{1}{x} = 0.\infty$

$\lim\limits _{x \rightarrow 0} x \cdot \frac{1}{x} = \lim\limits _{x \rightarrow 0} 1 = 1
$

4. $\infty-\infty$

Condition: Occurs when a limit involves the difference of two terms, each approaching infinity.
Transformation: To resolve $\infty-\infty$, transform it into a more manageable form:
- Algebraic manipulation: Combine terms or rewrite the expression to reveal a $\frac{0}{0}$ or $\frac{\infty}{\infty}$ form.
- Factorization: Rearrange terms to simplify the expression.

Example: Evaluate $
\lim\limits _{x \rightarrow \infty}(x+\sqrt{x})-x$

$\lim\limits _{x \rightarrow \infty}(x+\sqrt{x})-x$ $=\infty -\infty$

$
\lim\limits _{x \rightarrow \infty}(x+\sqrt{x})-x: \lim\limits _{x \rightarrow \infty} \sqrt{x}=\infty
$

5. $0^0$

Condition: Occurs when a limit involves an expression of the form $x^x$ when $x \rightarrow 0$.
Transformation: To resolve $0^0$, consider using:
- Exponential and logarithmic techniques: Rewrite the expression using natural logarithms or exponentials to simplify the evaluation.

6. $\infty^0$

Condition: Occurs when a limit involves an expression of the form $\infty^0$.
Transformation: To resolve $\infty^0$, transform it into a form amenable to limit laws:
- Logarithmic manipulation: Use logarithms to simplify the expression.

7. $1^{\infty}$

Condition: Occurs when a limit involves an expression of the form $1^{\infty}$.
Transformation: To resolve $1^{\infty}$, consider using:

- Exponential and logarithmic techniques: Rewrite the expression using natural logarithms or exponentials to simplify the evaluation.

Recommended Video Based on Limits on Indeterminate Forms

Solved Examples Based On Limits on Indeterminate Forms:

Example 1: Let $f: R \rightarrow R_{\text {be a continuously differentiable function such that }} f(2)=6$ and $f^{\prime}(2)=\frac{1}{48} \int_6^{f(x)} 4 t^3 d t=(x-2) g(x) \lim\limits _6 g(x)$, then $x \rightarrow 2$ is equal to : [JEE Main 2018]

1) $18$

2) $24$

3) $12$

4) $36$

Solution:

L - Hospital Rule -

1-Hospital Rule -
In the form of $\frac{0}{0}$ and $\frac{\infty}{\infty}$ wedifferentiate $\frac{N^r}{D^r}$ separately.

$
\Rightarrow \lim\limits _{x \rightarrow a} \frac{f(x)}{g(x)}=\lim\limits _{x \rightarrow a} \frac{f^{\prime}(x)}{g^{\prime}(x)}
$

- wherein

$
\lim\limits _{x \rightarrow a} \frac{\frac{d}{d x} f(x)}{\frac{d}{d x} g(x)}
$

Where $f(x)$ and $g(x)=0$

Evaluation of limits : (algebraic limits) : (Method of direct substitution) -
$\lim\limits _{x \rightarrow a} f(x)$ defines by direct substituting $x=a$

$
e x: \lim\limits _{x \rightarrow 1} \frac{x^2+x+1}{x^2+x-1}=3
$

- wherein

Means at $x=a, f(x)$ defined.

$
\begin{aligned}
f: R \rightarrow R \quad f(2)=6 \\
\begin{aligned}
\lim\limits _{x \rightarrow 2} g(x) & =\lim\limits _{x \rightarrow 2} \frac{\int_6^{f(x)} 4 t^3 d t}{x-2} \\
& =\lim\limits _{x \rightarrow 2} \frac{4 \cdot f^3(x) \cdot f^{\prime}(x)}{1} \\
& =4 f^3(2) f^{\prime}(2)=\frac{1}{48} \\
& =4 \times(6)^3 \times \frac{1}{48} \\
& =6 \times 36 \times \frac{1}{12}=18
\end{aligned}
\end{aligned}
$

Hence, the answer is the option (1).

Example 2: $\lim\limits _{x \rightarrow 1} \frac{\int_0^{(x-1)^2} t \cdot \cos \left(t^2\right) d t}{(x-1) \sin (x-1)}$ is equal to? [JEE Main 2020]
1) $-\frac{1}{2}$
2) $\frac{1}{2}$
3) Does not exist
4) $0$

Solution:
Given,

$
\lim\limits _{x \rightarrow 1} \frac{\int_0^{(x-1)^2} t \cdot \cos \left(t^2\right) d t}{(x-1) \sin (x-1)}
$

Apply L'Hôpital's rule

$
\begin{aligned}
& \Rightarrow \lim\limits _{x \rightarrow 1} \frac{\int_0^{(x-1)^2} t \cdot \cos \left(t^2\right) d t}{(x-1) \sin (x-1)}=\lim\limits _{x \rightarrow 1} \frac{2(x-1)^3 \cos \left((x-1)^4\right)}{\sin (x-1)+(x-1) \cos (x-1)} \\
& \Rightarrow \lim\limits _{x \rightarrow 1} \frac{\int_0^{(x-1)^2} t \cdot \cos \left(t^2\right) d t}{(x-1) \sin (x-1)}=\lim\limits _{x \rightarrow 1} \frac{2(x-1)^2 \cos (x-1)^4}{\frac{\sin (x-1)}{(x-1)}+\cos (x-1)}=0
\end{aligned}
$
Hence, the answer is the option (4).

Example 3:

$\lim _{x \rightarrow 0} \frac{\sin ^2 x}{\sqrt{2}-\sqrt{1+\cos x}}$ equals
1) $
4 \sqrt{2}
$

2) $
\sqrt{2}
$

3) $
2 \sqrt{2}
$

4) $
4
$

Solution:

$
\lim _{x \rightarrow 0} \frac{\sin ^2 x}{\sqrt{2}-\sqrt{1+\cos x}}
$

normalizing :

$
\begin{aligned}
& : \frac{\left(\sin ^2 x\right)(\sqrt{2}+\sqrt{1+\cos x})}{(2-1-\cos x)} \\
& =\left(\sin ^2 x\right)=1-\cos ^2 x=(1-\cos x)(1+\cos x) \\
& \lim _{x \rightarrow 0} \frac{(1-\cos x)(1+\cos x)(\sqrt{2}+\sqrt{1+\cos x})}{(1-\cos x)} \\
& =\lim _{x \rightarrow 0}(1+\cos x)(\sqrt{2}+\sqrt{1+\cos x}) \\
& =(1+1)(\sqrt{2}+\sqrt{1+1}) \\
& =2(2 \sqrt{2}) \\
& =4 \sqrt{2}
\end{aligned}
$
Hence, the answer is the option 1.

Example 4: Which of the following is not indeterminate form when $x$ tends to zero?
$\frac{x+1}{x+2}$
2) $\frac{\sin x}{x}$
3) $\frac{\tan x}{x}$
4) $\frac{1-\cos x}{x^2}$

Solution: On direct substitution (A) gives $1 / 2$ while (B),(C).(D) are of form $\frac{0}{0}$
So $(A)$ is correct
Hence, the answer is the option 1.
Question 5 : Which of the following is not an indeterminate form when $x$ approaches zero?
1) $(1+x)^{1 / x}$
2) $(\cos x)^{1 / x^2}$
3) $(\tan (\pi / 4+x))^{1 / x}$
4) $(1+x)^{x+2}$

Solution:
(1). (2) and (3) all are of the form $1^{\infty}$, while (D) is directly known approaching i.e 1

So (4) is correct
Hence, the answer is the option 4.

Summary

Indeterminate forms can be evaluated by applying appropriate transformations—L'Hôpital's Rule, factorization, logarithmic manipulation—which further dispel the ambiguity. The development of this machinery not only enhances problem-solving skills in Calculus but also provides a deeper understanding of mathematical ideas paramount for later developments in many scientific and engineering disciplines.