Indirect Substitution in Integral

Integration by indirect substitution is one of the important parts of Calculus, which applies to measuring the change in the function at a certain point. Mathematically, it forms a powerful tool by which slopes of functions are determined, the maximum and minimum of functions found, and problems on motion, growth, and decay, to name a few. These concepts of integration have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main: Study Materials | High Scoring Topics | Preparation Guide

JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs

This Story also Contains

1. Indirect Substitution in Integral
2. Solved Examples Based on Indirect Substitution in Integral
3. Summary

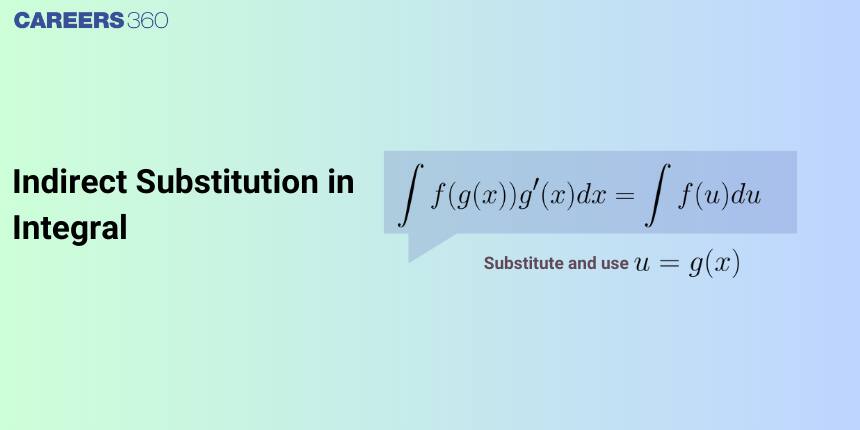
In this article, we will cover the concept of Integration by indirect substitution. This concept falls under the broader category of Calculus, which is a crucial Chapter in class 12 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

Indirect Substitution in Integral

Integration is the reverse process of differentiation. In integration, we find the function whose differential coefficient is given. The rate of change of a quantity y concerning another quantity x is called the derivative or differential coefficient of y about x. Geometrically, the Differentiation of a function at a point represents the slope of the tangent to the graph of the function at that point.

Substitution is one of the basic methods for calculating indefinite integrals. This technique transforms a complex integral into a simpler one by changing the variable of integration. It is especially useful for integrals involving composite functions where a direct integration approach is difficult.

Indirect Substitution involves transforming the integral into a form where we can use the properties of another integral. This technique is helpful when the integrand can be written as a product of functions, where one of the functions is an integral of the other.

Let’s go through some illustrations

Illustration 1: Evaluate $\int \frac{3{\mathrm{x}}^4+4{\mathrm{x}}^3}{{({\mathrm{x}}^4+\mathrm{x}+1)}^2}dx$
Here,

$\begin{array}{rl}I& =\int \frac{3x^4+4x^3}{{(x^4+x+1)}^2}dx=\int \frac{x^3(3x+4)}{x^8{(1+\frac{1}{x^3}+\frac{1}{x^4})}^2}dx\\ & =\int \frac{(\frac{3}{x^4}+\frac{4}{x^5})}{{(1+\frac{1}{x^3}+\frac{1}{x^4})}^2}dx\end{array}$
Put $1+\frac{1}{{\mathrm{x}}^3}+\frac{1}{{\mathrm{x}}^4}=\mathrm{t}$

$\begin{array}{rl}\Rightarrow & (-\frac{3}{{\mathrm{x}}^4}-\frac{4}{{\mathrm{x}}^5})dx=dt\\ \mathrm{I}& =-\int \frac{\mathrm{dt}}{{\mathrm{t}}^2}=\frac{1}{\mathrm{t}}+\mathrm{c}\\ & =\frac{1}{1+\frac{1}{{\mathrm{x}}^3}+\frac{1}{{\mathrm{x}}^4}}+c=\frac{{\mathrm{x}}^4}{{\mathrm{x}}^4+x+1}+c\end{array}$

Illustration 2: Evaluate $\int (x^{3m}+x^{2m}+x^m){(2x^{2m}+3x^m+6)}^{1/m}dx,x>0$ here $m$ is any natural number.
$\begin{array}{rl}& \text{ Here, }\mathrm{I}=\int ({\mathrm{x}}^{3\mathrm{m}}+{\mathrm{x}}^{2\mathrm{m}}+{\mathrm{x}}^{\mathrm{m}}){(2{\mathrm{x}}^{2\mathrm{m}}+3{\mathrm{x}}^{\mathrm{m}}+6)}^{1/\mathrm{m}}\mathrm{dx}\\ & =\int (x^{3m}+x^{2m}+x^m)\frac{{(2x^{3m}+3x^{2m}+6x^m)}^{1/m}}{x}dx\\ & =\int (x^{3m-1}+x^{2m-1}+x^{m-1}){(2x^{3m}+3x^{2m}+6x^m)}^{1/m}dx\\ & \text{ Put }2x^{3m}+3x^{2m}+6x^m=t\\ & \Rightarrow 6m(x^{3m-1}+x^{2m-1}+x^{m-1})dx=dt\end{array}$

$\therefore$ Eq. (i) becomes,

$\begin{array}{rl}& I=\int t^{1/m}\frac{dt}{6m}=\frac{1}{6m}\cdot \frac{t^{(1/m)+1}}{(1/m)+1}+C\\ & I=\frac{1}{6(m+1)}{[2x^{3m}+3x^{2m}+6x^m]}^{\frac{m+1}{m}}+C\end{array}$

Sometimes, to solve integration, it is useful to write the integral as a sum of two related integrals which can be evaluated by making suitable substitutions.

Some examples of algeebraic Twins are

$\begin{array}{c}\int \frac{2x^2}{x^4+1}dx=\int \frac{x^2+1}{x^4+1}dx+\int \frac{x^2-1}{x^4+1}dx\\ \int \frac{2}{x^4+1}dx=\int \frac{x^2+1}{x^4+1}dx-\int \frac{x^2-1}{x^4+1}dx\\ \int \frac{2x^2}{x^4+1+k{x}^2}dx,\int \frac{2}{(x^4+1+k{x}^2)}dx\end{array}$

Integration of the form:

1. $\int f(x+\frac{1}{x})(1-\frac{1}{x^2})dx$

Put $x+\frac{1}{x}=t\Rightarrow (1-\frac{1}{x^2})dx=dt$
2. $\int f(x-\frac{1}{x})(1+\frac{1}{x^2})dx$

Put $x-\frac{1}{x}=t\Rightarrow (1+\frac{1}{x^2})dx=dt$
3. $\int \frac{x^2+1}{x^4+k{x}^2+1}dx$

Divide numerator and denominator by $x^2$
4. $\int \frac{x^2-1}{x^4+k{x}^2+1}dx$

Divide numerator and denominator by $x^2$

Some Examples of trigonometric Twins are

$\begin{array}{rl}& \int \sqrt{\tan x}dx,\int \sqrt{\cot x}dx\\ & \int \frac{1}{{\sin }^{n}x+{\cos }^{n}x}dx,n=4,6\dots \\ & \int \frac{\pm \sin x\pm \cos x}{a\pm b\sin x\cos x}dx\end{array}$

Some Illustriation to see see how to solve such questions.
$\begin{array}{rl}& \text{ Illustration 1: }\text{ Evaluate }\int \sqrt{\tan x}dx\\ & \text{ put }\tan x=u^2\Rightarrow {\sec }^{2}xdx=2udu\\ & \Rightarrow \mathrm{dx}=\frac{2\mathrm{udu}}{1+{\mathrm{u}}^4}\\ & \therefore \mathrm{I}=\int \mathrm{u}\cdot \frac{2\mathrm{udu}}{1+{\mathrm{u}}^4}=\int \frac{2{\mathrm{u}}^2}{1+{\mathrm{u}}^4}\mathrm{du}\\ & =\int \frac{u^2+1}{u^4+1}du+\int \frac{u^2-1}{u^4+1}du\\ & =\int \frac{1+1/u^2}{u^2+1/u^2}du+\int \frac{1-1/u^2}{u^2+1/u^2}du\\ & =\int \frac{1+1/u^2}{(u-1/u{)}^2+2}du+\int \frac{1-1/u^2}{(u+1/u{)}^2-2}du\end{array}$
$\begin{array}{rl}\mathrm{I}& =\int \frac{\mathrm{ds}}{{\mathrm{s}}^2+(\sqrt{2}{)}^2}+\int \frac{\mathrm{dr}}{{\mathrm{r}}^2-(\sqrt{2}{)}^2}\\ [s=u& -\frac{1}{u}\text{ and }r=u+\frac{1}{u}]\\ & =\frac{1}{\sqrt{2}}{\tan }^{-1}\left(\frac{s}{\sqrt{2}}\right)+\frac{1}{2\sqrt{2}}\log \left|\frac{r-\sqrt{2}}{r+\sqrt{2}}\right|+c\\ & =\frac{1}{\sqrt{2}}[{\tan }^{-1}\left(\frac{u-1/u}{\sqrt{2}}\right)+\frac{1}{2}\log \left(\frac{u+\frac{1}{u}-\sqrt{2}}{u+\frac{1}{u}+\sqrt{2}}\right)]+C\end{array}$

substitute back $u=\sqrt{\tan x}$

Illustration 2: $$ Evaluate $\int \frac{1}{{\sin }^{4}x+{\cos }^{4}x}\mathrm{dx}$
Here, $I=\int \frac{1}{{\sin }^{4}x+{\cos }^{4}x}dx$
Dividing numerator and denominator by ${\cos }^{4}x$, we get

$\begin{array}{rl}& I=\int \frac{{\sec }^{4}x}{{\tan }^{4}x+1}dx\\ & I=\int \frac{{\sec }^{2}x(1+{\tan }^{2}x)}{1+{\tan }^{4}x}dx\end{array}$
Put

$\begin{array}{rl}\tan & x=u\Rightarrow {\sec }^{2}xdx=du\\ I& =\int \frac{u^2+1}{u^4+1}du\\ I& =\int \frac{1+1/u^2}{u^2+1/u^2}du=\int \frac{1+1/u^2}{(u-1/u{)}^2+2}du\end{array}$
Again, put $s=u-\frac{1}{u}$

$\therefore I=\int \frac{ds}{s^2+2}=\frac{1}{\sqrt{2}}{\tan }^{-1}\left(\frac{s}{\sqrt{2}}\right)+C$

Recommended Video Based on Indirect Substitution in Integral

Solved Examples Based on Indirect Substitution in Integral

Example 1: Evaluate $\int \frac{(x^2-1)dx}{(x^4+3x^2+1){\tan }^{-1}(x+\frac{1}{x})}$

1) $-\ln |{\tan }^{-1}(x-\frac{1}{x})|+c$
2) $\ln |{\tan }^{-1}(x-\frac{1}{x})|+c$
3) $\ln {\tan }^{-1}(x+\frac{1}{x})|+c$
4) $-\ln {\tan }^{-1}(x+\frac{1}{x})+c$

As we learned

Put $(x+\frac{1}{x})=t$

Integral can be written as

$\int \frac{(1-\frac{1}{x^2})dx}{[{(x+\frac{1}{x})}^2+1]{\tan }^{-1}(x+\frac{1}{x})}$

Let $(x+\frac{1}{x})=t$.

Differentiating we get $(1-\frac{1}{x^2})dx=dt$

Hence, $I=\int \frac{dt}{(t^2+1){\tan }^{-1}t}$

Now make one more substitution tan^-1t = u. Then

$\frac{dt}{t^2+1}=du$ and $\mathrm{I}=\int \frac{\mathrm{du}}{11}=\ln |u|+c$

$I=\ln |{\tan }^{-1}t|+c=\ln |{\tan }^{-1}(x+\frac{1}{x})|+c$

Hence, the answer is the option 3.

Example 2: The value of the integral $\int {(x+\frac{1}{x})}^{n+5}\left(\frac{x^2-1}{x^2}\right)dx$ is equal to

11) $\frac{{(x+\frac{1}{x})}^{n+6}}{n+6}+c$
2) ${\left(\frac{x^2+1}{x^2}\right)}^{n+6}(n+6)+c$
3) ${\left(\frac{x}{x^2+1}\right)}^{n+6}(n+6)+c$

4) None of these

Solution

As we learnt

We put $(x+\frac{1}{x})=t$

$I=\int p^{n+5}dp$ If $x+\frac{1}{x}=p$ then, $(1-\frac{1}{x^2})dx=dp$

$\begin{array}{rl}& \therefore I=\int {(x+\frac{1}{x})}^{n+3}\left(\frac{x^2-1}{x^2}\right)dx=\int p^{n+5}dp=\frac{p^{n+6}}{n+6}+c=\\ & \frac{{(x+\frac{1}{x})}^{n+6}}{n+6}+c\end{array}$

Hence, the answer is the option 1.

Example 3: The integral $\int \frac{dx}{(x+1{)}^{\frac{3}{4}}(x-2{)}^{\frac{5}{4}}}$ is equal to:

1) $4{\left(\frac{x+1}{x-2}\right)}^t+C$
2) $4{\left(\frac{x-2}{x+1}\right)}^t+C$
3) $-\frac{4}{3}{\left(\frac{x+1}{x-2}\right)}^{\frac{1}{4}}+C$
4) $-\frac{4}{3}{\left(\frac{x-2}{x+1}\right)}^{\frac{1}{4}}+C$

Solution

Integration by substitution -

$I=\frac{dx}{(x+1{)}^{\frac{3}{4}}(x-2{)}^{\frac{5}{4}}}$

$I=\int \frac{dx}{(x+1{)}^{\frac{3}{4}}(x-2{)}^{\frac{5}{4}}}\frac{(x-2{)}^{\frac{3}{4}}}{(x-2{)}^{\frac{3}{4}}}$

$=\int \frac{dx}{{\left(\frac{x+1}{x-2}\right)}^{\frac{3}{4}}(x-2{)}^2}$

Let $\frac{x+1}{x-2}=t$

Differentiating 1) on both sides

$\frac{(x-2)-(x+1)}{(x-2{)}^2}dx=dt$

$\Rightarrow \frac{dx}{(x-2{)}^2}=-\frac{dt}{3}$

Thus I= $\int \frac{-dt}{3t^{\frac{3}{4}}}$

$=\frac{-1}{3}\times \frac{1}{\frac{1}{4}}{t}^{\frac{1}{4}}+c$

$=-\frac{4}{3}{t}^{\frac{1}{4}}+c$

$I=-\frac{4}{3}{\left(\frac{x+1}{x-2}\right)}^{\frac{1}{4}}+c$

Hence, the answer is the option 3.

Example 4: For x>0, let $f(x)={\int }_1^x\frac{\log t}{1+t}dt$. Then $f(x)+f\left(\frac{1}{x}\right)$ is equalto

1) $\frac{1}{4}(\log x{)}^2$
2) $\frac{1}{2}(\log x{)}^2$
3) $\log x$
4) $\frac{1}{4}\log x^2$

Solution
$\begin{array}{rl}& f(x)={\int }_1^x\frac{\log t}{1+t}dx\\ & f\left(\frac{1}{x}\right)={\int }_1^{\frac{1}{2}}\frac{\log t}{1+t}dt\end{array}$
Put t= (substitution)

$\begin{array}{rl}& f\left(\frac{1}{x}\right)={\int }_1^1\frac{\log t}{1+t}dt={\int }_1^2\left(\frac{-\log z}{1+\frac{1}{z}}\right)\times \frac{-1}{z^2}dz\\ & ={\int }_1^z\left(\frac{+\log z}{1+z}\right)\times \frac{1}{z}dz\end{array}$

Substituting z = x in (i) we get f(x).

Thus,

$\begin{array}{rl}& f(x)+f\left(\frac{1}{x}\right)={\int }_1^x\frac{\log t}{1+t}(1+\frac{1}{t})dt\\ & ={\int }_1^x\frac{\log t}{t}dt\\ & =\frac{(\log x{)}^2}{2}-0\end{array}$

Hence, the answer is the option 2.

Example 5: If $\int \frac{\log (t+\sqrt{1+t^2})}{\sqrt{1+t^2}}dt=\frac{1}{2}(g(t){)}^2+C$ where C is a constant, then g(2) is equal to :

1) $2\log (2+\sqrt{5})$
2) $\log (2+\sqrt{5})$
3) $\frac{1}{\sqrt{5}}\log (2+\sqrt{5})$
4) $2^{\frac{1}{\log }(2+\sqrt{5}})$

Solution

Integration by substitution -

The functions when on substitution of the variable of integration to some quantity give any one of the standard formulas.

- wherein

Since $\int f(x)dx=\int f(t)dt=\int f(\theta )d\theta$ all variables must be converted into a single variable,$(\mathrm{tor}\theta )$

$\begin{array}{rl}& \int \frac{\log (t+\sqrt{1+t^2})}{\sqrt{1+t^2}}dt=\frac{1}{2}(g(t){)}^2+C\\ & LHS=\int \frac{\log (t+\sqrt{1+t^2})}{\sqrt{1+t^2}}dt\\ & \frac{1}{t+\sqrt{1+t^2}}\times (1+\frac{2t}{2\sqrt{1+t^2}})dt=dm\\ & \frac{dt}{\sqrt{1+t^2}}=dm\\ & \text{ we get }\int \log (t+\sqrt{1+t^2})=m\\ & \text{ LHS }=\frac{1}{2}[\log (1+\frac{m^2}{2}+C\\ & {\text{ So, }g(t)=\log (1+\sqrt{1+t^2})]}^2+C\\ & \text{ Put }t=2,\text{ we get }g(2)=\log (2+\sqrt{5})\end{array}$

Hence, the answer is the option 2.

Summary

Indirect substitution is a useful technique in integration that allows us to simplify and solve complex integrals. Mastery of integration is essential for progressing in algebra, calculus, and applied mathematics, offering valuable tools for both theoretical and practical problem-solving.