Integration as an Inverse Process of Differentiation
Integration is one of the important parts of Calculus, which applies to measuring the change in the function at a certain point. Mathematically, it forms a powerful tool by which slopes of functions are determined, the maximum and minimum of functions found, and problems on motion, growth, and decay, to name a few. These concepts of integration have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.
This Story also Contains
- Integration
- Standard Integration Formulae
- Solved Examples Based On Integration
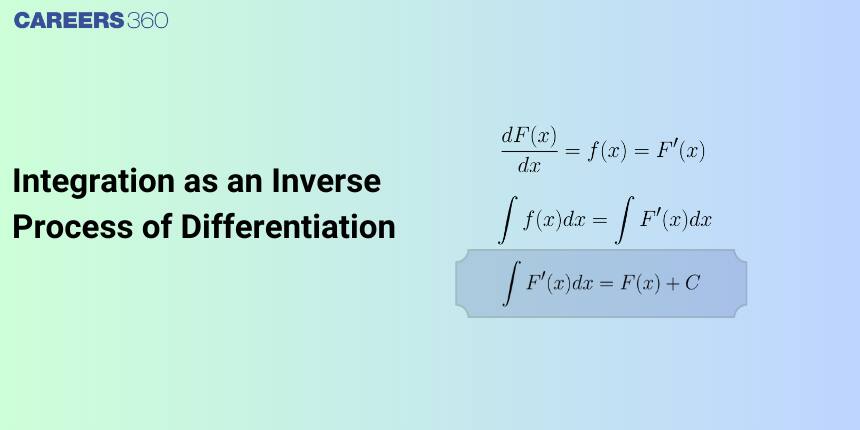
In this article, we will cover the concept of Integration. This concept falls under the broader category of Calculus, which is a crucial Chapter in class 12 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of seventeen questions have been asked on this concept, including one in 2014, one in 2018, six in 2019, four in 2020, three in 2021, and two in 2023.
Integration
Integration is the reverse process of differentiation. In integration, we find the function whose differential coefficient is given. The rate of change of a quantity y concerning another quantity x is called the derivative or differential coefficient of y concerning x. Geometrically, the Differentiation of a function at a point represents the slope of the tangent to the graph of the function at that point.
Integral is based on a limiting procedure which approximates the area of a curvilinear region by breaking the region into thin vertical slabs.”
For example,
$\begin{aligned} & \frac{d}{d x}(\sin x)=\cos x \\ & \frac{d}{d x}\left(x^2\right)=2 x \\ & \frac{d}{d x}\left(e^x\right)=e^x\end{aligned}$
In the above example, the function $\cos (x)$ is the derivative of $\sin (x)$. We say that $\sin (x)$ is an antiderivative (or an integral) of $\cos (x)$. Similarly, $x^2$ and $e^x$ are the antiderivatives (or integrals) of $2 x$ and $\mathrm{e}^{\mathrm{x}}$ respectively.
Also note that the derivative of a constant (C) is zero. So we can write the above examples as:
$\begin{aligned}
& \frac{d}{d x}(\sin x+c)=\cos x \\
& \frac{d}{d x}\left(x^2+c\right)=2 x \\
& \frac{d}{d x}\left(e^x+c\right)=e^x
\end{aligned}$
Thus, the anti-derivatives (or integrals) of the above functions are not unique. There exist infinitely many anti-derivatives of each of these functions which can be obtained by selecting C arbitrarily from the set of real numbers.
For this reason, C is referred to as an arbitrary constant. C is the parameter by varying which one gets different anti-derivatives (or integrals) of the given function.
If the function $F(x)$ is an antiderivative of $f(x)$, then the expression $F(x)+C$ is the indefinite integral of the function $f(x)$ and is denoted by the symbol $\int f(x) d x$.
By definition,
$\int \mathrm{f}(\mathrm{x}) \mathrm{dx}=\mathrm{F}(\mathrm{x})+\mathrm{c}$, where $\mathrm{F}^{\prime}(\mathrm{x})=\mathrm{f}(\mathrm{x})$ and 'c' is constant.
Here,
$\int \mathrm{f}(\mathrm{x}) \mathrm{dx}=\mathrm{F}(\mathrm{x})+\mathrm{c}$, where $\mathrm{F}^{\prime}(\mathrm{x})=\mathrm{f}(\mathrm{x})$ and ' $\mathrm{e}^{\prime}$ is constant.
Here,
| Symbols / Terms / Phrases | Meaning |
| $\int f(x) d x$ | Integral of $f$ with respect to $x$ |
| $f(x)$ in $\int f(x) d x$ | Integrand |
| $x$ in $\int f(x) d x$ | Variable of integration |
| An integral of $f$ | A function $F$ such that $F^{\prime}(x)=f(x)$ |
Here,
Rules of integration
f(x) and g(x) are functions with antiderivatives ∫ f(x) and ∫ g(x) dx. Then,
(a) $\int \mathrm{kf}(x) d x=k \int f(x) d x$ for any constant $k$.
(b) $\int(f(x)+g(x)) d x=\int f(x) d x+\int g(x) d x$
(c) $\int(f(x)-g(x)) d x=\int f(x) d x-\int g(x) d x$
Standard Integration Formulae
Since, $\frac{d}{d x}(F(x))=f(x) \Leftrightarrow \int f(x) d x=F(x)+c$
Based on this definition and various standard formulas (which we studied in Limit, Continuity, and Differentiability) we can obtain the following important integration formulae,
1. $\frac{\mathrm{d}}{\mathrm{dx}}(\mathrm{kx})=\mathrm{k} \Rightarrow \int \mathrm{kdx}=\mathrm{kx}+\mathrm{C}$, where k is a constant
2. $\frac{\mathrm{d}}{\mathrm{dx}}\left(\frac{\mathrm{x}^{\mathrm{n}+1}}{\mathrm{n}+1}\right)=\mathrm{x}^{\mathrm{n}}, \mathrm{n} \neq-1 \Rightarrow \int \mathrm{x}^{\mathrm{n}} \mathrm{dx}=\frac{\mathrm{x}^{\mathrm{n}+1}}{\mathrm{n}+1}+\mathrm{C}, \mathrm{n} \neq-1$
3. $\frac{\mathrm{d}}{\mathrm{dx}}(\log |\mathrm{x}|)=\frac{1}{\mathrm{x}} \Rightarrow \int \frac{1}{\mathrm{x}} \mathrm{dx}=\log |\mathrm{x}|+\mathrm{C}$, when $\mathrm{x} \neq 0$
4. $\frac{d}{d x}\left(e^x\right)=e^x \Rightarrow \int e^x d x=e^x+C$
5. $\frac{d}{d x}\left(\frac{a^x}{\log _e a}\right)=a^x, a>0, a \neq 1 \Rightarrow \int a^x d x=\frac{a^x}{\log _e a}+C$
Recommended Video Based on Integration
Solved Examples Based On Integration
Example 1: If a curve passes through the point $(1,-2)$ and has a slope of the tangent at any point $(x, y)$ on it as $\frac{x^2-2 y}{x}$, then the curve also passes through the point:
1) $(\sqrt{3}, 0)$
2) $(3,0)$
3) $(-1,2)$
4) $(-\sqrt{2}, 1)$
Solution
Equation of the tangent -
To find the equation of the tangent we need either one slope + one point or two points.
$
\therefore\left(y-y_0\right)=m\left(x_0-y_0\right)
$
or $\left(y-y_2\right)=\frac{y_2-y_1}{x_2-x_1}\left(x-x_2\right)$
where $\left(x_0, y_0\right)$ is the point on the curve
Geometrical interpretation of dy / dx -
$
\begin{aligned}
& \therefore \frac{d y}{d x}=\tan \theta \\
& \quad \frac{\mathrm{d} y}{\mathrm{~d} x}+\frac{2 y}{x}=x \\
& \text { If } e^{\int \frac{2}{x} d x}=e^{2 \ln x}=x^2 \\
& y\left(x^2\right)=\int x \cdot x^2 d x \\
& x^2 y=\frac{x^4}{4}+c \\
& \therefore y(1)=-2 \\
& \Rightarrow c=-\frac{9}{4}
\end{aligned}
$
$y=\frac{x^4-9}{4 x^2}$ which passes through $(\sqrt{3}, 0)$
Hence, the answer is the option (1).
Example 2: If the area (in sq. units) of the region $\left\{(x, y): y^2 \leq 4 x, x+y \leq 1, x \geq 0, y \geq 0\right\}$ is $a \sqrt{2}+b$, then $a-b$ is equal to :
1) $-\frac{2}{3}$
2) $\frac{10}{3}$
3) 6
4) $\frac{8}{3}$
Solution
Area between two curves -
If we have two functions intersection each other.First find the point of intersection. Then integrate to find area
$\int_o^a[f(x)-9(x)] d x$
- wherein

Indefinite integrals for Algebraic functions -
$\frac{\mathrm{d}}{\mathrm{d} x} \frac{\left(x^{\mathrm{n}+1}\right)}{n+1}=x^n \mathbb{m}^{\mathrm{j} 0} d x=\frac{x^{n+1}}{n+1}+c$
- wherein
Where $n \neq-1$
$\left\{(x, y): y^2 \leqslant 4 x ; x+y \leqslant 1, x \geqslant 0, y \geqslant 0\right\}$

$
\begin{aligned}
A & =\int_0^{3-2 \sqrt{2}} 2 \sqrt{x} d x+\frac{1}{2}(1-(3-2 \sqrt{2}))(1-(3-2 \sqrt{2})) \\
& =\frac{2\left[x^{\frac{3}{2}}\right]_0^{3-2 \sqrt{2}}}{\frac{3}{2}}+\frac{1}{2}(2 \sqrt{2}-2)(2 \sqrt{2}-2) \\
& =\frac{8 \sqrt{2}}{3}+\left(-\frac{10}{3}\right) \\
a & =\frac{8}{3}, b=\frac{-10}{3} \\
a & -b=\frac{8}{3}-\left(-\frac{10}{3}\right)=\frac{18}{3}=6
\end{aligned}
$
Example 3: The area (in sq. units) of the region $A=\left\{(x, y): x^2 \leq y \leq x+2\right\}$ is:
1) $\frac{10}{3}$
2) $\frac{9}{2}$
3) $\frac{31}{6}$
4) $\frac{13}{6}$
Solution
Area between two curves -
If we have two functions intersection each other.First find the point of intersection. Then integrate to find area
$
\int_a^b[f(x)-g(x)] d x
$
Here,
Point of intersection of $x^2=y$ and $y=x+2$ are $(-1,1)$ and $(2,4)$

$y=x+2$
Required Area,
$
\begin{aligned}
& =\int_{-1}^2\left(x+2-x^2\right) d x \\
& =\left[\frac{x^2}{2}+2 x-\frac{x^3}{3}\right]_{-1}^2 \\
& =\frac{9}{2}
\end{aligned}
$
Example 4: If the area ( in sq. units) bounded by the parabola $y^2=4 \lambda x$ and the line $y=\lambda x, \lambda>0$, is $\frac{1}{9}$, then $\lambda$ is equal to:
1) $2 \sqrt{6}$
2) 48
3) 24
4) $4 \sqrt{3}$
Solution
Area between two curves -
If we have two functions intersection each other. First find the point of intersection. Then integrate to find area
$\int_0^a[f(x)-9(x)] d x$
- wherein

Indefinite integrals for Algebraic functions -
$\frac{\mathrm{d}}{\mathrm{d} x} \frac{\left(x^{\mathrm{n}+1}\right)}{n+1}=x^n \int_{\mathrm{s}} x^n d x=\frac{x^{n+1}}{n+1}+c$
-wherein
Where $n \neq-1$

the parabola $y^2=4 \lambda x$ and the line $y=\lambda x$
If $\lambda>0$, then
$\begin{aligned}
\text { Area } & =\int_0^{4 / \lambda}(2 \sqrt{\lambda} \sqrt{x}-\lambda x) d x=\frac{1}{9} \\
& \Rightarrow\left[\frac{2 \sqrt{\lambda} x^{\frac{3}{2}}}{3 / 2}-\frac{\lambda x^2}{2}\right]_0^{4 / \lambda}=\frac{1}{9} \\
& =\frac{4}{3} \sqrt{\lambda} \frac{8}{\lambda^{\frac{1}{2}}}-\lambda \frac{8}{\lambda^2}=\frac{1}{9} \\
& \Rightarrow \frac{32}{3 \lambda}-\frac{8}{\lambda}=\frac{1}{9} \\
& \Rightarrow \frac{8}{3 \lambda}=\frac{1}{9} \\
& \Rightarrow \lambda=24
\end{aligned}$
$\text { If } f\left(\frac{x-4}{x+2}\right)=2 x+1, \quad(x \in \mathbb{R}-[1,-2])$
Example 5:
Then $\int f(x) d x$ equals
(where C is a constant of integration)
1) $12 \log _e|1-x|+3 x+C$
2) $-12 \log _e|1-x|-3 x+C$
3) $12 \log _c|1-x|-3 x+C$
4) $-12 \log _e|1-x|+3 x+C$
Solution
$\begin{aligned}
& \frac{x-4}{x+2}=y \Rightarrow x=\frac{2 y+4}{1-y} \\
& f(y)=2\left(\frac{2 y+4}{1-y}\right)+1=\frac{3 y+9}{1-y} \\
& \text { Now }_{\uparrow} f(x)=\frac{3 x+9}{1-x}
\end{aligned}$
Thus
$\begin{aligned}
& \int f(x) d x=\int \frac{3 x+9}{1-x} d x=\int\left(-3+\frac{12}{-x+1}\right) d x \\
& =-3 x-12 \ln |-x+1|+C
\end{aligned}$
Hence, the answer is the option 2.
Frequently Asked Questions (FAQs)
Integration is the reverse process of differentiation.
An integration of cos x is sin x.
In physics, integration is used to calculate quantities such as work, energy, and center of mass.
The rate of change of a quantity y concerning another quantity x is called the derivative or differential coefficient of y concerning x.
The other name of integration is antiderivative.