Intersection of Set, Properties of Intersection

Intersection of Sets in Set Theory

In set theory and discrete mathematics, a set is defined as a collection of distinct elements grouped together as a single unit. These elements can be numbers, letters, objects, or even real-life items. Among the basic set operations, one of the most important is the intersection of sets, which helps us identify similarities between groups.

The intersection of sets represents the common elements shared between two or more sets. Instead of combining everything like union, intersection keeps only the overlapping elements. This concept is widely used in Venn diagrams, probability, logic, and data analysis problems.

Intersection of Sets: Definition and Meaning

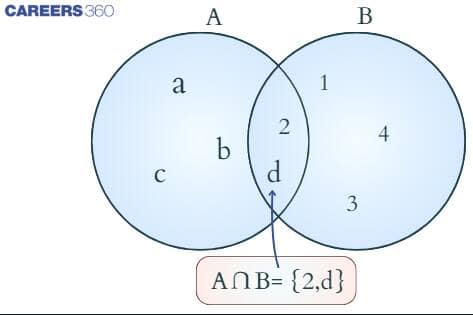
The intersection of sets $A$ and $B$ is the set of all elements that belong to both $A$ and $B$.

In simple words, it contains only the elements that are present in both sets at the same time.

Intersection of Sets Notation and Symbol

The symbol used for intersection is $\cap$

It is read as “intersection”.

Symbolically, $A \cap B = \{x : x \in A \text{ and } x \in B\}$

This means an element must belong to both $A$ and $B$ to be included in the result.

Disjoint Sets in Set Theory

If two sets have no common elements, their intersection becomes the empty set.

$A \cap B = \phi$

Such sets are called disjoint sets.

This means there is no overlap between them.

How to Find the Intersection of Sets Step-by-Step

Finding the intersection of sets is very straightforward if you follow a systematic approach.

Step 1: Write all given sets clearly.

Step 2: Compare the elements of each set.

Step 3: Select only the common elements.

Step 4: Place these common elements inside curly brackets.

Step 5: The resulting set is the intersection.

Think of it like this:
Union → combine everything
Intersection → keep only common

Examples of Intersection of Sets with Solutions

Example 1: Alphabet Sets

Let $A = \{i, a, f, h, s\}$
$B = \{f, m, s, h, a, i\}$

Common elements are $a, s, h, i, f$

So, $A \cap B = \{a, s, h, i, f\}$

Example 2: Numerical Sets

Let $A = \{2, 4, 6, 8\}$
$B = \{2, 3, 5, 8\}$

Common elements are $2$ and $8$

Thus, $A \cap B = {2, 8}$

Example 3: Disjoint Sets

Let $A = \{2, 4, 6, 8\}$
$B = \{1, 3, 5, 7\}$

There are no common elements.

So, $A \cap B = \phi$

Hence, $A$ and $B$ are disjoint sets.

Example 4: Intersection of Three Sets

Let $A = \{2, 4, 6, 8\}$
$B = \{2, 3, 8\}$
$C = \{2, 3, 5, 8\}$

Common elements in all three sets are $2$ and $8$

Therefore, $A \cap B \cap C = \{2, 8\}$

Cardinality of the Intersection of Sets Formula

The cardinal number of a set is the total number of elements present in the set. For example, if $\operatorname{Set} A=\{1,2,3,4,5,7,8\}$, then the cardinal number (represented as $\mathrm{n}(\mathrm{A})$ ) $=8$.

Consider two sets $A$ and $B$. Let $A=\{2,4,5,9,10,11,18,21\}, B=\{1,2,3,5,7,8,11,12,13\}$ and $A \cap B=\{2,5,11\}$, and the cardinal number of $A$ intersection $B$ is represented by $n(A \cap B)=3$.

The cardinality of $A \cap B$ can also be found by the formula, $n(A \cap B)=n(A)+$ $n(B)-n(A \cup B)$. Let's verify this formula for the above example, where $n(A)=8, n(B)=9$, and $A \cup B=\{1,2,3,4,5,6,7,8,10,11,12,13,14,21\}$. Note that $n(A \cup B)=14$ here. Then

$ \begin{aligned} & n(A \cap B)=n(A)+n(B)-n(A \cup B) \\ & n(A \cap B)=8+9-14 \\ & n(A \cap B)=3 \end{aligned} $

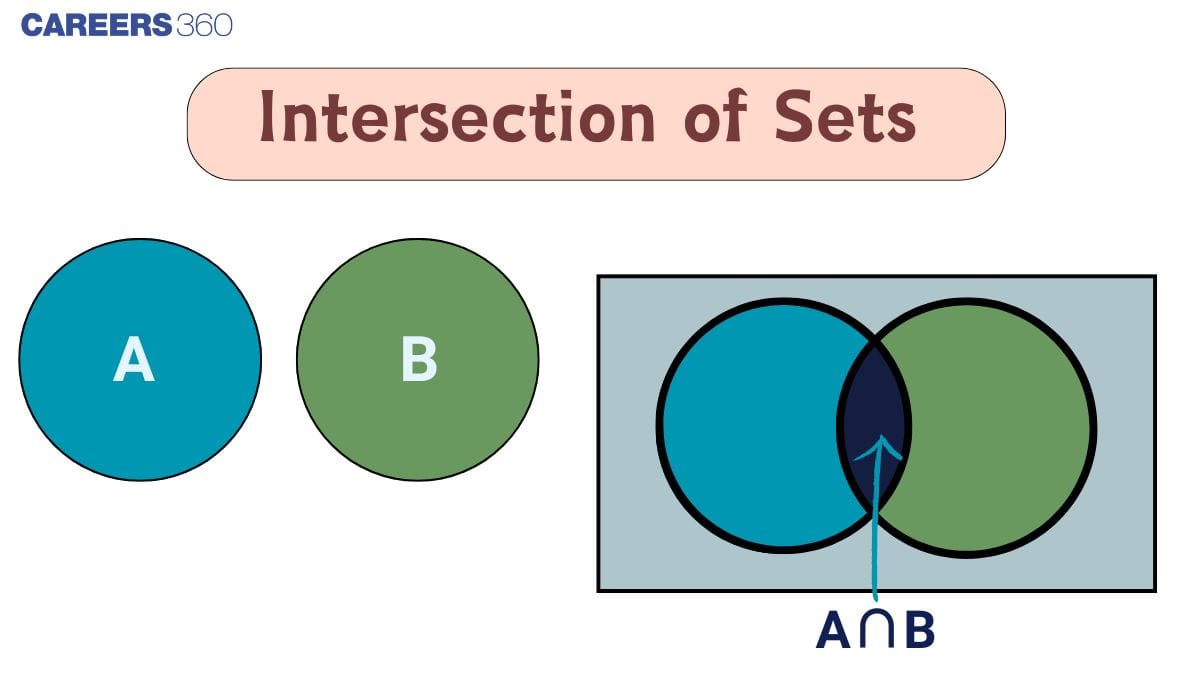
Venn Diagram for Intersection of Sets

Now, let us look into the Venn diagram of the intersection of sets.

Intersection of two sets

The intersection of two sets signifies that the common element is present in both sets.

Intersection of three sets

The intersection of three sets signifies that the common element is present in all three sets.

Properties of the Intersection of Sets

This section explains the key algebraic and logical properties that govern the intersection operation in set theory. Understanding these properties of the intersection of sets is essential for solving problems in mathematics, computer science, and data analysis. These rules help simplify complex expressions, especially when working with Venn diagrams, set identities, and Boolean algebra.

Commutative Property of Intersection

The commutative law of intersection states that the order in which two sets are intersected does not affect the result. That is:

$A \cap B = B \cap A$

This means the set of common elements remains the same regardless of the order. For example, if $A = \{1, 2, 3\}$ and $B = \{2, 3, 4\}$, then:

$A \cap B = \{2, 3\} = B \cap A$

This property is useful when rearranging set expressions for simplification.

Associative Property of Intersection

The associative property allows you to group sets in any order when performing multiple intersections:

$(A \cap B) \cap C = A \cap (B \cap C)$

This rule helps in solving complex problems where three or more sets are involved. For instance, with $A = \{1, 2\}$, $B = \{2, 3\}$, and $C = \{2, 4\}$, the intersection will always result in:

$A \cap B \cap C = \{2\}$

irrespective of how the sets are grouped.

Idempotent Property of Intersection

The idempotent law states that intersecting a set with itself returns the same set:

$A \cap A = A$

This shows that intersection is a stabilising operation; applying it repeatedly doesn't change the result. It's a fundamental property used in algebraic proofs and simplifying expressions in set theory and logic.

Identity and Domination Laws in Intersection

These two laws describe how intersection behaves with the universal set and the empty set:

• Identity Law: $A \cap U = A$
  where $U$ is the universal set. Intersecting a set with the universal set leaves it unchanged.
• Domination Law: $A \cap \emptyset = \emptyset$
  where $\emptyset$ is the empty set. No elements are common between any set and the empty set, so the result is always empty.

These properties help in defining the boundaries of sets in both pure mathematics and real-world logic systems.

Distributive Laws Involving Union and Intersection

The distributive property connects the operations of union and intersection:

• Intersection distributes over union:
  $A \cap (B \cup C) = (A \cap B) \cup (A \cap C)$
• Union distributes over the intersection:
  $A \cup (B \cap C) = (A \cup B) \cap (A \cup C)$

These identities are crucial for simplifying complex set expressions, especially in topics like Boolean algebra, database querying, and logic gates.

Intersection with Empty Set and Universal Set

The behaviour of the intersection operation with these two special sets is:

• With an empty set:
  $A \cap \emptyset = \emptyset$
• With universal set: $A \cap U = A$

This gives the idea that the universal set acts as a neutral element, while the empty set acts as an absorbing element in set intersection.

Difference Between Union and Intersection of Sets

In set theory and discrete mathematics, union and intersection of sets are two fundamental set operations used to compare and combine groups of elements. While both operations work on multiple sets, their purpose is completely opposite.

The union of sets collects all elements from the given sets, whereas the intersection of sets selects only the elements that are common to all sets. Understanding this difference is essential for solving Venn diagram problems, probability questions, logical reasoning, and data classification tasks.

Let’s break it down clearly.

Union of Sets – Meaning

The union operation merges two or more sets into one larger set.

It includes:

• elements in set $A$

• elements in set $B$

• elements common to both

Mathematically,

$A \cup B = \{x : x \in A \text{ or } x \in B\}$

So basically, nothing is left out.

Intersection of Sets – Meaning

The intersection operation finds similarities between sets.

It includes: only elements that appear in both sets

Mathematically,

$A \cap B = \{x : x \in A \text{ and } x \in B\}$

Here, we keep only the overlapping elements.

Key Differences Between Union and Intersection

Example for Better Understanding

Let $A = \{1, 2, 3\}$
$B = \{3, 4, 5\}$

Union

$A \cup B = \{1, 2, 3, 4, 5\}$
(All elements combined)

Intersection

$A \cap B = \{3\}$
(Only common element)

Memory Trick

If you ever feel confused in exams, remember:

Union → everything together
Intersection → only common

Or simply:

• $\cup$ looks like a cup → collects everything

• $\cap$ looks like a cap → covers only the overlap

It works every time.

Real-Life Examples of Set Intersection

Some real-life examples of the intersection of sets are

• Consider two gardens $A$ and $B$. The first garden has the set of flowers $\{rose, tulip, sunflower, jasmine\}$. The second garden has the set of flowers $\{ lily, lotus, rose, tulip, daisy\}$. The intersection of sets of flowers in the gardens $A$ and $B$ is $\{rose, tulip\}$.
• Let us consider two genres of books in the library. Let $A$ be the set of books of the science fiction genre and $B$ be the set of books of the mystery genre. The intersection of $A$ and $B$ is the set of science fiction mystery books.
• Let $A$ be the set of all students who play football and $B$ be the set of all students who play cricket. Then the intersection of sets $A$ and $B$ is the set of all students who play both football and cricket.

Solved Examples Based On the Intersection of Sets

Example 1: If $A \cup B=P, A \cap B=Q, B \cap A=R$ and $B \cup A=S$, then which of the following is true?
1) $P=R$
2) $Q=R$
3) $Q=S$
4) $P=Q$

Solution:
According to the property,
$A \cap B=B \cap A$
$\mathrm{Q}=\mathrm{R}$.
Hence, the answer is option 2.

Example 2: Which of the following is the associative property of intersection?
1) $(A \cup B) \cup C=A \cup(B \cup C)$
2) $(A \cap B) \cup C=A \cup(B \cap C)$
3) $A \cap(B \cup C)=(A \cap B) \cup C$
4) $(A \cap B) \cap C=A \cap(B \cap C)$

Solution:
The Associative Property of an intersection is:
$A \cap(B \cap C)=(A \cap B) \cap C$
Hence, the answer is option 4.

Example 3: If $A=\phi$ and $A \cap B=C$, then which of the following is true?
1) $A=B$
2) $A=C$
3) $B=C$
4) $\mathrm{B}=\phi$

Solution:
As we have learned that $\phi \cap \mathrm{A}=\phi$
So, $C=A \cap B=\phi \cap B=\phi$
Thus $\mathrm{A}=\mathrm{C}$.
Hence, the answer is option 2.

Example 4: If $A$ and $B$ are equal sets, then which of the following is NOT true?
1) $A \cap B=A$
2) $A \cap B=B$
3) $A \cup B=A \cap B$
4) $A \cap B=\phi$

Solution:
$A \cap B=A \cap A=A$ : so option (1) is true
As $A=B$, so $A \cap B=A=B$ : so option (2) is true
Also, $A \cup B=A \cup A=A$
As $A \cap B=A$, so $A \cup B=A \cap B$: so option (3) is true.
Therefore, the incorrect option is 4.
Hence, the answer is option 4.

Example 5: Given $A \cap B=\{5,7,9\}, A \cap C=\{3,8,7\}$. Find the value of $A \cap(B \cup C)$
1) $\{7\}$
2) $\{3,8,7\}$
3) $\{3,5,7,8,9\}$
4) $\{5,7,9\}$

Solution:

$ \begin{aligned} & A \cap(B \cup C)=(A \cap B) \cup(A \cap C) \quad \text { [Using Distributive Property] } \\ & =\{5,7,9\} \cup\{3,8,7\} \\ & =\{3,5,7,8,9\} \end{aligned} $

Hence, the answer is option 3.

List of Topics Related to the Intersection of Sets

To understand the concept of the intersection of sets thoroughly, it’s important to explore related foundational topics in set theory. Concepts like roster and set builder forms, power sets, complements, and De Morgan's Laws provide the necessary background to master set operations. These interconnected topics not only support a deeper understanding of intersections but also strengthen problem-solving skills in mathematics and logic. In this section, you’ll find a list of key topics that are essential for grasping the intersection of sets and its properties.

NCERT Resources

Mastering the chapter on Sets in Class 11 Mathematics requires the right study materials. NCERT resources such as detailed solutions, concise revision notes, and exam-oriented exemplar problems help strengthen conceptual understanding and problem-solving skills. Whether you're preparing for school exams or competitive tests, these materials provide complete support for systematic learning. In this section, explore the most useful NCERT resources for Chapter 1: Sets.

NCERT Solutions for Class 11 Chapter 1 Sets

NCERT Notes for Class 11 Chapter 1 Sets

NCERT Exemplar for Class 11 Chapter 1 Sets

Practice Questions on the Intersection of Sets

Mastering any mathematical concept comes through continuous practice. To help strengthen your understanding of the topic, we have given below some practice questions on the Intersection of sets in mathematics. They will test your knowledge of formulas, important properties and general application of knowledge.

To practice questions based on the Intersection of Set - Practice Questions, click here.

You can practice the next topics of Sets below: