Inverse Trigonometric Function Formulas for Complementary Angles

Inverse trigonometric functions can be defined as the inverses of the basic trigonometric functions - sine, cosine, tangent, cotangent, secant, and cosecant. We know that trigonometric functions are periodic and hence, man-one in their actual domain. So, to define an inverse trigonometric function, we have to restrict its actual domain to make the function injective. In real life, we use the inverse trigonometric function for determining the depth of the hole or the angle of inclination.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Inverse Trigonometric Function Formulas for Complementary angles:
2. Summary
3. Understanding inverse functions based on complementary angles is crucial in various fields of mathematics, including calculus, algebra, and trigonometry, as they provide a way to reverse the operation of a function and solve equations involving functions and their inverses. The function f (f-1 ( x )), helps in solving many types of problems and makes our calculation easier.

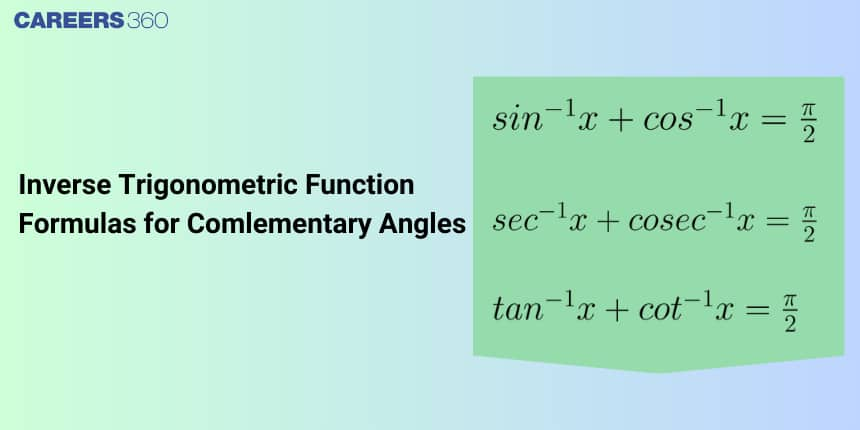
Inverse Trigonometric Function Formulas for Complementary angles:

To use inverse trigonometric functions, we need to understand that an inverse trigonometric function “undoes” what the original trigonometric function “does,” as is the case with any other function and its inverse. In other words, the domain of the inverse function is the range of the original function and vice versa.

For example, If $f(x)=\sin x$, then we would write $f^{-1}(x)=\sin ^{-1} x$. Be aware that $\sin ^{-1} x$ does not mean $1 / \sin x$.. The following examples illustrate the inverse trigonometric functions:

1. $\sin (\pi / 6)=1 / 2$, then $\pi / 6=\sin ^{-1}(1 / 2)$
2. $\cos (\pi)=-1$, then $\pi=\cos ^{-1}(-1)$
3. $\tan (\pi / 4)=1$, then $(\pi / 4)=\tan ^{-1}(1)$

We know that the inverse of a function is defined when the function is one-one and onto. But we also know that trigonometric functions are periodic and hence many-one in their domain.

So, to make the inverse of trigonometric functions to be defined, the actual domain of trigonometric function must be restricted to make it a one-one function and its co-domain should be restricted to make it an onto function.

The domain of the sine function is R and the range is [-1, 1]. If we restrict its domain to$[-\pi / 2, \pi / 2]$ then it becomes one-one and if we restrict its co-domain to [-1,1], then it becomes onto. So for this new domain and range, the inverse of our function y = sin(x) is defined. Domain for this inverse function y = sin^-1(x) will be a range of$y=\sin (x):[-1,1]$ and its range will be equal to the domain of$y=\sin (x):[-\pi / 2, \pi / 2]$

The sine function can be restricted to any of the intervals $[-3 \pi / 2,-\pi / 2],[-\pi / 2, \pi / 2],[\pi / 2,3 \pi / 2]$ and so on. It becomes one-one in all these intervals and it is also onto (if codomain is [-1,1]). We can, therefore, define the inverse of the sine function in each of these intervals. But by convention, we take the domain as $[-\pi / 2, \pi / 2]$, and this domain is called the Principal domain/ Principal value branch of $y=\sin (x)$..

So if $f[-\pi / 2, \pi / 2] \rightarrow[-1,1]$ and $f(x)=\sin (x)$, then its inverse is

$
[-1,1] \rightarrow[-\pi / 2, \pi / 2] \text { and } f^{-1}(x)=\sin ^{-1}(x)
$

In a similar way, we define other trigonometric functions by restricting their domain and co-domains.

So, the range of the inverse trigonometric function is the restricted domain of the corresponding trigonometric function.

1. $\sin ^{-1} x+\cos ^{-1} x=\frac{\pi}{2}$, for all $x \in[-1,1]$
2. $\tan ^{-1} x+\cot ^{-1} x=\frac{\pi}{2}$, for all $x \in R$
3. $\sec ^{-1} x+\operatorname{cosec}^{-1} x=\frac{\pi}{2}$ for all $x \in(-\infty,-1) \cup[1, \infty)$

Proof:

$
\begin{aligned}
& \text { Let } \sin ^{-1} x=\theta \\
& \text { where } \theta \in[-\pi / 2, \pi / 2] \\
& \Rightarrow \quad \begin{array}{l}
-\frac{\pi}{2} \leq-\theta \leq \frac{\pi}{2} \\
\Rightarrow \quad 0 \leq \frac{\pi}{2}-\theta \leq \pi \\
\Rightarrow \\
\quad \frac{\pi}{2}-\theta \in[0, \pi] \\
\text { Now, } \sin ^{-1} x=\theta \\
\text { Or, } \quad x=\sin \theta \\
\Rightarrow \quad x=\cos \left(\frac{\pi}{2}-\theta\right) \\
\Rightarrow \quad \cos ^{-1} \mathrm{x}=\frac{\pi}{2}-\theta
\end{array} \quad[\because \mathrm{x} \in[-1,1] \text { and }(\pi / 2-\theta) \in[0, \pi]] \\
& \Rightarrow \quad \theta+\cos ^{-1} \mathrm{x}=\frac{\pi}{2}
\end{aligned} \quad
$
From Eqs.(i) and (ii), we get $\sin ^{-1} x+\cos ^{-1} x=\pi / 2$.

Similarly, we get the other results

Summary

Understanding inverse functions based on complementary angles is crucial in various fields of mathematics, including calculus, algebra, and trigonometry, as they provide a way to reverse the operation of a function and solve equations involving functions and their inverses. The function f (f^-1 ( x )), helps in solving many types of problems and makes our calculation easier.

Recommended Video :

Solved Examples Based on Complementary Angles:

Example 1: If If $\cos ^{-1} x-\cos ^{-1} \frac{y}{2}=\alpha$ then $4 x^2-4 x y \cos \alpha+y^2$ is equal to:

1) 4

$\begin{aligned} & \text { 2) } 2 \sin 2 a \\ & \text { 3) }-4 \sin ^2 d \\ & \text { 4) } 4 \sin ^2 d\end{aligned}$

Solution

As we learned in

Formulae of Inverse Trigonometric Functions -

$\cos ^{-1} x+\cos ^{-1} y=\cos ^{-1}\left(x y-\sqrt{1-x^2} \sqrt{1-y^2}\right)$

- wherein

$x \geqslant 0, y \geqslant 0$

$\cos ^{-1} x-\cos ^{-1} \frac{y}{2}=\alpha$

$\Rightarrow \cos ^{-1}\left(\frac{x y}{2}+\sqrt{1-x^2} \sqrt{1-\frac{y^2}{4}}\right)=\alpha$

$\begin{aligned} & \Rightarrow \cos \alpha=\frac{x y}{2}+\sqrt{1-x^2} \sqrt{1-\frac{y^2}{4}} \\ & =\frac{1}{2}\left\{x y+\sqrt{1-x^2} \sqrt{4-y^2}\right\} \ldots \ldots(1)\end{aligned}$

Squaring, we get

$\begin{aligned} & \cos ^2 \alpha=\frac{1}{4}\left[x^2 y^2+\left(1-x^2\right)\left(4-y^2\right)+2 x y \sqrt{1-x^2} \sqrt{4-y^2}\right] \\ & =\frac{1}{4}\left[2 x^2 y^2-4 x^2-y^2+4\right]+\frac{x y}{2} \sqrt{1-x^2} \sqrt{4-y^2} \\ & \cos ^2 \alpha=1+\frac{1}{4}\left[2 x^2 y^2-4 x^2-y^2\right]+\frac{x y}{2} \sqrt{1-x^2} \sqrt{4-y^2} \\ & \Rightarrow 1-\cos ^2 \alpha=\frac{1}{4}\left[4 x^2+y^2-2 x^2 y^2\right]-\frac{x y}{2} \sqrt{1-x^2} \sqrt{4-y^2} \\ & \Rightarrow 4 \sin ^2 \alpha=4 x^2+y^2-2 x^2 y^2-2 x y \sqrt{1-x^2} \sqrt{4-y^2 \ldots \ldots(2)} \\ & =4 x^2+y^2-2 x^2 y^2-2 x y[2 \cos \alpha-x y \\ & \Rightarrow 4 \sin ^2 \alpha=4 x^2+y^2-4 x y \cos a\end{aligned}$

Hence, the answer is the option (4).

Example 2: Let $\tan ^{-1} y=\tan ^{-1} x+\tan ^{-1}\left(\frac{2 x}{1-x^2}\right)$ where $|x|<\frac{1}{\sqrt{3}}$

Then the value of is :

1) $\frac{3 x-x^3}{1-3 x^2}$
2) $\frac{3 x+x^3}{1-3 x^2}$
3) $\frac{3 x-x^3}{1+3 x^2}$ $\qquad$
4) $\frac{3 x+3}{1+3 x^2}$

Solution

$|x|<\frac{1}{\sqrt{3}} \Rightarrow \tan ^{-1} x<30^{\circ}, \tan ^{-1} \frac{2 x}{1-x^2}<60^{\circ}$

$\Rightarrow \tan ^{-1} y<90^{\circ}$

$\tan ^{-1} y=\tan ^{-1} x+\tan ^{-1} \frac{2 x}{1-x}$

$ =\tan ^{-1} x+2 \tan ^{-1} x$

$ =3 \tan ^{-1} x$
$
y=\tan \left(3 \tan ^{-1} x\right) \quad\left[\because \tan 3 x=\frac{3 \tan x-\tan ^3 x}{1-3 \tan ^2 x}\right]
$
$
\begin{aligned}
& =\frac{3 \tan \left(\tan ^{-1} x\right)-\left[\tan ^{\left.\left(\tan ^{-3} x\right)\right]}\right.}{1-3\left(\tan ^{-2} \tan ^{-1} x\right)^2} \\
& =\frac{3 x-x^3}{1-3 x^2}
\end{aligned}
$
Hence, the answer is the option 1.

Example 3: If $\cos ^{-1}\left(\frac{2}{3 x}\right)+\cos ^{-1}\left(\frac{3}{4 x}\right)=\frac{\pi}{2}\left(x>\frac{3}{4}\right)$, then is equal to:

1) $\frac{\sqrt{145}}{12}$

2) $\frac{\sqrt{ } 145}{10}$

3) $\frac{\sqrt{146}}{12}$

4) 4) $\frac{\sqrt{145}}{11}$

Solution

Given:

$\cos ^{-1}\left(\frac{2}{3 x}\right)+\cos ^{-1}\left(\frac{3}{4 x}\right)=\frac{\pi}{2}$

This can be written as:

$\cos ^{-1}\left(\frac{2}{3 x}\right)=\frac{\pi}{2}-\cos ^{-1}\left(\frac{3}{4 x}\right)$

$\Rightarrow \cos \left(\cos ^{-1}\left(\frac{2}{3 x}\right)\right)=\cos \left(\sin ^{-1}\left(\frac{3}{4 x}\right)\right)$

$\Rightarrow$ $\frac{2}{3(x)}=\frac{\sqrt{16 x^2-9}}{4 x}$

$\Rightarrow 16 x^2-9=\frac{64}{9}$

$\Rightarrow$ $x= \pm \frac{\sqrt{145}}{12}$

But $x>\frac{3}{4}$

So, $x=\frac{\sqrt{145}}{12}$

Hence, the answer is the option (1).

Example 4: If $\cos ^{-1} x-\cos ^{-1} \frac{y}{2}=\alpha$ , where$-1<x<1,-2 \leq y \leq 2, x \leq \frac{y}{2}$, then for all $x, y, 4 x^2-4 x y \cos \alpha+y^2$ is equal to :

1) $4 \sin ^2 a$
2) $2 \sin ^2 a$
3) $\sin ^2 \alpha-2 x^2 y$
4) $4 \cos ^2 a+2 x^2 y^2$

Solution

Formulae of Inverse Trigonometric Functions -

$\cos ^{-1} x+\cos ^{-1} y=\cos ^{-1}\left(x y-\sqrt{1-x^2} \sqrt{1-y^2}\right)$

- wherein

$x \geqslant 0, y \geqslant 0$

Trigonometric Identities -

$\sin ^2 \Theta+\cos ^2 \Theta=1$

$1+\tan ^2 \Theta=\sec ^2 \Theta$

$1+\cot ^2 \Theta=\operatorname{cosec}^2 \Theta$

- wherein

They are true for all real values of $\theta$

$\cos ^{-1} x-\cos ^{-1} \frac{y}{2}=\alpha$

where $-1 \leq x<1,-2 \leq y \leq 2, x \leq \frac{y}{2}$

$\cos \alpha=\cos \left(\cos ^{-1} x-\cos ^{-1} \frac{y}{2}\right)$

$=\cos \left(\cos ^{-1} x\right) \cdot \cos \left(\cos ^{-1} \frac{y}{2}\right)+\sin \left(\cos ^{-1} x\right) \sin \left(\cos ^{-1} \frac{y}{2}\right)$

$\begin{array}{r}=\frac{x y}{2}+\sqrt{1-x^2} \sqrt{1-\frac{y^2}{4}} \\ \cos \alpha-\frac{x y}{2}=\sqrt{1-x^2} \sqrt{1-\frac{y^2}{4}}\end{array}$

Squaring both sides

$\begin{aligned} & \cos ^2 \alpha+\frac{x^2 y^2}{4}-x y \cos \alpha=\left(1-x^2\right)\left(1-\frac{y^2}{4}\right) \\ & 1-4 x^2-y^2=4 \cos ^2 \alpha-4 x y \cos \theta \\ & =4\left(1-\cos ^2 a\right.\end{aligned}$

Hence, the answer is the option (1).

Example 5: If $\sin ^{-1} x+\sin ^{-1} y+\sin ^{-1} z=\frac{3 \pi}{2}$, then the value of $\sum \frac{\left(x^{101}+y^{101}\right)\left(x^{505}+y^{505}\right)}{\left(x^{303}+y^{303}\right)\left(x^{606}+y^{606}\right)}$

1) 1

2) 2

3) 3

4) 4

Solution

As we learned

$\sin ^{-1} x+\sin ^{-1} y+\sin ^{-1} z=\frac{3 \pi}{2}$

Is only possible if x=y=z=1 then $\sin ^{-1} x=\sin ^{-1} y=\sin ^{-1} z=\frac{\pi}{2}$

$\sum \frac{\left(x^{101}+y^{101}\right)\left(x^{500}+y^{500}\right)}{\left(x^{303}+y^{303}\right)\left(x^{606}+y^{606}\right)}=$

$\frac{\left(x^{101}+y^{101}\right)\left(x^{505}+y^{505}\right)}{\left(x^{303}+y^{303}\right)\left(x^{606}+y^{606}\right)}+\frac{\left(y^{101}+z^{101}\right)\left(y^{505}+z^{505}\right)}{\left(y^{303}+z^{303}\right)\left(y^{606}+z^{606}\right)}+\frac{\left(z^{101}+x^{101}\right)\left(z^{505}+x^{505}\right)}{\left(z^{303}+x^{303}\right)\left(z^{606}+x^{606}\right)}$

$=3$

Hence, the answer is the option 3.