Limit Using Expansion: Formula, Series

Limits are fundamental to the study of calculus and mathematical analysis, playing a crucial role in defining concepts such as continuity, derivatives, and integrals. One powerful method to evaluate limits is using series expansions, particularly the Taylor and Maclaurin series expansions. This technique not only simplifies complex limit problems but also provides deeper insight into the behavior of functions near specific points.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Limit Using Expansion
2. Solved Examples Based On Limits Using Expansion
3. Summary

In this article, we shall discuss the concept of Limit Using Expansion. This falls under the broader category of Calculus, which is one significant chapter within Class 11 Mathematics. It is essential not only for board exams but also for competitive exams, including Joint Entrance Examination Main and other entrance exams, namely: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of two questions have been asked on this topic in JEE Main from 2013 to 2023 including one in 2021, and one in 2022.

Limit Using Expansion

Expansions, also known as the Taylor Series, are powerful tools for representing functions as infinite sums of simpler terms. Using the expansion of some functions is one of the easier methods of finding the limit of expression. The following expansion formulas which are also known as Taylor series, are very useful in evaluating various limits.

The Taylor series of a function $f(x)$ at a point a is given by:

$f(x)=f(a)+f^{\prime}(a)(x-a)+\frac{f^{\prime \prime}(a)}{2!}(x-a)^2+\frac{f^{\prime \prime \prime}(a)}{3!}(x-a)^3+\cdots$

When point $a$ is zero, the Taylor series is called the Maclaurin series:

$f(x)=f(0)+f^{\prime}(0) x+\frac{f^{\prime \prime}(0)}{2!} x^2+\frac{f^{\prime \prime \prime}(0)}{3!} x^3+\cdots$

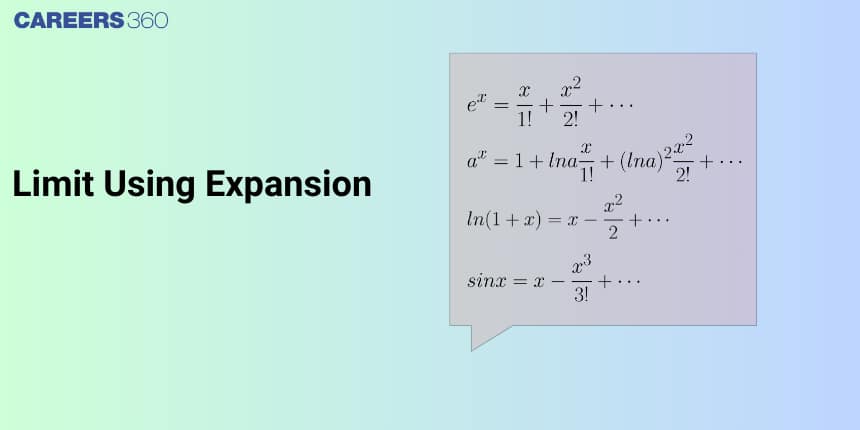
Important Expansions

Using the expansions is one of the methods to find the limits. The following expansion formulas which is also known as Taylor series, are very useful in evaluating various limits.

1. Logarithmic Expansion: $\log (1+x)=x-\frac{x^2}{2}+\frac{x^3}{3}-\frac{x^4}{4}+\cdots$
2. Exponential Expansion: $e^x=1+\frac{x^2}{2!}+\frac{x^3}{3!}+\frac{x^4}{4!}+\cdots$
3. Power Expansion: $a^x=1+x \log a+\frac{(x \log a)^2}{2!}+\frac{(x \log a)^3}{3!}+\cdots$
4. Sine Expansion:

$
\sin x=x-\frac{x^3}{3!}+\frac{x^5}{5!}-\cdots
$

5. Cosine Expansion:

$
\cos x=1-\frac{x^2}{2!}+\frac{x^4}{4!}-\cdots
$

6. Tangent Expansion:

$
\tan x=x+\frac{x^3}{3}+\frac{2 x^5}{15}+\cdots
$

Important Limits

Several important limits can be evaluated using series expansions. These limits often serve as foundational results in calculus.

1. $\begin{aligned} & \text { 1. } \lim\limits _{x \rightarrow 0} \frac{\sin x}{x}=1 \\ & \text { 2. } \lim\limits _{x \rightarrow 0} \frac{1-\cos x}{x^2}=\frac{1}{2} \\ & \text { 3. } \lim\limits _{x \rightarrow 0} \frac{\tan x}{x}=1 \\ & \text { 4. } \lim\limits _{x \rightarrow 0} \frac{e^x-1}{x}=1 \\ & \text { 5. } \lim\limits _{x \rightarrow 0} \frac{\log (1+x)}{x}=1\end{aligned}$

Recommended Video Based on Limits using Expansion

Solved Examples Based On Limits Using Expansion

Example 1: If $\lim\limits _{\mathrm{x} \rightarrow 0} \frac{\alpha \mathrm{e}^{\mathrm{x}}+\beta \mathrm{e}^{-\mathrm{x}}+\gamma \sin \mathrm{x}}{\mathrm{x} \sin ^2 \mathrm{x}}=\frac{2}{3}$, where $\alpha, \beta, \gamma \in \mathbf{R}_{\text {, then }}$ which of the following is NOT correct? [JEE Main 2022]
1) $\alpha^2+\beta^2+\gamma^2=6$
2) $\alpha \beta+\beta \gamma+\gamma \alpha+1=0$
3) $\alpha \beta^2+\beta \gamma^2+\gamma \alpha^2+3=0$
4) $\alpha^2-\beta^2+\gamma^2=4$

Solution:

$
\lim\limits _{x \rightarrow 0} \frac{\alpha\left(1+x+\frac{x^2}{2!}+\cdots\right)+\beta\left(1-x+\frac{x^2}{2!}-\frac{x^3}{3!}+\cdots\right)+x\left(x-\frac{x^3}{3!}+\cdots\right)}{x^3}
$
Constant terms should be zoro

$
\Rightarrow \alpha+\beta=0
$

coeff. of $x$ should be zero

$
\begin{aligned}
& \quad \Rightarrow \alpha-\beta+\gamma=0 \\
& \lim\limits _{x \rightarrow 0} x^3 \frac{\left.\frac{\alpha}{3!}-\frac{\beta}{3!}+\frac{\gamma}{3!}\right)+\mathrm{x}^4\left(\frac{\alpha}{3!}-\frac{\beta}{3!}-\frac{\gamma}{3!}\right)}{\mathrm{x}^3}=\frac{2}{3} \\
& \Rightarrow \frac{\alpha}{2}+\frac{\beta}{2}=0 \\
& \frac{\alpha}{6}-\frac{\beta}{6}-\frac{\gamma}{6}=2 / 3 \\
& \Rightarrow \alpha=1, \beta=-1, \quad \gamma=-2
\end{aligned}
$
Hence the answer is the option 3.

Example 2: If $0<a, b<1$ and $\tan ^{-1} a+\tan ^{-1} b=\frac{\pi}{4}$, then the value of $(a+b)-\left(\frac{a^2+b^2}{2}\right)+\left(\frac{a^3+b^3}{3}\right)-\left(\frac{a^4+b^4}{4}\right)+\ldots \ldots \ldots \cdot$ is: [JEE Main 2021]
1) $e$

$
{ }_{2)} \log _e\left(\frac{e}{2}\right)
$

3) $\log _e 2$
4) $e^2-1$

Solution:

$
\begin{aligned}
& \tan ^{-1} a+\tan ^{-1} b=\frac{\pi}{4} \quad 0<a, b<1 \\
& \Rightarrow \frac{a+b}{1-a b}=1 \\
& a+b=1-a b \\
& (a+1)(b+1)=2
\end{aligned}
$
$
\begin{aligned}
& \text { Now }\left[a-\frac{a^2}{2}+\frac{a^3}{3}+\ldots\right]+\left[b-\frac{b^2}{2}+\frac{b^3}{3}+\ldots\right] \\
& =\log _e(1+a)+\log _e(1+b) \\
& \left(\because \operatorname{expansion} \text { of } \log _e(1+x)\right) \\
& =\log _e[(1+a)(1+b)] \\
& =\log _e 2
\end{aligned}
$
Hence, the answer is the option 3.

Example 3: Find the limit $\lim\limits _{x \rightarrow 0} \frac{2^x-1}{(1+x)^{1 / 2}-1}=$
1) $\log 2$
2) $\log 4$
3) $\log \sqrt{2}$
4) None of these

Solution:
Using the expansion of some functions is one of the easier methods to finding the limit of expression. The following expansion formulas which is also known as Taylor series, are very useful in evaluating various limits.
As we know

$
\begin{aligned}
& a^x=1+x\left(\log _e a\right)+\frac{x^2}{2!}\left(\log _e a\right)^2+\frac{x^3}{3!}\left(\log _e a\right)^3+\ldots \ldots \ldots \\
& (1+x)^n=1+n x+\frac{n(n-1)}{2!} x^2+\frac{n(n-1)(n-2)}{3!} x^3+\ldots \ldots \ldots \\
& \lim\limits _{x \rightarrow 0} \frac{2^x-1}{(1+x)^{1 / 2}-1}=\frac{x \log _e 2+\frac{x^2}{2!}\left(\log _e 2\right)^2+\frac{x^3}{3!}\left(\log _e 2\right)^3+\ldots \ldots \ldots}{\frac{1}{2} x+\frac{\frac{1}{2}\left(\frac{1}{2}-1\right)}{2!} x^2+\frac{\frac{1}{2}\left(\frac{1}{2}-1\right)\left(\frac{1}{2}-2\right)}{3!} x^3+\ldots \ldots \ldots} \\
& =2 \log _e 2 \\
& =\log _e 4
\end{aligned}
$
Hence, the answer is the option 2.

Example 4: Find the limit $\lim\limits _{x \rightarrow 0} \frac{2 x\left(e^x-1\right)}{1-\cos x}$

1) $4$

2) $1$

3) $2$

4) $0$

Solution:

$\begin{aligned} & \lim\limits _{x \rightarrow 0} \frac{2 x\left(e^x-1\right)}{1-\cos x} \\ & =\lim\limits _{x \rightarrow 0} \frac{2 x\left(e^x-1\right)}{2 \cdot \sin ^2 \frac{x}{2}} \\ & =4 \lim\limits _{x \rightarrow 0}\left[\frac{(x / 2)^2}{\sin ^2 \frac{x}{2}}\right]\left(\frac{e^x-1}{x}\right) \\ & =4\end{aligned}$

Hence, the answer is the option 1.

Example 5: Find the limit $\lim\limits _{x \rightarrow 1} \frac{\log x}{x-1}=$

1) $1$

2) $0$

3) $-1$

4) $2$

Solution:

$\begin{aligned} & \lim\limits _{x \rightarrow 1} \frac{1}{x-1}=\lim\limits _{x \rightarrow 1} \frac{\log (x-1)+1]}{x-1} \\ & \text { put } \mathrm{x}-1=\mathrm{t} \\ &= \lim\limits _{t \rightarrow 0} \frac{\log (t+1)}{t} \because \log (1+x)=x-\frac{x^2}{2}+\frac{x^3}{3}-\frac{x^4}{4}+\ldots \ldots \ldots \\ &= 1\end{aligned}$

Hence, the answer is the option 1.

Summary

The expansion using limits is a useful part in the calculus, particularly by the Taylor Series which represents functions as infinite sums of simpler terms. Calculus was created to describe how the quantities change. The concept of limit is the cornerstone on which the development of calculus rests.