Limits of Trigonometric Functions

Limits are one of the most basic ideas in calculus, where one can learn how functions behave as they approach particular points. The application of the Trigonometric Limits can calculate the limits of these functions according to the continuity of the function, considering the function of the trigonometric equation. In real life, we use trigonometric equations for making roof inclination, installing ceramic tiles,building and navigating directions and lot more.

JEE Main: Study Materials | High Scoring Topics | Preparation Guide

JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs

This Story also Contains

1. Trigonometric Limits
2. Solved Examples Based On Trigonometric Limits
3. Summary

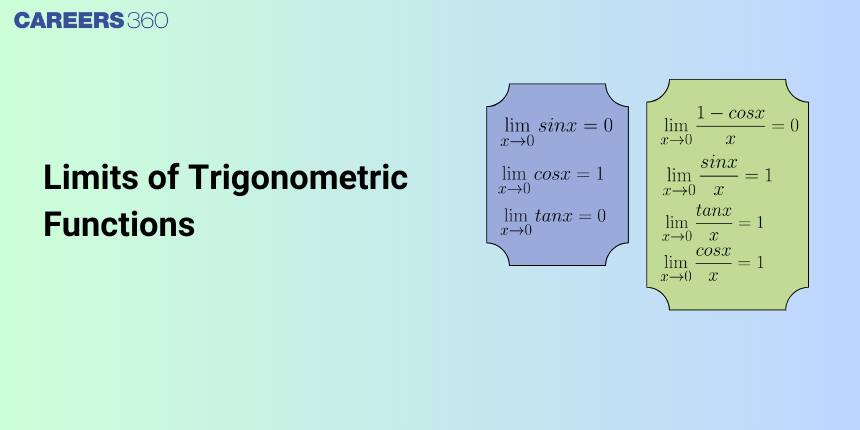
In this article, we will cover the concept of the Trigonometric Limits. This topic falls under the broader category of Calculus, which is a crucial chapter in Class 11 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of twenty-seven questions have been asked on this topic in JEE Main from 2013 to 2023 including one in 2013, three in 2014, two in 2015, one in 2016, one in 2017, two in 2019, two in 2020, seven in 2021, six in 2022 and two in 2023.

Trigonometric Limits

Trigonometric equations are, as the name implies, equations that involve trigonometric functions. Trigonometric equations are satisfied only for some values (finite or infinite in number) of the angles.

The following facts about functions in general come in handy in calculating the trigonometric functions:

Theorem 1: Let f and g be two real valued functions with the same domain such that $f(x)\le g(x)\text{for all }\mathrm{x}$ in the domain of definition, For some $a$, if both $\underset{x\to a}{lim}f(x)$ and $\underset{x\to a}{lim}g(x)$ exist, then $\underset{x\to a}{lim}f(x)\le \underset{x\to a}{lim}g(x)$

Theorem 2: In the trigonometric limit, apart from using the method of direct substitution, factorization, and rationalization (same as given in algebraic limits), we can use the following formula.

(i) $\underset{x\to 0}{lim}\frac{\sin x}{x}=1$
(ii) $\underset{\mathrm{x}\to 0}{lim}\frac{\tan \mathrm{x}}{\mathrm{x}}=1$

As $\underset{x\to 0}{lim}\frac{\tan x}{x}=\underset{x\to 0}{lim}\frac{\sin x}{x}\times \frac{1}{\cos x}$

$=\underset{x\to 0}{lim}\frac{\sin x}{x}\times \underset{x\to 0}{lim}\frac{1}{\cos x}=1\times 1$

(iii) $\underset{\mathbf{x}\to \mathbf{a}}{lim}\frac{\sin (\mathbf{x}-\mathbf{a})}{\mathbf{x}-\mathbf{a}}=\mathbf{1}$

As $\underset{x\to a}{lim}\frac{\sin (x-a)}{x-a}=\underset{h\to 0}{lim}\frac{\sin ((a+h)-a)}{(a+h)-a}$

$\begin{array}{rl}& =\underset{h\to 0}{lim}\frac{\sin h}{h}\\ & =1\end{array}$

(iv) $\underset{\mathrm{x}\to \mathrm{a}}{lim}\frac{\tan (\mathrm{x}-\mathrm{a})}{\mathrm{x}-\mathrm{a}}=\mathbf{1}$
(v) $\underset{x\to a}{lim}\frac{\sin (f(x))}{f(x)}=1$, if $\underset{x\to a}{lim}f(x)=0$

Similarly, $\underset{x\to a}{lim}\frac{\tan (f(x))}{f(x)}=1$, if $\underset{x\to a}{lim}f(x)=0$
(vi) $\underset{x\to 0}{lim}\cos x=1$
(vii) $\underset{\mathrm{x}\to 0}{lim}\frac{{\sin }^{-1}\mathrm{x}}{\mathrm{x}}=1$

As $\underset{x\to 0}{lim}\frac{{\sin }^{-1}x}{x}=\underset{y\to 0}{lim}\frac{y}{\sin y}[\because {\sin }^{-1}x=y]$
$=1$
(viii) $\underset{\mathrm{x}\to 0}{lim}\frac{{\tan }^{-1}\mathrm{x}}{\mathrm{x}}=1$

Recommended Video Based on Trigonometric Limits

Solved Examples Based On Trigonometric Limits

Example 1: $\underset{x\to 0}{lim}\frac{(1-\cos 2x{)}^2}{2x\tan x-x\tan 2x}$ is [JEE Main 2016]
1) $-2$
2) $-\frac{1}{2}$
3) $\frac{1}{2}$
4) $2$

Solution:

$\begin{array}{rl}& \underset{x\to 0}{lim}\frac{(1-\cos 2x{)}^2}{2x\tan x-x\tan 2x}\\ & \underset{x\to 0}{lim}\frac{4{\sin }^{4}x}{x(2\tan x-\tan 2x)}\\ & \because 1-\cos 2x=2{\sin }^{2}x\\ & \underset{x\to 0}{lim}4{\left(\frac{\sin x}{x}\right)}^4\frac{x^3}{(2\tan x-\tan 2x)}=\underset{x\to 0}{lim}\frac{4x^3}{(2\tan x-\tan 2x)}\\ & \because \underset{x\to 0}{lim}\frac{\sin x}{x}=1\end{array}$

Now use series expansion

$\begin{array}{rl}& \begin{array}{rl}& \underset{x\to 0}{lim}\frac{4x^3}{(2\tan x-\tan 2x)}\\ & \underset{x\to 0}{lim}\frac{4x^2}{2(x+\frac{x^3}{3}+\frac{2x^5}{15}+\dots )-(2x+\frac{(2x{)}^3}{3}+2\cdot \frac{(2x{)}^5}{15}+\dots )}\\ & \underset{x\to 0}{lim}\frac{4x^3}{2x+\frac{2x^3}{3}+\frac{4x^5}{15}+\dots -2x-\frac{8x^3}{3}-\frac{64x^5}{15}-\dots }\\ & \underset{x\to 0}{lim}\frac{4}{\frac{2}{3}+\frac{4x^2}{15}+\dots -\frac{8}{3}-\frac{64x^2}{15}-\dots }\\ & \underset{x\to 0}{lim}\frac{4}{\frac{2}{3}-\frac{8}{3}}=-2\end{array}\\ & \text{ Hence, the answer is the option }1.\end{array}$

Example 2:

$\underset{x\to 0}{lim}\frac{x\cot (4x)}{{\sin }^{2}x{\cot }^2(2x)}$ is equal to :
[JEE Main 2019]
1) $1$
2) $2$
3) $4$
4) $0$

Solution:

Evalution of Trigonometric limit -

$\begin{array}{rl}& \underset{x\to a}{lim}\frac{\sin (x-a)}{x-a}=1\\ & \underset{x\to a}{lim}\frac{\tan (x-a)}{x-a}=1\end{array}$

put $x=a+h$ where $h\to 0$
Then it comes

$\begin{array}{rl}& \underset{h\to 0}{lim}\frac{\sin h}{h}=\underset{h\to 0}{lim}\frac{\tan h}{h}=1\\ & \therefore \underset{x\to 0}{lim}\frac{\sin x}{x}=1\text{ and }\\ & \therefore \underset{x\to 0}{lim}\frac{\tan x}{x}=1\\ & \underset{x\to 0}{lim}\frac{x\cot 4x}{{\sin }^{2}x{\cot }^{2}2x}=\underset{x\to 0}{lim}\frac{x{\tan }^{2}2x}{{\sin }^{2}x\tan 4x}\\ & =\underset{x\to 0}{lim}\frac{x\left(\frac{{\tan }^{2}2x}{4x^2}\right)\times 4x^2}{\frac{{\sin }^{2}x}{x^2}\times x^2\left(\frac{\tan 4x}{4x}\right)\times 4x}\\ & =1\end{array}$
$\begin{array}{rl}& \left(\underset{z\to 0}{lim}\right)\frac{\tan z}{z}=1\left(\underset{z\to 0}{lim}\right)\frac{\sin z}{z}=1\\ & \text{ Hence, the answer is the option }1.\end{array}$

Example 3: $\underset{x\to \frac{\pi }{2}}{lim}\frac{\cot x-\cos x}{(\pi -2x{)}^3}$ equals:
[JEE Main 2017]
1) $\frac{1}{16}$
2) $\frac{1}{8}$
3) $\frac{1}{4}$
4) $\frac{1}{24}$

Solution:

$\begin{array}{rl}& \begin{array}{rl}& \underset{x\to \frac{\pi }{2}}{lim}\frac{\cot x-\cos x}{(\pi -2x{)}^3}\\ & \text{ Put }x=\frac{\pi }{2}-h\\ & \Rightarrow \underset{h\to 0}{lim}\frac{\cot (\frac{\pi }{2}-h)-\cos (\frac{\pi }{2}-h)}{{[\pi -2(\frac{\pi }{2}-h)]}^3}\\ & \Rightarrow \underset{h\to 0}{lim}\frac{\tan h-\sin h}{(\pi -\pi +2h{)}^3}\\ & \Rightarrow \underset{h\to 0}{lim}\frac{\tanh (1-\cos h)}{8h^3}\\ & \Rightarrow \frac{1}{8}\underset{h\to 0}{lim}\frac{\tan h}{h}\cdot \frac{2{\sin }^2\frac{h}{2}}{h^2}\\ & \Rightarrow \frac{1}{8}\underset{h\to 0}{lim}\frac{\tan h}{h}\cdot \frac{2{\sin }^2\frac{h}{2}}{4\cdot {\left(\frac{h}{2}\right)}^2}\\ & \Rightarrow \frac{1}{8}\times 1\times \frac{1}{2}=\frac{1}{16}\end{array}\\ & \text{ Hence, the answer is the option }1.\end{array}$

Example 4: $\underset{x\to 0}{lim}\frac{(1-\cos 2x)(3+\cos x)}{x\tan 4x}$ is equal to :

[JEE Main 2013]
1) $2$
2) $-0.25$
3) $0.5$
4) $1$

Solution:

$\begin{array}{rl}& \underset{x\to 0}{lim}\frac{(1-\cos 2x)(3+\cos x)}{x\tan 4x}\\ & \underset{x\to 0}{lim}\frac{2{\sin }^{2}x(3+\cos x)}{4x^2\frac{\tan 4x}{4x}}\\ & =\frac{1}{2}\times 4=2\end{array}$

Hence, the answer is the option (1).

Example 5 : If $\alpha$ is the positive root of the equation, $p(x)=x^2-x-2=0$, then $\underset{x\to {\alpha }^{+}}{lim}\frac{\sqrt{1-\cos (p(x))}}{x+\alpha -4}$ is equal to: [JEE Main 2020]
$\frac{3}{2}$
2) $\frac{3}{\sqrt{2}}$
3) $\frac{1}{\sqrt{2}}$
4) $1$

Solution:

$\begin{array}{rl}& \begin{array}{rl}& x^2-x-2=0\\ & \text{ roots are }2\mathrm{\&}-1\\ & \Rightarrow \underset{x\to 2^{+}}{lim}\frac{\sqrt{1-\cos (x^2-x-2)}}{(x-2)}\\ & =\underset{x\to 2^{+}}{lim}\frac{\sqrt{2{\sin }^2\frac{(x^2-x-2)}{2}}}{(x-2)}\\ & =\underset{x\to 2^{+}}{lim}\frac{\sqrt{2}\sin \left(\frac{(x-2)(x+1)}{2}\right)}{(x-2)}\\ & =\frac{3}{\sqrt{2}}\end{array}\\ & \text{ Hence, the answer is an option (2). }\end{array}$

Summary

In conclusion, By applying the Law of Sines, the Law of Cosines, and other relevant formulas, one can systematically find all the limits as The concept of limit is the cornerstone on which the development of calculus rest.