Multiplication Of Vectors by a Scalar Quantity

A quantity that has magnitude but no direction is called a scalar quantity (or scalar), e.g., mass, volume, density, speed, etc. A quantity that has magnitude as well as a direction in space and follows the triangle law of addition is called a vector quantity, e.g., velocity, force, displacement, etc. Multiplication of vector quantity with scalar quantity results in vector quantity. In real life, we use vectors for tracking objects that are in motion, and localization of places and things.

This Story also Contains

1. Multiplication of a vector by a scalar
2. Geometric visualization of the multiplication of a vector by a scalar
3. Vector Quantity
4. Representation of a Vector
5. Components of Vector
6. Solved Examples Based on Multiplication Of Vectors And Scalar Quantity

In this article, we will cover the concept of Multiplication of a Vector by a Scalar. This topic falls under the broader category of Vector Algebra, which is a crucial chapter in Class 11 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of fifteen questions have been asked on this topic in JEE Main from 2013 to 2023 including one in 2014, one in 20217, and two in 2019.

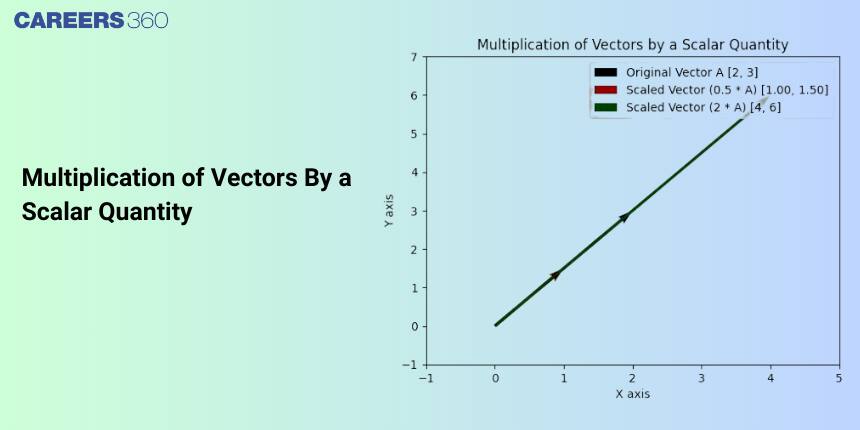
Multiplication of a vector by a scalar

If a is a vector and "λ" is a scalar (i.e. a real number), then λa is a vector whose magnitude is |λ| times that of a and whose direction is the same (or opposite) that of a according as the value of λ is positive (or negative).

Then the product of vector a by scalar λ denoted by is called the multiplication of vector a by the scalar λ. Also, the magnitude of vector λ*a is |λ| times the magnitude of vector a.

Note that λa is collinear to the vector a.

If

$
\tilde{\mathbf{a}}=\mathrm{a}_1 \hat{\mathbf{i}}+\mathrm{a}_2 \hat{\mathbf{j}}+\mathrm{a}_3 \hat{\mathbf{k}}
$

then,

$
\lambda \tilde{\mathbf{a}}=\left(\lambda \mathrm{a}_1\right) \hat{\mathbf{i}}+\left(\lambda \mathrm{a}_2\right) \hat{\mathbf{j}}+\left(\lambda \mathrm{a}_3\right) \hat{\mathbf{k}}
$
Properties of multiplication of a vector by a scalar $a$ and $b$ are vectors, $\lambda$ and $\gamma$ are scalars.
1. $\lambda(-\tilde{\mathbf{a}})=(-\lambda)(\tilde{\mathbf{a}})=-(\lambda \tilde{\mathbf{a}})$
2. $(-\lambda)(-\tilde{\mathbf{a}})=\lambda \tilde{\mathbf{a}}$
3. $\quad \lambda(\gamma \tilde{\mathbf{a}})=(\lambda \gamma)(\tilde{\mathbf{a}})=\gamma(\lambda \tilde{\mathbf{a}})$
4. $(\lambda+\gamma) \tilde{\mathbf{a}}=\lambda \tilde{\mathbf{a}}+\gamma \tilde{\mathbf{a}}$
5. $\quad \lambda(\tilde{\mathbf{a}}+\tilde{\mathbf{b}})=\lambda(\tilde{\mathbf{a}})+\lambda(\tilde{\mathbf{b}})$

Geometric visualization of the multiplication of a vector by a scalar

Vector Quantity

A quantity that has magnitude as well as a direction in space and follows the triangle law of addition is called a vector quantity, e.g., velocity, force, displacement, etc.

We denote vectors by boldface letters, such as a or $\vec{a}$.

Representation of a Vector

A vector is represented by a directed line segment (an arrow). The endpoints of the segment are called the initial point and the terminal point of the vector. An arrow from the initial point to the terminal point indicates the direction of the vector.

The length of the line segment represents its magnitude. In the above figure, $\mathrm{a}=\mathrm{AB}$, and the magnitude (or modulus) of vector a is denoted as
(Distance between the initial and terminal point).
The arrow indicates the direction of the vector.

Components of Vector

Let the points $A(1,0,0), B(0,1,0)$ and $C(0,0,1)$ on the $x$-axis, $y$-axis and $z$-axis, respectively. Then, clearly.
$|\overrightarrow{O X}|=1 .|\overrightarrow{O B}|=1$ and $|\overrightarrow{O C}|=1$

The vectors, $\overrightarrow{O A}, \overrightarrow{O B}$ and $\overrightarrow{O C}$ each having magnitude 1 , are called unit vectors along the axes OX, OY, and OZ, respectively, and denoted by $\hat{\mathrm{i}} \hat{\mathrm{j}}$, and $\hat{\mathbf{k}}$ respectively.
Similarly $\overrightarrow{\mathrm{QP}}_1=\overrightarrow{\mathrm{OS}}=y \hat{\mathbf{j}}$ and $\overrightarrow{\mathrm{OQ}}=x \hat{\mathbf{i}}$

Therefore,

$
\begin{aligned}
& \overrightarrow{\mathrm{OP}_1}=\overrightarrow{\mathrm{OQ}}+\overrightarrow{\mathrm{QP}_1}=x \hat{i}+y \hat{j} \\
& \overrightarrow{\mathrm{OP}}=\overrightarrow{\mathrm{OP}_1}+\overrightarrow{\mathrm{P}_1 \mathrm{P}}=x \hat{i}+y \hat{j}+z \hat{k}
\end{aligned}
$
Hence, the position vector of P with reference to O is given by

$
\overrightarrow{\mathrm{OP}}(\text { or } \vec{r})=x \hat{i}+y \hat{j}+z \hat{k}
$
And, the length of any vector $\vec{r}=x \hat{i}+y \hat{j}+z \hat{k}$ is given by

$
|\vec{r}|=|x \hat{i}+y \hat{j}+z \hat{k}|=\sqrt{x^2+y^2+z^2}
$

Recommended Video Based on Multiplication of Vectors and Scalar Quantity

Solved Examples Based on Multiplication Of Vectors And Scalar Quantity

Example 1: Let $\vec{a}-2 \hat{i}+\lambda_1 \vec{j}+3 \hat{k} \cdot \vec{b}=1 \hat{i}+\left(3-\lambda_2\right) \hat{j}+6 \hat{k}$ and $\vec{c}=3 \hat{i}+6 \hat{j}+\left(\lambda_i-1\right) k$ be three vectors such that $\vec{b}=2 \vec{a}$
[JEE MAINS 2019]
and 2 is perpendicular to Then a possible value of is:

Solution: Given, $\square$
$4+\left(3-\lambda_2\right) 2+6 k=4 i+2 \lambda_i+6 \lambda$
E23-xin
$E>2 \lambda_1+\lambda_2=3 \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots(1)$
Given and $\vec{C}$ is perpendicular
So. ${ }_2^2=0$
$E 36^2+6 \lambda 1+3(\lambda u-1)=0$
Now,
$\left.\sum_1 \lambda_2 \lambda_n\right)=\left(\lambda_1, 3-2 \lambda_1-1-2 \lambda_1\right.$
$\left.\frac{-1}{2}, 1,0\right)$
satisties this.
Hence, the answer is $(-1 / 2,4,0)$

Example 2: Let $\vec{\alpha}=(\lambda-2) \vec{a}+\vec{b}$ and $\vec{\beta}=(4 \lambda-2) \vec{a}+3 \vec{b}$ be two given vectors where vectors $\vec{a}$ and $\vec{b}$ are non-collinear. The value of $\lambda$ for which vectors $\vec{\alpha}$ and $\vec{\beta}$ are collinear is:
SolutionGiven vectors are

$
\begin{aligned}
& \vec{\alpha}=(\lambda-2) \vec{a}+\vec{b} \\
& \vec{\beta}=(4 \lambda-2) \vec{a}+3 \vec{b}
\end{aligned}
$
As $\vec{\alpha}, \vec{\beta}$ are collinear, and $\vec{\alpha}$ and $\vec{b}$ are non-collinear, hence:

$
\begin{aligned}
& \frac{\lambda-2}{4 \lambda-2}=\frac{1}{3} \\
& \Rightarrow \lambda=-4
\end{aligned}
$
Hence, the answer is -4
Example 3: Let $\vec{a}, \vec{b}$ are such two vectors such that $\vec{b}=5 \vec{a}$ and $|\vec{a}|=2$ than $|\vec{b}|_{\text {equals: }}$
Solution: Scalar multiplication - If $\vec{a}$ is a vector and $m$ is a scalar, then $m \vec{a}$ is a vector whose modulus is $m$ times $\vec{a}$.

$
\because \vec{b}=5 \vec{a} \Rightarrow|\vec{b}|=5|\vec{a}| \Rightarrow|\vec{b}|=5 \times 2=10
$
Hence, the answer is 10

Example 4: The non-zero vectors $\vec{a}, \vec{b}$ and $\vec{c}$ are related by $\vec{a}=8 \vec{b}$ and $\vec{c}=-7 \vec{b}$. Then the angle between $\vec{a}$ and $\vec{c}$ is
Solution: Collinear Vectors - Two vectors are said to be collinear if and only if there exists a scalar m such as that $\vec{a}=m \vec{b}, \mathrm{~m}$ is a Scalar.

$
\begin{aligned}
& \vec{a} \cdot \vec{c}=8 \vec{b}(-7 \vec{b}) \\
= & -56|\vec{b}|^2<0
\end{aligned}
$
Also $\vec{a}$ and $\vec{b}$ collinear where as $\vec{b}$ and $\vec{c}$ collinear
$\Rightarrow \vec{a}$ and $\vec{c}$ collinear
So, the angle between $\vec{a}$ and $\vec{c}=\pi$
Hence, the answer is $\pi$
Example 5: If $\vec{a}=2 \hat{i}-3 \hat{j}+\hat{k}, \vec{b}=\hat{i}+\hat{j}-\hat{k}$, then $2 \vec{a}+4 \vec{b}$ equals
Solution: $2 \vec{a}=4 \hat{i}-6 \hat{j}+2 \hat{k}, 4 \vec{b}=4 \hat{i}+4 \hat{j}-4 \hat{k}$

$
\therefore 2 \vec{a}+4 \vec{b}=8 \hat{i}-2 \hat{j}-2 \hat{k}
$
Hence, the answer is $8 \hat{i}-2 \hat{j}-2 \hat{k}$