Power Set: Definition, Equation, Formula, Examples, Calculator

Power set

Edited By Komal Miglani | Updated on Jul 02, 2025 06:37 PM IST

Consider a small garden with three types of plants: It includes roses, tulips, and daisies. The power set incorporates everything that has been noted above, including the empty set, every plant as an individual member, combinations of two plants, and all three plants as the power set members. The set of all subsets of a set is called the power set.

This Story also Contains

What is a Power Set?
Cardinality of Power Set
Properties of Power Set
Solved Examples Based on Power Set

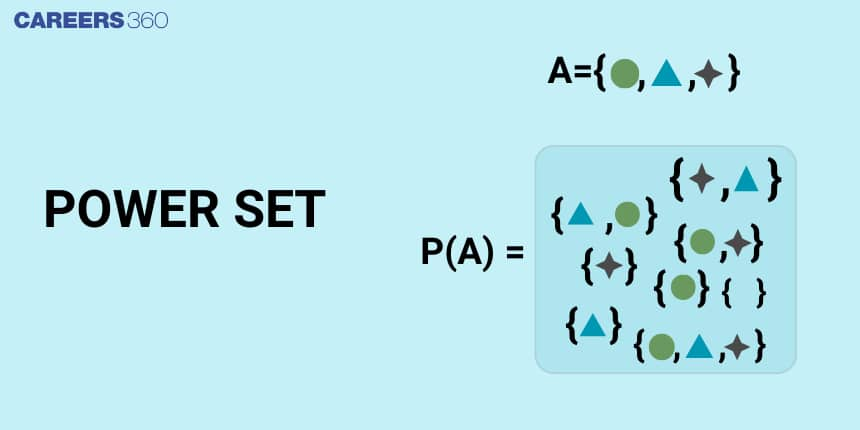
Power set

Power sets determine the links among various subsets of elements. This means that the power set contains all the possible subsets of a particular set, depicting all the possibilities in a certain range of the set. Sets are very fundamental concepts in mathematics, which have applications across various domains like statistics, calculus, computer science, etc.

In this article, we will cover the concept of power sets. This concept falls under the broader category of sets, a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of three questions have been asked on this concept, including one in 2014, one in 2022, and one in 2023.

What is a Power Set?

The set of all subsets of a set $S$ is called the power set of ' $S$ '. It is denoted by $P(S)$. It also includes the empty set and the set itself. The power set always contains $2^n$ elements, where $n$ is the number of elements in the original set.

Power Set Definition: The collection of all subsets of a set $A$ is called the power set of $A$. It is denoted by $P(A)$.

Power Set Example: Let $\mathrm{A}=\{\mathrm{a}, \mathrm{b}, \mathrm{c}\}$, then
$P(A)=\{\varphi,\{a\},\{b\},\{c\},\{a, b\},\{b, c\},\{c, a\},\{a, b, c\}\}$

Cardinality of Power Set

The number of distinct elements in a finite set $A$ is called the Cardinal number or cardinality of set $A$ and it is denoted by $n(A)$.

The power set formula to find the cardinality(number of elements) of the power set of any set $A$ is $2^n$ where $n$ is the number of elements in $A$.

Eg. Let $\mathrm{A}=\{\mathrm{a}, \mathrm{b}, \mathrm{c}\}$. The cardinality of the set $A$ is $3$. The cardinality of the power set of $A$, $P(A)$ is $2^n = 2^3 = 8$.

Properties of Power Set

The properties of the power set are,

The power set of any set is non-empty.
Each element of a Power set is a set.
Number of elements in $P(A)=$ Number of subsets of $\operatorname{set} A
=2 ^{n(A)}$ where $n(A)$ is the number of elements in set $A$
It is much larger than the original set.
The power set of a finite set has finite number of elements.
For a set of natural numbers, we can do one-to-one mapping of the resulting set, $P(S)$ with the real numbers.
$P(S)$ of set $S$, if operated with the union of sets, the intersection of sets and complement of sets, denotes the example of Boolean Algebra.

NEET Highest Scoring Chapters & Topics

This ebook serves as a valuable study guide for NEET exams, specifically designed to assist students in light of recent changes and the removal of certain topics from the NEET exam.

Download E-book

Solved Examples Based on Power Set

Example 1: Power set of $\{1,2,3\}$ is

$
\begin{aligned}
& \text { 1) } P(A)=\{\phi,\{1\},\{2\},\{3\},\{1,2\},\{1,3\},\{2,3\}\} \\
& \text { 2) } P(A)=\{\{1\},\{2\},\{3\},\{1,2\},\{1,3\}\{2,3\},\{1,2,3\}\} \\
& \text { 3) } P(A)=\{\phi,\{1\},\{2\},\{3\},\{1,2\},\{1,3\},\{2,3\},\{1,2,3\}\}
\end{aligned}
$
4) None of the above

Solution

As we learned
In this Question,
Option (3) shows all the subsets of $\{1,2,3\}$
Hence, the answer is the option 3.

Example 2: Find the number of elements in the power set of $\{1,2,3,4\}$.
1) $8$
2) $16$
3) $32$
4) $64$

Solution
As we learned
In this Question,
The power set of $\{1,2,3,4\}$ will have $16$ elements.
Hence, the answer is the option 2.

Example 3: Let $\mathrm{A}=\{1,2,3,4,5,6,7\}$ and $\mathrm{B}=\{3,6,7,9\}$. Then the number of elements in the set $\{\mathrm{C} \subseteq \mathrm{A}: \mathrm{C} \cap \mathrm{B} \neq \phi\}$ is. $\qquad$ .

Solution

Total subsets of $\mathrm{A}=2^7=128$
Subsets of $A$ such that $C \cap B=\phi$

$
\begin{aligned}
& =\text { Subsets of }\{1,2,4,5\}=2^4=16 \\
& \text { Required Subsets }=128-16=112
\end{aligned}
$

Hence, the answer is $112$.

Example 4: The number of elements in the power set of $P(P(P(\phi))$ is
1) $1$
2) $2$
3) $3$
4) $4$

Solution

Number of elements in $\mathrm{P}(\varphi)$ is $2^0=1$
Number of elements in $\mathrm{P}(\mathrm{P}(\varphi))$ is $2^1=2$
Number of elements in $\mathrm{P}(\mathrm{P}(\mathrm{P}(\varphi)))$ is $2^2=4$
Hence, the answer is the option 4.

List of Topics Related to Power Set

Roster And Set Builder Form Of Sets	Universal Set
Equal And Equivalent Sets	Difference Of Set
Singleton Set	De-morgan's Laws

Frequently Asked Questions (FAQs)

1. Define power set.

The set of all subsets of a set $A$ is called the power set of ' $A$ '. It is denoted by $P(A)$.

2. What is the power set of empty set?

An empty set is a null set, which does not have any elements present in it. Therefore, the power set of the empty set has only one element which is the empty set only.

3. What is the power set of $a=\{1,2,3\}$?

The power set of set $a = {1,2,3}$ is $P(a) = \{\{\}, \{1\}, \{2\}, \{3\}, \{1,2\}, \{2,3\}, \{1,3\}, \{1,2,3\}\}$.

4. How is power set calculated?

The power set can be framed by including the null set, all possible singleton set, all other possible subsets and the original set.

5. What is the cardinality of power set?

The cardinality of a set refers to the number of elements in a set. The cardinality of the power set of any set $A$ can be calculated by $2^n$ where $n$ is the number of elements in the set $A$.

6. What is the cardinality of a power set?

The cardinality (number of elements) of a power set is 2^n, where n is the number of elements in the original set. This is because each element can either be included or excluded in a subset, giving 2 choices for each element.

7. How does the size of a power set compare to the size of the original set?

The size of a power set is always larger than the original set (except for the empty set). If the original set has n elements, its power set will have 2^n elements.

8. What is the power set of {0, 1}?

The power set of {0, 1} is {∅, {0}, {1}, {0, 1}}. It includes all possible combinations of the elements, including the empty set and the full set.

9. What is the power set of the empty set?

The power set of the empty set is a set containing only the empty set. P(∅) = {∅}

10. How do you find the power set of a set with one element?

For a set with one element, say {a}, its power set is {∅, {a}}. It contains the empty set and the set itself.

11. What is a power set?

A power set is the set of all possible subsets of a given set, including the empty set and the set itself. It represents all combinations of elements that can be formed from the original set.

12. How do you denote a power set?

A power set is typically denoted by P(S) or 2^S, where S is the original set. For example, if S = {a, b}, then P(S) represents its power set.

13. Does a power set always include the empty set?

Yes, a power set always includes the empty set (∅) as one of its elements. The empty set is a subset of every set, including the original set itself.

14. What is the relationship between a set and its power set?

A set is always a subset of its own power set. In fact, it is one of the elements of the power set, along with all its other subsets.

15. Can a power set contain duplicate elements?

No, a power set cannot contain duplicate elements. Each subset in the power set is unique, even if the original set contains duplicates.

16. How is the concept of power sets used in mathematics?

Power sets are used in various areas of mathematics, including combinatorics, probability theory, and topology. They help in counting possibilities, defining topological spaces, and understanding set relationships.

17. How are power sets used in computer science?

In computer science, power sets are used in algorithms for generating all possible combinations, in set-based data structures, and in certain areas of complexity theory and formal language theory.

18. What is the connection between power sets and the concept of a metric space?

In the study of metric spaces, the power set of the underlying set is often used to define the collection of open sets, which forms a topology. This connection links set theory, topology, and metric spaces.

19. Can a power set be empty?

No, a power set is never empty. Even for an empty set, its power set contains one element: the empty set itself. P(∅) = {∅}

20. How many elements are in the power set of a set with 3 elements?

The power set of a set with 3 elements has 2^3 = 8 elements. This includes the empty set, three 1-element subsets, three 2-element subsets, and the original set itself.

21. Is the original set always the largest element in its power set?

Yes, the original set is always the largest element in its power set because it contains all the elements of the original set.

22. Can elements of a power set be sets themselves?

Yes, elements of a power set are always sets. Each element in a power set is a subset of the original set, and subsets are themselves sets.

23. What is the difference between a subset and an element of a power set?

Every subset of a set is an element of its power set, but not every element of a power set is necessarily a subset of another element in the power set.

24. How does set theory relate to power sets?

Power sets are a fundamental concept in set theory. They help in understanding relationships between sets and are crucial in defining many advanced set-theoretic concepts.

25. Can you have a power set of an infinite set?

Yes, you can have a power set of an infinite set. However, the cardinality of the power set of an infinite set is always greater than the cardinality of the original set.

26. What is the power set axiom in set theory?

The power set axiom states that for any set A, there exists a set P(A) that contains all subsets of A. This axiom ensures the existence of power sets in set theory.

27. What is the connection between power sets and binary numbers?

There's a one-to-one correspondence between subsets in a power set and binary numbers. Each subset can be represented by a binary number where 1 indicates the presence of an element and 0 indicates its absence.

28. How do you list all elements of a power set systematically?

One systematic way is to use binary representations. List all binary numbers from 0 to 2^n - 1 (where n is the number of elements in the original set), and use 1s and 0s to indicate which elements are included in each subset.

29. What is the power set of a power set called?

The power set of a power set is sometimes referred to as the "second-order power set" or "iterated power set". It's denoted as P(P(S)) where S is the original set.

30. How does the concept of power sets relate to Boolean algebra?

Power sets have a strong connection to Boolean algebra. The operations on power sets (union, intersection, complement) correspond to Boolean operations (OR, AND, NOT) on the characteristic functions of sets.

31. Can two different sets have the same power set?

No, two different sets cannot have the same power set. The power set uniquely determines the original set, a property known as extensionality in set theory.

32. What is the relationship between power sets and the concept of functions?

The set of all functions from set A to {0,1} is in one-to-one correspondence with the power set of A. Each function corresponds to a unique subset of A.

33. How do power sets relate to the concept of cardinality in set theory?

Power sets play a crucial role in understanding different levels of infinity. Cantor's theorem states that the cardinality of a power set is always strictly greater than the cardinality of the original set.

34. What is the power set of the set of natural numbers?

The power set of the set of natural numbers is uncountably infinite. Its cardinality is 2^ℵ₀ (2 to the power of aleph-null), which is equal to the cardinality of the real numbers.

35. What is the connection between power sets and the concept of a sigma-algebra in measure theory?

A sigma-algebra is a subset of the power set of a given set, closed under countable set operations. The power set itself is always a sigma-algebra, often the largest one considered for a given set.

36. How do power sets relate to the concept of a topology?

In topology, a topology on a set X is defined as a subset of the power set of X that satisfies certain axioms. The power set itself is always a valid topology, known as the discrete topology.

37. What is the relationship between power sets and the concept of a partition of a set?

While a power set contains all possible subsets of a set, a partition is a specific subset of the power set where the subsets are non-empty, mutually exclusive, and their union is the entire set.

38. How does the concept of power sets extend to fuzzy sets?

In fuzzy set theory, the power set concept is extended to fuzzy power sets, where each element in the power set has a degree of membership between 0 and 1, rather than just being present or absent.

39. What is the connection between power sets and the concept of a lattice in order theory?

The power set of any set, when ordered by set inclusion, forms a complete lattice. This connection is fundamental in order theory and provides a concrete example of a Boolean algebra.

40. How do power sets relate to the concept of a Boolean ring in algebra?

The power set of any set forms a Boolean ring under the operations of symmetric difference (as addition) and intersection (as multiplication). This connection bridges set theory and abstract algebra.

41. What is the role of power sets in defining the concept of measurable sets in measure theory?

In measure theory, measurable sets are often defined as elements of a sigma-algebra, which is a special subset of the power set. The power set provides the largest possible collection of measurable sets.

42. How do power sets relate to the concept of a state space in probability theory?

In probability theory, the state space (the set of all possible outcomes) is often represented by a set S, and events are subsets of S. The collection of all possible events is thus the power set of S.

43. What is the significance of power sets in combinatorial optimization?

Power sets are crucial in combinatorial optimization problems, especially those involving subset selection. The power set represents all possible combinations that need to be considered in exhaustive search algorithms.

44. How do power sets relate to the concept of a Venn diagram?

A Venn diagram for n sets can be thought of as a visual representation of the power set of a set with n elements. Each region in the diagram corresponds to a unique subset in the power set.

45. What is the connection between power sets and the binomial theorem?

The number of k-element subsets in a power set of an n-element set is given by the binomial coefficient (n choose k). This connection links power sets to the binomial theorem and Pascal's triangle.

46. How do power sets relate to the concept of a Boolean function in logic?

A Boolean function of n variables can be represented as a subset of the power set of an n-element set. This connection is fundamental in the study of Boolean algebras and digital logic design.

47. What is the role of power sets in defining the concept of a filter in topology?

In topology and set theory, a filter on a set X is a special subset of the power set of X that satisfies certain properties. The power set provides the context in which filters are defined and studied.

48. How do power sets relate to the concept of a closure operator in mathematics?

A closure operator on a set S is a function from the power set of S to itself, satisfying certain axioms. The power set provides the domain and codomain for this important mathematical concept.

49. What is the significance of power sets in the foundations of mathematics?

Power sets play a crucial role in the foundations of mathematics, particularly in set theory. They are essential in defining cardinal numbers, constructing higher-order sets, and in formulating important axioms and theorems.

50. How do power sets relate to the concept of a complete lattice in order theory?

The power set of any set, when ordered by inclusion, forms a complete lattice. This means that every subset of the power set has both a supremum (least upper bound) and an infimum (greatest lower bound).

51. How do power sets relate to the concept of a Boolean algebra in mathematical logic?

The power set of any set, equipped with the operations of union, intersection, and complement, forms a Boolean algebra. This provides a concrete example of Boolean algebras, which are fundamental in logic and computer science.

52. What is the role of power sets in defining the concept of a σ-field in probability theory?

In probability theory, a σ-field (or σ-algebra) is a subset of the power set of the sample space, closed under certain operations. The power set provides the largest possible σ-field for any given sample space.

53. How do power sets relate to the concept of a topology in terms of open sets?

A topology on a set X is defined as a collection of subsets of X (i.e., a subset of the power set of X) that includes both X and the empty set, and is closed under finite intersections and arbitrary unions.

54. What is the significance of power sets in the study of set-theoretic paradoxes?

Power sets play a crucial role in many set-theoretic paradoxes, such as Russell's paradox. These paradoxes often arise from considering "too large" collections, highlighting the complexities and limitations of naive set theory.

55. How do power sets relate to the concept of a universal set in set theory?

The concept of a universal set (a set containing all possible elements) is problematic in standard set theory because its power set would lead to contradictions. This limitation, related to power sets, led to the development of more sophisticated set theories.