Projection Formula & Overview

Projection is used to find the analyses of one side as per the other side. This concept is particularly useful in solving problems involving triangles, vectors, and their components. It connect the sides and angles of the triangle. The law of tangents or tangent rule is a statement about the relationship between the tangents of two angles of a triangle and the lengths of the opposing sides. The law of tangents can be used in any case where two sides and the included angle, or two angles and a side, are known. In real life, we use the Law of Tangents to calculate the angle of celestial objects such as stars, moon, etc.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

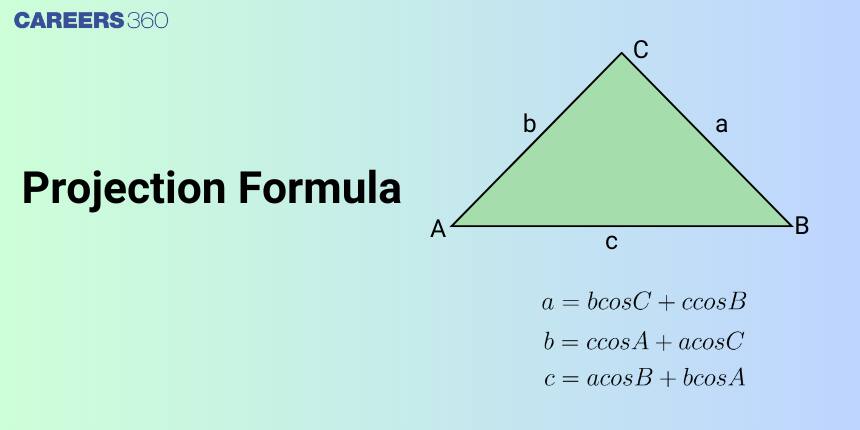
Projection Formula

In a geometric sense, projecting a vector onto another vector involves finding the shadow or footprint of one vector along the direction of another. Given two vectors a and $\mathbf{b}$, the projection of $\mathbf{a}$ onto $\mathbf{b}$ is a vector that lies along $\mathbf{b}$ and represents the component of $\mathbf{a}$ in the direction of b\mathbf\{b\}b.

In the $\triangle A B C$,
Projection of $A B$ on $B C$ is $B D$ and Projection of $A C$ on $B C$ is $D C$

Now,

$
\begin{aligned}
& B D=c \cos B \text { and } D C=b \cos C \\
& \text { and, } B C=a=B D+D C \\
& =c \cos B+b \cos C \\
& a=c \cos B+b \cos C
\end{aligned}
$

In a similar way, other projection formula can be derived
1. $a=c \cos B+b \cos C$
2. $b=c \cos A+a \cos C$
3. $c=b \cos A+a \cos B$

In any triangle ABC we have a

a sinA = b sinBb = c sinC = 2R ……………………. (1)

Now convert the above relation into sides in terms of angles in terms of the sides of any triangle.

a/sin A = 2R

⇒ a = 2R sin A ……………………. (2)

b/sin B = 2R

⇒ b = 2R sin B ……………………. (3)

c/sin c = 2R

⇒ c = 2R sin C ……………………. (4)

Case 1.

(i) a = b cos C + c cos B

Now, b cos C + c cos B

= 2R sin B cos C + 2R sin C cos B

= 2R sin (B + C)

= 2R sin (π - A), [Since, A + B + C = π]

= 2R sin A

= a [From (2)]

Therefore, a = b cos C + c cos B.

Case 2.

(ii) b = c cos A + a cos C

Now, c cos A + a cos C

= 2R sin C cos A + 2R sin A cos C

= 2R sin (A + C)

= 2R sin (π - B), [Since, A + B + C = π]

= 2R sin B

= b [From (3)]

Therefore, b = c cos A + a cos C.

Therefore, a = b cos C + c cos B.

Case 3.

(iii) c = a cos B + b cos A

Now, a cos B + b cos A

= 2R sin A cos B + 2R sin B cos A

= 2R sin (A + B)

= 2R sin (π - C), [Since, A + B + C = π]

= 2R sin C

= c [From (4)]

Therefore, c = a cos B + b cos A.

Therefore, a = b cos C + c cos B.

Recommended Video Based on Projection Formula

Solved Examples Based on Projection Formula

Example 1: In Triangle ABC , point D is on BC is such that $A D \perp B C$ and E is the middle point of BC and $b^2+2 a^2=c^2$ distance between D and E is?
1) b
2) $\frac{a+b+c}{2}$
3) $\frac{b+c}{2}$
4) a

Solution:

$\begin{aligned}
D E & =E C-D C \\
& =\frac{a}{2}-b \cos C \\
& =\frac{a}{2}-b \frac{a^2+b^2-c^2}{2 a b} \\
& =\frac{a}{2}-b \frac{a^2-\left(c^2-b^2\right)}{2 a b} \\
& =\frac{a}{2}-\frac{a^2-\left(2 a^2\right)}{2 a} \\
& =a
\end{aligned}$

Example 2: In triangle $A B C$ if
$a+c-\frac{3 b}{2}=a \sin ^2\left(\frac{C}{2}\right)+c \sin ^2\left(\frac{A}{2}\right)$ then a,b,c are in ?

1) A.P.

2) G.P.

3) H.P.

4) None of these

Solution

Diagram-

$\begin{aligned}
& \quad a+c-\frac{3 b}{2}=a \sin ^2\left(\frac{C}{2}\right)+c \sin ^2\left(\frac{A}{2}\right) \\
& a+c-\frac{3 b}{2}=a\left[\frac{1-\cos C}{2}\right]+c\left[\frac{1-\cos A}{2}\right] \\
& c \cos A+a \cos C=3 b-a-c \\
& b=3 b-a-c
\end{aligned}$

by above triangle $c \cos A+a \cos C=b$
a+c=2 b
$$

$a, b, c$ in $A . P$.
Example 3: The value of $b \cos C+c \cos B$ is:
1) $-a$
2) a
3) b
4) c

Solution
Given that,

$
b \cos C+c \cos B
$

Using the law of cosine,

$\begin{aligned}
& b \cos C+c \cos B=b\left[\frac{\left(a^2+b^2-c^2\right)}{2 a b}\right]+c\left[\frac{\left(c^2+a^2-b^2\right)}{2 c a}\right] \\
& b \cos C+c \cos B=\left[\frac{\left(a^2+b^2-c^2+c^2+a^2+b^2\right)}{2 a}\right]
\end{aligned}$

$
b \cos C+c \cos B=\frac{2 a^2}{2 a}
$

$b \cos C+c \cos B=a$
Hence, the answer is the option (2).

Example 4: If the sides of the $\triangle A B C$ are $a=3, b=2$ and $c=4$, then the value of $3 \cos B+2 \cos C$ is:
1) $\frac{4}{5}$
2) $-\frac{4}{5}$
3) $\frac{2}{5}$
4) $-\frac{2}{5}$

Solution
Given that,
$\begin{aligned}
& 3 \cos B+2 \cos C \\
& \cos B=\frac{a^2+c^2-b^2}{2 a c} \\
& \cos B=\frac{9+16-4}{24} \\
& \cos B=\frac{21}{24}
\end{aligned}$

$\begin{aligned}
& \cos C=\frac{a^2+b^2-c^2}{2 a b} \\
& \cos C=\frac{-1}{4}
\end{aligned}$
Therefore,

$\begin{aligned}
& 3 \cos B+2 \cos C=2 \times \frac{21}{24}+2 \times \frac{-1}{4} \\
& 3 \cos B+2 \cos C=\frac{21}{12}-\frac{1}{2} \\
& 3 \cos B+2 \cos C=\frac{4}{5}
\end{aligned}$
Hence, the answer is the option (1).

Example 5: If the area of the $\triangle A B C$ be $\Delta$, then the value of $b^2 \sin 2 C+c^2 \sin 2 B$ is:
1) $3 \Delta$
2) $2 \Delta$
3) $4 \Delta$
4) $5 \Delta$

Solution
Given that,

$
\begin{aligned}
& b^2 \sin 2 C+c^2 \sin 2 B \\
& b^2 \sin 2 C+c^2 \sin 2 B=b^2 2 \sin C \cos C+c^2 2 \sin B \cos B \\
& b^2 \sin 2 C+c^2 \sin 2 B=2 b \cos C \cdot b \sin C+2 c \cos B \cdot c \sin B
\end{aligned}
$

We know that,

$
\frac{a}{\sin B}=\frac{c}{\sin C} \Rightarrow b \sin C=c \sin B
$

Thus,

$
\begin{aligned}
& b^2 \sin 2 C+c^2 \sin 2 B=2 b \cos C \cdot c \sin B+2 c \cos B \cdot c \sin B \\
& b^2 \sin 2 C+c^2 \sin 2 B=2 c \sin B(b \cos C+c \cos B)
\end{aligned}
$

We know that,

$
a=b \cos C+c \cos B
$
Therefore,

$b^2 \sin 2 C+c^2 \sin 2 B=2 c \sin B \cdot a^{b^2 \sin 2 C+c^2 \sin 2 B=4 \cdot \frac{1}{2} a c \sin B} b^2 \sin 2 C+c^2 \sin 2 B=4 \Delta$

Hence, the answer is the option (3).