Roster and Set Builder Form

Sets are a foundational concept in mathematics, central to various fields such as statistics, geometry, and algebra. Roster and Set Builder sets are the basic elemental concepts in mathematics that one could use to explain real-life examples of the said topic. Consider a typical classroom scenario: suppose only a teacher who intends to classify learners based on their preferences in class contents would need such a relationship. Through this, she names a set of all the students who have an interest in mathematics to ensure she only tests the skills and knowledge of those who have an interest in the subject.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

In this article, we will cover the concept of the sets and their different forms. This concept falls under the broader category of sets relation and function, a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

It is a well-defined collection of distinct objects and it is usually denoted by capital letters $\mathrm{A}, \mathrm{B}$, $\mathrm{C}, \mathrm{S}, \mathrm{U}, \mathrm{V}$ $\qquad$
Example: $A=\{1,2,3\}$
All the objects that form a set are called its elements or members. These are usually denoted by small letters, i.e. $x, y, z \ldots$.

If $x$ is an element of a set $A$, we write $x \in A$ and read as ' $x$ belongs to $A$ '.
If $x$ is not an element of a set $A$, we write $x \notin A$ and read as ' $x$ does not belong to $A$ '.
Example: $A=\{1,2,3\}$, then $2 \in A(2$ belongs to set $A)$ and $4 \notin A(4$ does not belong to set $A)$

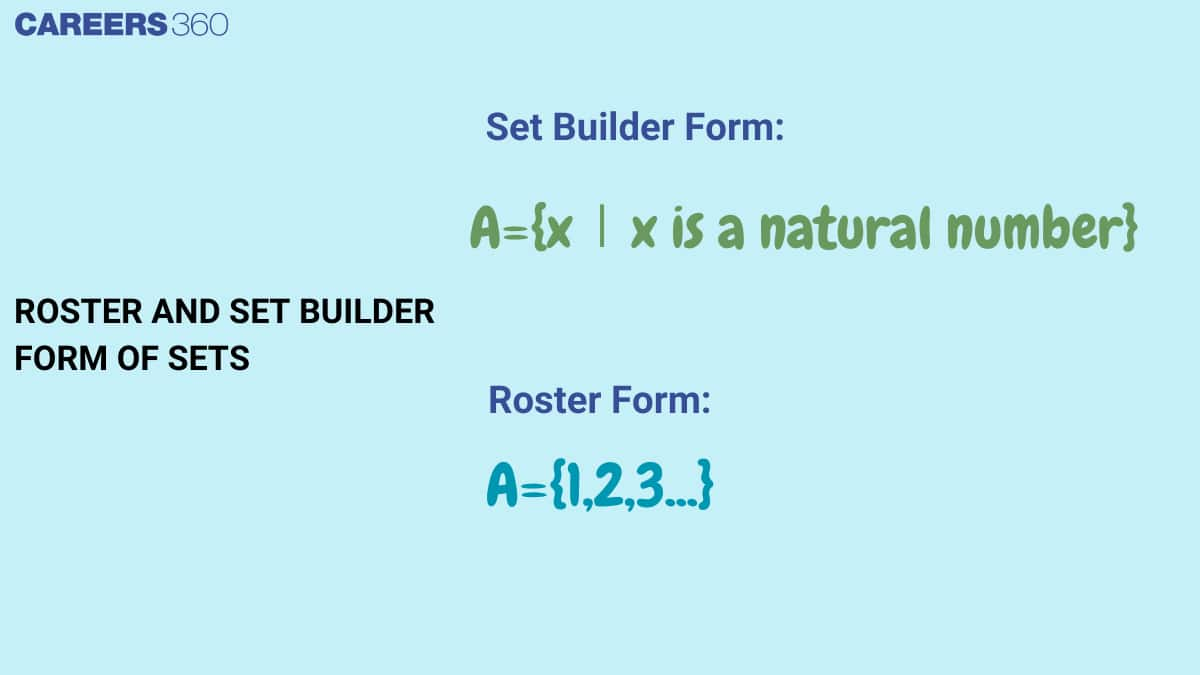
There are two methods of representing a set - Roster (or Tabular) form & Set-builder Form.

The Roster form is one way to specify a set, where the elements are listed explicitly within curly brackets. For example, the set of the first five natural numbers is expressed as $\{1,2,3,4,5\}$. This notation is straightforward and is commonly used when the elements of the set are finite and clear.

In roster form, all the elements of a set are listed, the elements are separated by commas and are enclosed within braces \{\} .

Example: $\{\mathrm{a}, \mathrm{e}, \mathrm{i}, \mathrm{o}, \mathrm{u}\}$ represents the set of all the vowels in the English alphabet in the roster from.

In roster form, the order in which the elements are listed is immaterial, i.e. the set of all natural numbers which divide 14 is $\{1,2,7,14\}$ can also be represented as $\{1,14,7,2\}$.

An element is not generally repeated in the roster form of a set, i.e., all the elements are taken as distinct. For example, the set of letters forming the word 'SCHOOL' is $\{\mathrm{S}, \mathrm{C}, \mathrm{H}, \mathrm{O}, \mathrm{L}\}$ or $\{\mathrm{H}$, $\mathrm{O}, \mathrm{L}, \mathrm{C}, \mathrm{S}\}$. Here, the order of listing elements has no relevance.

What is a Set-builder Form?

In set-builder form, all the elements of a set possess a single common property that is not possessed by any element outside the set. If Z contains all values of x for which the condition q(x) is true, then we write

$$
Z=\{x: q(x)\} \text { or } Z=\{x \mid q(x)\}
$$

Where, ': ' or '|' is read as 'such that'
eg. The $\operatorname{set} A=\{0,1,8,27,64, \ldots$.$\} can be written in Set Builder form as$
$A=\left\{x^3: X\right.$ is a non-negative integer $\}$

What is a Set-builder Form?
In set-builder form, all the elements of a set possess a single common property that is not possessed by any element outside the set. If $Z$ contains all values of $x$ for which the condition $q(x)$ is true, then we write

$$
Z=\{x: q(x)\} \text { or } Z=\{x \mid q(x)\}
$$

Where, ': ' or ' $\mid$ ' is read as 'such that'
eg. The $\operatorname{set} A=\{0,1,8,27,64, \ldots\}$ can be written in Set Builder form as
$A=\left\{x^3: X\right.$ is a non-negative integer $\}$

Summary

We concluded that the sets are the important aspects of mathematics that help to learn the foundational concept which helps in statistics and in other branches. The main application of sets and their different form is in various fields such as statistics, geometry, and algebra.

Recommended Video :

Solved Examples Based On the Sets, Roster, and Set Builder form of Sets:
Example 1: Which of the following sets differs from the other three?
1) $A=\{x: x$ is odd, $x \in Z\}$
2) $B=\{x: x$ is not divisible by $2, x \in Z\}$
3) $C=\{x: x$ is the half of an even integer $\}$
4) $D=\{x: x$ is not even, $x \in Z\}$

Solution:
Half of an even integer can be even as well as an odd integer. Eg: half of 4 is 2 .
All other options denote odd integers.
So, 3 is different from others.
Hence, the answer is the option 3.
Example 2: Which of the following is a set?
1) The list of all the bright colors.
2) The list of all the dull colors.
3) The list of all colors in the Rainbow.
4) The list of all the good colors.

Solution:
As we learned
$A$ set is a well-defined collection of objects. eg. $A=\{1,2,3\}$.
In this Question,
Bright, dull, and good colors are not well-defined as it is different for different people. But, the list of all colors in the rainbow is definite and well-defined. So, it is a set.

We can decide with respect to any color, say green, whether it will lie in the set or not. So, it is a well-defined collection. We cannot do this in case of bright, dull or good colors.

Hence, the answer is the option 3.
Example 3: Which of these sets are written in the Roster Form?
1) $A=\{x: x$ is a vowel $\}$
2) $B=\{a, e, i, o, u\}$
3) $\mathrm{C}=\{1<\mathrm{x}<2, \mathrm{x}$ is a natural number $\}$
4) $D=\{x: x$ is an even number $\}$

Solution:
As we learned, in roster form, all the elements of a set are listed, and the elements are separated by commas and are enclosed within braces \{\} .

Clearly, option 2 is written in roster form.
Hence, the answer is the option 2.

Example 5: Which of the following sets has an infinite number of elements?
1) $A=\{x: x$ is an odd number on dice $\}$
2) $B=\{x: x$ is a prime number $\}$
3) $\mathrm{C}=\{\mathrm{x}: \mathrm{x}$ is a factor of 24$\}$
4) $D=\{x: x$ is an even prime number $\}$

Example 4: Which of the following is not a set?
1) The collection of all licensed drivers in the class.
2) The collection of students in the class above the age 15 .
3) The collection of all the young students in the class.
4) The collection of all students with names starting from ' $A$ '.

Solution:
As we learned
A set is a well-defined collection of objects.
In this question,
"The collection of young students" is not a set because the term young is not well defined.
In all other options, we can identify the elements present in those collections, so they are sets.
Hence, the answer is the option 3.
Example 5: Which of the following sets has an infinite number of elements?
1) $A=\{x: x$ is an odd number on dice $\}$
2) $B=\{x: x$ is a prime number $\}$
3) $\mathrm{C}=\{\mathrm{x}: \mathrm{x}$ is a factor of 24$\}$
4) $D=\{x: x$ is an even prime number $\}$

Solution

Option $1=\{1,3,5\}$ : so finite number of elements.
Option $2=\{2,3,5,7, \ldots\}$ : so infinite number of elements.
Option $3=\{1,2,3,4,6,8,12,24\}$ : so finite number of elements.
Option $4=\{2\}$ : so finite number of elements.
Hence, the answer is the option 2.

Frequently Asked Questions(FAQ)-
1. What is a set?

Ans: A set is a collection of distinct objects, considered whole. These objects are called elements or members of the set.
2. What are the different forms of sets?

Ans: There are two forms in which we can represent the sets. They are:
Roster form: $\mathrm{A}=\{2,4,6,8,10,12,14,16,18\}$
Set builder form: $A=\{x: x=2 n, n \in N$ and $1 \leq n \leq 20\}$
3. What is the roster form of a set?

Ans: The Roster form is one way to specify a set, where the elements are listed explicitly within curly brackets.
4. Can you write a set in roster format and put it into set builder format? Ans: Yes, we can write.

5. What is the set-builder form?
Ans: The Set Builder form that uses a rule to specify the members of the set.