Solutions of Equations of the Form a cos Ө + b sin Ө = c

Introduction to Trigonometric Equations

Trigonometric equations involve trigonometric functions such as $\sin \theta$, $\cos \theta$, $\tan \theta$, $\cot \theta$, $\sec \theta$, and $\csc \theta$. These equations are satisfied only for specific angle values, which may be finite or infinite due to the periodic nature of trigonometric functions. For example, the equation $2 \sin x = 1$ has a solution $x = \pi/6$ in the interval $[0, \pi]$.

Trigonometric ratios are periodic functions:

• $\sin x$, $\cos x$, $\sec x$, $\csc x$ have period $2\pi$

• $\tan x$, $\cot x$ have period $\pi$

This periodicity allows us to generalize solutions of trigonometric equations using their respective periods.

Types of Solutions in Trigonometric Equations

Trigonometric equations have two main types of solutions: principal solutions within one period and general solutions that include all possible values using periodicity. Understanding these helps solve equations efficiently across different intervals.

Principal Solution

The solutions of a trigonometric equation lying in the interval $[0, 2\pi)$ are called principal solutions.
For example, if $2 \sin \theta = 1$, then $\theta = \pi/6$ and $5\pi/6$ are the principal solutions.

General Solution

Because trigonometric functions repeat periodically, an equation may have infinitely many solutions. The complete set of all possible solutions is called the general solution.
For $2 \sin \theta = 1$, the general solution is:

$\theta = \pi/6 + 2n\pi \quad \text{or} \quad \theta = 5\pi/6 + 2n\pi, \quad n \in \mathbb{Z}$

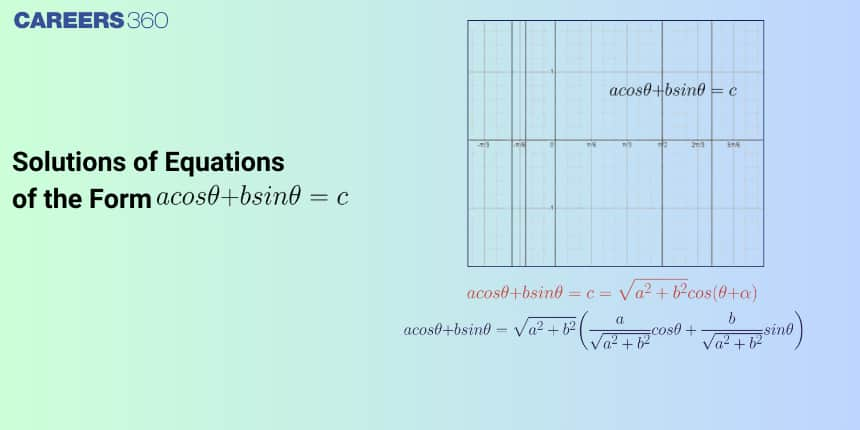
Solutions of Equations of the Form $a \cos \theta + b \sin \theta = c$

Equations of the form $a \cos \theta +b \sin \theta=c$ are solved by converting into a single trigonometric function using the identity $\sqrt{a^2+b^2}$. These solutions play a key role in simplifying trigonometric equations and finding general and specific angle values efficiently.

Transforming the Equation Using Auxiliary Angle

For equations of the type $a \cos \theta + b \sin \theta = c$, we can transform them using:

$a = r \cos \phi, \quad b = r \sin \phi, \quad r = \sqrt{a^2+b^2}, \quad \phi = \tan^{-1} \frac{b}{a}$

Then the equation becomes:

$r (\cos \phi \cos \theta + \sin \phi \sin \theta) = c \quad \Rightarrow \quad \cos(\theta - \phi) = \frac{c}{r}$

Using $A \sin \theta \pm B \cos \theta$ for simplification

Similarly, for expressions in the form $A \sin \theta \pm B \cos \theta$, we can rewrite:

$A \sin \theta \pm B \cos \theta = \sqrt{A^2 + B^2} \sin(\theta \pm \beta), \quad \tan \beta = \frac{B}{A}$

This standard formula is highly useful in trigonometry maximum and minimum value calculations and solving simultaneous trigonometric equations.

Conditions for Real Solutions

• If $|c| > \sqrt{a^2 + b^2}$, there is no real solution, since $\cos(\theta - \phi)$ must lie in $[-1,1]$.

• If $|c| \leq \sqrt{a^2 + b^2}$, put:

$\frac{|c|}{\sqrt{a^2+b^2}} = \cos \alpha$

Then the general solution is:

$\theta = \phi \pm \alpha + 2n\pi, \quad n \in \mathbb{Z}$

Working Rules for Solving $a \cos \theta + b \sin \theta = c$

1. First check if $|c| \leq \sqrt{a^2+b^2}$.

2. If $|c| > \sqrt{a^2+b^2}$, the equation has no real solution.

3. If $|c| \leq \sqrt{a^2+b^2}$, divide both sides by $r = \sqrt{a^2+b^2}$ and transform using the auxiliary angle method.

Solved Examples Based on Solutions of Equations of the Form a $\cos \theta+b \sin \theta=c$

Example 1: If $[\sin x]+[\sqrt{2} \cos x]=-3, x \in[0,2 \pi] {\text {( }[\text { ] }}$ denotes the greatest integer function) then $x$ belongs to

Solution:

$[\sin x] + [\sqrt{2} \cos x] = -3$

$\Rightarrow [\sin x] = -1 \text{ and } [\sqrt{2} \cos x] = -2$

or $-1 \leq \sin x < 0$

and $-2 \leq \sqrt{2} \cos x < -1$

or $-1 \leq \sin x < 0$

and $-\sqrt{2} < \cos x < -\frac{1}{\sqrt{2}}$

or $ -1 \leq \sin x < 0$

and $-1 \leq \cos x < -\frac{1}{\sqrt{2}}$

$\therefore \quad x \in (\pi, 2 \pi) \text{ and } x \in \left(\frac{3 \pi}{4}, \frac{5 \pi}{4}\right)$

$\therefore \quad x \in (\pi, 2 \pi) \cap \left(\frac{3 \pi}{4}, \frac{5 \pi}{4}\right)$

$\therefore x \in \left(\pi, \frac{5 \pi}{4}\right)$

Hence, the answer is $\left(\pi, \frac{5 \pi}{4}\right)$.

Example 2: The general solution of the equation $\sum_{r=1}^n \cos \left(r^2 x\right) \sin (r x)=\frac{1}{2}$.
Solution:

$\sum_{r=1}^n \cos \left(r^2 x\right) \sin (r x) = \frac{1}{2}$

$\Rightarrow \quad \sum_{r=1}^n 2 \cos \left(r^2 x\right) \sin (r x) = 1$

$\Rightarrow \sum_{r=1}^n [\sin \{r(r+1) x\} - \sin \{r(r-1) x\}] = 1$

$\Rightarrow \quad \sin \{n(n+1) x\} - \sin 0 = 1$

$\Rightarrow \quad \sin (n(n+1) x) = 1$

$\therefore \quad n(n+1) x = \left(2 m \pi + \frac{\pi}{2}\right), \quad m \in I$

$\therefore \quad x = \frac{(4 m + 1)}{n(n+1)} \cdot \frac{\pi}{2}, \quad m \in I$

$\frac{(4 m + 1)}{n(n+1)} \cdot \frac{\pi}{2}, \quad m \in I$

Example 3: Find the general solution of $\sec \theta+\sqrt{3} \tan \theta=1$
Solution:

$\sec \theta + \sqrt{3} \tan \theta = 1$

$\frac{1}{\cos \theta} + \sqrt{3} \frac{\sin \theta}{\cos \theta} = 1$

$\sqrt{3} \sin \theta - \cos \theta = -1$

$a = \sqrt{3}, \quad b = -1, \quad \sqrt{a^2 + b^2} = \sqrt{3 + 1} = 2$

Dividing the equation by 2:

$-\cos \left(\theta + \frac{\pi}{3}\right) = -\frac{1}{2}$

$\cos \left(\theta + \frac{\pi}{3}\right) = \cos \frac{\pi}{3}$

$\theta + \frac{\pi}{3} = 2 n \pi \pm \frac{\pi}{3}$

$\theta = 2 n \pi \pm \frac{\pi}{3} - \frac{\pi}{3}$

Hence, the answer is $2 n \pi \pm \frac{\pi}{3} - \frac{\pi}{3}$
Example 4: The general solution of the equation $\sqrt{3} \sin x+\cos x=1$ is:

Solution:

$\sqrt{3} \sin x + \cos x = 1$

$a = \sqrt{3}, \quad b = 1, \quad \sqrt{a^2 + b^2} = \sqrt{3 + 1} = 2$

Dividing the equation by 2:

$\frac{\sqrt{3}}{2} \sin x + \frac{1}{2} \cos x = \frac{1}{2}$

$\sin \frac{\pi}{3} \sin x + \cos \frac{\pi}{3} \cos x = \frac{1}{2}$

$\cos \left(x - \frac{\pi}{3}\right) = \cos \frac{\pi}{3}$

$x - \frac{\pi}{3} = 2 n \pi \pm \frac{\pi}{3}$

$x = 2 n \pi \pm \frac{\pi}{3} + \frac{\pi}{3}$

Hence, the answer is $2 n \pi \pm \frac{\pi}{3} + \frac{\pi}{3}$

Example 5: What is the Range of $t$ , where $t \epsilon[-\pi, \pi]$ and $\sin x+\sin (t+x)+\sin (t-x)=1$ has real roots?

Solution:

$\sin x + \sin (t + x) + \sin (t - x) = 1$

$\sin x + 2 \sin t \cdot \cos x = 1$

Here, $a = 2 \sin t$, $b = 1$, and $c = 1$.

For real roots, $|c| \leq \sqrt{a^2 + b^2}$.

$1 \leq \sqrt{1 + 4 \sin^2 t}$

$1 \leq \sqrt{1 + 4 \sin^2 t}$ can be squared (assuming both sides positive):

$1 \leq 1 + 4 \sin^2 t$

$\Rightarrow 4 \sin^2 t \geq 0$

$\Rightarrow \sin t \geq 0$

$\Rightarrow t \in [0, \pi]$

Hence, the answer is $[0, \pi]$.

List of Topics Related to the Solutions of Equations of the Form a cos Ө + b sin Ө = c

Below is the list of key topics related to solving equations of the form $a \cos \theta + b \sin \theta = c$, covering methods, solutions, and important identities.

NCERT Resources

Below is a list of NCERT resources for trigonometric functions, including Chapter 3 notes, solutions, and exemplar problems, useful for understanding concepts, practicing questions, and preparing effectively.

NCERT Class 11 Chapter 3 - Trigonometric Functions Notes

NCERT Class 11 solutions for Chapter 3 - Trigonometric Functions

NCERT Exemplar solutions for Class 11 Chapter 3 - Trigonometric Functions

Practice Questions based on Trigonometric Functions

Below is a set of practice questions based on topics related to trigonometric functions. These questions cover ratios, identities, series, and maximum-minimum value problems to help strengthen your understanding and problem-solving skills in trigonometry.