Trigonometric Ratios of Allied Angles

Allied Angles are a pair of angles whose sum or difference is a multiple of 90 degrees or π/2 radians. 40° and 140° are allied angles because their sum is 180°. So, Allied angles are a pair of angles. In real life, we use the Trigonometric Ratios of Allied Angles to measure the height of a building or mountain.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Trigonometric Ratios of Allied Angles
2. Algorithm to Find Trigonometric Ratios of Any Angle in Terms of Those of Positive Acute Angle
3. Solved Examples Based on Trigonometry Ratios of Allied Angles

In this article, we will cover the concept of Trigonometric Ratios of Allied Angles. This category falls under the broader category of Trigonometry, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Questions based on this topic have been asked frequently in JEE Mains.

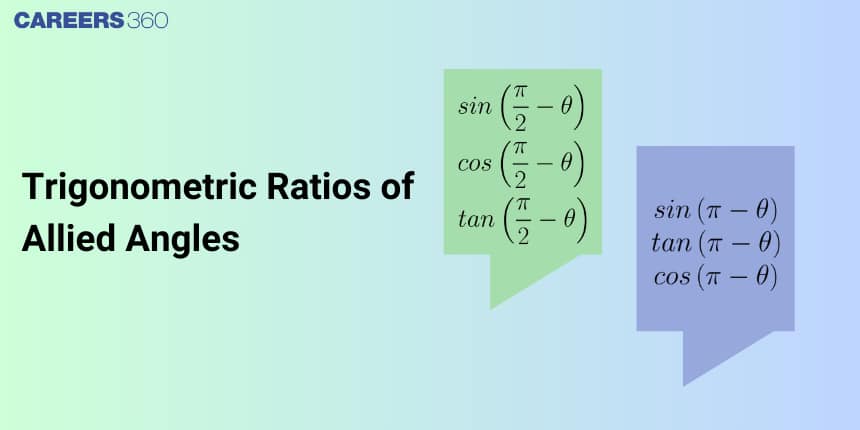
Trigonometric Ratios of Allied Angles

When the sum or difference of two angles is either zero or a multiple of $90^{\circ}$, then they are called allied angles. e.g., $30^{\circ}$ and $60^{\circ}$ are allied angles because their sum is $90^{\circ}$. Angles $-x, 90^{\circ} \pm x, 180^{\circ} \pm x$, and $360^{\circ} \pm x$, are angles allied to the angle $x$ if measured in degrees.

The Cartesian Plane is divided into four quadrants each comprising $90^{\circ}$. Different trigonometric ratios have distinct properties in these quadrants such as all T -ratios being positive in the 1 st quadrant which ranges from $0^{\circ}$ to $90^{\circ}$ and only sine and cosecant being positive in the 2nd quadrant, tan and cot being positive in 3rd quadrant and cos and sec being positive in 4th quadrant. Thus, when one angle transitions from one quadrant to another, the trigonometric ratio changes its sign or its complementary.
In general, for angle $x$, allied angles are $(n \times 90 \pm x)$ or $n * \pi / 2 \pm x, n \in$ I

Trigonometric Ratios of Allied Angles when n is even

- $\sin \left(180^{\circ}-\theta\right)=\sin (\theta)$
- $\cos \left(180^{\circ}-\theta\right)=-\cos (\theta)$
- $\tan \left(180^{\circ}-\theta\right)=-\tan (\theta)$
- $\csc \left(180^{\circ}-\theta\right)=\csc (\theta)$
- $\sec \left(180^{\circ}-\theta\right)=-\sec (\theta)$
- $\cot \left(180^{\circ}-\theta\right)=-\cot (\theta)$
- $\sin \left(180^{\circ}+\theta\right)=-\sin (\theta)$
- $\cos \left(180^{\circ}+\theta\right)=-\cos (\theta)$
- $\tan \left(180^{\circ}+\theta\right)=\tan (\theta)$
- $\csc \left(180^{\circ}+\theta\right)=-\csc (\theta)$
- $\sec \left(180^{\circ}+\theta\right)=-\sec (\theta)$
- $\cot \left(180^0+\theta\right)=\cot (\theta)$

Trigonometric Ratios of Allied Angles when n is odd

- $\sin \left(90^{-}-\theta\right)=\cos (\theta)$
- $\cos \left(90^{\circ}-\theta\right)=\sin (\theta)$
- $\tan \left(90^{\circ}-\theta\right)=\cot (\theta)$
- $\csc \left(90^0-\theta\right)=\sec (\theta)$
- $\sec \left(90^{\circ}-\theta\right)=\csc (\theta)$
- $\cot \left(90^{\circ}-\theta\right)=\tan (\theta)$
- $\sin \left(90^{\circ}+\theta\right)=\cos (\theta)$
- $\cos \left(90^{\circ}+\theta\right)=-\sin (\theta)$
- $\tan \left(90^{\circ}+\theta\right)=-\cot (\theta)$
- $\csc \left(90^{\circ}+\theta\right)=\sec (\theta)$
- $\sec \left(90^0+\theta\right)=-\csc (\theta)$
- $\cot \left(90^{\circ}+\theta\right)=-\tan (\theta)$

AID TO REMEMBER

1. All the trigonometric functions of a real number of the form $2 n(\pi / 2) \pm x(n \in I)(i$.e. an even multiple of $\pi / 2 \pm x)$ are numerically equal to the same function of $x$, with sign depending on the quadrant in which terminal side of the angles lies.

For example $\cos (\pi+x)=\cos (2(\pi / 2)+x)=-\cos (x)$, the -ve sign was chosen because $(\pi+x)$ lies in 3rd quadrant and 'cos' is -ve in third quadrant.
2. All the trigonometric functions of a real number of the form $(2 n+1) \pi / 2 \pm x(n \in I)$ (i.e. an even multiple of $\pi / 2 \pm x)$ is numerically equal to the co-function of $x$, with sign depending on the quadrant in which terminal side of the angles lies.

Note that 'sin' and 'cos' are co-functions of each other, 'tan' and 'cot' are co-functions of each other, and 'sec' and 'cosec' are co-functions of each other.
For example: $\sec (\pi / 2+x)=-\operatorname{cosec}(x)$, as $(\pi / 2+x)$ lies in the 2 nd quadrant and 'sec' is $-v e$ in $2 n d$ quadrant.

$\begin{array}{r|r|r|r|r|r|r} \hline \theta & \sin \theta & \operatorname{cosec} \theta & \cos \theta & \sec \theta & \tan \theta & \cot \theta \\ \hline -\theta & -\sin \theta & -\operatorname{cosec} \theta & \cos \theta & \sec \theta & -\tan \theta & -\cot \theta \\ 90^{\circ}-\theta & \cos \theta & \sec \theta & \sin \theta & \operatorname{cosec} \theta & \cot \theta & \tan \theta \\ 90^{\circ}+\theta & \cos \theta & \sec \theta & -\sin \theta & -\operatorname{cosec} \theta & -\cot \theta & -\tan \theta \\ 180^{\circ}-\theta & \sin \theta & \operatorname{cosec} \theta & -\cos \theta & -\sec \theta & -\tan \theta & -\cot \theta \\ 180^{\circ}+\theta & -\sin \theta & -\operatorname{cosec} \theta & -\cos \theta & -\sec \theta & \tan \theta & \cot \theta \\ 270^{\circ}-\theta & -\cos \theta & -\sec \theta & -\sin \theta & -\operatorname{cosec} \theta & \cot \theta & \tan \theta \\ 270^{\circ}+\theta & -\cos \theta & -\sec \theta & \sin \theta & \operatorname{cosec} \theta & -\cot \theta & -\tan \theta \\ 360^{\circ}-\theta & -\sin \theta & -\operatorname{cosec} \theta & \cos \theta & \sec \theta & -\tan \theta & -\cot \theta \\ \hline \end{array}$

Algorithm to Find Trigonometric Ratios of Any Angle in Terms of Those of Positive Acute Angle

Step I: See whether the given angle a is positive or negative if it is negative, make it positive as follows:

$
\sin (-X)=-\sin X, \cos (-X)=\cos X, \tan (-X)=-\tan X
$

Step II: Express the positive angle obtained in step I in the form $\mathrm{a}=90 \operatorname{deg}^* \mathrm{n} \pm \mathrm{X}$ where X is an acute angle.
Step III: Determine the quadrant in which the terminal side of the angle lies which determines the sign of the given trigonometric function.
Step IV: In step II, if n is an odd integer, then
$\sin a= \pm \cos X, \cos a= \pm \sin X, \tan a= \pm \cot X, \sec a=\operatorname{cosec}$, etc. The sign on RHS will be the sign obtained in step III.
In step II, if $n$ is even integer, then $\sin a= \pm \sin X, \cos a= \pm \cos X, \tan a= \pm \tan X, \sec a= \pm \sec X$, etc. The sign on RHS will be the sign obtained in step III.

Recommended Video Based on Trigonometry Rations of Allied Angles

Solved Examples Based on Trigonometry Ratios of Allied Angles

Example 1: The value of

$
\cos ^3\left(\frac{\pi}{8}\right) \cdot \cos \left(\frac{3 \pi}{8}\right)+\sin ^3\left(\frac{\pi}{8}\right) \cdot \sin \left(\frac{3 \pi}{8}\right) \text { is: }
$

[JEE MAINS 2020]
Solution
Using $\cos \left(\frac{3 \pi}{8}\right)=\sin \left(\frac{\pi}{2}-\frac{3 \pi}{8}\right)$

$
=\sin \left(\frac{\pi}{8}\right)
$

Now,

$
\begin{aligned}
& \cos ^3 \frac{\pi}{8} \cos \frac{3 \pi}{8}+\sin ^3 \frac{\pi}{8} \sin \frac{3 \pi}{8} \\
& =\cos ^3 \frac{\pi}{8} \sin \frac{\pi}{8}+\sin ^3 \frac{\pi}{8} \cos \frac{\pi}{8} \\
& =\sin \frac{\pi^2}{8} \cos \frac{\pi}{8}\left(\cos ^2 \frac{\pi}{8}+\sin ^2 \frac{\pi}{8}\right) \\
& =\frac{1}{2}\left(2 \sin \frac{\pi}{8} \cos \frac{\pi}{8}\right) \\
& =\frac{1}{2} \sin \frac{2 \pi}{8} \\
& =\frac{1}{2 \sqrt{2}}
\end{aligned}
$

Example 2: Let $f$ be an odd function defined on the set of real numbers such that for

$
\begin{aligned}
& x \geqslant 0 \\
& f(x)=3 \sin x+4 \cos x
\end{aligned}
$
Then $f(x)$ atx $=-\frac{11 \pi}{6}$ is equal to:
[JEE MAINS 2014]
Solution

$
\begin{aligned}
& f(x)=3 \sin x+4 \cos x \\
& f\left(\frac{-11 \pi}{6}\right)=3 \sin \left(\frac{-11 \pi}{6}\right)+4 \cos \left(\frac{-11 \pi}{6}\right) \\
& f\left(\frac{-11 \pi}{6}\right)=-3 \sin \left(\frac{11 \pi}{6}\right)+4 \cos \left(\frac{11 \pi}{6}\right) \\
& f\left(\frac{-11 \pi}{6}\right)=-3 \sin \left(2 \pi-\frac{\pi}{6}\right)+4 \cos \left(2 \pi-\frac{\pi}{6}\right) \\
& f\left(\frac{-11 \pi}{6}\right)=+3 \sin \left(\frac{\pi}{6}\right)+4 \cos \left(\frac{\pi}{6}\right) \\
& f\left(\frac{-11 \pi}{6}\right)=\frac{3}{2}+4 \frac{\sqrt{3}}{2}
\end{aligned}
$
Hence, the answer is $\frac{3}{2}+2 \sqrt{3}$

Example 3: The value of $\sin ^2\left(1^{\circ}\right)+\sin ^2\left(2^{\circ}\right) \ldots .+\sin ^2\left(90^{\circ}\right)$ is
Solution

Allied Angles
- $\sin (90-\theta)=\cos (\theta)$
- $\cos (90-\theta)=\sin (\theta)$

Now,

$
\begin{aligned}
& \sin ^2\left(1^o\right)+\sin ^2\left(2^{\circ}\right) \ldots .+\sin ^2\left(90^{\circ}\right) \\
& =\sin ^2\left(1^{\circ}\right)+\sin ^2\left(2^o\right) \ldots \sin ^2\left(44^{\circ}\right)+\sin ^2\left(45^{\circ}\right)+\cos ^2\left(44^{\circ}\right)+\ldots+\cos ^2\left(1^{\circ}\right)+\cos ^2\left(0^{\circ}\right)
\end{aligned}
$
Rearranging

$
\begin{aligned}
& =\left[\sin ^2\left(1^{\circ}\right)+\cos ^2\left(1^{\circ}\right)\right]+\left[\sin ^2\left(2^{\circ}\right)+\cos ^2\left(2^{\circ}\right)\right]+\ldots+\left[\sin ^2\left(44^{\circ}\right)+\cos ^2\left(44^{\circ}\right)\right]+ \\
& \sin ^2\left(45^{\circ}\right)+\cos ^2\left(0^{\circ}\right) \\
& =44+\frac{1}{2}+1 \\
& =45 \frac{1}{2}
\end{aligned}
$
Hence, the answer is 45.5 .

Example 4:The value of $\frac{\sin 37^{\circ}}{\cos 53^{\circ}}+\frac{\cos 53^{\circ}}{\sin 37^{\circ}}$ is
Solution
Allied Angles
- $\sin (90-\theta)=\cos (\theta)$
- $\cos (90-\theta)=\sin (\theta)$

Now,

$
\begin{aligned}
& =\frac{\sin 37^{\circ}}{\cos 53^{\circ}}+\frac{\cos 53^{\circ}}{\sin 37^{\circ}} \\
& =\frac{\sin (90-53)^{\circ}}{\cos 53^{\circ}}+\frac{\cos (90-37)^{\circ}}{\sin 37^{\circ}} \\
& =\frac{\cos 53^{\circ}}{\cos 53^{\circ}}+\frac{\sin 37^{\circ}}{\sin 37^{\circ}} \\
& =2
\end{aligned}
$
Hence, the answer is 2 .

Example 5: The value of the expression

$
\sin (-\theta)+\cos (-\theta)+\cot (-\theta) \times \tan (-\theta) \text { is }
$

Solution
We know, signs of Trigonometric Functions
In the fourth quadrant, the angle is measured as negative, $x$ is positive and $y$ is negative, so only $\cos \theta$ and $\sec \theta$ are positive.
Since,

$
\begin{aligned}
& \sin (-\theta)=-\sin \theta \\
& \cos (-\theta)=\cos \theta \\
& \cot (-\theta)=-\cot \theta
\end{aligned}
$

$
\text { and } \tan (-\theta)=-\tan \theta
$
So, the given expression becomes

$
\begin{aligned}
& -\sin \theta+\cos \theta+\cot \theta \cdot \tan \theta \\
& =-\sin \theta+\cos \theta+1
\end{aligned}
$
Hence, the answer is $-\sin \theta+\cos \theta+1$