Trigonometric Ratios of Compound Angles

The algebraic sum of 2 or more angles can be called a compound angle. Trigonometric identities are used to represent compound angles. Trigonometric ratios of compound angles have diverse applications in science and technology. In real life, we use the Trigonometric Ratios of Allied Angles to measure the angle of the roof, creating parallel and perpendicular walls.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

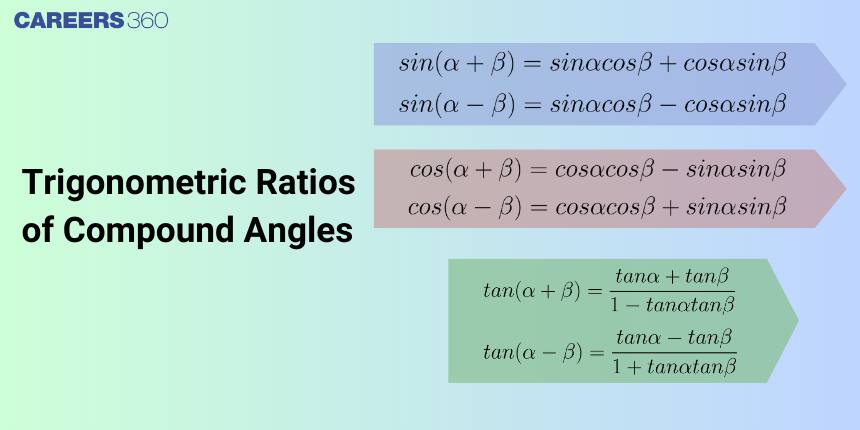
In this article, we will cover the concept of Trigonometric Ratio for Compound Angles. This category falls under the broader category of Trigonometry, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of twelve questions have been asked on this concept, including one in 2015, one in 2017, one in 2019, one in 2020, two in 2021, two in 2022 and three in 2023.

Compound Angles

The sum or difference of two or more angles is called a compound angle. If $A, B$, and $C$ are any angle then $A+B, A-B, A+B+C, A+B-C$, etc all are compound angles.

Trigonometric Ratio for Compound Angles

Trignometric Ratios of compound angles are given below:
1. $\cos (\alpha-\beta)=\cos \alpha \cos \beta+\sin \alpha \sin \beta$
2. $\cos (\alpha+\beta)=\cos \alpha \cos \beta-\sin \alpha \sin \beta$
3. $\sin (\alpha-\beta)=\sin \alpha \cos \beta-\cos \alpha \sin \beta$
4. $\sin (\alpha+\beta)=\sin \alpha \cos \beta+\cos \alpha \sin \beta$
5. $\tan \alpha+\tan \beta=\frac{\tan \alpha+\tan \beta}{1-\tan \alpha \tan \beta}$

6. $\tan \alpha-\tan \beta=\frac{\tan \alpha-\tan \beta}{1+\tan \alpha \tan \beta}$

7. $
\cot \alpha-\cot \beta=\frac{\cot \alpha \cot \beta-1}{\cot \alpha+\cot \beta}
$

8. $
\cot \alpha-\cot \beta=\frac{\cot \alpha \cot \beta+1}{\cot \alpha-\cot \beta}
$
Derivations of compound angles

1. $\cos (\alpha-\beta)$

Let's consider two points on the unit circle. Point $P$ is at an angle $\alpha$ from the positive $x$-axis with coordinates ( $\cos \alpha, \sin \alpha$ ) and point $Q$ is at an angle of $\beta$ from the positive $x$-axis with coordinates ( $\cos \beta$, sin $\beta$ ). Note the measure of angle POQ is $\alpha-\beta$. Label two more points: $A$ at an angle of $(\alpha-\beta)$ from the positive $x$-axis with coordinates $(\cos (\alpha-\beta)$, $\sin (\alpha-\beta))$; and point $B$ with coordinates $(1,0)$. Triangle $P O Q$ is a rotation of triangle $A O B$ and thus the distance from $P$ to $Q$ is the same as the distance from $A$ to $B$.

We can find the distance from $P$ to $Q$ using the distance formula.

$
\begin{aligned}
d_{P Q} & =\sqrt{(\cos \alpha-\cos \beta)^2+(\sin \alpha-\sin \beta)^2} \\
& =\sqrt{\cos ^2 \alpha-2 \cos \alpha \cos \beta+\cos ^2 \beta+\sin ^2 \alpha-2 \sin \alpha \sin \beta+\sin ^2 \beta}
\end{aligned}
$

Similarly, using the distance formula we can find the distance from A to B.

$
\begin{aligned}
& d_{A B}=\sqrt{(\cos (\alpha-\beta)-1)^2+(\sin (\alpha-\beta)-0)^2} \\
& \quad=\sqrt{\cos ^2(\alpha-\beta)-2 \cos (\alpha-\beta)+1+\sin ^2(\alpha-\beta)} \\
& =\sqrt{\left(\cos ^2(\alpha-\beta)+\sin ^2(\alpha-\beta)\right)-2 \cos (\alpha-\beta)+1} \\
& =\sqrt{1-2 \cos (\alpha-\beta)+1} \\
& =\sqrt{2-2 \cos (\alpha-\beta)}
\end{aligned}
$

Because the two distances are the same, we set them equal to each other and simplify

$
\begin{aligned}
\sqrt{2-2 \cos \alpha \cos \beta-2 \sin \alpha \sin \beta} & =\sqrt{2-2 \cos (\alpha-\beta)} \\
2-2 \cos \alpha \cos \beta-2 \sin \alpha \sin \beta & =2-2 \cos (\alpha-\beta)
\end{aligned}
$

Finally, we subtract $2$ from both sides and divide both sides by $-2$

$
\cos \alpha \cos \beta+\sin \alpha \sin \beta=\cos (\alpha-\beta)
$

2. $\cos (\alpha+\beta)$

$
\begin{aligned}
& =\cos (\alpha-(-\beta)) \\
& =\cos \alpha \cos (-\beta)+\sin \alpha \sin (-\beta) \\
& =\cos \alpha \cos \beta-\sin \alpha \sin \beta
\end{aligned}
$

Hence, $\cos (\alpha+\beta)=\cos \alpha \cos \beta-\sin \alpha \sin \beta$

3. Sine Compound Angle Formulae

If $A$ and $B$ are angles,

$
\begin{aligned}
& \sin (A+B) \sin (A-B)=\sin ^2 A-\sin ^2 B \\
& \sin (A-B)=\sin A \cos B-\cos A \sin B
\end{aligned}
$

Proof
We have

$
\begin{aligned}
\sin (\alpha-\beta) & =\cos \left(90^{\circ}-(\alpha-\beta)\right) \\
& =\cos \left(\left(90^{\circ}-\alpha\right)+\beta\right) \\
& =\cos \left(90^{\circ}-\alpha\right) \cos \beta-\sin \left(90^{\circ}-\alpha\right) \sin \beta \\
& =\sin \alpha \cos \beta-\cos \alpha \sin \beta \\
\sin (\alpha+\beta) & =\sin (\alpha-(-\beta)) \\
& =\sin \alpha \cos (-\beta)-\cos \alpha \sin (-\beta) \\
& =\sin \alpha \cos \beta+\cos \alpha \sin \beta
\end{aligned}
$

4. Tangent compound angle formulae

$
\begin{aligned}
& \tan (\alpha+\beta)=\frac{\tan \alpha+\tan \beta}{1-\tan \alpha \tan \beta} \\
& \tan (\alpha-\beta)=\frac{\tan \alpha-\tan \beta}{1+\tan \alpha \tan \beta}
\end{aligned}
$

Proof:
Finding the sum of two angles formula for tangent involves taking the quotient of the sum formulas for sine and cosine and simplifying,

$
\begin{aligned}
\tan (\alpha+\beta) & =\frac{\sin (\alpha+\beta)}{\cos (\alpha+\beta)} \\
& =\frac{\sin \alpha \cos \beta+\cos \alpha \sin \beta}{\cos \alpha \cos \beta-\sin \alpha \sin \beta}
\end{aligned}
$

[Divide the numerator and denominator by $\cos \alpha \cos \beta$ ]

$
\begin{aligned}
& =\frac{\frac{\sin \alpha \cos \beta+\cos \alpha \sin \beta}{\cos \alpha \cos \beta}}{\frac{\cos \alpha \cos \beta-\sin \alpha \sin \beta}{\cos \alpha \cos \beta}} \\
& =\frac{\frac{\sin \alpha}{\cos \alpha}+\frac{\sin \beta}{\cos \beta}}{1-\frac{\sin \alpha \sin \beta}{\cos \alpha \cos \beta}} \\
& =\frac{\tan \alpha+\tan \beta}{1-\tan \alpha \tan \beta}
\end{aligned}
$

For the difference of tangent, put - $\beta$ in place of $\beta$ in the above result

5. Cot Formulae

$
\begin{aligned}
& \cot (\alpha+\beta)=\frac{\cot \alpha \cot \beta-1}{\cot \alpha+\cot \beta} \\
& \cot (\alpha-\beta)=\frac{\cot \alpha \cot \beta+1}{\cot \beta-\cot \alpha}
\end{aligned}
$

Proof:

$
\begin{aligned}
\cot (\alpha+\beta) & =\frac{\cos (\alpha+\beta)}{\sin (\alpha+\beta)} \\
& =\frac{\cos \alpha \cos \beta-\sin \alpha \sin \beta}{\sin \alpha \cos \beta+\cos \alpha \sin \beta} \\
& =\frac{\frac{\cos \alpha \cos \beta-\sin \alpha \sin \beta}{\sin \alpha \sin \beta}}{\frac{\sin \alpha \cos \beta+\cos \alpha \sin \beta}{\sin \alpha \sin \beta}}
\end{aligned}
$

[Divide the numerator and denominator by $\sin \alpha \sin \beta$ ]

$
\begin{aligned}
& =\frac{\frac{\cos \alpha \cos \beta}{\sin \alpha \sin \beta}-\frac{\sin \alpha \sin \beta}{\sin \alpha \sin \beta}}{\frac{\sin \alpha \cos \beta}{\sin \alpha \sin \beta}+\frac{\cos \alpha \sin \beta}{\sin \alpha \sin \beta}} \\
& =\frac{\cot \alpha \cot \beta-1}{\cot \beta+\cot \alpha}
\end{aligned}
$

For the difference of cotangent, put - $\beta$ in place of $\beta$ in the above result

$
\begin{aligned}
\cot (\alpha+\beta) & =\frac{\cos (\alpha+\beta)}{\sin (\alpha+\beta)} \\
& =\frac{\cos \alpha \cos \beta-\sin \alpha \sin \beta}{\sin \alpha \cos \beta+\cos \alpha \sin \beta} \\
& =\frac{\frac{\cos \alpha \cos \beta-\sin \alpha \sin \beta}{\sin \alpha \sin \beta}}{\frac{\sin \alpha \cos \beta+\cos \alpha \sin \beta}{\sin \alpha \sin \beta}}
\end{aligned}
$

[Divide the numerator and denominator by $\sin \alpha \sin \beta$ ]

$
\begin{aligned}
& =\frac{\frac{\cos \alpha \cos \beta}{\sin \alpha \sin \beta}-\frac{\sin \alpha \sin \beta}{\sin \alpha \sin \beta}}{\frac{\sin \alpha \cos \beta}{\sin \alpha \sin \beta}+\frac{\cos \alpha \sin \beta}{\sin \alpha \sin \beta}} \\
& =\frac{\cot \alpha \cot \beta-1}{\cot \beta+\cot \alpha}
\end{aligned}
$

For the difference of cotangent, put - $\beta$ in place of $\beta$ in the above result

Some more results

1.

2.

3.

Transformation of products into sum or difference of sines and cosines

(a) 2 sin A cos B = sin (A+B) + sin (A-B)

(b) 2 cos A cos B = cos (A+B) + cos (A-B)

(c) 2 cos A sin B = sin (A+B) – sin (A-B)

(d) 2 sin A sin B = cos (A-B) – cos (A+B)

Multiple and submultiple angles

(a) sin 2A = 2 sin A cos A = 2 tan A/(1+tan²A)

(b) cos 2A = cos²A – sin²A = 2cos²A-1 = 1-2 sin²A

(c) tan 2A = 2 tan A/(1-tan²A)

(d) sin 3A = 3 sinA – 4 sin³A

(e) cos 3A = 4 cos³A – 3 cos A

(f) tan 3A = (3 tan A- tan³A)/(1-3tan²A)

Summary

Compound angle formulas are powerful tools for analyzing and manipulating trigonometric functions. With the help of these formulas, we can find the sum and difference between trignometric identities. Mastery of these identities enhances problem-solving skills and deepens the understanding of trigonometry's applications across various disciplines.

Solved Examples Based on Trignometric ratios of compound angles

Example 1: The value of $\tan 9^{\circ}-\tan 27^{\circ}-\tan 63^{\circ}+\tan 81^{\circ}$ is
[JEE MAINS 2023]
1) 4
2)3
3)2
4)1

Solution

$
\begin{aligned}
& \left(\tan 9^{\circ}+\cot 9^{\circ}\right)-\left(\tan 27^{\circ}+\cot 27^{\circ}\right) \\
& \frac{1}{\sin 9^{\circ} \cos 9^{\circ}}-\frac{1}{\sin 27^{\circ} \cos 27^{\circ}} \\
& \frac{2}{\sin 18^{\circ}}-\frac{2}{\sin 54^{\circ}} \\
& \frac{2(4)}{\sqrt{5}-1}-\frac{2(4)}{(\sqrt{5}+1)} \\
& \frac{8(\sqrt{5}+1)}{4}-\frac{8(\sqrt{5}-1)}{4} \\
& 2[(\sqrt{5}+1)-(\sqrt{5}-1)] \\
& =4
\end{aligned}
$

Hence, the answer is the option 4.

Example 2:

Example 2: |f $\qquad$
[JEE MAINS 2023]
1)2
2) $4-2 \sqrt{3}$
3) $5-\frac{3}{2} \sqrt{3}$
4) 4

Solution

$
\begin{aligned}
& \tan 15^{\circ}+\frac{1}{\tan 75^{\circ}}+\frac{1}{\tan 105^{\circ}}+\tan 195^{\circ}=2 \mathrm{a} \\
& \Rightarrow \tan 15^{\circ}+\frac{1}{\cot 15^{\circ}}-\frac{1}{\cot 15^{\circ}}+\tan 15^{\circ}=2 \mathrm{a} \\
& \Rightarrow \tan 15^{\circ}+\tan 15^{\circ}-\tan 15^{\circ}+\tan 15^{\circ}=2 \mathrm{a} \\
& \Rightarrow 2 \tan 15^{\circ}=2 \mathrm{a} \\
& \Rightarrow \mathrm{a}=\tan 15^{\circ} \\
& \mathrm{a}+\frac{1}{\mathrm{a}}=\tan 15^{\circ}+\frac{1}{\tan 15^{\circ}} \\
& =\tan 15^{\circ}+\cot 15^{\circ} \\
& =2-\sqrt{3}+2+\sqrt{3} \\
& \Rightarrow \mathrm{a}+\frac{1}{\mathrm{a}}=4
\end{aligned}
$

Example 4:
If $\cot \alpha=1$ and $\sec \beta=-\frac{5}{3}, \underline{\text { where }} \pi<\alpha<\frac{3 \pi}{2}$ and $\frac{\pi}{2}<\beta<\pi$, then the value of $\tan (\alpha+\beta)$ and the quadrant in which $\alpha+\beta$ lies, respectively are : [JEE MAINS 2022]
1) $-\frac{1}{7}$ and $I V^{\text {th }}$ quadrant
2) 7 and $I^{\text {st }}$ quadrant
3) ${ }^{-7}$ and $I V^{\text {th }}$ quadrant
$\frac{1}{7}$ and $\mathrm{I}^{\text {st }}$ quadrant
Solution
$\cot \alpha=1$
$\Rightarrow \tan \alpha=1$
and $\sec \beta=-\frac{5}{3}$
$\Rightarrow \tan \beta=-\frac{4}{3}$ (As $\beta \in \mathbb{I I}$ quadrant $)$
$\therefore \tan (\alpha+\beta)=\frac{\tan \alpha+\tan \beta}{1-\tan \alpha \cdot \tan \beta}$

$
=\frac{1-\frac{4}{3}}{1+\frac{4}{3} \cdot 1}=-\frac{1}{7}
$

As $\pi<\alpha<\frac{3 \pi}{2}$ and $\frac{\pi}{2}<\beta<\pi$
$\Rightarrow \quad \frac{3 \pi}{2}<\alpha+\beta<\frac{5 \pi}{2} \Rightarrow$ IV or I quadrant
Also $\tan (\alpha+\beta)<0 \Rightarrow$ IV th quadrant
Hence the answer is option 1.

Example 5: Let be defined as
$f(x+y)+f(x-y)=2 f(x) f(y), f\left(\frac{1}{2}\right)=-1$.
1) $\operatorname{cosec}^2(21) \cos (20) \cos (2)$
2) $\sec ^2(1) \sec (21) \cos (20)$
3) $\operatorname{cosec}^2(1) \operatorname{cosec}(21) \sin (20)$
4) $\sec ^2(21) \sin (20) \sin (2)$

Solution
$f(x+y)+f(x-y)=2 f(x) \cdot f(y)$
clearly $f(x)=\cos (a x)$
Then, the value of $\sum_{k=1}^{20} \frac{1}{\sin (k) \sin (k+f(k))}$ is equal to
[JEE MAINS 2021]

Now $f\left(\frac{1}{2}\right)=-1$

$
\begin{aligned}
& \Rightarrow-1=\cos \left(-\frac{a}{2}\right) \\
& \Rightarrow \quad-\frac{a}{2}=\pi \Rightarrow a=-2 \pi \\
& f(x)=\cos (-2 \pi x)
\end{aligned}
$
For $k \in N \Rightarrow f(k)=1$.

$
\begin{aligned}
& \text { Now, } \frac{1}{\sin (k) \cdot \sin (k+f(k)} \\
& =\frac{1}{\sin (k) \cdot \sin (k+1)} \\
& =\frac{\sin ((k+1)-k)}{\sin 1(\sin (k) \cdot \sin (k+1))} \\
& =\frac{1}{\sin 1} \cdot(\cot (k)-\cot (k+1)) \\
& \therefore \text { Required sum }=\frac{1}{\sin 1}(\cot 1-\cot 21) \\
& =\frac{1}{\sin 1}\left(\frac{\cos 1}{\sin 1}-\frac{\cos 21}{\sin 21}\right)
\end{aligned}
$

$
\begin{aligned}
& =\frac{\sin (21-1)}{\sin ^2 1 \cdot \sin 21} \\
& =\operatorname{cosec}^2(1) \operatorname{cosec}(21) \cdot \sin (20)
\end{aligned}
$
Hence, the answer is the option 3.

Frequently Asked Questions (FAQs)
Q1)What are compound angles?
Answer: The sum or difference of two or more angles is called a compound angle. If $A, B$, and $C$ are any angle then $A+B, A-B, A+B+C, A+B-C$ etc all are compound angles.
Q2)Give sine compound angle formula.
Answer: Sine compound angle formulas are given by

$
\begin{aligned}
& \sin (\alpha-\beta)=\sin \alpha \cos \beta-\cos \alpha \sin \beta \\
& \sin (\alpha+\beta)=\sin \alpha \cos \beta+\cos \alpha \sin \beta
\end{aligned}
$

Q3)Proof tangent compound addition angle formula?
Answer: Finding the sum of two angles formula for tangent involves taking the quotient of the sum formulas for sine and cosine and simplifying,

$
\begin{aligned}
\tan (\alpha+\beta) & =\frac{\sin (\alpha+\beta)}{\cos (\alpha+\beta)} \\
& =\frac{\sin \alpha \cos \beta+\cos \alpha \sin \beta}{\cos \alpha \cos \beta-\sin \alpha \sin \beta}
\end{aligned}
$

Q4) Give the cos compound angle formula.|
Answer: The Cos compound angle formula is given by

$
\begin{aligned}
& \cos (\alpha-\beta)=\cos \alpha \cos \beta+\sin \alpha \sin \beta \\
& \cos (\alpha+\beta)=\cos \alpha \cos \beta-\sin \alpha \sin \beta
\end{aligned}
$

Q5)What is $\sin (A+B) \sin (A-B)$ ?
Answer:

$
\begin{aligned}
\sin (A+B) \sin (A-B) & =(\sin A \cos B+\cos A \sin B)(\sin A \cos B-\cos A \sin B) \\
& =\sin ^2 A \cos ^2 B-\cos ^2 A \sin ^2 B \\
& =\sin ^2 A\left(1-\sin ^2 B\right)-\left(1-\sin ^2 A\right) \sin ^2 B \\
& =\sin ^2 A-\sin ^2 A \sin ^2 B-\sin ^2 B+\sin ^2 A \sin ^2 B=\sin ^2 A-\sin ^2 B \\
& =\left(1-\cos ^2 A\right)-\left(1-\cos ^2 B\right)=\cos ^2 B-\cos ^2 A
\end{aligned}
$