Truth Table: AND, OR, NAND, NOR, Conditional and Bi-conditional

In mathematical logic and philosophy, a statement or proposition is a declarative sentence that is either true or false. The truth value of a statement indicates whether it is true (denoted by "$T$") or false (denoted by "$F$"). This binary nature of truth values is fundamental to logical reasoning, enabling us to analyze and construct complex logical expressions systematically.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

Truth Value of a Statement

As we know that a statement is either true or false. The truth or falsity of a statement is called truth value.

If the statement is true, then truth value is “$T$”

If the statement is false, then truth value is “$F$”

Truth Table

A table indicating the truth value of one or more statements is called a truth table.

Truth table of one statement ‘$p$’ is

$\begin{array}{|c|}\hline \mathrm{\;\;\;\;\;}p\mathrm{\;\;\;\;\;}\mathrm{\;\;\;} \\ \hline \mathrm{T}\\ \hline \mathrm{F} \\ \hline\end{array}$

Truth table for two statement ‘$p$’ and ‘$q$’ is

$\begin{array}{|c|c|}\hline \mathrm{\;\;\;\;\;}p\mathrm{\;\;\;\;\;}&\mathrm{\;\;\;\;\;}q\mathrm{\;\;\;\;\;} \\ \hline \mathrm{T}& \mathrm{T} \\ \hline \mathrm{T}&\mathrm{F} \\ \hline \mathrm{F}&\mathrm{T}\\\hline \mathrm{F}&\mathrm{F} \\ \hline\end{array}$

In the case of n statements, there are $2^n $ distinct possible arrangements of truth values of statements.

Truth Table for Negation of a Statement

The truth value of the negation of a statement is always opposite to the truth value of the original statement.

$\begin{array}{|c|c|}\hline \mathrm{\;\;\;\;\;}p\mathrm{\;\;\;\;\;}&\mathrm{\;\;\;}\sim p\mathrm{\;\;\;\;\;} \\ \hline \mathrm{T}& \mathrm{F} \\ \hline \mathrm{F}&\mathrm{T} \\ \hline\end{array}$

Truth Table of Conjunction and Disjunction:

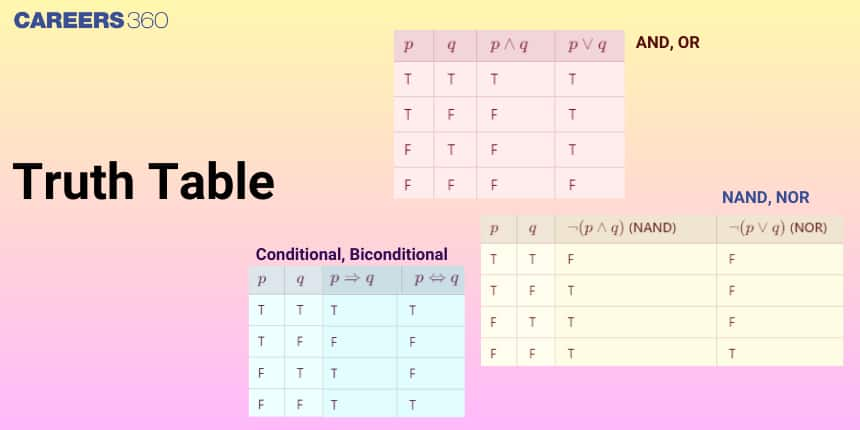
$\begin{array}{|c|c|c|c|c|c|c|c|c|c|}\hline \mathrm{\;\;\;\;\;}p\mathrm{\;\;\;\;\;}&\mathrm{\;\;\;}\sim p\mathrm{\;\;\;}&\mathrm{\;\;\;\;\;}q\mathrm{\;\;\;\;\;}&\mathrm{\;\;\;}\sim q\mathrm{\;\;\;} &\mathrm{\;\;\;}p\wedge q\mathrm{\;\;}&\mathrm{\;\;\;}\sim p\wedge \sim q \mathrm{\;\;}&\mathrm{\;\;\;}p\vee q\mathrm{\;\;}&\mathrm{\;\;\;}\sim\left (p\vee q \right )\mathrm{\;\;} \\ \hline \mathrm{T}&\mathrm{F} & \mathrm{T} &\mathrm{F}&\mathrm{T}&\mathrm{F} &\mathrm{T}&\mathrm{F}\\ \hline \mathrm{T}&\mathrm{F} & \mathrm{F} &\mathrm{T}&\mathrm{F}&\mathrm{F} & \mathrm{T}&\mathrm{F}\\ \hline \mathrm{F}&\mathrm{T} & \mathrm{T} &\mathrm{F}&\mathrm{F}&\mathrm{F} & \mathrm{T}&\mathrm{F}\\ \hline \mathrm{F}&\mathrm{T} & \mathrm{F} &\mathrm{T}&\mathrm{F}&\mathrm{T}& \mathrm{F}&\mathrm{T} \\ \hline\end{array}$

Negation of a Negation

Negation of negation of a statement is the statement itself. Equivalently, we write: $\sim (\sim p) \rightarrow p$

Truth Table

$\begin{array}{|c|c|c|}\hline \mathrm{\;\;\;\;\;}p\mathrm{\;\;\;\;\;}&\mathrm{\;\;\;\;\;}\sim p\mathrm{\;\;\;\;\;} &\mathrm{\;\;\;\;\;}\sim\left (\sim p \right )\mathrm{\;\;\;\;\;} \\ \hline \mathrm{T}& \mathrm{F}&\mathrm{T} \\ \hline \mathrm{F}&\mathrm{T}&\mathrm{F} \\ \hline\end{array}$

Truth Table for Conditional Statement:

A Conditional Statement is false only when p is true and q is false. In all other cases this is true.

$\begin{array}{|c|c|c|}\hline \mathrm{\;\;\;\;\;}p\mathrm{\;\;\;\;\;}&\mathrm{\;\;\;\;\;}q\mathrm{\;\;\;\;\;} &\mathrm{\;\;\;}p\rightarrow q\mathrm{\;\;} \\ \hline \mathrm{T}& \mathrm{T} & \mathrm{T}\\ \hline \mathrm{T}&\mathrm{F}& \mathrm{F} \\ \hline \mathrm{F}&\mathrm{T}& \mathrm{T}\\\hline \mathrm{F}&\mathrm{F} & \mathrm{T}\\ \hline\end{array}$

Truth Table for Biconditional Statements:

A biconditional statement is true when both $p$ and $q$ are true or when both $p$ and $q$ are false

$\begin{array}{|c|c|c|}\hline \mathrm{\;\;\;\;\;}p\mathrm{\;\;\;\;\;}&\mathrm{\;\;\;\;\;}q\mathrm{\;\;\;\;\;} &\mathrm{\;\;\;}p\leftrightarrow q\mathrm{\;\;} \\ \hline \mathrm{T}& \mathrm{T} & \mathrm{T}\\ \hline \mathrm{T}&\mathrm{F}& \mathrm{F} \\ \hline \mathrm{F}&\mathrm{T}& \mathrm{F}\\\hline \mathrm{F}&\mathrm{F} & \mathrm{T}\\ \hline\end{array}$

Relation Between Set Notation and Truth Table

Sets can be used to identify basic logical structure of statements.

Let us understand with an example of two sets $p \{1,2\}$ and $q \{2,3\}$

$\begin{array}{|c|c|c|}\hline\quad p\vee q\quad & \quad p\cup q\quad&\quad 1,2,3\quad \\ \hline p\wedge q& p\cap q&2 \\ \hline p^c& \sim p & 3,4 \\ \hline q^c& \sim q&1,4 \\ \hline\end{array}$

Using this relation we get

$\begin{array}{|c|c|c|c|c|c|c|c|c|c|c|}\hline \text{Element } & \mathrm{\;\;\;\;\;}p\mathrm{\;\;\;\;\;}&\mathrm{\;\;\;}q\mathrm{\;\;\;}&\mathrm{\;\;\;\;\;}\sim p\mathrm{\;\;\;\;\;}&\mathrm{\;\;\;}\sim q\mathrm{\;\;\;} &\mathrm{\;\;\;}p\wedge q\mathrm{\;\;}&\mathrm{\;\;}p\vee q\mathrm{\;\;}&\sim\left (p\wedge q \right )\mathrm{\;\;}&\sim p\wedge\sim q\mathrm{\;\;} \\ \hline \hline 1& \mathrm{T}&\mathrm{F} & \mathrm{F} &\mathrm{T}&\mathrm{F}&\mathrm{T}&\mathrm{T}&\mathrm{F} \\ \hline2& \mathrm{T}&\mathrm{T} & \mathrm{F} &\mathrm{F}&\mathrm{T}&\mathrm{T}&\mathrm{F}&\mathrm{F} \\ \hline 3& \mathrm{F}&\mathrm{T} & \mathrm{T} &\mathrm{F}&\mathrm{F}&\mathrm{F}&\mathrm{T}&\mathrm{F} \\ \hline4& \mathrm{F}&\mathrm{F} & \mathrm{T} &\mathrm{T}&\mathrm{F}&\mathrm{F}&\mathrm{T}&\mathrm{T} \\ \hline\end{array}$

Recommended Video

Solved Examples

Example 1: The contrapositive of the statement “I go to school if it does not rain” is :

1) If it rains, I do not go to school.

2) I do not go to school, it rains.

3) If it rains, I go to school.

4) If I go to school, it rains.

Solution

Symbol of If $p$ then $q$ is $p \rightarrow q$ or $p \Rightarrow q$
The contrapositive of $p \rightarrow q$ is $\sim q \rightarrow p$

We need to examine the given statement if says If it does not rain, then I go to school

So contrapositive will be

If I do not go to school, it rains

Example 2: The negation of the statement “If I become a teacher, then I will open a school” is

1) I will become a teacher and I will not open a school

2) Either I will not become a teacher or I will not open a school

3) Neither I will become a teacher nor I will open a school

4) I will not become a teacher or I will open a school

Solution

The given statement is " If I become a teacher, then I will open a school’ "

Negation of the given statement is

"I will become a teacher and I will not open a school"

$
(\because \sim(p \rightarrow q)=p \wedge \sim q)
$

Negation of Conditional Statement -

$
\sim(p \Rightarrow q) \equiv p \wedge \sim q
$

Example 3: Which of the following is true for an if-then statement $p \Rightarrow q$ is true?
1) If $p$ is true, $q$ must be true
2) If $p$ is false, $q$ must be false
3) If $q$ is false, $p$ must be false
4) none of these

Solution

As we have learned

Validating Statements with 'If then' -

By assuming that $p$ is true, prove that $q$ must be true. By assuming that $q$ is false, prove that $p$ must be false.

If $P$ is true, $q$ must be true and If $q$ is false, $p$ must be false

Example 4: Which of the options is sufficient condition for $p \Leftrightarrow q$ to be true ?
1) $p \Rightarrow q$ and $q \neq p$
2) $p \Rightarrow q$ or $q \Rightarrow p$
3) $p \Rightarrow q$ and $q \Rightarrow p$
4) $p \neq q$ and $q \neq p$

Solution

As we have learned

Validating Statements with "If and only if' -

If $p$ is true, then $q$ is true. If $q$ is true then $p$ is true.
Both $p \Rightarrow q$ or $q \Rightarrow p$ must be true
Example 5: What is truth table for $\sim(p \wedge q)$ ?
1) $TTTT$
2) $FFFT$
3) $TTF$
4) $FTTT$

Solution

Construction of truth table -

We prepare table of rows and columns. We write variables denoting sub-statements and we write the truth value of sub statement to get compound statement.

Since truth table for $p \wedge q$ is $TFFF$
For $\sim(p \wedge q)$ is $FTTT$

Summary
Truth values and truth tables are essential tools in logical reasoning, providing a clear and systematic way to analyze the truth or falsity of statements. They are fundamental in the study of logic, computer science, mathematics, and philosophy.