Vector and Scalar - Definition, Vector Addition and Subtraction, Differences, Solved Problems
In our day-to-day life, we come across many questions such as 'What is your height?' and 'How should a football player hit the ball to give a pass to another player on his team?" Observe that a possible answer to the first query may be 1.5 m, a quantity that involves only one value (magnitude) which is a real number. Such quantities are called scalars. However, an answer to the second query is a quantity (called force) which involves muscular strength (magnitude) and direction (in which another player is positioned). Such quantities are called vectors. In real life, we use vectors for tracking objects that are in motion, and localization of places and things.
This Story also Contains
- Physical quantities
- Representation of a Vector
- Position Vector
- Vector Addition and Subtraction
- Difference Between Scalars and Vectors
- Solved Examples Based on Vectors and Scalars
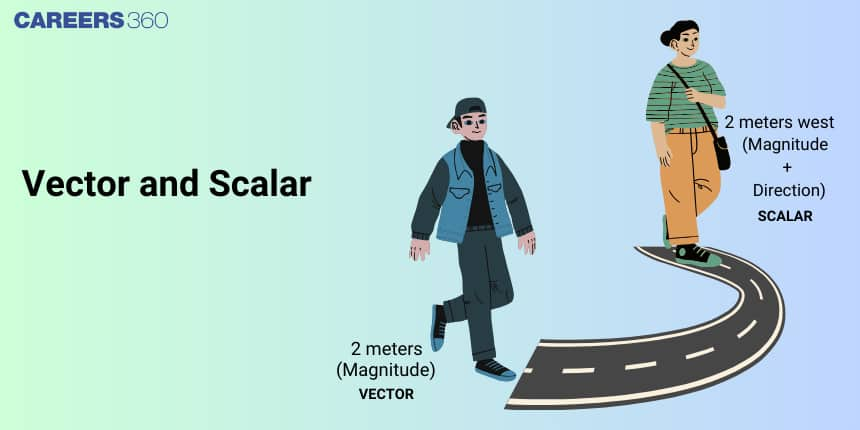
In this article, we will cover the concept of Vectors and Scalars. This topic falls under the broader category of Vector Algebra, which is a crucial chapter in Class 11 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of seven questions have been asked on this topic in JEE Main from 2013 to 2023 including two in 2021.
Physical quantities
A quantity can have magnitude or both magnitude and directions based on that quantity are divided into two categories- Scalar quantities and Vector quantities
Scalar Quantity
A quantity that has magnitude but no direction is called a scalar quantity (or scalar), e.g., mass, volume, density, speed, etc. A scalar quantity is represented by a real number along with a suitable unit.
Vector Quantity
A quantity that has magnitude as well as a direction in space and follows the triangle law of addition is called a vector quantity, e.g., velocity, force, displacement, etc.
In this text, we denote vectors by boldface letters, such as a or $\vec{a}$.
Representation of a Vector
A vector is represented by a directed line segment (an arrow). The endpoints of the segment are called the initial point and the terminal point of the vector. An arrow from the initial point to the terminal point indicates the direction of the vector.

The length of the line segment represents its magnitude. In the above figure, a = AB, and the magnitude (or modulus) of vector a is denoted as $|\vec{a}|=|\overrightarrow{A B}|=A B$ (Distance between the Initial and terminal point).
The arrow indicates the direction of the vector.
Position Vector
In two dimensional system
Let P be any point in the x-y plane, having coordinates (x, y) with respect to the origin O(0, 0, 0).
Then, the vector $\overrightarrow{O P}$ having O and P as its initial and terminal points, respectively, is called the position vector of the point P with respect to O.
It can also be expressed as $\overrightarrow{O P}=\vec{r}=x \hat{\mathbf{i}}+y \hat{\hat{\mathbf{i}}}$. The vectors $x \hat{\mathbf{i}}$ and $y \hat{j}$ are called the perpendicular components of vector r. Where $\hat{\mathbf{i}}$ and $\hat{\mathbf{j}}$ are unit vectors (vectors of length equal to 1) parallel to the positive X -axis and positive Y -axis respectively.

The magnitude of $\tilde{\mathbf{r}}=\sqrt{\mathrm{x}^2+\mathrm{y}^2}$ and if $\theta$ is the inclination of $\tilde{\mathbf{r}}$ w.r.t. $\mathrm{X}-$ axis, then, $\theta=\tan ^{-1}\left(\frac{\mathrm{y}}{\mathrm{x}}\right)$.
In three dimensional system
Let P be any point in space, having coordinates (x, y, z) with respect to the origin O(0, 0, 0).
Then, the vector $\overrightarrow{O P}$ having O and P as its initial and terminal points, respectively, is called the position vector of the point P with respect to O.
OP vector can also be expressed as $\overrightarrow{O P}=\vec{r}=x \hat{\mathbf{i}}+y \hat{\mathbf{j}}+z \hat{\mathbf{k}}$
This form of any vector is called its component form. Here, x, y, and z are called the scalar components.
Sometimes x, y, and z are also called rectangular components.
Using the distance formula, the magnitude of $\overrightarrow{O P}$ or $\vec{r}$ is given by
$\overrightarrow{\mathrm{OP}} \mid=\sqrt{x^2+y^2+z^2}$

Where $\hat{\mathbf{i}}, \hat{\mathbf{j}}$ and $\hat{\mathbf{k}}$ are unit vectors parallel to the positive $X$-axis, $Y$-axis, and $Z$-axis respectively.
Vector Addition and Subtraction
Vector addition is the operation in which two vectors are added to get their sum. Since each vector may have its own direction, the process of addition of vectors is different from adding two scalars.
If a and b are two vectors, then their subtraction, or difference is called vector subtraction $\vec{a}-\vec{p}$ is defined as $\vec{a}+(-\vec{b})$, where $(-\vec{b})$ is the negative of vector b has equal magnitude but opposite direction that of b. Graphically, it is depicted by drawing a vector from the terminal point of b to the terminal point of a.
Difference Between Scalars and Vectors
The difference between Scalars and Vectors is given below:
| Vector | Scalar |
| A physical quantity with both magnitude and direction. | A physical quantity with only magnitude. |
| Represented by a number (magnitude), direction using a unit cap or arrow at the top and unit | Represented by a number (magnitude) and unit |
| Quantity symbol in bold and an arrow sign above | Quantity symbol |
| Example- Velocity and Acceleration | Example- Mass and Temperature |
| Direction is required | Direction not required |
Recommended Video Based on Vectors and Scalars
Solved Examples Based on Vectors and Scalars
Example 1: Let O be the origin. Let $\mathrm{t} \overrightarrow{O P}=x \hat{i}+y \hat{j}-\hat{k}$ and $\overrightarrow{O Q}=-\hat{i}+2 \hat{j}+3 x \hat{k}, x, y \in R, x>0$ be such that $\overrightarrow{P Q}=\sqrt{20}$ and the vecto$\overrightarrow{Q P}$ is perpendicular to $\overrightarrow{O Q} \cdot$ If $\overrightarrow{O R}=3 i+z \hat{j}-7 \hat{k}, z c R$ is coplanar with $\overrightarrow{O P}$ and $\overrightarrow{O Q}$, then the value of $\vec{N}^2+y^2+z^2$ is equal to : [JEE MAINS 2021]
1) $9$
2) $7$
3) $2$
4) $1$
Solution
$\overrightarrow{\mathrm{OP}}=x \hat{i}+y \hat{j}-\hat{k}$ and $\overrightarrow{\mathrm{OQ}}=-\hat{i}+2 \hat{\mathrm{j}}+3 x \hat{k}$
and
$\mathrm{OP} \perp \mathrm{OQ}$
so, $-x+2 y-3 x=0 \Rightarrow y=2 x$
$\begin{aligned} & \overrightarrow{P Q}=\overrightarrow{O P}-\overrightarrow{O Q} \\ & \overrightarrow{P Q}=(\mathrm{x}+1) \hat{\mathrm{i}}+(\mathrm{y}-2) \hat{\mathrm{j}}+(3 \mathrm{x}+1) \hat{\mathrm{k}}\end{aligned}$
$\begin{aligned} & |\overrightarrow{P Q}|=\sqrt{20} \\ & (x+1)^2+(y-2)^2+(3 x+1)^2=20 \\ & (x+1)^2+(2 x-2)^2+(3 x+1)^2=20 \\ & 14 x^2+6=20 \Rightarrow x^2=1 \\ & \Rightarrow x=1, y=2\end{aligned}$
$\overrightarrow{\mathrm{OP}}, \overrightarrow{\mathrm{OQ}}, \overrightarrow{\mathrm{OR}}$ are coplanar
$
\begin{aligned}
& \Rightarrow\left|\begin{array}{ccc}
\mathrm{x} & \mathrm{y} & -1 \\
-1 & 2 & 3 \mathrm{x} \\
3 & \mathrm{z} & -7
\end{array}\right|=0 \\
& \Rightarrow\left|\begin{array}{ccc}
1 & 2 & -1 \\
-1 & 2 & 3 \\
3 & z & -7
\end{array}\right|=0
\end{aligned}
$
$\begin{aligned} & \Rightarrow 1(-14-3 z)-2(7-9)-1(-z-6)=0 \\ & \Rightarrow z=-2 \\ & \therefore x^2+y^2+z^2=1+4+4=9\end{aligned}$
Hence, the answer is option 1) 9
Example 2: A vector $\vec{d}$ has components 3p and 1 with respect to a rectangular cartesian system. This system is rotated through a certain angle about the origin in the counterclockwise sense. If, with respect to the new system, $\vec{a}$ has components $p+1$ and $\sqrt{10}$, then a value of $p$ is equal to: [JEE MAINS 2021]
|1) 1
2) -1
3) $\frac{4}{5}$
4) $-\frac{5}{4}$
Solution: Given
$\begin{aligned} & \vec{A}=3 p \hat{i}+\hat{\mathrm{j}}, \\ & \overrightarrow{A^{\prime}}=(\mathrm{p}+1) \hat{i}+\sqrt{10} \hat{j} \\ & \overrightarrow{\mathrm{A}}|=| \vec{A}^{\prime} \mid,(\text { No Change in magnitude })\end{aligned}$

$\begin{aligned} & \Rightarrow \sqrt{9 p^2+1}=\sqrt{(p+1)^2+10} \\ & 9 \mathrm{p}^2+1=\mathrm{p}^2+2 \mathrm{p}+1+10 \\ & 8 \mathrm{p}^2-2 \mathrm{p}-10=0 \\ & 4 \mathrm{p}^2-\mathrm{p}-5=0 \\ & (4 \mathrm{p}-5)(\mathrm{p}+1)=0 \\ & \mathrm{p}=-1, \mathrm{p}=\frac{5}{4}\end{aligned}$
Hence, the answer is option 2) -1
Example 3: Which of the following can't be represented using a directed line segment?
1) Force
2) Velocity
3) Acceleration
4) Mass
Solution: We know that Directed line segment - A is called the initial point and B is called the terminal point.

Mass has no direction, so it can't be represented using a directed line segment
Hence, the answer is option 4) Mass
Example 4: Which of the following are not scalars?
1) Velocity
2) Mass
3) Temperature
4) None of these
Solution: Velocity has magnitude as well as direction, but mass & temperature don't have direction
Hence, the answer is the option (1).
Example 5: Which of the following are not vectors?
1) Velocity
2) Force
3) Acceleration
4) Mass
Solution: Velocity, Force & acceleration all have magnitudes and directions, only mass doesn't have a direction so it is not a vector.
Hence, the answer is the option (4).
Frequently Asked Questions (FAQs)
A quantity that has magnitude but no direction is called a scalar quantity (or scalar), e.g., mass, volume, density, speed, etc. A scalar quantity is represented by a real number along with a suitable unit.
A quantity that has magnitude as well as a direction in space and follows the triangle law of addition is called a vector quantity, e.g., velocity, force, displacement, etc.
A vector is represented by a directed line segment (an arrow). The endpoints of the segment are called the initial point and the terminal point of the vector. An arrow from the initial point to the terminal point indicates the direction of the vector.
Vector can be expressed as $\overrightarrow{O P}=\vec{r}-x \hat{\mathbf{i}}+y \hat{\mathbf{j}}$. The vectors $x \hat{\mathbf{i}}$ and $y \hat{i}$ are called the perpendicular components of vector r. where $\hat{\mathbf{i}}$ and $\hat{\mathbf{j}}$ are unit vectors (vectors of length equal to 1) parallel to the positive X-axis and positive Y-axis respectively.
Vector can be expressed as $\overrightarrow{O P}=\vec{r}=x \hat{\mathbf{i}}+y \hat{\mathbf{j}}+z \hat{\mathbf{k}}$ Here, x, y, and z are called the scalar components. $\hat{\mathbf{i}}, \hat{\mathbf{j}}$ and $\hat{\mathbf{k}}$are unit vectors parallel to the positive X-axis, Y-axis, and Z-axis respectively.