Derivation of Lens Formula - Definition, FAQs
Lenses are pieces of curved glass or some transparent material, and they bend light to create images. Lenses are commonly found in cameras, eyeglasses, microscopes and telescopes. The formation of an image by lenses is understood with the help of the lens formula, a mathematical expression that relates the object distance (u), image distance (v), and focal length (f) of a lens. In this article, we shall derive the lens formula step by step using simple ray diagrams and basic geometry, all for the benefit of a simple-to-understand concept for students and for application to numerical problems.
This Story also Contains
- What Is A Lens?
- What is the Lens Formula?
- State Lens Makers' Formula
- What Is a Thin Lens?
- Derive Lens Maker’s Equation
- Lens Formula Derivation
- Image Formation With A Thin Lens: Characteristics
What Is A Lens?
The lens is a transparent object that may be made of glass or plastic, and it bends (or refracts) light rays to create images. These are lenses with curved surfaces that primarily use the principle of refraction. Lenses are found in eyeglasses, cameras, microscopes, and magnifying glasses.
Lenses are based on two basic types:
- Convex lens (converging lens): Thicker in the middle, bending light rays to bring them together, and can create real or virtual images.
- Concave lens (diverging lens): Thinner in the middle, diverging light rays, and always forming a virtual image.
The lens is divided into two types depending on how the light rays behave when they travel through the lens. These are further divided into the following types.
.png)
What is the Lens Formula?
The lens equation or lens formula is an equation that links the focal length, image distance, and object distance.
$\frac{1}{v}-\frac{1}{u}=\frac{1}{f}$
where,
v is the distance of the image from the optical centre of the lens, u is the distance of the object from the optical centre of the lens and f is the focal length of the lens.
State Lens Makers' Formula
The relationship between a lens's focal length, the refractive index of its material, and the radii of curvature of its two surfaces is known as the lens maker's formula. Lens manufacturers use it to build lenses with a specific power from glass with a specific refractive index. The focal length, f, is described by the lens maker's formula:
$\frac{1}{f}=(n-1)\left(\frac{1}{R_1}-\frac{1}{R_2}\right)$
where
R1 and R2 are the radii of curvature, and n is the index of refraction
Focal length: The focal length of an optical system is the inverse of the system's optical power; it measures how strongly the system converges or diverges light. A system with a positive focal length converges light, while one with a negative focal length diverges light.
Power: It is the reciprocal of the focal length and is measured in diopters (D). Power is positive for converging lenses and negative for diverging lenses.
Commonly Asked Questions
The back focal length (BFL) is the distance from the rear vertex of a lens to its focal point. While the simple lens formula assumes a thin lens, it can be extended to thick lenses where the BFL becomes relevant. In such cases, the formula helps in understanding how the effective focal length differs from the BFL due to the lens's thickness and shape.
The lens formula itself doesn't account for chromatic aberration, but it forms the basis for understanding it. Different wavelengths of light have slightly different refractive indices, leading to different effective focal lengths (f) for each color. By applying the lens formula separately for different wavelengths, we can understand how images of different colors form at slightly different positions, causing chromatic aberration.
While the lens formula doesn't directly address light-gathering ability, it's related to the concept. Lenses with shorter focal lengths (larger 1/f) can have larger apertures relative to their focal length (smaller f-number). This allows more light to be gathered, which is crucial in low-light photography and astronomy. The formula helps in understanding the trade-offs between focal length, aperture, and light-gathering power.
The lens formula relates directly to diopters, the unit of measurement for lens power. Since diopters are defined as the reciprocal of the focal length in meters (D = 1/f), the lens formula (1/f = 1/u + 1/v) can be rewritten in terms of diopters. This helps in calculating the required lens power for vision correction based on a person's near and far points.
While the simple lens formula assumes a thin lens with coincident principal planes, it forms the basis for understanding more complex systems. In thick lenses or multi-lens systems, principal planes are the theoretical planes where refraction is considered to occur. The lens formula can be adapted to use distances measured from these planes, helping to analyze more complex optical setups.
What Is a Thin Lens?
A thin lens is defined as one whose thickness is insignificant in comparison to its curvature radii. The thickness (t) is significantly lower than the two curvature radii R1 and R2.
The focal length, image distance, and object distance are all connected in the lens formula for concave and convex lenses. The given formula can be used to establish this link.
$$\frac{1}{u}+\frac{1}{v}=\frac{1}{f}$$
where,
f is the focal length of the lens
v is the distance of the generated image from the lens' optical center
u is the distance between an item and the optical center of the lens.
Also read -
- NCERT Solutions for Class 11 Physics
- NCERT Solutions for Class 12 Physics
- NCERT Solutions for All Subjects
Different Types Of Thin Lens
There are two types of thin lenses- convex lens and concave lens. Read the below table to learn more about these.
| Converging (convex) Lens | Diverging (concave) Lens |
| • The focal length is positive (+ve) | • The focal length is negative (-ve) |
| • A convex lens is thicker at the center and thinner at the edges | • A concave lens is thicker at the edges and thinner at the center |
| • Use for correction of long-sightedness | • Use for correction of short-sightedness |
| • On passing the light through the lens, it bends the light rays towards each other | • On passing the light through the lens, it bends the light rays away from each other |
| • The image formed can be real, virtual, enlarged, or diminished | • The image formed is always virtual and diminished |
| • The principal focus is real | The principal focus is virtual |
| • It is also called a positive lens | • It is also called a negative lens |
| • Human eye, camera | • Lights, flashlights |
Derive Lens Maker’s Equation
.png)
The lens maker's formula is derived using the assumptions listed below.
- Consider the thin lens in the picture above, which has two refracting surfaces with curvature radii R1 and R2, respectively.
- Assume that the surrounding medium and the lens material have refractive indices of na and nb, respectively.
- Consider an object O placed on the principal axis of the thin lens.
- Assume a ray from object O incident on the convex surface of the lens at point A which has a radius of curvature R1
- It forms an image at Q.
- For convex lens $\mathrm{n}_1=\mathrm{n}_{\mathrm{a}}, \mathrm{n}_2=\mathrm{n}_{\mathrm{b}}$
The whole derivation of the lens maker formula is provided further below. We can say that, using the formula for refraction at a single spherical surface,
For the first surface,
Object Distance = -u
Image Distance = v = x
Radius of Curvature= R1
Thus the formula becomes,
$\frac{n_2}{v}-\frac{n_1}{u}=\frac{n_2-n_1}{R_1} \ldots$
By substituting we get
$\frac{n_b}{x}-\frac{n_a}{u}=\frac{n_b-n_a}{R_1} \ldots$ --------------(1)
- The ray gets refracted due to the concave surface at point B after getting refracted at point A and reaches the principal axis at point I.
- The image Q of object O due to the convex surface is taken as the object for the concave surface.
- Object distance PQ = u = x, Image distance = PI = v, radius of curvature = -R2For concave surface , n1 = nb, n2 = na
For the second surface,
$\frac{n_2}{v}-\frac{n_1}{x}=\frac{n_2-n_1}{-R_2}$ --------------- ( 2)
Now adding equation (1) and (2),
$\begin{aligned} & \frac{n_a}{v}-\frac{n_b}{u}=\left(n_b-n_a\right)\left[\frac{1}{R_1}-\frac{1}{R_2}\right] \\ \\& \Rightarrow \frac{1}{v}-\frac{1}{u}=\left(\frac{n_b}{n_a}-1\right)\left[\frac{1}{R_1}-\frac{1}{R_2}\right]\end{aligned}$
We know that,
$\frac{1}{v}-\frac{1}{u} =\frac{1}{f} $
Hence the equation becomes,
$\frac{1}{f}=\left(\frac{n_2}{n_1}-1\right)\left[\frac{1}{R_1}-\frac{1}{R_2}\right]$
Therefore, we can say that,
$\frac{1}{f}=(\mu-1)\left(\frac{1}{R_1}-\frac{1}{R_2}\right)$
Where μ is the material's refractive index.
This is the derivation of the lens maker formula. Examine the constraints of the lens maker's formula to have a better understanding of the lens maker's formula derivation.
Also read-
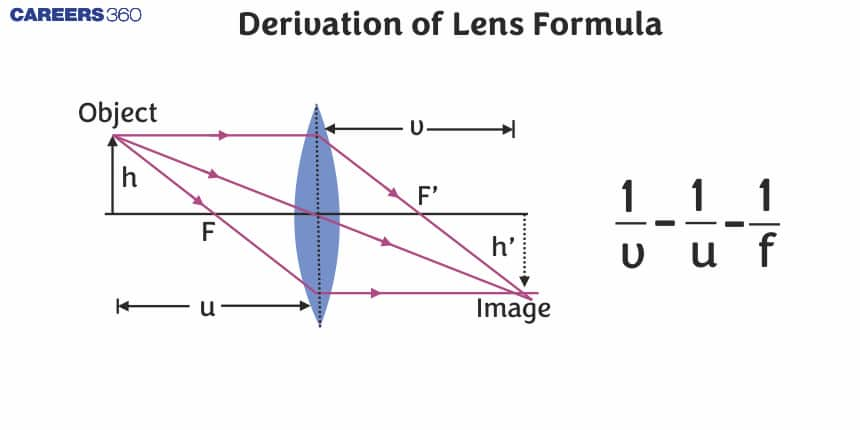
Lens Formula Derivation
Let AB represent an object at a distance greater than the focal length f of the convex lens that is located at right angles to the primary axis. The image A1B1 is generated between O and F1 on the same side as the item, and it is virtual and erect.
.png)
- Focul length = OF1 = f
- Object distance = OA =u
- Image distance = OA1 = v
$\triangle O A B$ and $\triangle O A^1 B^1$ are similar
$\left[\begin{array}{l}\because \angle B A O=\angle B^1 A^1 O=90^{\circ}, \text { vertex is common for both the triangles } \\ \text { so } \angle A O B=\angle A^1 O B^1, \therefore \angle A B O=\angle A^1 B^1 O\end{array}\right]$
$\frac{A^1 B^1}{A B}=\frac{O A^1}{O A}---(1)$
$\triangle O C F_1$ and $\triangle F_1 A^1 B^1$ are similar
$\frac{A^1 B^1}{O C}=\frac{A^1 F_1}{O F_1}$
But from the ray diagram, we see that OC = AB
$\begin{aligned} & \frac{A^1 B^1}{A B}=\frac{A^1 F_1}{O F_1}=\frac{O F_1-O A^1}{O F_1} \\ & \frac{A^1 B^1}{A B}=\frac{O F_1-O A^1}{O F_1}---(2)\end{aligned}$
From equation (1) and equation (2), we get
$\begin{aligned} & \frac{O A^1}{O A}=\frac{O F_1-O A^1}{O F_1} \\ & \frac{-v}{-u}=\frac{-f--v}{-f} \\ & \frac{v}{u}=\frac{-f+v}{-f} \\ & -v f=-u f+u v\end{aligned}$
Dividing throughout by uvf
$-\frac{1}{u}=-\frac{1}{v}+\frac{1}{f}$
$\frac{1}{f}=\frac{1}{v}-\frac{1}{u}$
Read more-
Image Formation With A Thin Lens: Characteristics
It's not enough to know the thin lens formula for convex lenses. The characteristics of a ray of light going through converging and diverging lenses must be understood.
- On the opposite side, parallel rays going through converging lenses will intersect at point f.
- Parallel rays seem to emerge from point f in front of diverging lenses.
- The direction of light rays traveling through the center of converging or diverging lenses does not change.
- Light rays that enter a converging lens through its focal point always exit parallel to the lens's axis.
- On the other side of a diverging lens, a light ray going towards the focal point will also emerge parallel to its axis.
A concave or divergent lens has a negative focal length. When the picture is generated on the side where the object is positioned, the image distance is also negative. The image is virtual in this case. A converging or convex lens, on the other hand, has a positive focal length.
Frequently Asked Questions (FAQs)
The lens formula is directly related to lens power addition. Since lens power is defined as P = 1/f, the formula can be rewritten in terms of power: P = 1/u + 1/v. This additive nature of lens powers is crucial in optometry, especially when combining lenses (like in bifocals) or calculating the total power of a lens system.
Yes, the lens formula explains this versatility of convex lenses. For objects beyond the focal point (u > f), the formula yields a positive v, indicating a real image. For objects within the focal length (u < f), v becomes negative, indicating a virtual image. This mathematical behavior aligns with the physical reality of how convex lenses interact with light rays at different object distances.
The lens formula is fundamental in calculating hyperfocal distance - the closest distance at which a lens can be focused while keeping objects at infinity acceptably sharp. By manipulating the formula and incorporating the concept of circle of confusion, photographers can determine the optimal focus setting to maximize depth of field, a crucial technique in landscape and architectural photography.
The lens formula shows that for real images to form (positive v), the term 1/u must be smaller than 1/f for convex lenses (positive f). This mathematically demonstrates that real images can only form when the object is beyond the focal point, revealing the crucial relationship between focal length and real image formation.
The lens formula helps explain depth of focus, which is related to depth of field but on the image side of the lens. Lenses with longer focal lengths (smaller 1/f) have a shallower depth of focus. This is because small changes in object distance (u) result in larger changes in image distance (v) for lenses with longer focal lengths, as evident from the formula's relationships.
Telecentric lenses are designed so that the chief rays are parallel to the optical axis in object or image space. While the basic lens formula doesn't directly describe telecentricity, it forms the foundation for understanding how these lenses work. By manipulating the object or image distance to approach infinity, we can create conditions where magnification becomes independent of object position, a key feature of telecentric systems.
In microscopy, the working distance is the space between the objective lens and the specimen. The lens formula helps understand how changing this distance (effectively changing u) affects the image formation. It shows that as the working distance decreases (smaller u), the image distance (v) increases, which relates to the magnification and the design of microscope objectives.
Yes, the lens formula helps explain this. For concave lenses (negative f), the formula always yields a negative v for positive u, indicating a virtual image. The magnification m = -v/u is always positive in this case, meaning the image is upright. This mathematical result aligns with the physical behavior of concave lenses diverging light rays.
While the lens formula doesn't directly calculate field of view, it helps explain the relationship. A shorter focal length (larger 1/f) allows objects at a given distance to form images closer to the lens. This geometrically translates to a wider field of view. Conversely, longer focal lengths result in narrower fields of view, a principle crucial in understanding lens selection in photography and telescope design.
The lens formula, combined with the magnification equation (m = -v/u), reveals that the maximum theoretical magnification occurs as the object approaches the focal point. As u approaches f, v approaches infinity, and the magnification (v/u) becomes very large. However, practical limitations prevent achieving infinite magnification, illustrating the theoretical limits of single-lens systems.
