Mirror Equation - Definition, Formula, Applications, FAQs

Spherical mirrors are used to produce a wide range of mirrors. If we know the object position and the focal length of the mirror, we may use the mirror equation formula of mirror and equations to determine where the image will develop. In the event of a real image, if we place a screen at that point, we will obtain the image of the object on the screen; otherwise, to capture the image, we must move the screen to various possible positions and check. In this article, we will discuss concave and convex mirrors, what is mirror equation, the convex mirror formula, the concave mirror formula, image formation by spherical mirrors, and applications of the mirror equation.

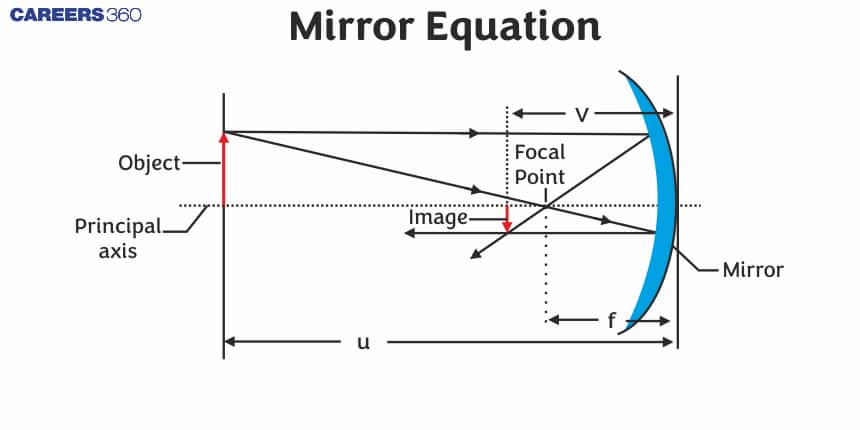
Spherical Mirrors: Concave and Convex Mirror

A curved mirror, on the other hand, can produce pictures that are larger or smaller than the item and can appear in front of or behind the mirror. In general, any curved surface will produce an image, though certain pictures may be so warped that they are unintelligible (think of funhouse mirrors). Curved mirrors are employed in a wide range of optical systems because they can provide such a diverse range of pictures.

There are two general types of spherical mirrors. When the reflecting surface is the sphere's outer side, the mirror equation is referred to as a convex mirror. If the inside surface is the reflecting surface, then the mirror equation is called a concave mirror.

What is Mirror Equation?

The spherical mirror equation is the relationship that connects the object distance and image distance. The mirror formula is more specifically used to calculate the focal length in terms of image and object distances. The focal length of a mirror equation is the distance between its pole and its major focus for small apertures. The symbol f represents the focal length. The mirror's focal length f is related to its radius of curvature (R) by the equation:

$f=\frac{R}{2}$

As a result, the mirror formula is given by

$$
\frac{1}{v}+\frac{1}{u}=\frac{1}{f}=\frac{2}{R}
$$

where,

• $v$ is the image distance
• $u$ is the object distance
• $R$ is the radius of curvature of the mirror
• $f$ is the focal length of the mirror

Sign Convention for Mirror

The mirror equation adheres to the following sign conventions:

1. The mirror's principal axis is drawn along the x-axis of the rectangular coordinate system, and its pole is drawn as the origin.

2 . The object is taken from the left side of the mirror, implying that light is incident on the mirror from the left.

3. All distances parallel to the mirror's major axis are measured from the mirror's pole.

4. Distances measured in the direction of incident light are considered positive.

5. Negative distances are those measured in the opposite direction of the incident light.

6. All heights measured upwards and perpendicular to the mirror's primary axis are considered positive.

7. The heights measured downwards and perpendicular to the mirror's major axis are considered negative.

As a result, the focal length of a concave mirror equation is negative, while the focal length of a convex mirror equation is positive. In addition, the object distance and object height are treated as negative and positive values, respectively.

Concave Mirror Equation

For concave mirrors, the mirror equation is given as,

$$\frac{1}{f}=\frac{1}{u}+\frac{1}{v}$$

where,

• $v$ is the image distance
• $u$ is the object distance
• $f$ is the focal length of the mirror

The effect of an object's position relative to the focal point of a mirror equation on the picture (concave)

1. When anything is placed between the focus point and the mirror.

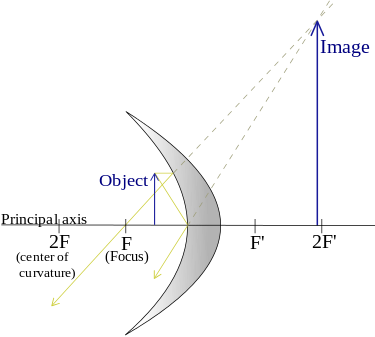
The nature of the image formula produced will be Virtual, Upright, and magnified.

2. When an object is present at the focal point of the mirror.

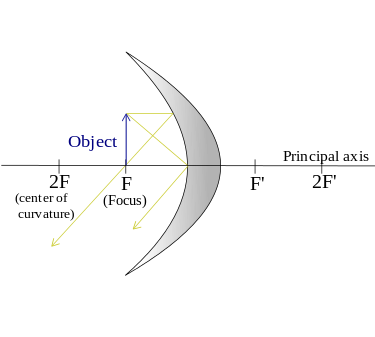
Because the rays due to the reflection of light are parallel and never intersect, no image formula is generated.

The picture distance approaches infinity as S approaches F, and the image formula can be actual or virtual, upright or inverted, depending on whether S approaches F from the left or right side.

3. When an object is put between the focus and the center of the curvature.

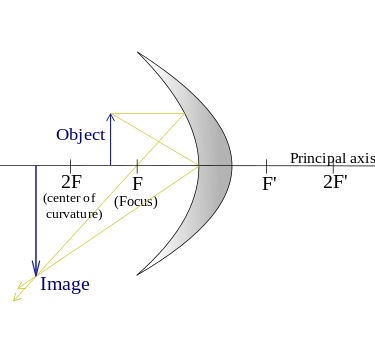
The resulting image will be a true/real image, Reversed (vertically) and enlarged (larger).

4. When an object is in the center of the curve.

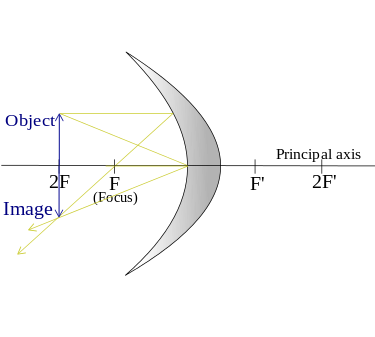
The resulting image will be a real image inverted (vertically), the same size.

At the center of the curvature, an image is generated.

5. When an object extends beyond the center of curvature.

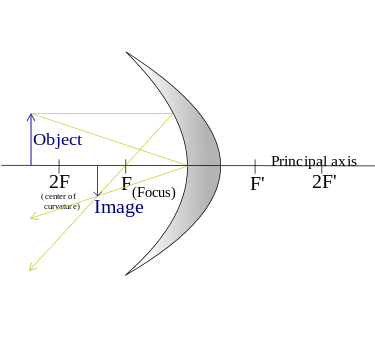
The resulting image will be a Real image, Reversed (vertically), Reduced (smaller).

As the object's distance grows, the picture approaches the focal point asymptotically.

As the image approaches F, the image size approaches 0 in the limit where S approaches infinity.

Convex Mirror Equation

The mirror formula for spherical mirrors like convex mirrors is the same as the general mirror equation,

$$
\frac{1}{f}=\frac{1}{u}+\frac{1}{v}
$$

where,

• $v$ is the image distance
• $u$ is the object distance
• $f$ is the focal length of the mirror

The effect of an object's position relative to the focal point of a mirror equation on the picture (convex)

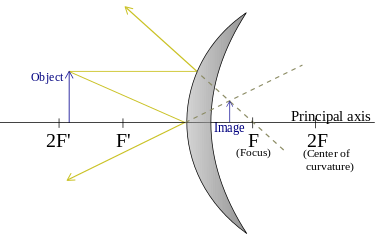
A convex mirror's image is always virtual (rays do not travel through the picture; their extensions do, as in a regular mirror), reduced as well as upright as the object gets closer to the mirror, the image grows larger until it is roughly the size of the object when it reaches the mirror. Convex mirrors are highly useful because, because everything seems smaller in the mirror, they cover a greater field of vision than a conventional plane mirror, making them ideal for seeing cars behind a driver's car on the road, viewing a larger area for surveillance, and so on.

Mirror Equation Uses

The Mirror equation is used in the following ways:

1. When the object distance and the focal length of the mirror equation are known, the mirror equation can be used to determine the image distance.
2. When we know the image distance and the focal length of the mirror, we can use the mirror equation to calculate the object distance.
3. The mirror equation allows us to calculate the focal length of the mirror equation simply by knowing the distance between the item and the image it generates.
4. When we use the mirror equation in conjunction with the magnification equation, we can obtain the value of either the image height or the object height when the other is specified.

Also read:

• NCERT Exemplar Solutions for All Subjects
• NCERT Notes For All Subjects
• NCERT Solutions for All Subjects