Harmonic Progression (H.P.) - Definition, Properties, Formulas, Examples

Harmonic progression (HP) is an essential concept in mathematics, particularly in number theory and mathematical analysis. Harmonic progression is obtained by taking the reciprocal of the terms of an arithmetic progression.A good example of harmonic progression is the Leaning Tower of Lire. Here a set of uniform sides blocks are stacked one over the other to achieve maximum sideways or lateral distance.The blocks have been stacked one-way distances of $\frac{1}{2}, \frac{1}{4}, \frac{1}{6}, \frac{1}{8},$...... one over the other. This stacked arrangement aims to achieve maximum sideway distances, such that the centre of gravity is maintained and it does not collapse.In this article, we will discuss the ‘harmonic progression’, ‘harmonic progression formula’, ‘sum of harmonic progression’, ‘what is harmonic progression’, ‘harmonic progression sum formula’, ‘harmonic progression example’ etc.

CAT 2024: 20 Free Mock Test | 10 Year PYQs | 60 Day Study Material | Most Scoring Concepts

XAT 2025: Section-wise Preparation Tips | Sample Paper

Don't Miss: SNAP 2024 Sample Papers | NMAT 2024 Sample Papers | MAT 2024 Sample Papers

This Story also Contains

1. What is Harmonic Progression?
2. Terms and Notations Used in Harmonic Progression
3. The general term of an HP
4. The sum of n terms of an HP
5. List of Harmonic Progression formulas
6. Harmonic mean
7. Properties of an HP
8. Tips and Tricks
9. Solved Examples

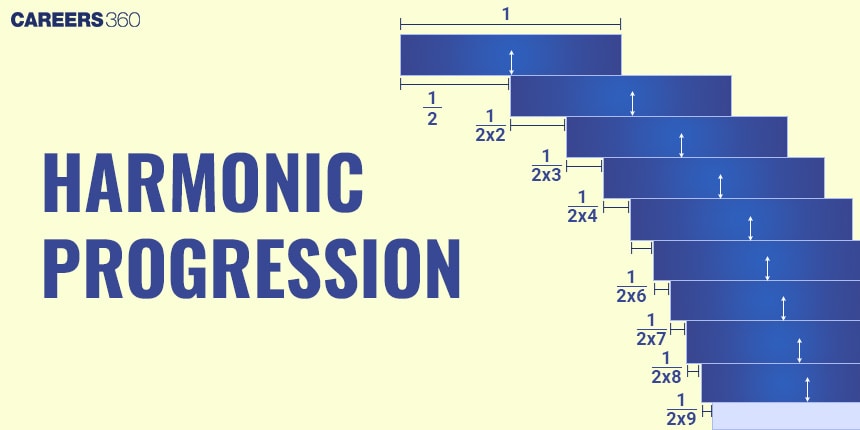
What is Harmonic Progression?

A Harmonic Progression (HP) is defined as a sequence of real numbers that are determined by taking the reciprocals of the arithmetic progression that does not contain 0.

If $a, a+d, a+2d, …..$ is an AP, then the terms of the HP will be $\frac{1}{a}, \frac{1}{a+d}, \frac{1}{a+2d}, …..$, where both $a$ and $d$ are non-zero numbers.

Comparison with Arithmetic Progression and Geometric Progression

Terms and Notations Used in Harmonic Progression

Harmonic progression (HP) is a sequence of numbers in which the reciprocals form an arithmetic progression (AP). Understanding the terms and notations used in harmonic progression is crucial for solving related problems. Here are some key terms and notations:

First term (?):

As the name suggests, the first term of an HP is the first number of the progression. It is usually represented by $a_1$ (or) $a$. For example, in the HP $\frac{1}{5}, \frac{1}{10}, \frac{1}{15}, \frac{1}{20}, …..$ the first term $a$ is $\frac{1}{5}$.

Common difference:

There is no common difference in HP, as the difference between any two consecutive terms is not constant, but we can still calculate the difference for each pair of consecutive terms in the HP. For example, in the HP $\frac{1}{5}, \frac{1}{10}, \frac{1}{15}, \frac{1}{20}, …..$ the difference between $\frac{1}{5}$ and $\frac{1}{10}$ is $\frac{1}{5} - \frac{1}{10} = \frac{1}{10}$.

The number of terms (n):

As the name suggests, the number of terms of an HP is the total number of terms present in the progression or in the sequence. It is usually denoted as $n$.

For example, $\frac{1}{2}, \frac{1}{3}, \frac{1}{4}, \frac{1}{5}$ is an example of an HP, where the number of terms ($n$) is 4.

The general term of an HP

To solve the problems regarding an HP, we need to find its general term. The general term of an HP can also be expressed as the nth term of the HP. Let’s discuss this in more detail:

The nth term of HP:

We know that the nth term of an AP is $t_n = a + (n-1)d$, where the first term is $a$, the common difference is $d$, and the number of terms is $n$.

Again, the nth term of an HP is equal to the reciprocal of the nth term of the corresponding AP.

So, the nth term of an HP is $t_n = \frac{1}{a + (n-1)d}$, where $a$ is the reciprocal of the first term, $d$ is the common difference of the reciprocal of the terms, and $n$ is the number of terms.

The sum of n terms of an HP

The sum of n terms an HP is $S_n = \frac{1}{d}\log{\frac{2a + (2n-1)d}{2a - d}}$, where $a$ is the reciprocal of the first term and $d$ is the common difference of the reciprocal of the terms.

List of Harmonic Progression formulas

Harmonic mean

The harmonic mean (HM) is a type of average, typically used when the average of rates or ratios is desired. It is especially useful in cases where the numbers are defined in relation to some unit (e.g., speed, density). It is calculated as the reciprocal of the arithmetic mean of the reciprocals of the given values.

$\frac{n}{\frac{1}{a_1}+\frac{1}{a_2}+\frac{1}{a_3}+......+\frac{1}{a_n}}$

For more information click here.

Properties of an HP

• Reciprocals of an HP will form an AP.

• No term of an HP can be zero.

• In a harmonic progression, the terms decrease if the common difference of the reciprocals is positive, and they increase if the common difference is negative.

• If $H$ is the Harmonic Mean of two numbers $a$ and $b$, then we can say that

$(H-2a)(H-2b)=H^2$

Tips and Tricks

• The sum of an HP generally involves calculating the sum of the reciprocals first, then converting them back to the original sequence.

• HM of two terms $a$ and $b$ is $\frac{2ab}{a+b}$

• HM of three terms $a, b$, and $c$ is $\frac{3abc}{ab+bc+ca}$

• For solving three unknown terms in an HP, the terms should be assumed as $\frac{1}{a-d}, \frac{1}{a}$ and $\frac{1}{a+d}$.

• For solving four unknown terms in an HP, the terms should be assumed as $\frac{1}{a-3d}, \frac{1}{a-d}, \frac{1}{a+d}$ and $\frac{1}{a+3d}$.

Solved Examples

Q.1. Find the 5th term of the HP 6, 3, 2, …..

1. $\frac{5}{6}$

2. $\frac{6}{5}$

3. $\frac{7}{5}$

4. $1$

Hint: Convert the given HP into an AP, then find the 5th term of that AP.

Solution:

Given: HP = 6, 3, 2, …..

The corresponding AP is $\frac{1}{6}, \frac{1}{3}, \frac{1}{2}, …...$

The first term ($a$) = $\frac{1}{6}$, common difference ($d$) = $\frac{1}{3} - \frac{1}{6} = \frac{1}{6}$.

Now, the 5th term of AP is $t_5 = a + (5 -1)d$ = $\frac{1}{6} + \frac{4}{6}$ = $\frac{5}{6}$

So, the corresponding 5th term of the HP is $\frac{6}{5}$.

Hence, the correct answer is option (2).

Q.2. Which of the following progression is helpful in deriving harmonic progression?

1. Algebraic progression

2. Geometric progression

3. Logarithm progression

4. Arithmetic progression

Hint: Harmonic progression is obtained by taking the reciprocal of the terms of an arithmetic progression.

Solution:

We know that a Harmonic Progression (HP) is defined as a sequence of real numbers that are determined by taking the reciprocals of the arithmetic progression.

So, the arithmetic progression is helpful in deriving harmonic progressions.

Hence, the correct answer is option (4).

Q.3. Find the 12th term of the harmonic progression, if the 5th term is $\frac{1}{16}$, and the 8th term is $\frac{1}{25}$.

1. $\frac{1}{32}$

2. $\frac{1}{34}$

3. $\frac{1}{37}$

4. $\frac{1}{35}$

Hint: The nth term of an HP is $t_n = \frac{1}{a + (n-1)d}$, where $a$ is the reciprocal of the first term, $d$ is the common difference of the reciprocal of the terms.

Solution:

Given: $t_5 = \frac{1}{16}$ and $t_8 = \frac{1}{25}$

Let the first term and the common difference of the corresponding AP be $a$ and $d$

⇒ $\frac{1}{a+(5-1)d}=\frac{1}{16}$ and $\frac{1}{a+(8-1)d}=\frac{1}{25}$

⇒ $\frac{1}{a+4d}=\frac{1}{16}$ and $\frac{1}{a+7d}=\frac{1}{25}$

⇒ $a+4d=16$ and $a+7d=25$

Subtracting the first equation from the second we get,

⇒ $3d = 9$ ⇒ $d = 3$

From this, we can get, $a = 16 -12$ ⇒ $a = 4$

So, the 12th term of the HP is $t_{12} = \frac{1}{4 + (12-1)3} = \frac{1}{37}$

Hence, the correct answer is option (3).

Q.4. Find the harmonic mean of 60 and 40.

1. 48

2. 24

3. 36

4. 50

Hint: Harmonic mean of two terms $a$ and $b$ is $\frac{2ab}{a+b}$

Solution:

The harmonic mean of 60 and 40 = $\frac{2×60×40}{60+40} = \frac{4800}{100} = 48$

Hence, the correct answer is option (1).

Q.5. Three numbers 5, p and 10 are in harmonic progression if p =?

1. 7

2. 8

3. $\frac{20}{3}$

4. $\frac{10}{3}$

Hint: In a harmonic progression, any term of the series is the harmonic mean of its neighbouring terms.

Solution:

If 5, p, and 10 are in HP then p is the harmonic mean of 5 and 10.

So, p = $\frac{2×5×10}{5+10} = \frac{100}{15} = \frac{20}{3}$

Hence, the correct answer is option (3).