Pipe and Cistern and concept of negative work: Definition, Formula, Questions

Pipes and cisterns and concept of negative work is an important part of Mathematics.
Pipe and cistern questions and answers are a significant part of competitive or normal exams. These problems require understanding the rates at which pipes fill or empty a tank. The basic pipe and cistern formula involves calculating the amount of work done by each pipe per unit time. For instance, if a pipe fills a tank in 5 hours, then the work rate of that pipe is $\frac{1}{5}$ part of the tank per hour.

Now, we can think what is the concept of negative work or what is the definition of negative work.
The concept of negative work is essential when dealing with pipes that empty the tank. Negative work occurs when the draining pipe reduces the water level, impacting the net rate of filling. For example, if a pipe empties a tank in 4 hours, its rate of work is $-\frac{1}{4}$ per hour. Understanding the negative concept example helps us to solve complex pipe and cistern questions.

To master these problems, we can use resources like pipe and cistern questions with solutions PDF, pipe and cistern mock test, and pipe and cistern questions for SSC. These resources provide various examples, including pipe and cistern CAT questions and practice materials like pipe and cistern questions PDF and pipe and cistern questions in English. Additionally, we can explain the concept of negative work with a p-v diagram, illustrating the impact of negative force or draining action.

Concept of Inlet and Outlet

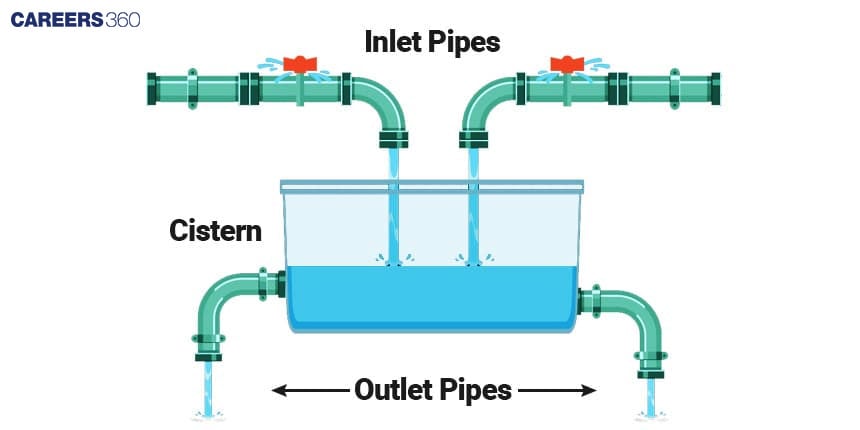
An inlet pipe is a type of type which fills the water tank/reservoir with water. Through this pipe, water comes and the water level rises. Its work rate is positive.

If an inlet pipe fills a tank in $x$ hours, its work rate is $\frac{1}{x}$ part per hour.

An outlet pipe is a type of pipe which empties the water tank/reservoir. Through this pipe, water comes out from the tank/reservoir and the water level decreases. Its work rate is negative.

If an outlet pipe empties a tank in $y$ hours, its work rate is $-\frac{1}{y}$ part per hour.

If both are opened simultaneously, then the net work rate is the difference between their work rate per hour.

So, if an inlet pipe fills a tank in $x$ hours and an outlet pipe empties a tank in $y$ hours, their net work rate per hour is $\frac{1}{x}-\frac{1}{y}=\frac{y-x}{xy}$

So, the tank is filled in $\frac{xy}{y-x}$ hours. (y > x)

When $y > x$, that means the tank is being filled.

When $x > y$, that means the tank is being emptied.

Example:

An inlet pipe can fill a tank in 30 hours. Because there is an outlet pipe, it is filled in 50 hours. How much time will the outlet pipe take to empty the filled tank?

Sol: The inlet pipe fills the tank in 30 hours.

So, it fills $\frac{1}{30}$th of the tank in 1 hour.

With the outlet pipe open, the inlet pipe fills $\frac{1}{50}$th the tank in 1 hour.

The outlet pipe empties in 1 hour $=\frac{1}{30} - \frac{1}{50} = \frac{5 - 3}{150} = \frac{2}{150} = \frac{1}{75}$

So, the outlet pipe will empty the full tank in 75 hours.

Important formulae

Types of Problems

Pipes and cisterns problems are very common questions in examinations, be it normal exams or competitive exams. There are various types of problems relating to pipes and cisterns. Important ones are discussed below.

When there are two or more inlet pipes

The work rate of the inlet pipe is always positive. So if there are two or more inlet pipes, then the tank/reservoir will fill quickly. Add their work rate per hour to get the net work rate per hour.

Pipe A fills a tank in $x$ hours, pipe B fills the tank in $y$ hours, and pipe C fills the tank in $z$ hours.

If they are opened together, then how long it will take to fill the tank?

Work rate of pipe A = $\frac{1}{x}$

Work rate of pipe B = $\frac{1}{y}$

Work rate of pipe C = $\frac{1}{z}$

Their combined work rate = $\frac{1}{x}+\frac{1}{y}+\frac{1}{z}=\frac{xy+yz+xz}{xyz}$

So, the tank will be filled in $\frac{xyz}{xy+yz+xz}$ hours.

Example:

A tank can be filled by pipe A in 2 hours and by pipe B in 6 hours. At 10 A.M., pipe A was opened. At what time will the tank be filled if pipe B is opened at 11 A.M.?

Sol: Given: A tank can be filled by pipe A in 2 hours and by pipe B in 6 hours.

Assume the tank's capacity is 6 units. (Least common multiple of 2 and 6)

Efficiency of a pipe = $\frac{\text{capacity of the tank}}{\text{time taken by the pipe}}$

⇒ Efficiency of pipe A = $\frac{6}{2}$ = 3 and Efficiency of pipe B = $\frac{6}{6}$ = 1

Pipe A was opened at 10 am and Pipe B was opened at 11 A.M.

Units of water filled by A in 1 hour (10 A.M. to 11 A.M.) = 3 units

⇒ Remaining units of water to be filled = 6 – 3 = 3 units

So, the time will be taken by pipe A and pipe B to fill the remaining tank = ($\frac{3}{3+1}$) × 60 = 45 minutes

So, the tank will be filled at 11:45 A.M.

When there is a combination of inlet and outlet

If both inlet and outlet pipes are opened simultaneously, then the net work rate is the difference between their work rate per hour.

So, if an inlet pipe fills a tank in $x$ hours and an outlet pipe empties a tank in $y$ hours, their net work rate per hour is $\frac{1}{x}-\frac{1}{y}=\frac{y-x}{xy}$

If there is more than one inlet or outlet pipe, then first combine the same category pipe's work rate per hour. Then subtract one from another to get the net work rate per hour.

If inlet pipe A fills a tank in $x$ hours, inlet pipe B fills the tank in $y$ hours and outlet pipe C empties a tank in z hours, their net work rate per hour is $\frac{1}{x}+\frac{1}{y}-\frac{1}{z}$

Example:

A tap can fill a cistern in 40 minutes and a second tap can empty the filled cistern in 60 minutes. By mistake without closing the second tap, the first tap was opened. In how many minutes will the empty cistern be filled?

Sol: Tap A fills $\frac{1}{40}$ part in 1 minute.

Tap B empties $\frac{1}{60}$ part in 1 minute.

Both pipe together in 1 minute can fill $=\frac{1}{40}-\frac{1}{60}=\frac{1}{120}$ part

Thus, time taken to fill the tank = 120 minutes

When inlet and outlet function alternatively

Sometimes, problems involve inlet and outlet pipes working alternately. In such cases, we first need to determine the work rates of both the inlet and outlet pipes. Then, we calculate the net work rate for the entire cycle.

Suppose an inlet A and outlet pipe B are alternately opened for an hour each when the tank is initially empty. A fills the tank in $x$ hours and B empties the tank in $y$ hours. So, in how many hours tank will be filled? (y > x)

Work rate of A in 1st hour = $\frac{1}{x}$

Work rate of B in 1st hour = $-\frac{1}{y}$

Total work rate of (A + B) in 2 hours = $\frac{1}{x}-\frac{1}{y}=\frac{y-x}{xy}$

So, the tank will be filled in 2 × $\frac{xy}{y-x}$ hours.

Example:

An inlet pipe can fill an empty tank in $4 \frac{1}{2}$ hours, while an outlet pipe drains a filled tank in $7 \frac{1}{5}$ hours. The tank is initially empty, and the two pipes are alternately opened for an hour each, till the tank is filled, starting with the inlet pipe. In how many hours will the tank be filled?

Sol: An inlet pipe can fill an empty tank in $4\frac{1}{2}$ hours while an outlet pipe drains a filled tank in $7\frac{1}{5}$ hours.

In 1 hour the inlet pipe can fill $\frac{2}{9}$th of the tank.

In 1 hour the outlet pipe can empty $\frac{5}{36}$th of the tank.

In 2 hours the pipes fill $\frac{2}{9} - \frac{5}{36} = \frac{8 - 5}{36}$ of the tank $=\frac{1}{12}$th of the tank

Tank filled in 22 hours $=\frac{1}{12}\times 11 = \frac{11}{12}$

Remaining work $=1-\frac{11}{12}=\frac{1}{12}$

After 22 hours it is the inlet pipe's turn, so the time taken by the inlet pipe to fill the $\frac{1}{12}$ part = $\frac{1}{12}\times \frac{9}{2} = \frac{3}{8}$ hours

So, the tank will be filled in $=22+\frac{3}{8} = 22\frac{3}{8}$ hours

To find the capacity of a tank

The total volume a tank can hold is the total capacity of that tank.

If there is only one inlet or outlet pipe, then we have to multiply the work rate by the total amount of water the pipe can deliver in one hour.

If an inlet pipe can fill a tank in $x$ hours and deliver y gallons of water in the tank in 1 hour, then the capacity of the tank is $xy$ gallons.

If there are two or more two pipes inlet and outlet, then we have to find the work rate per hour. Then multiply by the total amount of water the pipes can deliver in one hour.

If an inlet pipe can fill a tank in $x$ hours and an outlet pipe empties the tank in $y$ hours. If they are opened together, they fill m gallons of water in an hour.

Their net work rate per hour is $\frac{1}{x}-\frac{1}{y}=\frac{y-x}{xy}$

So, the tank is filled in $\frac{xy}{y-x}$ hours. (y > x)

Capacity of the tank = $m × \frac{xy}{y-x}$

Example:

Two inlet pipes can fill a cistern in 10 and 12 hours respectively and an outlet pipe can empty 80 gallons of water per hour. All three pipes working together can fill the empty cistern in 20 hours. What is the capacity (in gallons) of the tank?

Sol: Let the time taken by the outlet pipe to empty the tank be $x$ hour.

One hour's work of pipe A = $\frac{1}{10}$

One hour's work of pipe B = $\frac{1}{12}$

One hour's work of pipe C = $-\frac{1}{x}$

When all pipes work together, the tank will be emptied in 20 hours.

So, $\frac{1}{10}+\frac{1}{12}-\frac{1}{x} =\frac{1}{20}$

⇒$\frac{1}{10}+\frac{1}{12}-\frac{1}{20} =\frac{1}{x}$

⇒$\frac{1}{x}=\frac{6+5-3}{60}$

⇒$\frac{1}{x}=\frac{8}{60}$

$\therefore x = \frac{15}{2}=7.5$

Therefore, the outlet pipe can empty the tank in 7.5 hours.

In one hour, it empties 80 gallons.

In 7.5 hours, it empties 80 × 7.5 = 600 gallons

So, the capacity of the tank is 600 gallons.

Concept of Work and Wages

Work and wage are very important to solve mathematical problems as well as real-life scenarios. To understand this concept properly we have to learn about efficiency.

Efficiency is the ability or time for a man to complete a task.

If a man completes a task in 6 hours, then the efficiency of that man is $\frac{1}{6}$ per hour.

Efficiency ratio is directly proportional to the men's or women's or labour’s wages.

Efficiency is inversely proportional to the time taken.

If a man can complete a task in 6 days and a woman can complete a task in 7 days, if they together works then how will the profits distributed?

Here, efficiency of a man in 1 day = $\frac{1}{6}$

Efficiency of a Woman in 1 day = $\frac{1}{7}$

So, profit ratio will be $\frac{1}{6}:\frac{1}{7}=7:6$

Example:

P, Q, and R can do a piece of work in 60 days, 100 days and 80 days respectively. They together work to finish the work and receive Rs. 2256. Then, P will get ___.

Sol: Given: P, Q and R can do a piece of work in 60 days, 100 days, and 80 days.

The total work of P, Q, and R is LCM of (60, 100, 80) = 1200 units

One day's work of P $=\frac{1200}{60}=20$

One day's work of Q $=\frac{1200}{100}=12$

One day's work of R $=\frac{1200}{80}=15$

The amount will be divided among P, Q, and R as per the ratio of the work done in 1 day.

The ratio of 1 day's work of P, Q, and R = 20 : 12 : 15

The amount received by P $=\frac{20}{20+12+15}\times 2256=\frac{20}{47}\times 2256 =960$

Hence, P will get Rs. 960.

Tips and Tricks

• If an inlet pipe fills a tank in $x$ hours, its work rate is $\frac{1}{x}$ part per hour.

• If an outlet pipe empties a tank in $y$ hours, its work rate is $-\frac{1}{y}$ part per hour.

• if an inlet pipe fills a tank in $x$ hours and an outlet pipe empties a tank in $y$ hours, their net work rate per hour is $\frac{1}{x}-\frac{1}{y}=\frac{y-x}{xy}$

• If the work rate per hour of the outlet pipe or leak is greater than the inlet pipe, then the tank is emptied.

• If the work rate per hour of the outlet pipe or leak is lesser than the inlet pipe, then the tank is emptied.

Practice Questions

Q1. A pipe can fill a tank in 24 hours. Due to a leakage at the bottom, it is filled in 36 hours. If the tank is half full, how much time will the leak take to empty the tank?

1. 48 hours

2. 72 hours

3. 36 hours

4. 24 hours

Hint: Let the total work = LCM of 24 and 36 = 72 units

Answer:

Given: A pipe can fill a tank in 24 hours.

Let the total work = LCM of 24 and 36 = 72 units

One hour work of filling pipe = 72 $\div$ 24 = 3 units

One hour of work filling pipe and the leakage = 72 $\div$ 36 = 2 units

One hour work of the leakage = 3 – 2 = 1 unit

Half capacity of the tank = half of 72 units = 36 units

So, the required time by the leak = $\frac{36}{1}$ = 36 hours

Hence, the correct answer is 36 hours.

Q2. Three pipes A, B, and C can fill a tank in 6 hours. After working together for 2 hours, C is closed and A and B fill the tank in 8 hours. The time (in hours) in which the tank can be filled by pipe C alone is:

1. 10

2. 12

3. 8

4. 9

Hint: Part of the tank was filled by C alone in an hour = (Part of the tank filled by A, B, and C together in an hour) – (Part of the tank filled by A and B together in an hour)

Answer:

Time taken by A, B, and C together to fill the tank = 6 hours

Part of the tank filled by A, B, and C together in an hour = $\frac{1}{6}$

Part of the tank filled by A, B, and C together in the first 2 hours = $\frac{2}{6}$

So, the remaining part of the tank filled by A and B together = 1 – $\frac{2}{6}$ = $\frac{4}{6}$

Time taken by A and B to fill the remaining part of the tank = 8 hours

Time taken by A and B to fill the full tank = 8 ÷ $\frac{4}{6}$ = $\frac{8 × 6}{4}$ = 12 hours

Part of the tank filled by A and B together in an hour = $\frac{1}{12}$

Part of the tank was filled by C alone in an hour

= (Part of the tank filled by A, B, and C together in an hour) – (Part of the tank filled by A and B together in an hour)

= $\frac{1}{6}$ – $\frac{1}{12}$

= $\frac{1}{12}$

So, the time taken by C alone to fill the tank = 12 hours

Hence, the correct answer is 12.

Q3. Pipe X can fill a tank in 20 hours and pipe Y can fill the tank in 35 hours. Both the pipes are opened at alternate hours. If pipe Y was opened first, then how much time (in hours) does it take to fill the tank?

1. $\frac{269}{11}$

2. $\frac{286}{11}$

3. $\frac{179}{7}$

4. $\frac{172}{7}$

Hint: Solve by taking the capacity of the tank as LCM of 20 and 35, then with the help of given conditions find the required value.

Answer:

Given: Pipe X can fill a tank in 20 hours.

Pipe Y can fill the tank in 35 hours.

Let the capacity of the tank = LCM of 20 and 35 = 140 units

Efficiency of X per hour = $\frac{140}{20}$ = 7 units

Efficiency of Y per hour = $\frac{140}{35}$ = 4 units

Pipe Y and pipe X are alternatively opened,

Tank filled in 2 hours = 7 + 4 = 11 units

So, the tank filled in 24 hours = 11 × 12 = 132 units

In the 25th hour, it is Y's turn and the tank will be filled = 132 + 4 = 136 units

The remaining (140 – 136) = 4 units will be filled by X in $\frac{4}{7}$ hours.

So, the total time = (25 + $\frac{4}{7}$) hours = $\frac{179}{7}$ hours

Hence, the correct answer is $\frac{179}{7}$ hours.

Q4. Two pipes, X and Y, can fill a cistern in 24 minutes and 32 minutes, respectively. If both the pipes are opened together, then after how much time (in minutes) should Y be closed so that the tank is full in 18 minutes?

1. 10

2. 8

3. 6

4. 5

Hint: Time taken to complete a work is inversely proportional to efficiency.

Answer:

Time taken by X alone to fill the tank = 24 minutes

Part of work done by X alone in a minute = $\frac{1}{24}$

Time taken by Y alone to fill the tank = 32 minutes

Part of work done by Y alone in a minute = $\frac{1}{32}$

Total time taken = 18 minutes

Part of work done by X in 18 minutes = $\frac{18}{24}$ = $\frac{3}{4}$

Work done by Y = 1–$\frac{3}{4}$ = $\frac{1}{4}$

Time taken by Y before it was closed = $\frac{\frac{1}{4}}{\frac{1}{32}}$ = 8 minutes

Hence, the correct answer is 8.

Q5. If two pipes function simultaneously, a tank is filled in 12 hours. One pipe fills the tank 10 hours faster than the other. How many hours does the faster pipe alone take to fill the tank?

1. 20 hours

2. 18 hours

3. 15 hours

4. 12 hours

Hint: If a pipe alone can fill a tank in $x$ hours, then part of the tank filled by the pipe in 1 hour is $\frac{1}{x}$.

Answer:

Let the slower pipe alone can fill the tank in $x$ hours. Then, faster pipe alone can fill the tank in ($x$ – 10) hours.

Part of the tank filled by the slower pipe in 1 hour = $\frac{1}{x}$

Part of the tank filled by the faster pipe in 1 hour = $\frac{1}{(x – 10)}$

Time taken to fill the tank when both pipes are opened simultaneously = 12 hours

So, part of the tank filled by both the pipes in 1 hour = $\frac{1}{12}$

According to the question,

$\frac{1}{x}$ + $\frac{1}{(x–10)}$ = $\frac{1}{12}$

⇒ 12($x$ – 10) + 12$x$ = $x$($x$ – 10)

⇒ 12$x$ – 120 + 12$x$ = $x$2 – 10$x$

⇒ $x$2 – 34$x$ + 120 = 0

⇒ $x$2 – 30$x$ – 4$x$ + 120 = 0

⇒ $x$($x$ – 30) – 4($x$ – 30) = 0

⇒ ($x$ – 4)($x$ – 30) = 0

⇒ $x$ = 4 or 30

Now, $x$ cannot be 4 as ($x$ – 10) will be negative.

⇒ $x$ = 30

Time taken by the faster pipe alone to fill the tank

= $x$ – 10 = 30 – 10 = 20 hours

Hence, the correct answer is 20 hours.

Q6. Kushal and Ripan accept a piece of work for Rs. 28,800. Working alone, one could do it in 36 days and the other, in 48 days. With the assistance of an expert, they finish it in 12 days. How much remuneration should the expert get?

1. Rs. 10,000

2. Rs. 18,000

3. Rs. 16,000

4. Rs. 12,000

Hint: The amount received by each of them is proportional to their efficiency.

Answer:

Time taken by the first person alone = 36 days

⇒ Part of work done by the first person in a day = $\frac{1}{36}$

Time taken by the second person alone = 48 days

⇒ Part of work done by the second person alone in a day = $\frac{1}{48}$

Let the time taken by the expert alone = $x$ days

⇒ Part of work done by the expert in a day = $\frac{1}{x}$

Time taken by all three working together = 12 days

⇒ Part of work done by all three in a day = $\frac{1}{12}$

According to the question,

$\frac{1}{36}$ + $\frac{1}{48}$ + $\frac{1}{x}=\frac{1}{12}$

⇒ $\frac{1}{x}=\frac{1}{12}-\frac{1}{36}-\frac{1}{48}=\frac{12-4-3}{144}=\frac{5}{144}$

⇒ Ratio of work done by Stanie, Paul, and the expert $=\frac{1}{36}:\frac{1}{48}:\frac{5}{144} = 4:3:5$

So, the amount received by the expert $=\frac{5}{4+3+5}×28800 = 5 × 2400 =$ Rs. $12000$

Hence, the correct answer is Rs. 12,000.

Q7. A and B were assigned to do a job for Rs. 1,200. Working alone, A can do it in 15 days and B, in 12 days. With the help of C, they can finish in 5 days. The share of the amount that C earns is:

1. Rs. 300

2. Rs. 400

3. Rs. 500

4. Rs. 600

Hint: If a man can do the work in $n$ days, then 1 day's work of the man $=\frac{1}{n}$.

Answer:

The time taken by A alone = 15 days

⇒ Part of the work done by A in 1 day = $\frac{1}{15}$

The time taken by B alone = 12 days

⇒ Part of the work done by B in 1 day = $\frac{1}{12}$

Let the time taken by C alone = $x$

⇒ Part of the work done by C in 1 day = $\frac{1}{x}$

The time taken by A, B, and C = 5 days

⇒ Part of the work done by A, B, and C together in 1 day = $\frac{1}{5}$

According to the question,

$\frac{1}{5}=\frac{1}{15}+\frac{1}{12}+\frac{1}{x}$

⇒ $\frac{1}{x}=\frac{1}{5}-\frac{1}{12}-\frac{1}{15}=\frac{12 – 5 – 4}{60} = \frac{1}{20}$

The ratio of the amounts A, B, and C earn $= \frac{1}{15} : \frac{1}{12} :\frac{1}{20} = 4: 5: 3$

Since total amount = 1200,

Share of the amount earned by C

= $\frac{3}{12}× 1200$

= Rs. $300$

Hence, the correct answer is Rs. 300.

Q8. A certain factory employed 600 men and 400 women and the average wage was Rs. 2.55 per day. If a woman got 50 paise less than a man, the daily wages of a man and a woman would be _____.

1. Man Rs. 2.75 and Woman Rs. 2.25

2. Man Rs. 5.30 and Woman Rs. 2.50

3. Man Rs. 2.50 and Woman Rs. 2.00

4. Man Rs. 3.25 and Woman Rs. 2.75

Hint: To determine a man and a woman's daily wages, first calculate the combined wages of 600 men and 400 women.

Answer:

Given: The factory employed 600 men and 400 women and the average wage was Rs. 2.55 per day.

The total wages of 600 men and 400 women are (600 + 400) × 2.55 = Rs. 2550

Let a man's wage be $x$.

So, a woman's wage $=(x-\frac{50}{100}) = x-0.5$

So, the total wages of the 600 men and 400 women $=600x+400(x-0.5)$

According to the question,

$600x+400(x-0.5)=2550$

$⇒1000x=2750$

$\therefore x=2.75$

So, a man's wage is Rs. 2.75.

⇒ A woman's wage = (2.75 – 0.5) = Rs. 2.25

Hence, the correct answer is Man Rs. 2.75 and Woman Rs. 2.25.

Q9. X and Y can complete a piece of work in 12 and 60 days respectively. They are contracted to complete the work together for Rs. 24,000. What will be the share of X?

1. Rs. 18,000

2. Rs. 16,000

3. Rs. 20,000

4. Rs. 21,000

Hint: The share is divided between X and Y in the 1 day's work ratio of X and Y.

Answer:

Given: X and Y can complete a piece of work in 12 and 60 days.

The total work of X and Y is LCM of (12, 60) = 60 units

1 day's work by X $=\frac{60}{12}=5$

1 day's work by Y $=\frac{60}{60}=1$

So, the ratio of their efficiency $= 5 : 1$

Share of X $=\frac{5}{6}\times 24000=$ Rs. $20000$

Hence, the correct answer is Rs. 20,000.

Q10. A water tap fills a tub in '$p$' hours and a sink at the bottom empties it in '$q$' hours. If $p < q$, both tap and sink are open, and the tank is filled in '$r$' hours, then:

1. $\frac{1}{r}$ = $\frac{1}{p}+\frac{1}{q}$

2. $\frac{1}{r}$ = $\frac{1}{p}-\frac{1}{q}$

3. $r = p + q$

4. $r = p - q$

Hint: If a pipe fills a tank in $n$ hours, then in 1 hour the pipe can fill $\frac{1}{n}$ part of the tank.

Answer:

Given: A water tap fills a tub in '$p$' hours and a sink at the bottom empties it in '$q$' hours where $p < q$.

So, in 1 hour the tap will fill $\frac{1}{p}$ part of the tub.

Also, in 1 hour the sink will empty $\frac{1}{q}$ part of the tub.

Now, part of the tub that will fill in 1 hour with both of them opened = ($\frac{1}{p}-\frac{1}{q}$)

Time taken to fill the whole tub = $\frac{1}{(\frac{1}{p}–\frac{1}{q})}$ hours, which is equal to $r$.

Therefore, $\frac{1}{(\frac{1}{p}–\frac{1}{q})}$ = $r$

⇒ $\frac{1}{r}$ = $\frac{1}{p}-\frac{1}{q}$

Hence, the correct answer is $\frac{1}{r}$ = $\frac{1}{p}-\frac{1}{q}$.