Quadratic Equations: Definition, Calculator, Questions, Formula, Examples

Quadratic equations are a fundamental part of algebra and mathematics as a whole. They appear in various fields such as physics, engineering, economics, and biology. Understanding how to solve quadratic equations is crucial for students and professionals alike, as it provides a foundation for more advanced mathematical concepts and real-world problem-solving. Topics such as quadratic equations, roots of quadratic equations, how to form a quadratic equation, and the quadratic equations formula are essential for mastering this subject. The sum and product of roots of quadratic equations in real life, their derivation, and their application are significant for various practical scenarios. Tools like a quadratic equations solver and resources like a quadratic equations word problems worksheet with answers help in grasping the concepts effectively. Solving quadratic equations and understanding their real-world applications, such as through quadratic equations word problems, is a vital skill, supported by resources like the sum and product of roots of quadratic equation calculator and related questions.

In this article, we are going to discuss quadratic equations for class 10, quadratic equations for SSC, and quadratic equations examples and answers.

What is a Quadratic Equation

A quadratic equation is a type of polynomial equation of degree two, meaning the variable can have a maximum power of 2. The graph of a quadratic equation is a parabola, which can open either upwards or downwards depending on the coefficients.

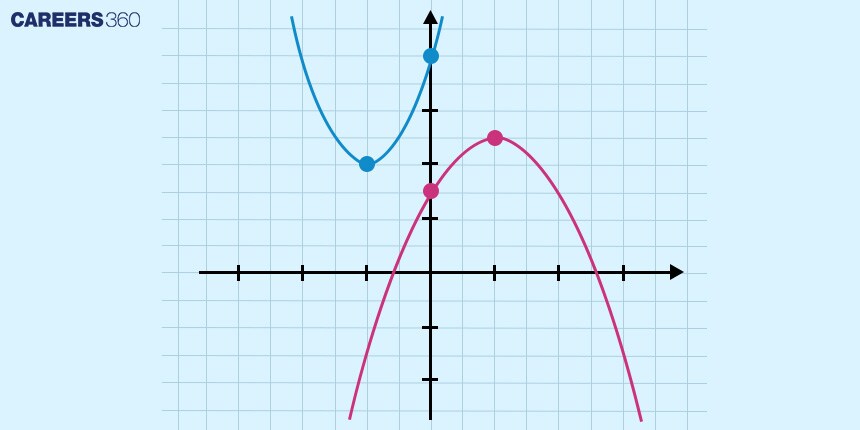
The standard form of a Quadratic Equation

The standard form of a quadratic equation is $ax^2+bx+c=0$, where $a,b,c$ are constants and $a \neq 0$.

Here, we can say $a$ is the co-efficient of $x^2$, $b$ is the co-efficient of $x$, and $c$ is the constant term of the quadratic equation.

Roots/Solution of a Quadratic Equation

The value of the variable for which the quadratic equation becomes equal to zeros are the solutions to the quadratic equation which are known as the roots of the equation. These roots can be real or complex numbers. A quadratic equation can have a maximum of two roots.

Methods of Solving a Quadratic Equation

There are several methods of solving a quadratic equation which include splitting the middle term, completing the square, or applying the quadratic formula. Let us discuss them one by one with proper examples.

Method of Splitting the middle term

This method involves expressing the middle term of a quadratic equation as the sum of two terms whose product is equal to the product of the coefficient of $x^2$ and the constant term of the quadratic equation.

Example: Solve $x^2+5x+6=0$ using the middle-term split method.

Solution:

$x^2+5x+6=0$

⇒ $x^2+(3+2)x+6=0$

⇒ $x^2+3x+2x+6=0$

⇒ $x(x+3)+2(x+3)=0$

⇒ $(x+3)(x+2)=0$

⇒ $x+3=0$ or $x+2=0$

⇒ $x=-3$ or $x=-2$

Hence, the solutions of $x^2+5x+6=0$ are -3 and -2.

Method of completing the square

The method of completing the square involves transforming a quadratic equation into a perfect square trinomial, making it easier to solve. This is done by adding and subtracting the same value, to rewrite the equation in the form $(x+p)^2=q$. Solving this equation gives the roots of the original quadratic equation.

Example: Solve $x^2+10x-11=0$ using the completing square method.

Solution:

$x^2+10x-11=0$

⇒ $x^2+10x+25-36=0$

⇒ $x^2+2×x×5+5^2-36=0$

⇒ $(x+5)^2-(6)^2=0$

⇒ $(x+5+6)(x+5-6)=0$

⇒ $(x+11)(x-1)=0$

⇒ $x=-11$ or $x=1$

Hence, the solutions of $x^2+5x+6=0$ are -11 and 1.

Quadratic Formula

The quadratic formula method is a straightforward way to find the roots of a quadratic equation. By substituting the coefficients of the quadratic equation into the formula given below, we can determine the solutions directly. This method works for all quadratic equations, regardless of whether they are factorable or not.

$x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}$

Where $a$ is the co-efficient of $x^2$, $b$ is the co-efficient of $x$, and $c$ is the constant term of the quadratic equation.

Example: Solve $x^2-10x+9=0$, using the quadratic formula.

Solution:

Comparing $x^2-10x+9=0$ with $ax^2+bx+c=0$ we get $a=1, b=-10, c= 9$

Using the quadratic formula we get,

$x=\frac{-(-10)\pm \sqrt{(-10)^2-4×1×9}}{2×1}$

⇒ $x=\frac{10\pm \sqrt{100-36}}{2}$

⇒ $x=\frac{10\pm \sqrt{64}}{2}$

⇒ $x=\frac{10\pm 8}{2}$

⇒ $x=\frac{10+8}{2}$ or $x=\frac{10-8}{2}$

⇒ $x=9$ or $x=1$

Hence, the solutions of $x^2-10x+9=0$ are 9 and 1.

Nature of roots

The nature of the roots of a quadratic equation refers to the type and characteristics of the solutions based on the discriminant ($b^2 - 4ac$). The discriminant helps to determine whether the roots are real or complex and whether they are distinct or equal. Understanding the nature of the roots provides insight into the behaviour of the quadratic equation's graph and its intersections with the x-axis.
The roots can be real and unequal, the roots can be equal, and the roots can be non-real. This can be calculated without solving the equation with the help of the value of the discriminant.
Now we will understand the concept of discriminant first.

Concept of Discriminant

The discriminant of a quadratic equation is a mathematical expression derived from its coefficients ($a,b,c$) of the equation $ax^2+bx+c=0$. It plays a crucial role in determining the nature of the roots of the equation. The discriminant is denoted by ‘D’ and it is calculated using the formula $D=b^2-4ac$.

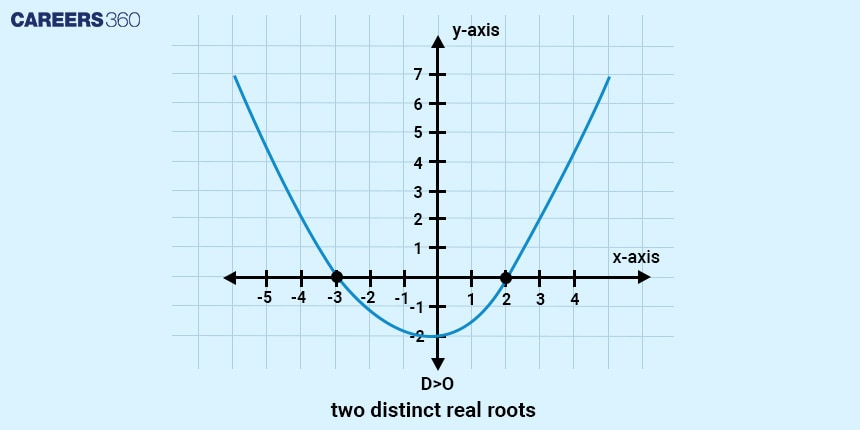
Condition of distinct real roots:

The condition for a quadratic equation to have two distinct real roots is when the value of the discriminant is greater than zero ($D>0$). This indicates that the quadratic equation has two different real solutions, meaning the parabola intersects the x-axis at two distinct points.

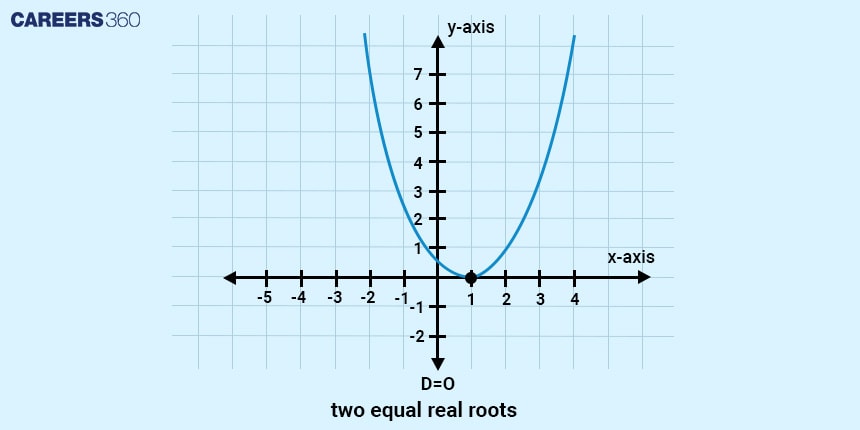
Condition of two equal real roots:

The condition for a quadratic equation to have two equal real roots is when the value of the discriminant equals zero ($D=0$). This means that the quadratic equation has a perfect square trinomial form, resulting in a single repeated solution. Graphically, the parabola representing the equation touches the x-axis at exactly one point.

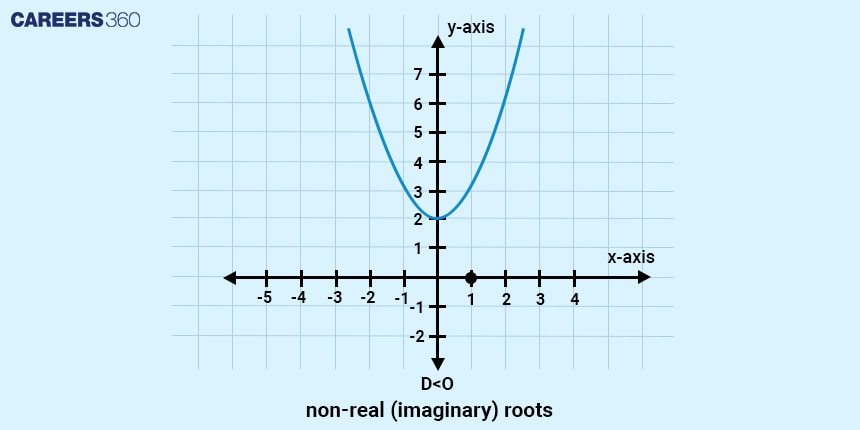
Condition of non-real roots

The condition of non-real roots for a quadratic equation occurs when the value of the discriminant is less than zero ($D<0$) i.e. the discriminant is negative. In such cases, the solutions to the quadratic equation are complex numbers. Geometrically, this means the parabola described by the equation does not touch the x-axis.

Relation between roots and coefficients of a quadratic equation

The relationship between the roots and coefficients of a quadratic equation reveals insightful information about the quadratic equation. These relationships include how the sum and product of the roots can be directly related to the coefficients of the equation.

Sum of roots

If $\alpha$ and $\beta$ are the two roots of a quadratic equation $ax^2+bx+c=0$, then the sum of the roots is given by the formula: $\alpha + \beta = \frac{-b}{a}$, where $a$ is the co-efficient of $x^2$, $b$ is the co-efficient of $x$ of the quadratic equation.

Illustration:

For equation $2x^2-6x+ 1= 0$

The sum of the roots = $\frac{6}{2}=3$

Product of roots

If $\alpha$ and $\beta$ are the two roots of a quadratic equation $ax^2+bx+c=0$, then the product of the roots is given by the formula: $\alpha \beta = \frac{c}{a}$, where $a$ is the co-efficient of $x^2$, $c$ is the constant term of the quadratic equation.

Illustration:

For equation $2x^2-6x+ 1= 0$

Product of the roots = $\frac{1}{2}$

Formation of Quadratic Equation using the sum of the roots and the product of the roots:

If S is the sum of the roots and P is the product of the roots. Then, the quadratic equation is given by
$x^2-Sx+P=0$

Illustration:

If the sum of the roots of a quadratic equation is $\frac{3}{2}$ and the product of roots is $\frac{1}{4}$, Then the quadratic equation is given by

$x^2-\frac{3}{2}x+ \frac{1}{4}= 0$

I.e. $4x^2-6x+ 1= 0$

Word Problems on Quadratic Equations
Word problems on quadratic equations can be of several types as given below:

• Problems related to speed, time, and distance.

• Problems on boats and streams

• Problems on ages

• Problems involving the formation of quadratic equations to find the unknown
  
  

Example: The ratio of the father's age to his son's age is 5 : 3. The product of the numbers representing their ages is 960. The ratio of their ages after 6 years will be:

Solution:

The ratio of the ages of father to his son = 5 : 3

Let the age of the father = $5x$

The age of the son = $3x$

Product of their ages = 960

⇒ $(5x)(3x) = 960$

⇒ $15x^2 = 960$

⇒ $x^2 = 64$

⇒ $x = 8$ (since age can not be negative)

So, the age of father = $5x$ = 5 × 8 = 40 years

⇒ The age of son = $3x$ = 3 × 8 = 24 years

The age of father and son after 6 years will be

⇒ Father's age = 40 + 6 = 46 years

⇒ Son's age = 24 + 6 = 30 years

⇒ The ratio of the ages of father to son = Father's age : Son's age = 46 : 30 = 23 : 15

Hence, the correct answer is $23:15$.

Tips and Tricks

• Always simplify the quadratic equation to the standard form before solving.

• Check the discriminant to understand the nature of the roots before solving.

• Practice different methods to find the one that works best for you.

• Use the quadratic formula when the equation is not easily factorable.

Practice Questions/Solved Examples

Q.1. What is the value of $x$ in the equation $\sqrt{\frac{1+x}{x}}-\sqrt{\frac{x}{1+x}}=\frac{1}{\sqrt6}$?

1. –2

2. 3

3. 2

4. None of these

Hint: Square both sides of the given equation and frame a quadratic equation to find its roots.

Solution:

Given: $\sqrt{\frac{1+x}{x}}-\sqrt{\frac{x}{1+x}}=\frac{1}{\sqrt6}$

Squaring both sides

⇒ $\left(\sqrt{\frac{1+x}{x}}-\sqrt{\frac{x}{1+x}}\right)^{2}=\left(\frac{1}{\sqrt6}\right)^{2}$

⇒ $\frac{1+x}{x} +\frac{x}{1+x}-2=\frac{1}{6}$

⇒ $\frac{1+x}{x} +\frac{x}{1+x}=\frac{13}{6}$

⇒ $\frac{1+x^{2}+2x+x^{2}}{x+x^{2}}=\frac{13}{6}$

⇒ $6+6x^{2}+12x+6x^{2}=13x+13x^{2}$

⇒ $6+12x^{2}+12x=13x+13x^{2}$

⇒ $x^{2}+x-6=0$

⇒ $x^{2}+3x-2x-6=0$

⇒ $x(x+3)-2(x+3)=0$

⇒ $(x+3)(x-2)=0$

⇒ $x=-3, 2$

From the given options we can say that the value of $x$ is 2.

Hence, the correct answer is option (3).

Q.2. The sum of twice a number and thrice its reciprocal is $\frac{25}{2}$. What is the number?

1. 7

2. 6

3. 5

4. 4

Hint: Let the number be $x$. Then use the given information to make a quadratic equation.

Solution:

Given: The sum of a twice a number and thrice its reciprocal is $\frac{25}{2}$.

Let the number be $x$.

According to the question,

$2x+\frac{3}{x}=\frac{25}{2}$

$\Rightarrow 4x^2+6=25x$

$\Rightarrow 4x^2-25x+6=0$

$\Rightarrow 4x^2-24x-x+6=0$

$\Rightarrow (4x-1)(x-6)=0$

$\Rightarrow (4x-1)=0$ or $(x-6)=0$

$\Rightarrow x=6$ or $\frac{1}{4}$

After checking the options, we get, $x=6$

Hence, the correct answer is option (2).

Q.3. If the sum of a non-zero number and thrice its reciprocal is $\frac{52}{7}$. Find the number.

1. 8

2. 9

3. 7

4. 6

Hint: Let the number be $x$. Then use the given information to make a quadratic equation.

Solution:

Given: The sum of a non-zero number and thrice its reciprocal is $\frac{52}{7}$.

Let the number be $x$.

According to the question,

$x+\frac{3}{x}=\frac{52}{7}$

$\Rightarrow 7x^2-52x+21=0$

$\Rightarrow (7x-3)(x-7)=0$

$\Rightarrow (7x-3)$ or $(x-7)=0$

$\Rightarrow x=7$ or $ \frac{3}{7}$

After checking the options, we get, $x=7$

Hence, the correct answer is option (3).

Q.4. If $(x-2)(x-p) = x^2-ax+6$, then the value of $(a - p)$ is:

1. 0

2. 1

3. 2

4. 3

Hint: Expand the brackets and compare both sides of the equation.

Solution:

Given: $(x-2)(x-p) = x^2-ax+6$

⇒ $x^2-px-2x+2p=x^2-ax+6$

⇒ $x^2-(p+2)x+2p=x^2-ax+6$

By comparing both sides of the equation,

$p+2=a$

⇒ $a-p=2$

Hence, the correct answer is option (3).

Q.5. If $x^{2}+y^{2}+2x+1=0$, then the value of $x^{31}+y^{35}$ is:

1. –1

2. 0

3. 1

4. 2

Hint: The addition of two positive squares is zero only if the numbers are zero individually. Use this information to solve the question.

Solution:

Given:

$x^{2}+y^{2}+2x+1=0$

⇒ $x^{2}+2x+1+y^{2}=0$

⇒ $(x+1)^2+y^2=0$

Since the addition of the squares of the two terms is zero,

So, both the terms are individually zero.

Therefore, $(x+1)^2=0$ and $y^2=0$.

⇒ $x=-1$ and $y=0$

Now, $x^{31}+y^{35}$

= $(-1)^{31}+(0)^{35}$

= –1

Hence, the correct answer is option (1).

Q.6. If the sum of the roots of a quadratic equation is 1 and the product of the roots is –20, find the quadratic equation.

1. $x^{2}–x–20=0$

2. $x^{2}+x+20=0$

3. $x^{2}+x–20=0$

4. $x^{2}–x+20=0$

Hint: By using the concept of a quadratic equation: $x^{2} – (\text{sum of the root})x+(\text{product of the root})=0$ finding the desired equation.

Solution:

Given: The sum of the roots = 1

The product of the roots is = –20

We know that quadratic equation: $x^{2}–(\text{sum of the root})x+(\text{product of the root})=0$

⇒ $x^{2}–(1)x+(–20)=0$

⇒ $x^{2}–x–20=0$

Hence, the correct answer is option (1).

Q.7. What is the difference between the cube and square of the common root of $x^2-8x+15=0$ and $y^2+2y-35=0$

1. 76

2. 100

3. 294

4. 318

Hint: Find the roots of both equations to get the common root using factorisation.

Solution:

Here,

$x^2-8x+15=0$

⇒ $(x-5)(x-3)=0$

So, $x=5,3$

$y^2+2y-35=0$

⇒ $(y-5)(y+7)=0$

So, $y=5,-7$

Here, the common root is 5.

So, the difference between the cube and square of 5 $=(5^3-5^2)=125-25=100$

Hence, the correct answer is option (2).

Q.8.

If $2\left [x^{2} +\frac{1}{x^{2}}\right]-2\left [x-\frac{1}{x} \right]-8=0$, what are the two values of $\left (x-\frac{1}{x} \right)\;$?

1. –1 or 2

2. 1 or –2

3. –1 or –2

4. 1 or 2

Hint: Use the formula, $a^2+b^2=(a-b)^2+2ab$, and factorisation by splitting the middle term.

Solution:

Given: $2\left [x^{2} +\frac{1}{x^{2}} \right]-2\left [ x-\frac{1}{x} \right]-8=0$

$⇒2\left [(x -\frac{1}{x})^{2}+2 \right]-2\left [ x-\frac{1}{x} \right ]-8=0$

Let $(x -\frac{1}{x})=t$

The equation becomes $2(t^{2}+2)-2t-8=0$

$⇒2t^{2}-2t-4=0$

$⇒t^{2}-2t+t-1=0$

$⇒(t-2)(t+1)=0$

$⇒t=-1,2$

So, $(x -\frac{1}{x})=-1,2$

Hence, the correct answer is option (1).

Q.9. If $x-\frac{1}{x}=1$, then what is the value of $\left (\frac{1}{x-1}-\frac{1}{x+1}+\frac{1}{x^{2}+1}-\frac{1}{x^{2}-1} \right)\;$?

1. $\pm \sqrt{5}$

2. $\frac{2}{5}$

3. $\pm\frac{2}{\sqrt{5}}$

4. $\pm\frac{\sqrt{5}}{2}$

Hint: Given: $x-\frac{1}{x}=1$ or, $x^{2}-1=x$ or, $x^{2}+1=x+2$

Substitute, $x^{2}-1=x$ and $x^{2}+1=x+2$

Solution:

Given: $x-\frac{1}{x}=1$

$⇒x^{2}-1=x$

$⇒x^{2}+1=x+2$

$\left (\frac{1}{x-1}-\frac{1}{x+1}+\frac{1}{x^{2}+1}-\frac{1}{x^{2}-1} \right)$

$=(\frac{2}{x^{2}-1}+\frac{1}{x^{2}+1}-\frac{1}{x^{2}-1})$

$=(\frac{1}{x^{2}-1}+\frac{1}{x^{2}+1})$

$=(\frac{2x^{2}}{(x^{2}-1)(x^{2}+1)})$

Using $x^{2}-1=x$ and $x^{2}+1=x+2$, we get,

$=(\frac{2x^{2}}{(x)(x+2)})$

$=\frac{2x}{x+2}$

Solving $x^{2}-x-1=0$, we get $x=\frac{1\pm\sqrt{5}}{2}$

So, $x+2=\frac{5\pm\sqrt{5}}{2}$

When $x=\frac{1+\sqrt{5}}{2}$, $\frac{2x}{x+2}=\frac{2}{\sqrt{5}}$

When $x=\frac{1-\sqrt{5}}{2}$, $\frac{2x}{x+2}=-\frac{2}{\sqrt{5}}$

Hence, the correct answer is option (3).

Q.10. If $\sqrt{x}=\sqrt{3}-\sqrt{5}$, then the value of $x^2-16x+6$ is:

1. $0$

2. $-2$

3. $2$

4. $4$

Hint: Square both sides of the equation twice to get the result.

Solution:

Given: $\sqrt{x}=\sqrt{3}-\sqrt{5}$

By squaring both the sides, we get,

$x=3+5-2\sqrt{15}$

⇒ $x-8=-2\sqrt{15}$

Again, squaring both the sides, we get,

$x^2-16x+64=60$

⇒ $x^2-16x+4=0$

$\therefore x^2-16x+6=2$

Hence, the correct answer is option (3).