Rational Numbers

Rational numbers are a subset of real numbers bridging the gap between integers and more complex numbers. The word 'rational' originated from the word 'ratio', which implies that rational numbers can be written as a ratio of two integers.

Defining the Rational Numbers

A rational number can be defined as a fraction, where the numerator and the denominator are both integers, and the denominator is not equal to zero.
Some examples of rational numbers are $\frac{2}{3}$, $\frac{0}{1}$, $-\frac{9}{8}$, 0.7 or $\frac{7}{10}$, 0.343434….. (which can be written as $\frac{34}{99}$) etc.

CAT 2024: 20 Free Mock Test | 10 Year PYQs | 60 Day Study Material | Most Scoring Concepts

XAT 2025: Section-wise Preparation Tips | Sample Paper

Don't Miss: SNAP 2024 Sample Papers | NMAT 2024 Sample Papers | MAT 2024 Sample Papers

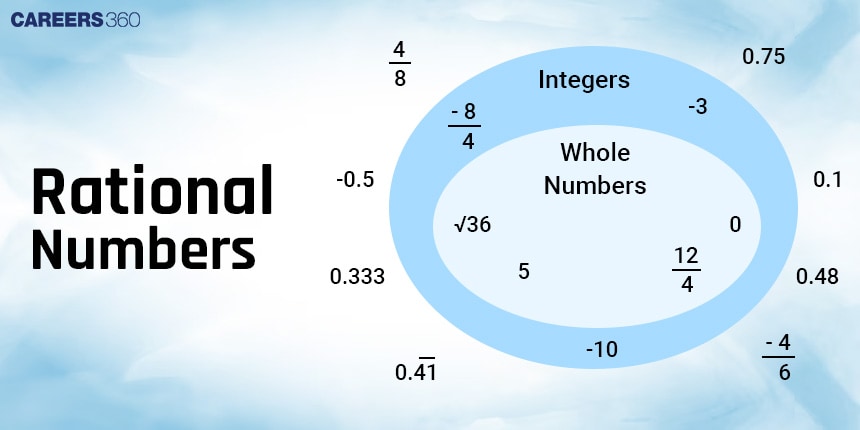
Differentiating Rational Numbers from Irrational Numbers:

Rational numbers can be expressed as the ratio of two integers such as $\frac{p}{q}$ where p and q are integers and q $\ne$ 0.

On the other hand, non-rational or irrational numbers can’t be expressed as a simple fraction of two integers.

For example $\pi$, $\sqrt{2}$, $\sqrt{3}$ are irrational numbers.

These numbers have decimal expansions that are non-repeating and non-terminating.

Note - Terminating decimals have a finite number of digits after the decimal point, and Non-terminating decimals have infinite digits after the decimal point.

How to identify rational numbers and Irrational numbers:

A number is rational if it has terminating or repeating decimals.

Examples: $\frac{1}{5}$ = 0.2, $\frac{3}{4}$ = 0.75, 0.3333…. = $\frac{3}{9}$ etc.

On the other hand, if a number has non-terminating or non-repeating decimals, then it is an irrational number.

Examples: 0.15734582…., 3.575775777…., $\pi$, $\sqrt{3}$ etc.

Arithmetic Operations on Rational Numbers:

In mathematics, arithmetic operations are the basic operations i.e. addition, subtraction, multiplication, and division, performed on integers. Let us discuss here how we can perform these operations on rational numbers, say $\frac{a}{b}$ and $\frac{c}{d}$.

• Addition: When we add $\frac{a}{b}$ and $\frac{c}{d}$, we need to make the denominator the same. Hence, we get $\frac{a}{b}+\frac{c}{d}=\frac{ad+bc}{bd}$.

• Example: $\frac{2}{3}+\frac{3}{4}=\frac{8+9}{12}=\frac{17}{12}$

• Subtraction: Similarly, if we subtract $\frac{a}{b}$ and $\frac{c}{d}$, then also, we also need to make the denominator the same, first, and then do the subtraction. Hence, we get $\frac{a}{b}-\frac{c}{d}=\frac{ad-bc}{bd}$.

• Example: $\frac{2}{3}-\frac{1}{2}=\frac{4-3}{6}=\frac{1}{6}$

• Multiplication: In the case of multiplication, while multiplying two rational numbers, the numerator and denominators of the rational numbers are multiplied, respectively. If $\frac{a}{b}$ is multiplied by $\frac{c}{d}$, then we get $\frac{a\times c}{b\times d}$.

• Example: $\frac{4}{5}\times \frac{3}{7}=\frac{4\times 3}{5\times 7}=\frac{12}{35}$

• Division: If $\frac{a}{b}$ is divided by $\frac{c}{d}$, then it is represented as:

• $\frac{a}{b}÷\frac{c}{d}=\frac{a}{b}\times \frac{d}{c}=\frac{ad}{bc}$
• Example: $\frac{2}{5}÷\frac{5}{6}=\frac{2}{5}\times \frac{6}{5}=\frac{12}{25}$

Properties of Rational Numbers:

The basic properties of rational numbers are as follows:

• Closure property: When any two rational numbers are added, subtracted, or multiplied the result of these operations will always give a rational number.

• The division does not come under closer property as division by 0 is not defined.

• Commutative property: The result remains the same when any rational numbers are added or multiplied in any order.

• For example, if a and b are two rational numbers then a + b = b + a and a $\times$ b = b $\times$ a
• Subtraction and division are not commutative for real numbers as a - b $\ne$ b - a and a $÷$ b $\ne$ b $÷$ a.

• Associative property: When any three rational numbers are added or multiplied the result remains the same irrespective of the way numbers are grouped.

• For example, if x, y and z are rational numbers, then x + (y + z) = (x + y) + z and x $\times$ (y $\times$ z) = (x $\times$ y) $\times$ z
• Subtraction and division are not associative as x - (y - z) $\ne$ (x - y) - z and x $÷$ (y $÷$ z) = (x $÷$ y) $÷$ z.

• Distributive property: For any three rational numbers a, b, and c we can say that,
  a $\times$ (b + c) = a $\times$ b + a $\times$ c
  a $\times$ (b - c) = a $\times$ b - a $\times$ c

• Additive identity and inverse property: The additive identity property of rational numbers states that the sum of any rational number and zero is the rational number itself. Here, 0 is the additive identity for rational numbers.

• Also, the additive inverse property of rational numbers states that if $\frac{a}{b}$ is a rational number, then there exists a rational number $-fracab$ such that,
• $\frac{a}{b}$ + ($-fracab$) = 0.
• Here, $-fracab$ is the additive inverse of $fracab$.

• Multiplicative identity and inverse property: The multiplicative identity property of rational numbers states that the product of any rational number and 1 is the rational number itself. Here, 1 is the multiplicative identity for rational numbers.

Also, the multiplicative inverse property of rational numbers states that if $\frac{a}{b}$ is a rational number, then there exists a rational number $fracba$ such that,

$\frac{a}{b}\times \left(\frac{b}{a}\right)=1$.

Here, $\frac{b}{a}$ is the multiplicative inverse of $\frac{a}{b}$.

Conversion of decimal numbers to fractions:

There are two types of decimals: terminating and non-terminating.

Terminating decimals have a finite number of digits after the decimal point.

Examples are 0.5, 2.41, 57.385 etc.

Non-terminating decimals have an infinite number of digits after the decimal point.

Examples are 0.23725485…, 2.353553555…., 1.232342345…. etc.

Converting terminating decimals to fractions:

Let's take a terminating decimal number as 0.57, now we are going to convert it into a fraction.
First, we determine the place value of the last digit of the decimal, 7.

The last digit is in the hundredth place, so we can write the fraction with a denominator of 100.
When writing as a fraction we don’t need the decimal point.
Therefore, 0.57 can be written as $\frac{57}{100}$.

Similarly 0.9 = $\frac{9}{10}$, 1.35 = $\frac{135}{100}$, 25.382 = $\frac{25382}{1000}$ etc.

Converting non-terminating but repeating decimals to fractions:

Let's take a non-terminating but repeating decimals as 0.4444….

Now let x = 0.4444….

Since there is one repetitive digit, multiplying x = 0.4444…. by 10

We get, 10x = 4.4444….

Subtracting both the equation we get, 9x = 4

Therefore, x = $\frac{4}{9}$

So, 0.4444…. = $\frac{4}{9}$

Let’s take another example - convert 1.3454545…. to a fraction.

Now, let y = 1.3454545….

The repeating digits are 64. So, let us multiply this equation by 10 such that we will have repeating digits after the decimal point.

We get, 10y = 13.454545…. _______(1)

Now, let us multiply y = 1.3454545….. by 1000 so that we will have the decimal point to the right of the repeating digits. So, 1000y = 1345.454545…. _______(2)

Subtracting equation (1) from equation (2) we get,

990y = 1332 i.e. y = $\frac{1332}{990}$

Therefore, 1.3454545…. = $\frac{1332}{990}$

There is also a short trick to convert the non-terminating but repeating decimals to fractions.

For the repeating decimals of the form $0.\overline{abc}$

$0.\overline{abc}$ = $\frac{\text{Repeated digits}}{\text{Number of 9's equal to the number of digits}}$

Also, for the repeating decimals of the form $p.a\overline{bc}$

$p.a\overline{bc}$ = $\frac{abc-pa}{\text{Number of 9's equal to the number of repeating terms followed by the number of 0’s equal to the number of non-repeating terms after the decimal point}}$

Examples: 0.232323…. = $\frac{23}{99}$

0.7444….. = $\frac{74-7}{90}$ = $\frac{67}{90}$

6.95454…. = $\frac{6954-69}{990}$ = $\frac{6885}{990}$

Practice Questions on rational numbers

Q.1. Find out which of the following is a rational number

1. 7

2. $\pi$

3. $\sqrt{5}$

4. 1.232232223….

Solution:

7 can be written as $\frac{7}{1}$, so it is a rational number.

$\pi$, $\sqrt{5}$, and 1.232232223…. These numbers can not be expressed as fractions, where the numerator and the denominator are both integers, and the denominator is not equal to zero, so these are not rational numbers.

Hence, the answer is the option (1).

Q.2. $\frac{1}{0}$ is a rational number. (True/False)

1. True

2. False

Solution:

A rational number can be defined as a fraction, where the numerator and the denominator are both integers, and the denominator is not equal to zero.

$\frac{1}{0}$ is not a rational number as the denominator of this fraction is zero.

Hence, the answer is the option (2).

Q.3. What is the additive inverse of $-\frac{5}{7}$?

1. 0

2. 1

3. $\frac{-6}{7}$

4. $\frac{5}{7}$

Solution:

We know that the sum of the number and its additive inverse is equal to 0.

So, the additive inverse of $-\frac{5}{7}$ is $\frac{5}{7}$, as $-\frac{5}{7}$ + $\frac{5}{7}$ = 0

Hence, the answer is the option (4).

Q.4. Find the number that should be added to $\frac{3}{5}$ to get the number $\frac{8}{9}$.

1. $\frac{12}{45}$

   $\frac{14}{45}$

   $\frac{13}{45}$

2. 0

Solution:

The number that should be added to $\frac{3}{5}$ to get the number $\frac{8}{9}$ is

$\frac{8}{9}-\frac{3}{5}=\frac{40-27}{45}=\frac{13}{45}$

Hence, the answer is the option (3).

Q.5. Find the value of $2.34\bar{5}$ in fraction.

1. 0

2. $\frac{2111}{900}$

3. $\frac{2111}{990}$

4. None of these

Solution:

$2.34\bar{5}$ = $\frac{2345-234}{900}$ = $\frac{2111}{900}$

Hence, the answer is the option (2).

Q.6. The addition and multiplication of rational numbers follow

1. Commutative property

2. Associative property

3. Both

4. None of these

Solution:

The addition and multiplication of rational numbers follow both the commutative property and the associative property.

Hence, the answer is the option (3).

Q.7. For any two rational numbers x and y we can say that x + y = y + x. (True/False)

1. True

2. False

Solution:

The addition of rational numbers is commutative, so for any two rational numbers x and y, we can say that x + y = y + x.

Hence, the answer is the option (1).

Q.8. What is the sum of the multiplicative inverse and the additive inverse of 5?

1. $-\frac{23}{5}$

2. $-\frac{24}{5}$

3. $\frac{24}{5}$

4. $-\frac{25}{5}$

Solution:

The multiplicative inverse of 5 is $\frac{1}{5}$.

The additive inverse of 5 is (-5).

So, the required sum = $\frac{1}{5}$ - 5 = $-\frac{24}{5}$

Hence, the answer is the option (2).

Q.9. The division of rational numbers is commutative. (True/False)

1. True

2. False

Solution:

The division of rational numbers is not commutative as 10 $÷$ 5 $\ne$ 5 $÷$ 10.

Hence, the answer is the option (2).

Q.10. Reciprocal of (-2) is:

1. 2

2. $\frac{1}{2}$

3. $-\frac{1}{2}$

4. $\frac{1}{3}$

Solution:

The reciprocal of a number is the inverse of that number.

So, the reciprocal of (-2) is ($-\frac{1}{2}$).

Hence, the answer is the option (3).

Q.11. The product of a nonzero rational number with an irrational number is always an irrational number. (True/False)

1. True

2. False

Solution:

The product of a non-zero rational number with an irrational number is always irrational.

For example, $\frac{2}{3}$ is a rational number and $\sqrt{3}$ is an irrational number

Now, $\frac{2}{3}\times \sqrt{3}=\frac{2}{\sqrt{3}}$ is an irrational number.

Hence, the answer is the option (1).

Q.12. Find the value of $0.\bar{2}+0.\bar{7}$.

1. $\frac{3}{4}$

2. 0

3. 2

4. 1

Solution:

$0.\bar{2}+0.\bar{7}$

= $\frac{2}{9}+\frac{7}{9}$

= $\frac{9}{9}$

= 1

Hence, the answer is the option (3).