Clock: Meaning, Reasoning Questions and Answers, Formula

Introduction to Clock Reasoning

Clock reasoning is one of the most frequently asked topics in logical reasoning and aptitude exams. It involves solving problems based on the position of the hour hand and minute hand of a clock. With the right clock reasoning formula and practice, students can easily solve these questions within seconds. Below, we explore why this topic is important and how it is applied in competitive exams.

Why Clock Reasoning is Important in Competitive Exams

Many exams such as SSC, Banking, Railways, and CAT include clock reasoning questions to test a candidate’s logical thinking and accuracy. These questions may look simple, but they require quick calculations using the clock reasoning formula in aptitude. Mastering this topic not only saves time during the exam but also helps boost the overall reasoning score.

Applications of Clock Reasoning in Logical Reasoning Tests

Clock reasoning questions are widely used to check a candidate’s problem-solving skills in logical reasoning tests. They are applied in scenarios like finding the angle between clock hands, identifying the mirror image of a clock, and calculating gain or loss of time in faulty clocks. These applications make it a vital section in clock reasoning mock tests and practice sets for government exams.

Basic Concepts of Clock Reasoning

Before applying shortcuts and formulas, it is important to understand the basic structure of a clock. Learning these concepts helps in solving clock reasoning questions and answers pdf without confusion.

Types of Angles in Clock Reasoning (Acute, Obtuse, Straight)

In clock reasoning, questions often involve identifying the types of angles formed by clock hands.

• An acute angle is less than 90°.

• An obtuse angle is more than 90° but less than 180°.

• A straight angle is exactly 180°.

These angle-based problems are common in clock reasoning questions for SSC and Banking exams, making it essential to remember their properties.

Structure of a Clock

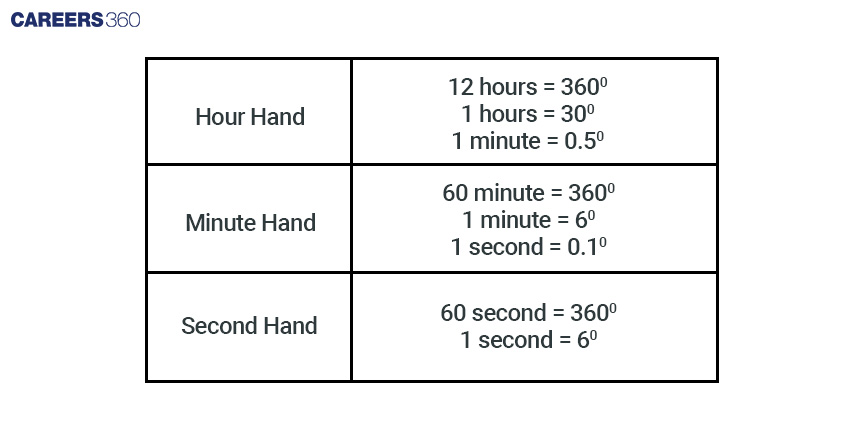
A clock has three hands, i.e. an hour hand, a minute hand, and a second hand. All three hands of a clock move simultaneously to indicate the time. A clock is a complete circle of 360° and there are a total of 12 equal divisions. From this, it is clear that 12 hours is equal to 360°. Similarly, 60 minutes is equal to 360°. Also, 60 seconds is equal to 360°. Also, the angle between any consecutive division is (360° ÷ 12 = 30°). This means 1 hour is equal to 30°. If 1 hour is equal to 30°, then 1 minute will be equal to (30° ÷ 60 = 0.5°). Similarly, we can calculate this for seconds as well.

A clock is a circle of 360 degrees, divided into 12 equal divisions. Each hour represents 30 degrees, and each minute represents 6 degrees. The key terms in clock in reasoning include the hour hand, minute hand, second hand, angle between hands, and overlapping positions. Knowing these basics makes the application of formulas much easier.
The following table shows all the necessary angular measurements in the clock -

Types of Questions Based on Clock Reasoning

The types of questions asked about the clock are as follows -

1. Angle Between the hands of a clock

2. Defective Clock

3. Image-Based Questions

Let’s understand all of the types of clock-based questions with the help of the examples -

1. Angle Between the Hands of a Clock

In this type of question, we need to determine the angle between the hour hand and the minute hand of a clock for a specific time. To tackle these types of questions, we must have the basic knowledge of the angles traced by different hands of the clock. To find the angle between the hands of a clock, we can use the following clock reasoning angle formula.

The angle between the hands of a clock = (30 × Hours) − (5.5 × Minutes)

Example: Determine the angle between the hour and minute hands of the clock at 7:30.

By using the above formula, here, hours = 7 and minutes = 30

So, Angle = (30 × 7) − (5.5 × 30) = 210 − 165 = 45°

In this type, the reverse case is also possible, i.e., to find the time when the angle is known. For this, we have another clock reasoning formula.

Time = 211 [(Hours × 30) ± Angle]

If the time is between the first half (12 to 6), then the sign will be + (plus), and if the time is in between the second half (6 to 12), then the sign will be - (minus).

Example: At what time between 3 and 4 o’clock, the hands make an angle of 10°.

Here, both 3 and 4 lie in the first half, so consider the + sign.

Time = 211 [(3 × 30) + 10] = 211 [90 + 10] = 211 × 100 = 18211

So, the hands of the clock will make an angle of 10° at exactly 3 o’clock 18 minutes 10.9 seconds or 18211minutes past 3 o’clock.

2. Defective Clock

In this type, there is a comparison of time between an accurate clock and a defective clock. The defective clock indicates that the time in the clock is either slow or fast compared to the actual time. The wrong time can either be fast or delayed by a few seconds/minutes/hours or a few days/weeks. Let’s understand the concept with the help of an example.

Example: A watch gained 10 seconds in 5 minutes and was set right at 11 AM. What time will it show at 11 PM on the same day?

The watch gains 10 seconds in 5 minutes. So, in 60 minutes or 1 hour, it will gain 120 seconds. From 11 AM to 11 PM, the total time is 12 hours.

Thus, in 12 hours, it will gain 1440 seconds or 24 minutes.

So, when the actual time is 11 PM, the watch will show 11:24 PM.

3. Image-Based Clock Questions

In this type, the problems will be based on a clock’s mirror image or water image. These questions can be solved by either using the figures that show the mirror or the water image as directed in the question or by the formula. But out of the two methods, the best method is to use the formula.

a) Mirror Image-Based

When the time is given in 12-hour clock format, directly the formula that is given below.

Time in mirror image = 11:60 - Original Time

When the time is given in 24-hour clock format, the first step is to convert the time to 12-hour clock format and then use the above formula.

Example: If it is 3:50 in the clock, then what will be the time in the mirror?

Time in mirror image = 11:60 - Original Time = 11:60 - 3:50 = 8:10

Example: If it is 15:50 on the clock, then what will be the time in the mirror?

First, convert 15:50 to 12-hour clock format, the time will be 3:50

b) Water Image-Based

Time in water image = 18:30 - Original Time (when the minute is less than 30)

Time in water image = 17:90 - Original Time (when the minute is more than 30)

Example: If it is 2:40 on the clock, then what will be the time in the water?

Time in water image = 17:90 - Original Time = 17:90 - 2:40 = 15:50

The time will be 15:50 or 3 hours 50 minutes.

Practice Resources on Clocks

The following are the recommended sources for the practice of the questions of the clock -

a) A Modern Approach to Verbal & Non-Verbal Reasoning by R.S. Aggarwal

b) Analytical Reasoning by M.K. Pandey

c) Logical and Analytical Reasoning by A.K. Gupta

d) Test of Reasoning by Edgar Thorpe

e) For more practice, you can solve online clock reasoning MCQs or clock reasoning questions and answers pdf, clock reasoning questions pdf, and clock reasoning pdf available online to ace the topic clock.

Question Weightage of Clock Reasoning in Competitive Exams

In past years, a good number of questions were seen on this topic, but nowadays in various competitive exams like SSC, Railways, and CUET, an aspirant can hardly expect 1-2 questions from this topic.

Verbal Reasoning Topics

Verbal reasoning is a core part of logical reasoning where questions are framed in words, statements, and numbers to test a candidate’s analytical and problem-solving ability. It checks how well you can interpret given information, draw conclusions, and apply reasoning skills in real-time scenarios. This section is highly important for SSC, Banking, Railway, CAT, UPSC, and other competitive exams. Below are the major verbal reasoning topics you should practice for exam success.

Clock Reasoning Questions for the Angle Between the Hands of a Clock

Q1. What will be the angle between two needles of a clock at 5:15?

A) 60°

B) 67.5° (correct)

C) 69°

D) 75°

Solution:
Given:
Hours = 5 and Minutes = 15

The angle between the hands of a clock = (30 × Hours) − (5.5 × Minutes)

So, Angle = (30 × 5) − (5.5 × 15) = 150 − 82.5 = 67.5°
Therefore, the angle between the hour hand and the minute hand at 5:15 is 67.5°. Hence, the second option is correct.

Q2. What will be the angle between the hour hand and the minute hand, if the clock shows 11:30?

A) 175°

B) 165° (correct)

C) 150°

D) 120°

Solution:
Given:
Hours = 11 and Minutes = 30

The angle between the hands of a clock = (30 × Hours) − (5.5 × Minutes)

So, Angle = (30 × 11) − (5.5 × 30) = 330 − 165 = 165°
Therefore, the angle between the hour hand and the minute hand at 11:30 is 165°. Hence, the second option is correct.

Q3. What will be the angle between the hour hand and the minute hand, if the clock shows 16:30?

A) 125°

B) 300°

C) 225°

D) 315°

Solution:
Given:
Hours = 16 and Minutes = 30

The angle between the hands of a clock = (30 × Hours) − (5.5 × Minutes)

So, Angle = (30 × 16) − (5.5 × 30) = 480 − 165 = 315°
Therefore, the angle between the hour hand and the minute hand at 16:30 is 315°. Hence, the fourth option is correct.

Q4. At what time between 4 and 5 o’clock, the hands make an angle of 45°.

A) 4:30

B) 3:30 (Correct)

C) 3:15

D) 3:45

Solution:

To calculate the time when the angle is given, use the following formula.

Time = 211 [(Hours × 30) ± Angle]

Here, both 4 and 5 lie in the first half, so consider the + sign.

Time = 211 [(4 × 30) + 45] = 211 [120 + 45] = 211 × 165 = 30

So, the hands of the clock will make an angle of 45° at exactly, 3:30. Hence, the second option is correct.

Q5. At what time between 9 and 10 o’clock, the hands make an angle of 50°.

A) 9:40 (Correct)

B) 9:20

C) 10:45

D) 9:50

Solution:

To calculate the time when the angle is given, use the following formula.

Time = 211 [(Hours × 30) ± Angle]

Here, both 9 and 10 lie in the second half, so consider the (-) sign.

Time = 211 [(9 × 30) - 50] = 211 [270 - 50] = 211 × 220 = 40

So, the hands of the clock will make an angle of 50° at exactly, 9:40. Hence, the first option is correct.

Clock Reasoning Questions for Defective Clock

Q1. A watch gained 5 seconds in 3 minutes and was set right at 9 AM. What time will it show at 9 PM on the same day?

A) 9:50

B) 10:20

C) 8:40

D) 9:20 (Correct)

Solution: The watch gains 5 seconds in 3 minutes. So, in 60 minutes or 1 hour, it will gain 100 seconds.

From 9 AM to 9 PM, the total time is 12 hours.

Thus, in 12 hours, it will gain 1200 seconds or 20 minutes.

So, when the actual time is 9 PM, the watch will show 9:20 PM. Hence, the fourth option is correct.

Q2. The clock was set on Monday at 5 AM. If the clock gains 30 minutes per hour, then what will be the time that the clock shows on Wednesday, 5 PM?

A) 11 PM, Friday

B) 11 PM, Thursday

C) 11 AM, Friday (Correct)

D) 11:30 PM, Thursday

Solution: The clock was set on Monday at 5 AM.

So, from Monday, 5 AM to Wednesday, 5 PM, the total time is 60 hours. Now, according to the given statement, the clock gains 30 minutes per hour. So, in total, the clock will gain 1800 minutes or 30 hours.

So, the clock will show the time 5 PM + 30 hours = 11 PM of Thursday on Wednesday, 5 PM.

Hence, the third option is correct.

Q3. An office has two wall clocks, one in the meeting room and the other in the boss’s cabin. The time displayed on both the clocks is 12 AM right now. The clock in the cabin gains 5 minutes every hour, whereas the one in the meeting room is slower by 5 minutes every hour. When will both the watches show at the same time again?

A) 72 hours (Correct)

B) 70 hours

C) 48 hours

D) 24 hours

Solution: The faster clock runs 5 minutes faster in 1 hour, and the slower clock runs 5 minutes slower in 1 hour.

Therefore, in 1 hour, the faster clock will trace 5 + 5 = 10 minutes more when compared to the slower clock. The following table shows the time difference between both the clocks.

From the above table, it is clear that in 6 hours, the faster clock will trace 60 minutes more when compared to the slower clock.

In 72 hours, the faster clock determines 12 hours more than the slower clock. At this point, both the clocks will show the same time, i.e., both the clocks will show the same time after exactly 72 hours.

Hence, the first option is correct.

Q4. The clock was set at 10 AM. If the clock gains 2 minutes per hour, then what will be the time that the clock shows at 11 PM on the same day?

A) 10:06 PM

B) 11:06 PM

C) 10:26 PM

D) 11:26 PM (Correct)

Solution: The watch gains 2 minutes per hour.

From 10 AM to 11 PM, the total time is 13 hours.

Thus, in 13 hours, it will gain 26 minutes.

So, when the actual time is 11 PM, the watch will show 11:26 PM. Hence, the fourth option is correct.

Q5. The clock was set at 1 PM. If the clock loses 30 seconds for every 5 minutes, then what will be the time that the clock shows at 9 PM on the same day?

A) 10:48 PM

B) 10:00 PM

C) 8: 48 PM

D) 9:48 PM (Correct)

Solution: The watch loses 30 seconds in 5 minutes. So, in 60 minutes or 1 hour, it will lose 360 seconds.

From 1 PM to 9 PM, the total time is 8 hours.

Thus, in 8 hours, it will lose 2880 seconds or 48 minutes.

So, when the actual time is 9 PM, the watch will show 9:48 PM. Hence, the fourth option is correct.

Clock Reasoning Questions for Image-Based Clock Questions

Q1. A clock shows 3:10 hours. What will be the time if it is seen in the mirror?

A) 6:10

B) 5:20

C) 8:50 (Correct)

D) 3:10

Solution: Time in mirror image = 11:60 - Original Time = 11:60 - 3:10 = 8:50

Hence, the third option is correct.

Q2. A clock shows 18:20 hours. What will be the time if it is seen in the mirror?

A) 4:40

B) 5:40 (Correct)

C) 8:10

D) 5:25

Solution: Time given = 18:20, since the given time is in 24-hour clock format. So, convert it to a 12-hour clock format. So, 18:20 → 6:20

Time in mirror image = 11:60 - Original Time = 11:60 - 6:20 = 5:40

Hence, the second option is correct.

Q3. A clock shows 5:10 hours. What will be the time if it is seen in the water?

A) 9:10

B) 5:20

C) 1:20 (Correct)

D) 3:10

Solution: Given time = 5:10
Since the minute is less than 30

Time in water image = 18:30 - Original Time = 18:30 - 5:10 = 13:20 or 1:20

Hence, the third option is correct.

Q4. If the water image of the clock shows 3:25, then what will be the actual time?

A) 3:25 (Correct)

B) 2:25

C) 5:50

D) 10:10

Solution: Since the minute is less than 30

Original Time = 18:30 - Time in water image = 18:30 - 3:25 = 15:05 or 3:25

Hence, the first option is correct.

Q5. If the mirror image of the clock shows 10:20, then what will be the actual time?

A) 7:50

B) 1:40 (Correct)

C) 6:20

D) 10:40

Solution: Original Time = 11:60 - Time in mirror image = 11:60 - 10:20 = 01:40

Hence, the second option is correct.

Clock Reasoning Questions for CAT/ APICET/ JIPMAT

Generally, 1 question of clock reasoning in the CAT exam and 2-3 questions in APICET and JIPMAT are seen in the exam.

Q1. The time in a clock is 20 minutes past 2. Find the angle between the hands of the clock.

1. 60 degrees

2. 120 degrees

3. 45 degrees

4. 50 degrees

Solution:

Angle =11/2m-30h

⇒ Angle = 11x 20/2 – 30 x 2= 110 -60 = 50

Hence, the fourth option is correct.

Q2. A clock loses 1% time during the first week and then gains 2% time during the next week. If the clock was set right at 12 noon on a Sunday, what would be the time that the clock would show exactly 14 days from when it was set right?[CAT 2016]

1. 1: 36: 48

2. 1: 40: 48

3. 1: 41: 24

4. 10: 19: 12

Solution:

One week has 7 * 24 = 168 hours.

If the clock loses 1% time during the first week, then it will show a time of 1% less than 168 hours = 1.68 hours less.

Subsequently, in the second week, it gains 3.36 hours more than the actual time.

As it lost 1.68 hours during the first week and then gained 3.36 hours the next week, the net gain = 1.68 hours.

So the clock will show a time which is 1.68 hours more than 12 Noon two weeks after the given time.

1.68 hours = 1 hour and 40.8 minutes = 1 hour + 40 minutes + 48 seconds.

i.e. 1: 40: 48 P.M. Hence, the second option is correct.

Clock Reasoning Questions for VITEEE/ CUET

Generally, 1-2 questions of the clock have been seen in the VITEEE and CUET exams.

Q-1) Directions: The image of a clock in a mirror is seen as 3:15. What is the right time?

1) 8:45

2) 10:45

3) 7:45

4) 9:45

Hint: Subtract the reflected time from 11:60 to get the actual time.

Solution:

Because the time 3:15 lies between 1:00 and 11:00, so to get the actual time subtract the reflected time from 11:60.

Actual time = 11:60 – 3:15 = 8:45

So, 8:45 is the right time. Hence, the first option is correct.

Common Mistakes to Avoid in Clock Reasoning

Even after learning the clock reasoning formulas and tricks, many candidates lose marks because of small errors. Understanding these common mistakes in clock reasoning questions can help students avoid confusion and solve problems with accuracy in exams like SSC, Banking, Railways, and CAT.

Misinterpreting AM and PM in Questions

One of the most frequent errors is misreading the time format in clock reasoning questions. Many aptitude tests give time in AM/PM format, but candidates often assume it is always a 12-hour format. This mistake can lead to wrong answers in angle between clock hands questions or overlapping problems. Always check whether the clock is shown in 12-hour or 24-hour format before solving.

Forgetting to Apply the Correct Formula

Another common error is forgetting or misusing the clock reasoning formula. Candidates sometimes calculate the angle between hour and minute hands using basic subtraction instead of applying the formula:

Angle = |(30 × Hour) – (11/2 × Minutes)|

Skipping this step or using incorrect substitution can easily result in errors, especially in clock reasoning aptitude tests where time is limited. Regular practice with clock reasoning mock test helps in avoiding such mistakes.

Confusing Mirror Image and Water Image Problems

Mirror image and water image questions in clock reasoning often confuse candidates. In mirror image of a clock, the reflection appears as if viewed in a mirror placed vertically, while in water image, the reflection is flipped as if seen on water below. Many students interchange these concepts and lose marks in mirror image clock reasoning questions for SSC exams. Practicing with examples of both types ensures clarity during the exam.

Non Verbal Reasoning Topics

Non-verbal reasoning is an important part of logical reasoning where problems are solved using diagrams, shapes, and figures instead of words or numbers. It tests a candidate’s ability to analyze patterns, visualize changes, and apply logic without relying on language skills. This section plays a vital role in exams like SSC, Banking, Railway, CAT, and other aptitude tests. Below are the major non-verbal reasoning topics you need to prepare for competitive exams.

About the Faculty
Tanu Gupta, with over a decade of experience as a reasoning faculty, specializes in preparing students for various entrance examinations and career development. Her extensive work with multiple educational platforms and institutions has honed her expertise in logical and analytical thinking. Her dedication to innovative teaching methods ensures these articles provide practical insights and expert guidance.