Addition and Subtraction of Algebraic Expressions

Addition And Subtraction Of Algebraic Expression

Edited By Team Careers360 | Updated on Jul 02, 2025 05:17 PM IST

Algebraic expression addition and subtraction are slightly more complicated than natural number operations. In order to learn the addition and subtraction of algebraic expressions, first we have to understand the algebraic expression. An algebraic expression is just an equation containing a combination of constants and variables.

This Story also Contains

Algebraic Expressions Addition And Subtraction
Horizontal Addition
Vertical Addition
Horizontal Subtraction
Vertical Subtraction

Let us take an example of algebraic expression: suppose your teacher asked you to convert the statement “Marks obtained by student A of class 10th is 10 marks more than the marks obtained by student B of 10th “ into mathematical form.

Now to express it in mathematical form suppose marks obtained by A are x and marks obtained by B are y now according to the statement x is 10 more than y.

In mathematical form, we write the above statement as

x=y+10

This expression is known as an Algebraic expression.

Algebraic Expressions Addition And Subtraction

In mathematics addition, subtraction and other mathematical operations are easily applicable to natural numbers. If we talk about mathematical operations on algebraic expressions it is not the same as in natural numbers. While applying addition and subtraction to algebraic expressions we must keep in mind that only terms which are having the same variable and exponent will be added or subtracted. We have to follow some steps for applying mathematical operations to an algebraic expression.

Methods of addition and subtraction are:

Horizontal
Vertical

Horizontal Addition

Steps in addition

Write each algebraic expression within a bracket in a single row with a plus sign in between them.
Now open all the brackets.
Write terms having the same variable and exponent with the appropriate sign in a bracket.
Add the coefficient of all the terms having the same variable and exponent.

To understand the above steps let us take an example of algebraic addition

First expression- \[2x + 3y + 1\]

Second expression- \[10x + 13y + 5\]

Follow above steps

\[(2x + 3y + 1) + (10x + 13y + 5)\]
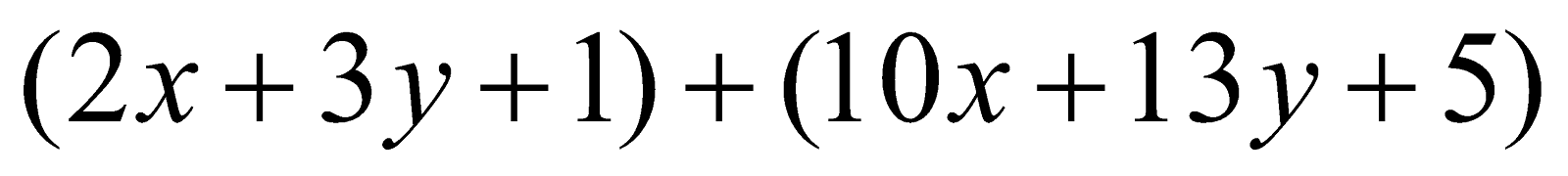
\[2x + 3y + 1 + 10x + 13y + 5\]
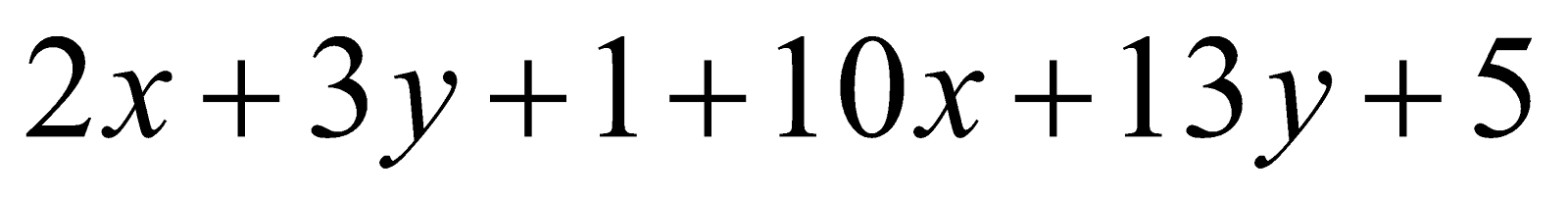
\[(2x + 10x) + (3y + 13y) + (1 + 6)\]
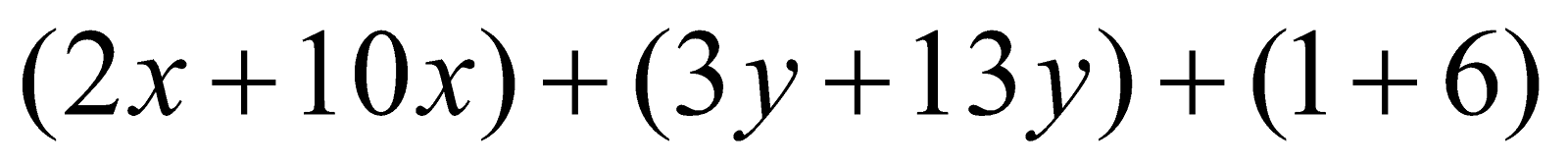
\[12x + 16y + 6\]

NEET Highest Scoring Chapters & Topics

This ebook serves as a valuable study guide for NEET exams, specifically designed to assist students in light of recent changes and the removal of certain topics from the NEET exam.

Download E-book

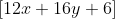
Vertical Addition

In this method, we have to write each expression in a different row. While writing expressions in different rows keep in mind that the terms having the same variables and exponent must be written one below the others along with their sign. Now we have to add all coefficients present in a particular column to get the value of the addition.

To understand the above steps let us take an example of algebraic addition

First expression- \[2x + 3y + 1\]

Second expression-\[10x + 13y + 5\]

Now add these two by using the vertical method

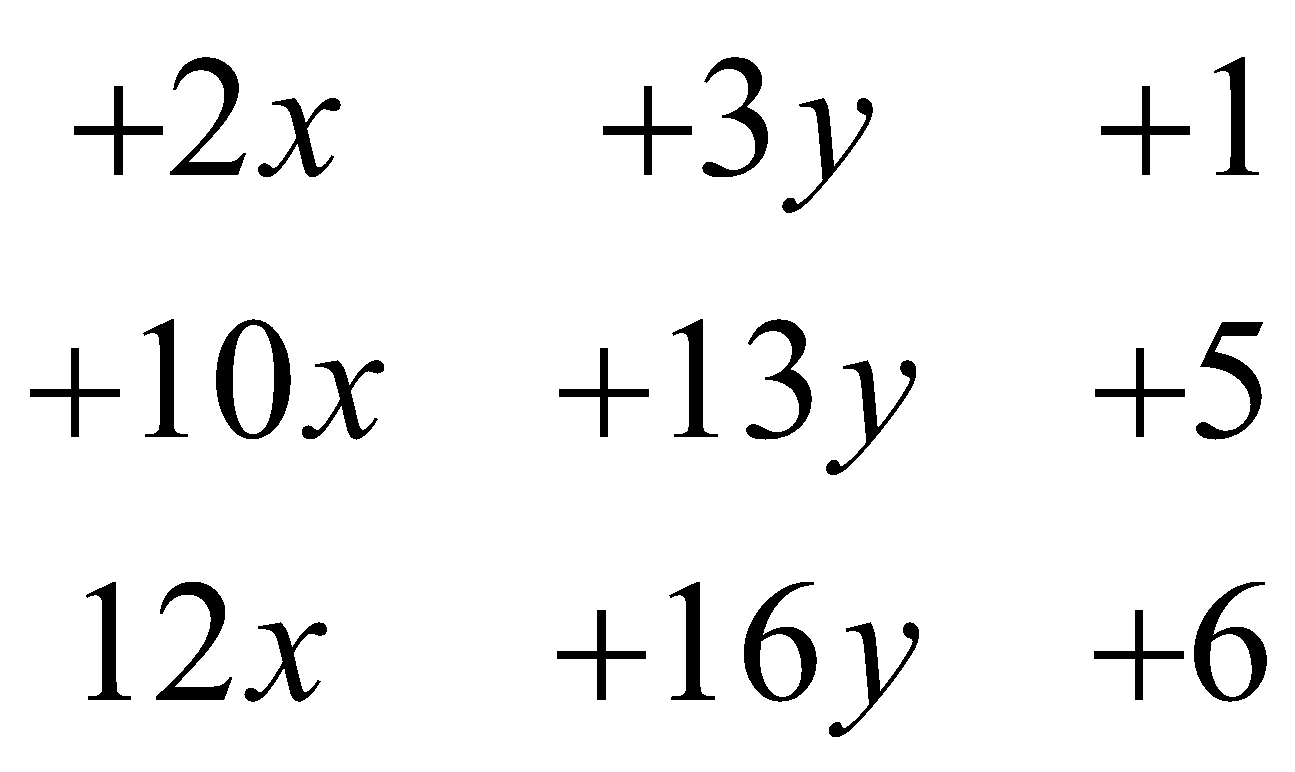
1706458074034

\begin{array}{ccc}

+2 x & +3 y & +1 \\

+10 x & +13 y & +5 \\

12 x & +16 y & +6

\end{array}

Horizontal Subtraction

Steps in subtraction

Write each algebraic expression within a bracket in a single row with a minus sign in between them.
Now open all brackets and apply the algebra of the sign of a term.
Write terms having the same variable and exponent with the appropriate sign in a bracket.
Subtract the coefficient of all the terms having the same variable and exponent.

To understand the above steps let us take an example of algebraic addition

First expression-

Second expression-

Now subtract first from second by using the horizontal method

Follow above steps

First expression- \[2x + 3y + 1\]

Second expression- \[10x + 13y + 5\]

Follow above steps

\[(2x + 3y + 1) - (10x + 13y + 5)\]
\[2x + 3y + 1 - 10x - 13y - 5\]
\[(2x - 10x) + (3y - 13y) + (1 - 5)\]
\[ - 8x - 10y - 4\]

Vertical Subtraction

In this method, we have to write each expression in a different row. While writing expressions in different rows keep in mind that the terms having the same variables and exponent must be written one below the others along with their sign. Now we have to subtract all coefficients present in a particular column to get the value of the addition.

Let us take an example to understand it

First expression-

Second expression-

Now subtract first from second by using the vertical method

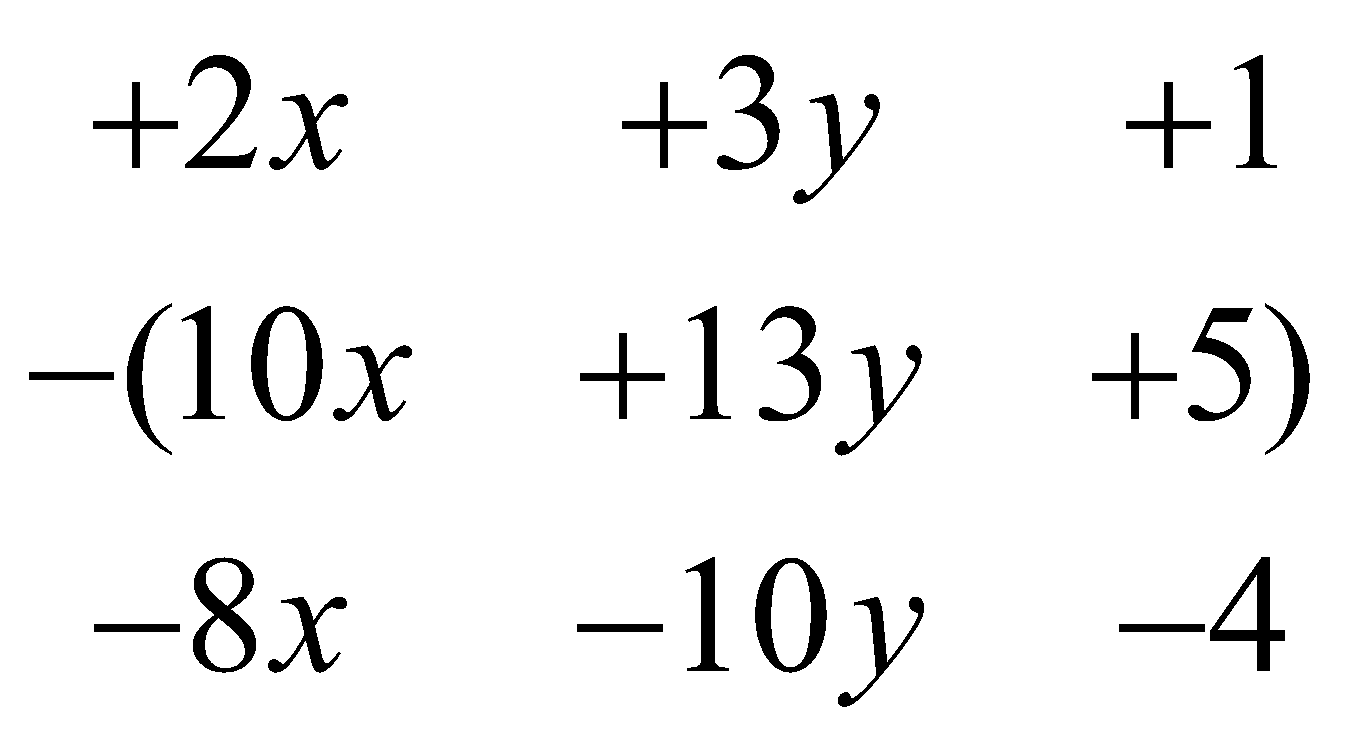
1706458077178

First expression- \[2x + 3y + 1\]

Second expression- \[10x + 13y + 5\]

Now subtract first from second by using vertical method

\begin{array}{ccc}

+2 x & +3 y & +1 \\

-(10 x & +13 y & +5) \\

-8 x & -10 y & -4

\end{array}

Frequently Asked Questions (FAQs)

1. Explain Algebraic Expression.

An algebraic expression is just an equation containing a combination of constants and variables

2. Explain the addition.

The process of joining two small numbers in mathematics and creating one larger number is known as an addition.

3. Explain the Subtraction.

Subtraction is the mathematical operation of taking a larger number and reducing it to a smaller one.

4. Give each step which should follow in the Subtraction of algebraic expression in the horizontal method.

Steps in subtraction

Write each algebraic expression within a bracket in a single row with a minus sign in between them.
Now open all brackets and apply the algebra of the sign of a term.
Write terms having the same variable and exponent with the appropriate sign in a bracket.
Subtract the coefficient of all the terms having the same variable and exponent.

5. Explain every step which must apply in addition of the algebraic expression in the horizontal method.

Steps in addition

Write each algebraic expression within a bracket in a single row with a plus sign in between them.
Now open all the brackets.
Write terms having the same variable and exponent with the appropriate sign in a bracket.
Add the coefficient of all the terms having the same variable and exponent.

6. Give an example of the Addition of algebraic expression in the horizontal method.

First expression-

Second expression-

Follow above steps

First expression- \[2x + 3y + 1\]

Second expression- \[10x + 13y + 5\]

Follow above steps

\[(2x + 3y + 1) + (10x + 13y + 5)\]
\[2x + 3y + 1 + 10x + 13y + 5\]
\[(2x + 10x) + (3y + 13y) + (1 + 6)\]
\[12x + 16y + 7\]

7. Give an example of Subtraction of algebraic expression in a horizontal method.

First expression-

Second expression-

Now subtract first from second by using the horizontal method

Follow above steps

First expression- \[2x + 3y + 1\]

Second expression- \[10x + 13y + 5\]

Follow above steps

\[(2x + 3y + 1) - (10x + 13y + 5)\]
\[2x + 3y + 1 - 10x - 13y - 5\]
\[(2x - 10x) + (3y - 13y) + (1 - 5)\]
\[ - 8x - 10y - 4\]

8. What is an algebraic expression?

An algebraic expression is a combination of variables, numbers, and mathematical operations. It can include letters representing unknown values, constants, and symbols for addition, subtraction, multiplication, or division. For example, 3x + 2y - 5 is an algebraic expression.

9. How do you identify like terms in algebraic expressions?

Like terms are terms that have the same variables raised to the same powers. To identify like terms, look for terms with identical variable parts. For example, in the expression 3x + 2y + 5x - 4, 3x and 5x are like terms because they both have x to the first power.

10. Why can't we add or subtract terms with different variables?

We can't add or subtract terms with different variables because they represent different quantities. It's similar to how we can't add apples and oranges directly. We can only combine like terms, which have the same variable parts.

11. How do you simplify an expression like 3x + 2y - 4x + 5y?

To simplify this expression, combine like terms: group terms with x and terms with y. 3x + 2y - 4x + 5y = (3x - 4x) + (2y + 5y) = -x + 7y.

12. What is the zero principle in algebra, and how does it relate to addition and subtraction?

The zero principle states that adding or subtracting zero from any quantity doesn't change its value. In algebra, this means that terms like +0x or -0y can be added or removed without affecting the expression's value. This principle is often used in simplification.

13. Why is the order of terms not important in addition of algebraic expressions?

The order of terms doesn't matter in addition because of the commutative property of addition. This property states that changing the order of addends doesn't change the sum. For example, a + b = b + a, so 3x + 2y is equivalent to 2y + 3x.

14. What is the importance of the associative property in algebraic addition?

The associative property states that the grouping of addends doesn't affect the sum. This allows us to rearrange terms in complex additions. For example, (a + b) + c = a + (b + c). This property is crucial when simplifying long algebraic expressions.

15. How do you handle expressions with mixed numbers as coefficients?

Convert mixed numbers to improper fractions before performing operations. For example, 2½x + 3¾x = (5/2)x + (15/4)x = (10/4)x + (15/4)x = (25/4)x = 6¼x.

16. What is the concept of "combining like radicals" in algebraic addition and subtraction?

Like radicals are terms with the same root and index. When adding or subtracting, combine the coefficients of like radicals. For example, 2√3 + 5√3 = 7√3, but 2√2 + 3√3 cannot be combined further.

17. How do you handle addition and subtraction of algebraic expressions with different units?

Terms with different units cannot be combined directly. Keep them separate in the final expression. For example, 3x meters + 2y centimeters cannot be simplified further unless a conversion between meters and centimeters is specified.

18. How do you add or subtract polynomials?

To add or subtract polynomials, align like terms and then combine them. For example, (3x² + 2x - 1) + (x² - 3x + 4) = 4x² - x + 3. Remember to keep the operation sign with each term when subtracting.

19. How do you deal with expressions containing exponents when adding or subtracting?

When adding or subtracting terms with exponents, only combine like terms (terms with the same variable and exponent). For example, x² + 3x - 2x² + 5x = -x² + 8x. You cannot combine x² and x as they are not like terms.

20. What is the role of the additive inverse in algebraic subtraction?

The additive inverse of a term is the negative of that term. In algebraic subtraction, we often use the principle that subtracting a term is the same as adding its additive inverse. For example, x - y is the same as x + (-y).

21. How do you simplify expressions with multiple variables like 2a + 3b - 4a + 5b?

Group like terms together and combine their coefficients. In this case, (2a - 4a) + (3b + 5b) = -2a + 8b. Remember, terms with different variables cannot be combined.

22. How do you handle expressions with fractional coefficients in addition and subtraction?

Treat fractional coefficients like any other coefficient. When adding or subtracting, combine like terms by adding or subtracting the fractional coefficients. For example, (1/2)x + (1/3)x = (5/6)x.

23. How do you add algebraic expressions?

To add algebraic expressions, combine like terms by adding their coefficients and keeping the variable part the same. For example, to add 3x + 2y and 4x - y, we get (3x + 4x) + (2y - y) = 7x + y.

24. How do you add or subtract algebraic expressions with literal coefficients?

Literal coefficients are letters representing unknown numbers. Treat them like any other coefficient, combining like terms. For example, ax + bx = (a + b)x, where a and b are literal coefficients.

25. What is the concept of "collecting like terms" in algebraic addition and subtraction?

Collecting like terms means combining terms with the same variables and exponents by adding or subtracting their coefficients. This process simplifies expressions. For example, in 2x + 3y + 5x - 2y, collecting like terms gives us 7x + y.

26. How do you add or subtract algebraic expressions with different powers of the same variable?

Terms with different powers of the same variable are not like terms and cannot be combined. For example, x² + 2x - 3x² = -2x² + 2x. The x² terms can be combined, but the x term remains separate.

27. What is the role of the number line in understanding addition and subtraction of algebraic expressions?

The number line can help visualize addition and subtraction of algebraic expressions, especially with positive and negative terms. Moving right represents addition, while moving left represents subtraction, similar to arithmetic operations with integers.

28. What common mistake do students make when subtracting expressions with parentheses?

A common mistake is forgetting to change the signs of all terms inside the parentheses when subtracting. For example, in 5x - (2x + 3), students might incorrectly write 5x - 2x + 3 instead of the correct 5x - 2x - 3.

29. How do you handle negative coefficients when adding or subtracting algebraic expressions?

Treat negative coefficients as you would negative numbers in arithmetic. When adding, keep the sign. When subtracting, change the sign. For example, 3x + (-2x) = x, and 3x - (-2x) = 3x + 2x = 5x.

30. How do you add or subtract algebraic fractions?

To add or subtract algebraic fractions, find a common denominator, then add or subtract the numerators while keeping the common denominator. For example, (x/2) + (y/3) = (3x/6) + (2y/6) = (3x + 2y)/6.

31. How does subtracting an algebraic expression differ from adding its negative?

Subtracting an algebraic expression is equivalent to adding its negative. For example, subtracting (3x - 2) is the same as adding (-3x + 2). This principle is based on the fact that subtracting a positive number is the same as adding a negative number.

32. What is the concept of "cancelling out" in algebraic addition and subtraction?

"Cancelling out" occurs when adding or subtracting terms results in a coefficient of zero, effectively eliminating that term. For example, in 5x - 2y + 3x - 5x, the x terms cancel out: (5x + 3x - 5x) - 2y = 3x - 2y.

33. What is the difference between coefficients and constants in algebraic expressions?

Coefficients are the numerical factors of terms containing variables, while constants are terms without variables. For example, in the expression 5x + 3y - 7, 5 and 3 are coefficients, and -7 is a constant.

34. What is the role of parentheses in algebraic expressions?

Parentheses in algebraic expressions group terms together and indicate that the operations inside should be performed first. They can also be used to distribute a factor to multiple terms. For example, 2(x + 3) means 2 is multiplied by both x and 3.

35. How do you simplify expressions with nested parentheses?

Work from the innermost parentheses outward, simplifying each set of parentheses before moving to the next. For example, in 2(3(x + 2) - 4), first simplify (x + 2), then multiply by 3, subtract 4, and finally multiply by 2.

36. What is the difference between combining like terms and factoring in algebraic expressions?

Combining like terms involves adding or subtracting coefficients of terms with the same variables and exponents. Factoring, on the other hand, involves finding a common factor among terms and expressing the polynomial as a product. For example, 3x + 6 can be factored as 3(x + 2).

37. What is the role of the distributive property in simplifying expressions like a(b + c) - d(e - f)?

The distributive property allows us to multiply each term inside the parentheses by the factor outside. In this case, a(b + c) - d(e - f) = ab + ac - de + df. This property is crucial for expanding and simplifying complex algebraic expressions.

38. What is the concept of "algebraic long division" and how does it relate to addition and subtraction?

Algebraic long division is a method for dividing polynomials. While it primarily involves division, addition and subtraction are crucial steps in the process.

39. What is the distributive property, and how is it used in algebraic addition and subtraction?

The distributive property allows us to multiply a factor by a sum or difference by distributing it to each term. For example, 2(x + y) = 2x + 2y. This property is useful when adding or subtracting expressions with parentheses.

40. What is the difference between monomial, binomial, and trinomial in the context of addition and subtraction?

A monomial is a single term (e.g., 3x), a binomial has two terms (e.g., 3x + 2), and a trinomial has three terms (e.g., 3x + 2y - 1). When adding or subtracting these, the result may have a different number of terms depending on whether like terms can be combined.

41. What is the importance of maintaining the equality of an equation when adding or subtracting on both sides?

When adding or subtracting the same quantity from both sides of an equation, we maintain the balance or equality. This principle is crucial in solving equations. For example, if x + 3 = 7, we can subtract 3 from both sides to isolate x: x + 3 - 3 = 7 - 3, so x = 4.

42. What is the concept of "terms" in algebraic expressions?

Terms are parts of an algebraic expression separated by addition or subtraction signs. Each term can contain numbers, variables, or both. For example, in 3x² - 2xy + 5, there are three terms: 3x², -2xy, and 5.

43. How do you add or subtract algebraic expressions involving absolute values?

Treat absolute value expressions as you would any other term, but remember that the result inside the absolute value bars is always non-negative. For example, |x| + |y| cannot be simplified further unless you know more about x and y.

44. What is the concept of "zero pairs" in algebraic addition and subtraction?

Zero pairs are terms that add up to zero, such as x and -x. Identifying and cancelling zero pairs can simplify expressions. For example, in 3x + 2y - x + 4, x and -x form a zero pair, leaving 2x + 2y + 4.

45. How do you add or subtract algebraic expressions with scientific notation?

When adding or subtracting expressions in scientific notation, adjust the exponents to match, then add or subtract the coefficients. For example, 3 × 10² + 4 × 10³ = 0.3 × 10³ + 4 × 10³ = 4.3 × 10³.

46. What is the role of the associative property in simplifying complex algebraic additions?

The associative property allows us to group terms in different ways without changing the result. This is useful in simplifying complex expressions. For example, (a + b) + c = a + (b + c), which can make calculations easier depending on the values involved.

47. How do you handle addition and subtraction of algebraic expressions with imaginary numbers?

Treat imaginary numbers like any other term, but keep the imaginary unit i separate. For example, (3 + 2i) + (4 - 5i) = 7 - 3i. Remember that i² = -1, but don't combine i terms with real terms.

48. What is the concept of "like bases" in exponential expressions, and how does it relate to addition and subtraction?

Terms with like bases but different exponents cannot be combined through addition or subtraction. For example, 2³ + 2⁴ cannot be simplified further. However, if the exponents are the same, you can add or subtract the coefficients: 3 × 2³ + 2 × 2³ = 5 × 2³.

49. How do you add or subtract algebraic expressions involving logarithms?

Logarithms with the same base and argument can be combined by adding or subtracting their coefficients. For example, 2 log₃(x) - log₃(x) = log₃(x). However, log₃(x) + log₃(y) cannot be combined further using addition or subtraction.

50. What is the importance of order of operations (PEMDAS/BODMAS) in algebraic addition and subtraction?

The order of operations ensures consistent evaluation of expressions. In the context of addition and subtraction, it's crucial to perform operations within parentheses first, then exponents, followed by multiplication and division (from left to right), and finally addition and subtraction (from left to right).

51. How do you handle addition and subtraction of algebraic expressions with different number bases?

Convert all terms to the same base before adding or subtracting. For example, to add a binary number 1010₂ and a decimal number 6₁₀, convert both to the same base: 1010₂ = 10₁₀, so 10₁₀ + 6₁₀ = 16₁₀.

52. What is the concept of "dimensional analysis" in algebraic addition and subtraction?

Dimensional analysis ensures that only quantities with the same dimensions (units) are added or subtracted. For example, you can add lengths to lengths, but not lengths to areas. This principle helps in checking the validity of algebraic operations in physics and engineering problems.

53. How do you add or subtract algebraic expressions involving matrices?

Matrix addition and subtraction are performed element by element, and only matrices of the same dimensions can be added or subtracted. For example, if A = [1 2; 3 4] and B = [5 6; 7 8], then A + B = [6 8; 10 12].

54. How do you handle addition and subtraction of algebraic expressions with different variable representations (e.g., x vs. x(t))?

Variables with different representations are treated as distinct variables. For example, x and x(t) cannot be combined as like terms. The expression 3x + 2x(t) cannot be simplified further unless additional information is provided about the relationship between x and x(t).