Algebra Formulas For Class 8
Algebraic Formulas are algebraic equations which are true for each and every value of the variable in it. The algebraic formulas that are valid for all values of variables in it are called algebraic identities. These identities are used to factorize polynomials and are used to compute algebraic expressions and solve different polynomials.
Algebraic expressions are known as collections of constants and variables connected to one or more operations like addition, subtraction, multiplication, and division. This expression contains monomial, binomial, trinomial and multinomial.Here monomial means having one term, binomial means having two terms, trinomial means having three terms, and multinomial means having more than three terms.
Constants which means a symbol having a fixed value.
Variables are collections of symbols that can give various numerical values.
Factor means quantities multiplied by each other to give a product and that product is known as a factor.
A factor of a non-constant term of an algebraic expression is called the coefficient of the remaining factor of a term. And these coefficients are of two types one is a numerical coefficient and another one is a literal coefficient.
Polynomials are of three types:
Linear polynomials having degree one.
Quadratic polynomial having degree two.
Cubic polynomial having degree three.
Algebraic Formulas
(a + b)^{2} = a^{2} + 2ab + b^{2}
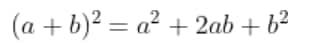
(a - b)^{2} = a^{2} - 2ab + b^{2}
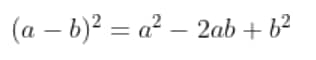
(a+b)(a - b) = a^{2} - b^{2}
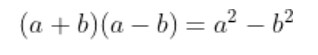
(x+a)(x+b) = x^{2} + (a+b)x + ab
![]()
(x+a)(x-b) = x^{2} + (a-b)x - ab
![]()
(x-a)(x+b) = x^{2} + (b-a)x - ab
![]()
(x-a)(x-b) = x^{2} - (a+b)x + ab
![]()
(a+b)^{3} = a^{3} + b^{3} + 3ab(a+b)
![]()
(a-b)^{3} = a^{3} - b^{3} - 3ab(a-b)
![]()
(a+b+c)^{2} = a^{2} + b^{2} + c^{2} + 2ab + 2bc + 2ac
![]()
(a+b-c)^{2} = a^{2} + b^{2} + c^{2} + 2ab - 2bc - 2ac
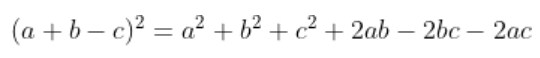
(a-b+c)^{2} = a^{2} + b^{2} + c^{2} - 2ab - 2bc + 2ac
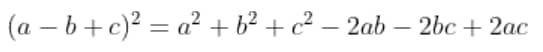
(a-b-c)^{2} = a^{2} + b^{2} + c^{2} - 2ab + 2bc - 2ac
![]()
Examples
(x+2)(x+5)
To solve this we can use a formula called: (x+a)(x+b) = x^{2} + (a+b)x + ab
![]()
Here a = 2 and b = 5
(x+2)(x+5) = x^{2} + (2+5)x + 10
![]()
(x+2)(x+5) = x^{2} + (7)x + 10
![]()
Hence, (x+2)(x+5) equals to x^{2} + (7)x + 10.
(x-3)(x+7)
To solve this we can use a formula called: (x-a)(x+b) = x^{2} + (b-a)x - ab
![]()
Here a = 3 and b = 7
(x-3)(x+7) = x^{2} + (7-3)x - 21
![]()
(x-3)(x+7) = x^{2} + 4x - 21
![]()
Hence, (x-3)(x+7) equals to x^{2} + 4x - 21.
Frequently Asked Questions (FAQs)
They are:
Associative Rule of Multiplication
Associative Rule of Addition.
Commutative Rule of Multiplication.
Commutative Rule of Addition.
Distributive Rule of Multiplication.
The degree of a cubic polynomial is 3.
The difference between a constant and a variable is that a constant has a fixed value while a variable has a value that can be varied.
An Exponent defines how many times a number gets multiplied by itself. For Example, 2^{3} which specifies 2*2*2 = 8.
Algebraic Formulas are the generalized arithmetic equations formed by mathematical symbols and phrases.