Algebra of Limits

The algebra of limits is one of the core topics in learning how to manipulate and combine functions at their limits. An understanding of calculus begins with the mastery of the algebra of limits. This is elementary knowledge to consider changes, optimize processes, and forecast trends in engineering, physics, and economics. It is from this algebra of limits that we can explore the behavior of a function in the vicinity of some particular point, even though, at one specific point, the function remains undefined. This simple idea enables us to solve the most complicated problems related to the real world and equations in mathematics, which include finding derivatives and integrals.

JEE Main: Study Materials | High Scoring Topics | Preparation Guide

JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs

This Story also Contains

1. Limit of a function
2. What is Algebra of Limits?
3. Solved Examples Based On Algebra Of Limits:

In this article, we will cover the concept of the Algebra of Limits. This topic falls under the broader category of Calculus, which is a crucial chapter in Class 11 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of ten questions have been asked on this topic in JEE Main from 2013 to 2023, two questions in 2018 and three in 2019, one in 2021, and four in 2024.

Limit of a function

If $x\to a,f(x)\to l$, then $l$ is called limit of the function $f(x)$ which is symbolically written as $\underset{x\to a}{lim}f(x)=l$.

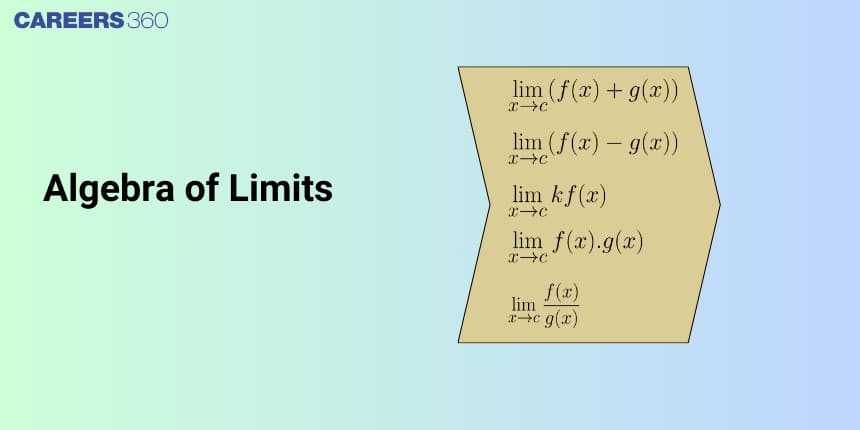
What is Algebra of Limits?

Algebra of limits operates under several rules or properties, making it possible to determine the limits of functions from their algebraic combinations. These rules make finding limits easier and are some of the most important tools within calculus. The basic algebraic operations include addition, subtraction, multiplication, and division of limits.

Let $\mathrm{f}(\mathrm{x})$ and $\mathrm{g}(\mathrm{x})$ be defined for all $x\ne$ $a$ over some open interval containing $a$. Assume that L and M are real numbers such that $x\to a$ then, $\underset{x\to a}{lim}f(x)=L$ and $\underset{x\to a}{lim}g(x)=M$. Then, each of the following statements hold:

Sum law for limits : $\underset{x\to a}{lim}(f(x)+g(x))=\underset{x\to a}{lim}f(x)+\underset{x\to a}{lim}g(x)=L+M$

Difference law for limits : $\underset{x\to a}{lim}(f(x)-g(x))=\underset{x\to a}{lim}f(x)-\underset{x\to a}{lim}g(x)=L-M$

Constant multiple law for limits : $\underset{x\to a}{lim}cf(x)=c\cdot \underset{x\to a}{lim}f(x)=cL$

Product law for limits : $\underset{x\to a}{lim}(f(x)\cdot g(x))=\underset{x\to a}{lim}f(x)\cdot \underset{x\to a}{lim}g(x)=L\cdot M$

Quotient law for limits : $\underset{x\to a}{lim}\frac{f(x)}{g(x)}=\frac{\underset{x\to a}{lim}f(x)}{\underset{x\to a}{lim}g(x)}=\frac{L}{M}$ for $M\ne 0$

Power law for limits : $\underset{x\to a}{lim}(f(x){)}^n={(\underset{x\to a}{lim}f(x))}^n=L^n$ for every positive integer $n$

Composition law of limit: $\underset{x\to a}{lim}(f\circ g)(x)=f(\underset{x\to a}{lim}g(x))=f(M)$, only if $\mathrm{f}(\mathrm{x})$ is continuous at $\mathrm{g}(\mathrm{x})=\mathrm{M}$.

If $\underset{x\to a}{lim}f(x)=+\mathrm{\infty }$ or $-\mathrm{\infty }$, then $\underset{x\to a}{lim}\frac{1}{f(x)}=0$

Recommended Video Based on Algebra of Limits

Solved Examples Based On Algebra Of Limits:

Example 1: $\underset{x\to \frac{\pi }{4}}{lim}\frac{{\cot }^{3}x-\tan x}{\cos (x+\frac{\pi }{4})}$ is: [JEE Main 2019]
1) $4\sqrt{2}$
2) $8$
3) $4$
4) $8\sqrt{2}$

Solution:
Evaluation of Trigonometric limit -
$\underset{x\to a}{lim}\frac{\sin (x-a)}{x-a}=1$
$\underset{x\to a}{lim}\frac{\tan (x-a)}{x-a}=1$
put $x=a+h$ where $h\to 0$
Then it comes

$\begin{array}{rl}& \underset{h\to 0}{lim}\frac{\sin h}{h}=\underset{h\to 0}{lim}\frac{\tan h}{h}=1\\ & \therefore \underset{x\to 0}{lim}\frac{\sin x}{x}=1\text{ and }\\ & \therefore \underset{x\to 0}{lim}\frac{\tan x}{x}=1\end{array}$
Limit of product/quotient -

Limit of product/quotient is the product/quotient of individual limits such that

$\begin{array}{rl}& \underset{x\to a}{lim}(f(x)\cdot g(x))\\ & =\underset{x\to a}{lim}f(x),\underset{x\to a}{lim}g(x),\text{ given that }f(x)\text{ and }g(x)\text{ are non-zero finite values }\\ & \underset{x\to a}{lim}\frac{f(x)}{g(x)}=\frac{\underset{x\to a}{lim}f(x)}{\underset{x\to a}{lim}g(x)},\text{ given that }f(x)\text{ and }g(x)\text{ are non-zero finite values }\end{array}$
Also $\underset{x\to a}{lim}kf(x)$

$=k\underset{x\to a}{lim}f(x)$
Using LH Rule

$\underset{x\to \frac{\pi }{4}}{lim}\frac{3{\cot }^{2}x(-{\csc }^{2}x-{\sec }^{2}x)}{-\sin (x+\frac{\pi }{4})}=8$
Hence, the answer is the option 2.

Example 2: $\stackrel{x\to 0}{lim}\frac{(27+x{)}^{1/3}-3}{9-(27+x{)}^{2/3}}$ equals [JEE Main 2018]
1) $\frac{1}{3}$
2) $-\frac{1}{3}$
3)$-\frac{1}{6}$
4) $\frac{1}{6}$

Solution:

As we have learned

Limit of product/quotient -

Limit of product is the product of individual limits such that

$\begin{array}{rl}& \underset{x\to a}{lim}f(x)\cdot g(x)\\ & =\underset{x\to a}{lim}f(x)\cdot \underset{x\to a}{lim}g(x)\\ & \text{ also }\underset{x\to a}{lim}kf(x)\\ & =k\underset{x\to a}{lim}f(x)\\ & \underset{x\to a}{lim}\frac{f(x)}{g(x)}=\frac{\underset{x\to a}{lim}f(x)}{\underset{x\to a}{lim}g(x)}\\ & \text{ using approximation }(1+x{)}^n\approx 1+nx\\ & \Rightarrow \underset{x\to 0}{lim}\frac{3[1+\frac{1}{3}\times \frac{x}{27}-1]}{9[1-1-\frac{x}{27}\times \frac{2}{3}]}\\ & =-1/6\end{array}$
Hence, the answer is the option 3.

Hence, the answer is the option 3.

Example 3: For each $t\in R$, let $[t]$ be the greatest integer less than or equal to $t$ then $\underset{x\to 1^{+}}{lim}\frac{(1-|x|+\sin |1-x|)\sin (\frac{\pi }{2}[1-x])}{|1-x|[1-x]}$ [JEE Main 2019]
1) equals $1$

2) equals $0$

3) equals $-1$

4) does not exist

Solution:
Limit of productiquotient is the product/quotient of individual limits such that

$\underset{x\to a}{lim}(f(x)\cdot g(x))$

$=\underset{x\to a}{lim}f(x)\cdot \underset{x\to a}{lim}g(x)$, given that $f(x)$ and $g(x)$ are non-zero finite values
$\underset{x\to a}{lim}\frac{f(x)}{g(x)}=\frac{\underset{x\to a}{lim}f(x)}{\underset{x\to a}{lim}g(x)}$, given that $f(x)$ and $g(x)$ are non-zero finite values

$\begin{array}{rl}& \text{ Also }\underset{x\to a}{lim}kf(x)\\ & =k\underset{x\to a}{lim}f(x)\end{array}$

Evaluation of Trigonometric limit -

$\begin{array}{rl}& \underset{x\to a}{lim}\frac{\sin (x-a)}{x-a}=1\\ & \underset{x\to a}{lim}\frac{\tan (x-a)}{x-a}=1\end{array}$

put $x=a+h$ where $h\to 0$
Then it comes

$\begin{array}{rl}& \underset{h\to 0}{lim}\frac{\sin h}{h}=\underset{h\to 0}{lim}\frac{\tan h}{h}=1\\ & \therefore \underset{x\to 0}{lim}\frac{\sin x}{x}=1\text{ and }\\ & \therefore \underset{x\to 0}{lim}\frac{\tan x}{x}=1\\ & \underset{x\to 1^{+}}{lim}\frac{(1-|x|+\sin |1-x|\sin (\frac{\pi }{2}[1-x]))}{|1-x|[1-x]}\\ & =\underset{x\to 1^{+}}{lim}\frac{(1-x)+\sin (x-1)}{(x-1)(-1)}\sin (\frac{\pi }{2}(-1))\\ & =\underset{x\to 1^{+}}{lim}(1-\frac{\sin (x-1)}{x-1})(-1)=0\end{array}$

Example 4: Let $f:R\to R$ and $g:R\to R$ be defined as $f(x)=\{\begin{array}{cl}x+a& x<0\\ |x-1|& x⩾0\end{array}$ and $g(x)=\{\begin{array}{cl}x+1& x<0\\ (x-1{)}^2+b& x⩾0\end{array}$. where $a,b$ are non-negative real numbers. If (gof) $(x)$ is continuous for all $x\in R$, then $\mathrm{a}+\mathrm{b}$ is equal to $$ [JEE Main 2021]

1) $1$

2) $2$

3) $3$

4) $4$

Solution:

$\begin{array}{rl}& g[f(x)]=\{\begin{array}{cc}f(x)+1& f(x)<0\\ (f(x)-1{)}^2+b& f(x)\ge 0\end{array}\\ & g[f(x)]=\{\begin{array}{cc}x+a+1& x+a<0\mathrm{\&}x<0\\ |x-1|+1& |x-1|<0\mathrm{\&}x\ge 0\\ (x+a-1{)}^2+b& x+a\ge 0\mathrm{\&}x<0\\ (|x-1|-1{)}^2+b& |x-1|\ge 0\mathrm{\&}x\ge 0\end{array}\\ & g[f(x)]=\{\begin{array}{cc}x+a+1& x\in (-\mathrm{\infty },-a)\mathrm{\&}x\in (-\mathrm{\infty },0)\\ |x-1|+1& x\in [-a,\mathrm{\infty })\mathrm{\&}x\in (-\mathrm{\infty },0)\\ (x+a-1{)}^2+b& x\in \varphi \\ (|x-1|-1{)}^2+b& x\in R\mathrm{\&}x\in [0,\mathrm{\infty })\end{array}\end{array}$

$g(f(x))$ is continuous

$\begin{array}{ll}\text{ at }x=-a& \text{ at }x=0\\ 1=b+1& (a-1{)}^2+b=b\\ b=0& a=1\\ \Rightarrow a+b=1\end{array}$
Hence, the answer is the option 1.

Example 5: Let $f,g$ and $h$ be the real valued functions defined on $\mathbb{R}$ as and $f(x)=\{\begin{array}{cl}\frac{x}{|x|},& x\ne 0\\ 1,& x=0\end{array},g(x)=\{\begin{array}{cl}\frac{\sin (x+1)}{(x+1)},& x\ne -1\\ 1,& x=-1\end{array}$ and $\mathrm{h}(\mathrm{x})=2[\mathrm{x}]-\mathrm{f}(\mathrm{x})$, where $[\mathrm{x}]$ is the greatest integer $\le \mathrm{x}$. Then the value of $x\to 1limg(h(x-1))$ is:
[JEE Main 2023]
1) $-1$
2) $0$
3) $\sin (1)$
4) $1$

Solution:

$\begin{array}{rl}& \underset{\delta \to 0}{lim}g(\mathrm{h}(-\delta ))\delta >0\\ & \underset{\delta \to 0}{lim}g(-2+1)\\ & \Rightarrow g(-1)=1\end{array}$

RHL

$\begin{array}{rl}& \underset{\delta \to 0}{lim}g(h(\delta ))\\ & \underset{\delta \to 0}{lim}g(2\times 0-1)\\ & \underset{\delta \to 0}{lim}g(-1)\\ & \underset{x\to 1}{lim}g(h(x-1)=1\end{array}$

Hence, the answer is the option 4.