Area of Isosceles Triangle - (Formulas, Derivation and Examples)
Triangles are used in wide range of mathematics field and have many applications. Isosceles triangle is triangle with two equal sides and angles. It has been used frequently in art and architecture, building design, etc. We come across this figure in daily life in the form of pizza slices, and other items as well.
JEE Main: Study Materials | High Scoring Topics | Preparation Guide
JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs
- Area of Isosceles Triangle
- Area of Isosceles Triangle in Different Conditions
- Area of Isosceles Right Triangle
- Solved Examples based on Area of Isosceles Triangle formula
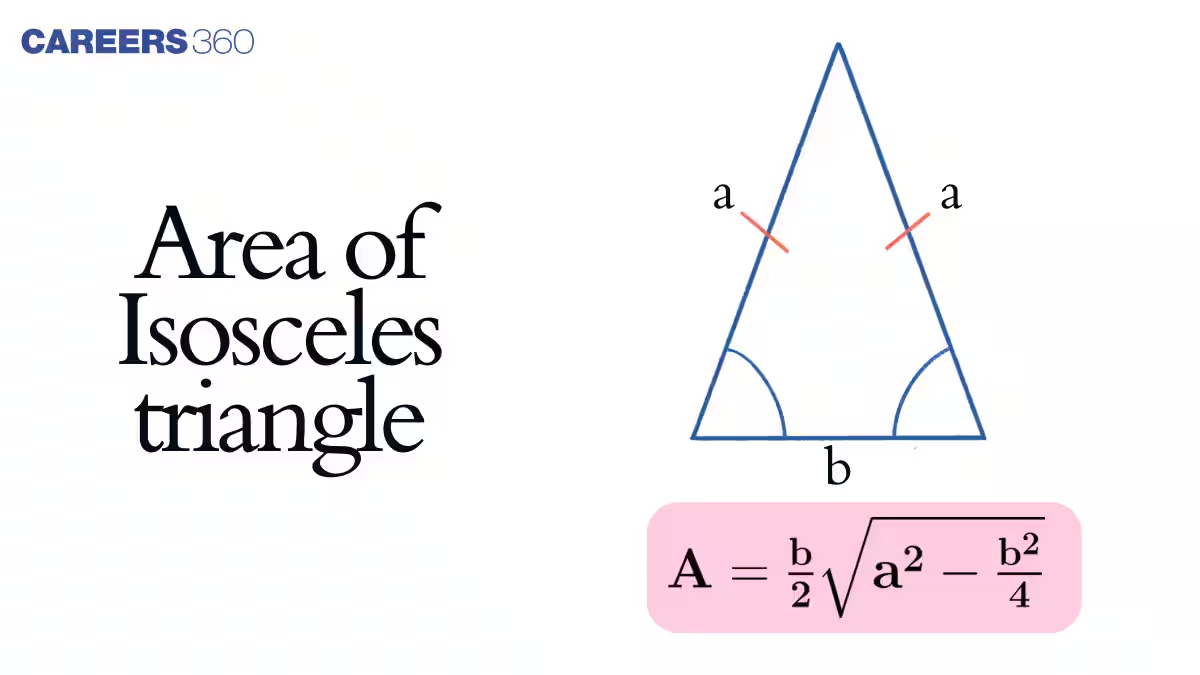
This article is about the concept of area of isosceles triangle. Let's see in detail what is the area of isosceles triangle class 9, various ways to find its area with the help of values given to us like formula of area of isosceles triangle, area of isosceles triangle by heron's formula, and much more.
Area of Isosceles Triangle
Isosceles triangle is a triangle that has any two of its sides equal in length and also 2 equal angles. If a perpendicular line is drawn from the point of intersection of two equal sides to the base of the unequal side, then two right-angle triangles are generated.
The area of isosceles triangle is defined as the space or region covered by it on a two dimensional plane. Following are few of its properties:
- The two equal sides of an isosceles triangle are known as legs and the angle between them is called the vertex angle.
- The side opposite to the vertex angle is called the base and base angles are always equal.
- The perpendicular from the vertex angle always bisects the base and also the vertex angle.
The area of isosceles triangle is usually expressed in square units like $\mathrm{m}^2, \mathrm{~cm}^2, \mathrm{in}^2, \mathrm{yd}^2$, etc.
The area of isosceles triangle is defined as the amount of region within the triangle in 2-D or x-y plane. The formula for area of isosceles triangle is equal to half the product of the base and its height.
Area of Isosceles Triangle Formula
The area of isosceles triangle can be easily calculated if it's height and base are known or if not known, we have some other methods to find out the area as we discuss furthur. We multiply the height with the base and divide it by 2, that finally results in the area of isosceles triangle. Since we know that area is represented in sq units, we write the final answers along with mentioning units such as sq m, sq cm, etc.
The area of isosceles triangle formula is expressed as follows:
Area $= \frac{1}{2} \times$ base $\times$ Height
(where b is the base and h is the height of triangle as we define with usual notations)
The perimeter of a figure is the sum of all of its sides. Hence, the perimeter of isosceles triangle $\mathrm{P}=2 \mathrm{a}+\mathrm{b}$
The altitude of isosceles triangle $h=\sqrt{ }\left(\frac{a^2-b^2}{4}\right)$
Area of Isosceles Triangle in Different Conditions
Area of isosceles triangle in different conditions include the area of isosceles triangle if only sides are known, area of isosceles triangle without height and area of isoceles triangle using trigonometry.
How to find the Area of Isosceles triangle if Only Sides are Known?

If we know all the sides of triangle, then the height or altitude can be calculated using the following formula:
Altitude of Isosceles Triangle $=\sqrt{ }\left(\frac{a^2-b^2}{4} \right)$
Area of Isosceles Triangle Formula Using Only Sides $\frac{1}{2}\left[\sqrt{ }\left(\frac{a^2-b^2}{4} \right) \times b\right]$
Where,
- $\mathrm{b}=$ base of isosceles triangle
- $\mathrm{h}=$ height of isosceles triangle
- $\mathrm{a}=$ length of two equal sides
From the diagram above,
$(A, B, C D$ are $E, F, G, H)$
$
\begin{aligned}
& \mathrm{FH}=\mathrm{HG}=\frac{1}{2} \mathrm{FG}=\frac{1}{2} \mathrm{~b} \\
& \mathrm{EF}=\mathrm{EG}=\mathrm{a}
\end{aligned}
$
Now we make the use of Pythagoras theorem for $\triangle \mathrm{EFG}$,
$
\begin{aligned}
& a^2=(\frac{b}{2})^2+(E H)^2 \\
& E H=\sqrt{ }\left(\frac{a^2-b^2}{4}\right)
\end{aligned}
$
The altitude of isosceles triangle $=\sqrt{ }\left(\frac{a^2-b^2}{4}\right)$
Formula to find area of isosceles triangle $=\frac{1}{2} \times b \times h$
Substituting the value for height:
Formula to find area of isosceles triangle using only sides $=\frac{1}{2} \left[\sqrt{ }\left(\frac{a^2-b^2}{4}\right) \times b\right]$
Area of Isosceles Triangle without height
The area of isosceles triangle can be found out using heron's formula when we do not know the height.
Area of isosceles triangle by heron's formula:
Area $=\sqrt{ [s(s-a)(s-b)(s-c)]}$
Where, $s= \frac{1}{2} (a+b+c)$
Now, we know that for an isosceles triangle,
$s= \frac{1}{2} (a+a+b)$ (since the two sides are equal)
$
\Rightarrow \mathrm{s}= \frac{1}{2} (2 \mathrm{a}+\mathrm{b})
$
Or, $\mathrm{s}=\mathrm{a}+(\frac{\mathrm{b}}{ 2})$
Area $=\sqrt{ [s(s-a)(s-b)(s-c)]}$
Or, Area $=\sqrt{ \left[s(s-a)^2(s-b)\right]}$
$\Rightarrow$ Area $=(\mathrm{s}-\mathrm{a}) \times \sqrt{[s(\mathrm{s}-\mathrm{b})]}$
Substituting the value of " s "
Area $=\left(\frac{a+b}{2}-a\right) \times \sqrt{\left[\left(\frac{a+b}{2}\right)\left(\frac{a+b}{2}-b\right)\right]}$.
Area $=\frac{b}{2} \times \sqrt{\left(\frac{a^2-b^2}{4}\right)}$
Area of Isosceles Triangle Using Trigonometry
Here we use the 2 equal sides of triangle and the angle between them,
Formula of area of isosceles triangle $=\frac{1}{2} \times b \times c \times \sin (\alpha)$
Else, when we use 2 angles and length between them,
Area of isosceles triangle $=\frac{[c^2 \times \sin (\beta) \times \sin (\alpha)}{2} \times \sin (2 \pi-\alpha-\beta)]$
The following table summarises the formula to find area of isosceles triangle.
| Using base and Height | $A = \frac{1}{2} \times b \times h$ where b = base and h = height |
| Using all three sides | $A= \frac{1}{2}\left[\sqrt{ \left(\frac{a^2-b^2}{4}\right)} \times b\right]$
|
| Using the length of 2 sides and an angle between them | $A = \frac{1}{2} × a × b × \sin(\alpha)$
|
| Using two angles and length between them | $A = \left.a^2 \times \sin (\beta) \times \sin (\alpha) / 2 \times \sin (2 \pi-\alpha-\beta)\right]$
|
| Area formula for an isosceles right triangle | $A = \frac{1}{2} × a^2$ a is the measure of equal sides |
Area of Isosceles Right Triangle
An isosceles right triangle is a triangle with two equal sides and a $90^\circ$ angle where the equal angles measure $45^\circ$ each. The area of isosceles right triangle is the area covered within the isosceles right trianlge in a two dimensional plane.
Area of Isosceles Right Triangle Formula

The formula for Isosceles Right Triangle Area $=\frac{1}{2} \times a^2$
(C,A,B replaced as $P, Q, R)$
We calculate the length of the hypotenuse as:
$
\begin{aligned}
& P R 2=a^2+a^2 \\
& P R=\sqrt{2a }
\end{aligned}
$
Area of isosceles triangle is $=\frac{1}{2} \times$ base $\times$ height
Area $=\frac{1}{2} \times a \times a=\frac{a^2}{2}$ square units
Area $=\frac{1}{2} \times$ base $\times$ height
Hence area of isosceles triangle is $=\frac{1}{2} \times a \times a=\frac{a^2}{2}$
Perimeter of Isosceles Right Triangle
Perimeter can be defined as sum of all sides of a triangle.
We take the 2 equal sides to be $r$. Using Pythagoras theorem, we find the unequal side to be $\mathrm{r} \sqrt{2}$
Therefore, perimeter of isosceles right triangle $=r+r+r \sqrt{ } 2 \mid$
$
\begin{aligned}
& =2 r+r \sqrt{2 } \\
& =r(2+\sqrt{ 2}) \\
& =r(2+\sqrt{2 })
\end{aligned}
$
Solved Examples based on Area of Isosceles Triangle formula
Example 1: Find the area of isosceles triangle given $\mathrm{b}=10 \mathrm{~cm}$ and $\mathrm{h}=12 \mathrm{~cm}$ ?
Solution:
Base of triangle $(b)=10 \mathrm{~cm}$
Height of triangle $(h)=12 \mathrm{~cm}$
The Area of Isosceles Triangle $=(\frac{1}{2}) \times b \times h$
$
\begin{aligned}
& =(\frac{1}{2}) \times 10 \times 12 \\
& =60 \mathrm{~cm}^2
\end{aligned}
$
Example 2: Find the length of the base of an isosceles triangle whose area is $240 \mathrm{~cm}^2$, and the altitude of the triangle is 20 cm .
Solution:
Area of triangle $=A=240 \mathrm{~cm}^2$
Height of triangle $(h)=20 \mathrm{~cm}$
The base of triangle $=\mathrm{b}=$ ?
Formula to find area of isosceles triangle $=(\frac{1}{2}) \times b \times h$
$240=(\frac{1}{2}) \times b \times 20$
$240= \frac{b \times 20}{2}$
$b= \frac{240 \times 2}{2}$
$\mathrm{b}=24 \mathrm{~cm}$
Thus, the base of the triangle is 24 cm .
Example 3: What is area of isosceles triangle given $\mathrm{h}=30 \mathrm{~cm}, \mathrm{~b}=10 \mathrm{~cm}$.
Solution:
Given, $\mathrm{a}=30 \mathrm{~cm}$
$
b=10 \mathrm{~cm}
$
Formula to find area of isosceles triangle
$
\begin{aligned}
& = \frac{b \times h}{2} \\
& = \frac{30 \times 10}{2} \mathrm{~cm}^2 \\
& =150 \mathrm{~cm}^2
\end{aligned}
$
$
A=150 \mathrm{~cm}^2
$
Example 4: Find the length of the base of an isosceles triangle whose area is $200 \mathrm{~cm}^2$, and the altitude of the triangle is 3 cm .
Solution:
Area of the triangle, $A=200 \mathrm{~cm}^2$
Height of the triangle $(h)=3 \mathrm{~cm}$
The base of the triangle $=\mathrm{b}=$ ?
Area of Isosceles Triangle $=(\frac{1}{2}) \times b \times h$
$
\begin{aligned}
& 200=(\frac{1}{2}) \times b \times 3 \\
& 200= \frac{b \times 3}{2} \\
& b= \frac{200 \times 2}{3}
\end{aligned}
$
$
\mathrm{b}=133.3 \mathrm{~cm}
$
Example 5: What is the area of isosceles triangle given the length of the base is 10 cm and height is 7 cm ?
Solution:
Base of the triangle $(b)=10 \mathrm{~cm}$
Height of the triangle $(\mathrm{h})=7 \mathrm{~cm}$
Area of Isosceles Triangle $=(\frac{1}{2}) \times \mathrm{b} \times \mathrm{h}$
$
\begin{aligned}
& =(\frac{1}{2}) \times 10 \times 7 \\
& =35 \mathrm{~cm}^2
\end{aligned}
$
List of Topics Related to Area of Isosceles Triangle
| Area of Circle | Area of Square |
| Area of Rectangle | Area of Sphere |
| Area | Area of Quadrilateral |
| Area of Parallelogram | Area and Perimeter |
| Area of Equilateral Triangle | cm to inches converter |
Frequently Asked Questions (FAQs)
The area of isosceles triangle in simple words mean the total space covered by the triangle.
We define an isosceles triangle as a triangle having two sides equal, that also means it has two equal angles.
The formula to find area of isosceles triangle are as follows:.
- Using base and Height: Area $= \frac{1}{2} \times b \times h$
- Using all three sides: Area $=\frac{1}{2} \left[\sqrt{ }\left( \frac{a^2-b^2}{4}\right) \times b\right]$
The perimeter of an isosceles triangle is generally defined as the length of the boundary of an isosceles triangle whereas the area of isosceles triangle is the region covered by it in 2-D space.
Formula to find area of isosceles triangle with 3 sides is given by Heron's formula, that is Area $=\sqrt{ } \mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c}) \mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})$. For an isosceles triangle, side $\mathrm{c}=$ side a. The general formula to find area of isosceles triangle is given as, Area of isosceles triangle $=\frac{b}{2} \times \sqrt{ }\left( \frac{a^2-b^2}{4}\right)$.
Also Read
20 Jan'25 04:15 PM
20 Jan'25 04:10 PM
20 Jan'25 04:08 PM
20 Jan'25 04:06 PM
20 Jan'25 03:48 PM
20 Jan'25 03:46 PM
20 Jan'25 03:34 PM