Area - Definition, Area of Shapes Formula

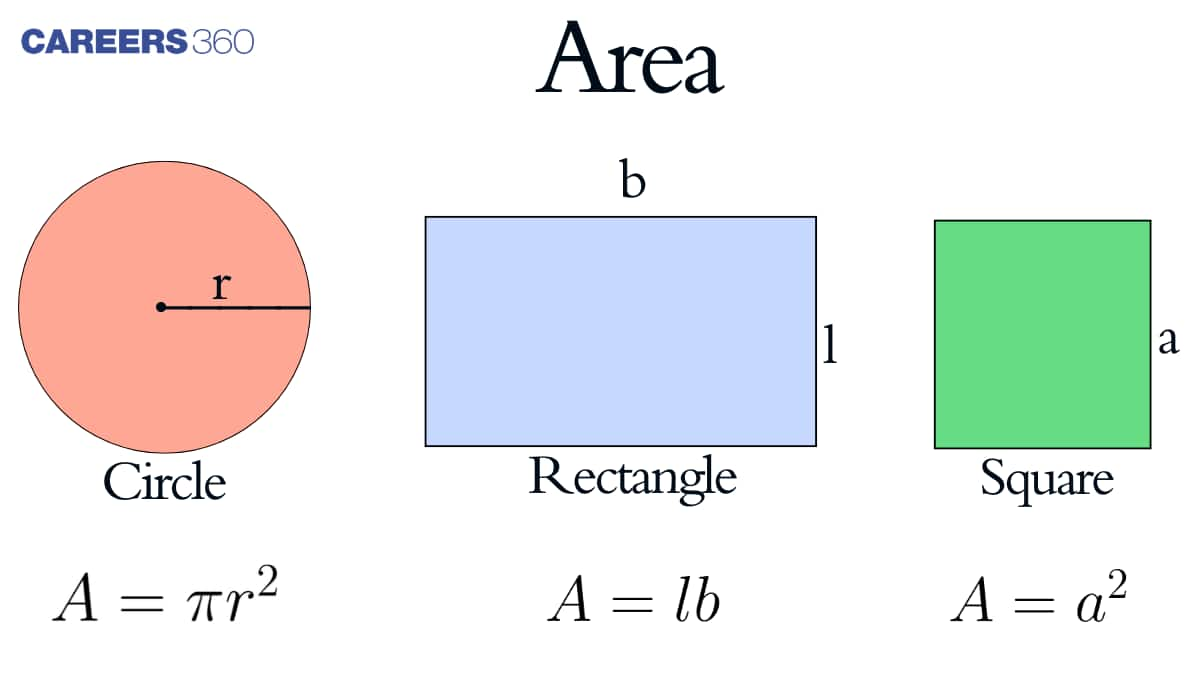
Area is the amount of space covered by a 2-D shape. The other way round, it is the quantity that measures the number of unit squares that cover the surface of a closed figure. For example, if we divide a figure in number of squares and then count those squares, it gives us the area for that shape. The standard unit of area is square units.

We have various shapes around us that have different shapes and sizes. Shapes can be found in various forms ranging from table as square, TV display as rectangle, bangle as circle, rubics cube as cube, a pipe as a cylinder, pizza slice as a triangle and much more. We come across them daily in some or the other way.

In this article we will dicuss about areas and perimeters of usual shapes that we come across our daily life such as triangle, rectangle, parallelogram, rhombus, cube, cuboid, etc. We will know about area of shapes, how do you find area and perimeter, area formulas, etc. We will look at the formulas for area and perimeter separately for every shape and also solve questions for a better understanding of the concept.

Area

Area is one of the fundamental concepts in geometry. Area is the amount of space covered within the shape. Technically, area measures the number of unit squares inside the given shape. Now, let us look into the formulas of area of shapes.

Triangle

A triangle is a three sided shape. There are three types of triangle. Namley, equilateral triangle, isoceles triangle and scalene triangle. Now let us look into the area of triangle.

Area of Triangle

Area of triangle is defined as the region covered by a triangle in an xy plane and is denoted as $A= \frac{1}{2} \times b \times h$ in general way, it applies to all types of triangle, be it scalene, isosceles, equilateral triangle. Units used are square units, like, $\mathrm{m}^2, \mathrm{~cm}^2$, in ${ }^2$, etc

Area of an Equilateral Triangle

From our previous knowledge we know that it is a triangle in which all the sides are equal. The perpendicular line that is drawn from the vertex of the triangle to the base divides the base into two equal parts as usual. Formula for area of equilateral triangle :

$A= \frac{(\sqrt{3})}{4} \times$ side $^2$

Area of an Isosceles Triangle

This type of triangle has two sides equal and the angles opposite to the equal sides are also equal. Formula for area of isosceles triangle used:

$A=\frac{1}{2} \times$ base $\times$ height

Rectangle

A rectangle is a four sided shape where opposite sides are equal and the diagonals are equal. The vertices of the rectangle makes $90^\circ$ with each other.

Area of Rectangle

Area of rectangle means the space covered by a rectangle in a 2-D plane which can also be called as an xy plane covering 2 axis. A rectangle is a type of quadrilateral, a 2-D shape that has equal number of sides and vertices that is 4. All the four angles measure 90 degrees. The opposite sides are always equal and parallel to one other.

Area of Rectangle Formula

Area $=$ Length $\times$ Breadth

$
\mathrm{A}=\mathrm{lb}
$

How to Calculate the Area of Rectangle

We follow the below steps to find the area of rectangle:

Step 1: We write dimensions of length, width as given in the question to us.

Step 2: Next, we multiply length, width values.

Step 3: At last, we write answer in square units along with the calculated numeric value of area.

Circle

A circle is a round shape with no corners or edges.

Area of Circle

It is the space covered by the circle in an x-y plane. Or the other way around, the space occupied within the boundary/circumference of a circle.

Area of Circle Formula

We can find the area of circle once we know the diameter from which we find radius.

We use the following formulas:

- Area of circle $=\pi \times \mathrm{r}^2$, (r=radius)
- Area of circle in terms of diameter $=(\frac{\pi}{ 4}) \times \mathrm{d}^2,(\mathrm{~d}=$ diameter $)$
- Area of circle in terms of circumference $=\frac{C^2 }{4} \pi$, (C=circumference $)$

Square

A square is a four sided shape where all the sides are equal and the diagonals are equal. The vertices of the square makes $90^\circ$ with each other.

Area of Square

Generally, area of square is stated as the number of square units required to fill the shape. In other words, the area of a square is the region within its boundary. It can also be calculated with the help of other dimensions, for example the diagonal and the perimeter of the square.

Area of Square Formula

For finding area of square, we multiply the length of its two sides, which are always equal in measurement and so the unit of the area is given in square units.

Area of square $=$ Side $\times$ Side $=\mathrm{S}^2$
Area of square using diagonals $= \frac{Diagonal ^2}{ 2}$.

Trapezium

A trapezium is a four sided shape with two parallel sides and two non parallel sides.

Area of Trapezium

We know from previous knowledge that a trapezium is a quadrilateral, which is defined as a shape with four sides and one set of parallel sides. Area of a trapezium depends upon the length of parallel sides and height of the trapezium. It is measured in square units.

Area of Trapezium Formula

Formula used to find the area of trapezium:

Area $=(\frac{1}{2}) h(a+b)$

where,

• a and b are the length of parallel sides/bases of the trapezium
• h is the height or distance between parallel sides.

How to Calculate Area of Trapezium?

We take help from below mentioned steps to find area of trapezium:

• Step 1: First, we find the dimensions trapezium, that is., length of parallel sides and height.
• Step 2: Next we add the length of parallel sides.
• Step 3: Now we multiply the sum of parallel sides with the height of trapezium.
• Step 4: At last, we multiply the above value by $\frac{1}{2}$ to get the final result .

Parallelogram

A parallelogram is a four sided shape with parallel and equal opposite sides.

Area of Parallelogram

Area of parallelogram is the region covered by the parallelogram in a x-y plane. If we try to recall, a parallelogram is a special type of quadrilateral that has the pair of opposite sides as parallel. The opposite sides are always of equal length and opposite angles are of equal measures.

Area of Parallelogram Formula

To find the area of parallelogram, we simply multiply the base of the perpendicular by its height. These both quantities are always perpendicular to each other, whereas the lateral side of the parallelogram is not perpendicular to the base.

Hence,

Area $=\mathrm{b} \times \mathrm{h}$ Square units(b=base, h=height)

How to Calculate the Area of Parallelogram?

We calculate area of parallelogram by using its base and height. It can also be calculated if its two diagonals are known along with any of their intersecting angles or if the length of the parallel sides is known, along with any of the angles between the sides.

Area of Parallelogram Using Sides

Let us suppose p and q are the set of parallel sides of a parallelogram and h is the height, then based on the length of sides and height of it, formula for the area is :

Area $=$ Base $\times$ Height
$\mathrm{A}=\mathrm{p} \times \mathrm{h} \quad$ [sq.unit]

Area of Parallelogram Without Height

If the height of the parallelogram is unknown to us, then we use the concepts of trigonometry to achieve our aim.

Formula used :

Area $=a b \sin (x)$

Where a , b are the length of adjacent sides of the parallelogram and x is the angle between them.

Area of Parallelogram Using Diagonals

It can also be calculated using its diagonal lengths. There are two diagonals for a parallelogram, which always intersect each other. Suppose the diagonals intersect each other at an angle p, then the area of the parallelogram is given by:

Area $=\frac{1}{2} \times d_1 \times d_2 \sin (p)$

Rhombus

A rhombus is a four sided shape with parallel and equal sides.

Area of Rhombus

Area of Rhombus is defined as the amount of space covered by a rhombus in a two-dimensional space.It has four sides that are equal in length and are always congruent. It is also a type of a quadrilateral.

How to Calculate Area of Rhombus?

There exist three methods to find the area of a rhombus:

• Method 1: By using Diagonals
• Method 2: With the help of Base and Height
• Method 3: By using Trigonometry (using side and angle)

Area of Rhombus Using Diagonals: Method 1

We consider a rhombus ABCD, that has two diagonals, AC & BD.

Step 1: First we find the length of diagonal $1, d_1$. It is the distance between $A$ and $C$. The diagonals of a rhombus are perpendicular to each other.

Step 2: Next , we find the length of diagonal 2, $d_2$, the distance between $B$ and $D$.
Step 3: Then, we multiply both the diagonals, $d_1$, and $d_2$.
Step 4: Finally we divide the result by 2 and get answer.

Area of Rhombus Using Base and Height: Method 2

Step 1: We start by finding the base and height of the rhombus. The base of the rhombus is one of its sides, and the height is the altitude which is perpendicular distance from the chosen base to the opposite side.

Step 2: Then we multiply the base and the calculated height to get result.

Area of Rhombus Using Trigonometry: Method 3

We use this way when the side and one of its internal angles are given.

• Step 1: We square the length of any of the sides.
• Step 2: Next, we multiply it by Sine of one of the angles.

Cylinder

A cylinder is simply a three-dimensional structure having circular bases which are parallel to each other. It does not possess any vertices. It has 2 main values involved which are radius and its height.

Area of Cylinder

Area of cylinder is defined as the sum of the curved surface and the area of two circular bases.

The Surface Area of Cylinder = Curved Surface + Area of Circular bases
S.A. (in terms of $\pi)=2 \pi r(h+r)$ sq.unit
$\pi(\mathrm{Pi})=3.142$ or $=\frac{22}{7}$
$r=$ Radius
h = Height

Area of a Cylinder Formula

Surface Area of Cylinder: Surface area of cylinder is defined as the area of the curved surface of any cylinder having a base radius ‘r’, and height ‘h’, generally known as Lateral Surface Area (LSA). Formula for surface area of cylinder:

CSA or LSA $=2 \pi \times r \times h$ Square units

Base Area of Cylinder: It is a circular shape. Area of the circular bases of cylinder = $=2\left(\pi r^2\right)$

Total Surface Area of Cylinder: It is equal to the sum of the areas of all its faces. The total surface area with radius ‘r’, and height ‘h’ is equal to the sum of the curved area and circular areas of the cylinder.

TSA $=2 \pi \times r \times h+2 \pi r^2=2 \pi r(h+r)$ Square units

Sphere

It is a solid curved surface in such a way that every point on the surface is the same distance from the centre. We can say that it is a 3D representation of a circle and cover areas in all x,y,z planes in geometry. We can find it in various forms around us like celestial bodies, football, basketball, etc.

Area of Sphere

Area of Sphere is the region covered by a surface of a spherical object in a three-dimensional space. As we have discussed earlier, if we try to spin a circle around a fixed axis and visualize this in our mind, we will find that we obtain the figure of a sphere.

How to Find Area of Sphere?

Now to find the area of the sphere, we follow the steps:

• First we find the radius of the sphere.
• Then we mention the value of radius in the surface area formula, i.e. ($4πr^2$).
• After this we solve the expression and evaluate the final value.

Surface Area of Sphere

Surface area of sphere is the area occupied by the curved surface of the sphere. Circular shapes take the shape of a sphere when we observe them as three-dimensional structures. For example, a globe, cricket ball or a soccer ball.

Formula of Surface Area of Sphere

The formula for surface area of sphere just depends on the radius of the sphere.

Surface Area of Sphere $=4 \pi r^2 ; r=$ radius
In terms of diameter, $\mathrm{S}=4 \pi(\frac{\mathrm{d}}{2})^{2 ;}$ d=diameter

Cube

A cube is a 3 dimensional shape with equal sides. A cube is made up of 6 squares as their faces.

Surface Area of Cube

The definition of surface area of a cube states that if the total surface area is equal to the sum of all the areas of the faces of the cube. Since the cube has six faces, therefore, the total surface area of a cube will be equal to sum of all six faces of cube.

Surface Area of Cube Formula

Total Surface Area of Cube (TSA) Formula
TSA of the cube is obtained by multiplying the square of its side length by 6 . Thus, the formula becomes " $6 \mathrm{a}^2$ ".

Total Surface Area of a Cube $=\left(6 \times\right.$ side $\left.^2\right)$ square units
Lateral Surface Area of Cube (LSA) Formula
LSA of the cube is obtained by multiplying the square of its side length by 4 . Thus, the formula becomes " $4 \mathrm{a}^2$ ".

Lateral Surface Area of a Cube $=\left(4 \times\right.$ side $\left.^2\right)$ square units.

Solved Examples based on Areas

Example 1: Find the area of square with a side of 2 cm .
Solution: Area of a square $=$ side $\times$ side. Here, side $=2 \mathrm{~cm}$
Substituting the values in formula for area of square, we get $2 \times 2=4$.
The area of the square $=4$ square $\mathrm{cm} .$

Example 2: The dimensions of a rectangle are 10 cm and 8 cm . Find area of rectangle.
Solution: The area of a rectangle is the product of its length and width, which can be represented by the formula: Area $=1 \times \mathrm{w}$.
we get area of the rectangle $=10 \times 8=80 \mathrm{~cm}^2$

Example 3: Can you find the area of circle with a radius of 6 cm ?
Solution: The radius of the circle $=6 \mathrm{~cm}$
Area of a circle is calculated by the formula $\pi r^2$
Hence, area of circle $=\frac{22}{7} \times 6 \times 6=1113.04$ square cm .

Example 4: The length of rectangular field is 11 m and width is 5 m . Find the area of the rectangular field.

Solution: Given, Length $=11 \mathrm{~m}$
Width $=5 \mathrm{~m}$
Area of rectangle $=$ length $\times$ width $=11 \times 5=55$ sq.m.

Example 5: Find area of rectangle of $\mathrm{b}=2 \mathrm{~cm}$ and $\mathrm{h}=4 \mathrm{~cm}$.
Solution: Area of rectangle $=\frac{1}{2} \times b \times h=\frac{1}{2} \times 2 \times 4=4 \mathrm{sq} \mathrm{cm}$.

List of Topics Related to Area