Arithmetico Geometric Series: Definition & Examples

Before learning the Arithmetic-Geometric Progression, let's revise the concept of sequence. A sequence is formed when terms are written in order such that they follow a particular pattern. Understanding AGP involves understanding the different principles of AP and GP. In real life, AGP is applicable in areas such as population dynamics, algorithms, etc.

JEE Main: Study Materials | High Scoring Topics | Preparation Guide

JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs

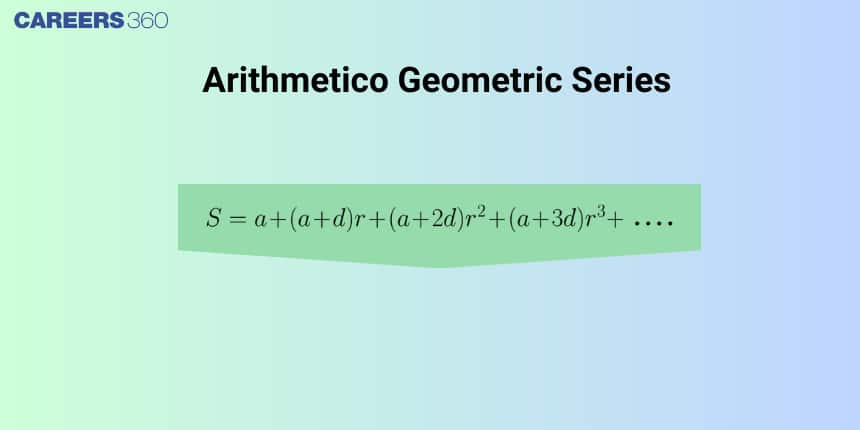
Arithmetic-Geometric Progression

Arithmetico-geometric progression is the combination of arithmetic and geometric series. This series is formed by taking the product of the corresponding elements of arithmetic and geometric progressions. In short form, it is written as A.G.P (Arithmetico-Geometric Progression).

Wherein-

An arithmetic progression is a sequence in which each term increases or decreases by a constant term or fixed number. This fixed number is called the common difference of an AP and is generally denoted by ' $d$ '.

A geometric progression or geometric sequence is a sequence where the first term is non-zero and the ratio between consecutive terms is always constant. The 'constant factor' is called the common ratio and is denoted by ' $r$ '. $r$ is also a non-zero number.

Properties of A.M. and G.M

A and G are arithmetic and geometric mean of ' $a$ ' and ' $b$ ', two real, positive and distinct number. Then,

• $a$ and $b$ are the roots of the equation $x^2-2Ax+G^2=0$.

• a and b are given by $A\pm \sqrt{(A+G)(A-G)}$.

Proof:

$\begin{array}{rl}& A=\frac{a+b}{2}\Rightarrow 2A=a+b\\ & G=\sqrt{ab}\Rightarrow G^2=ab\end{array}$

$a$ and $b$ are the roots of the equation, then

$\begin{array}{rl}& x^2-2(\text{ sum of roots })x+\text{ products of roots }=0\\ & \Rightarrow x^2-(a+b)+ab=0\\ & \Rightarrow x^2-2Ax+G^2=0\end{array}$
Roots of the equation are

$\begin{array}{rl}& x=\frac{2A\pm \sqrt{(-2A{)}^2-4\cdot 1\cdot G^2}}{2}\\ & x=A\pm \sqrt{(A+G)(A-G)}\end{array}$
Let the given AP be $a,(a+d),(a+2d),(a+3d)$, $$
And, the GP is $1,r,r^2,r^3$, $$
Multiplying the corresponding elements of the above progression, we get, $a,(a+d)r,(a+2d)r^2,(a+3d)r^3,\dots \dots$

This is a standard Arithmetico-Geometric Progression.
Eg: $1,3x,5x^2,7x^3,9x^4,\dots \dots$

The sum of n-terms of an Arithmetic-Geometric Progression

Let $S_n$ denote the sum of $n$ terms of a given sequence. Then,

${\mathrm{S}}_{\mathrm{n}}=a+(a+d)r+(a+2d)r^2+\dots \dots +(a+(n-1)d)r^{n-1}$
Multiply both side of eq (i) by ' r '

$r{\mathrm{S}}_{\mathrm{n}}=ar+(a+d)r^2+(a+2d)r^3+\dots +(a+(n-1)d)r^n$
Subtract eq (ii) from eq (i)

$\begin{array}{rl}& (1-r){\mathrm{S}}_{\mathrm{n}}=a+[dr+d{r}^2+d{r}^3+\dots .+d{r}^{n-1}]-[a+(n-1)d]r^n\\ & \Rightarrow (1-r){\mathrm{S}}_{\mathrm{n}}=a+dr\left(\frac{1-r^{n-1}}{1-r}\right)-[a+(n-1)d]r^n\\ & \Rightarrow {\mathbf{S}}_{\mathbf{n}}=\frac{\mathbf{a}}{\mathbf{1}\mathbf{-}\mathbf{r}}+\mathbf{d}\mathbf{r}\left(\frac{\mathbf{1}-{\mathbf{r}}^{\mathbf{n}-\mathbf{1}}}{(\mathbf{1}-\mathbf{r}{)}^{\mathbf{2}}}\right)-\frac{[\mathbf{a}+(\mathbf{n}-\mathbf{1})\mathbf{d}]{\mathbf{r}}^{\mathbf{n}}}{\mathbf{1}-\mathbf{r}}\end{array}$

The sum of infinte terms of an Arithmetic-Geometric Progression:

The infinite terms can not be solved mentally, so we will have to find a general approach.

Let's denote the AGP by: $a,(a+d)r,(a+2d)r^2,(a+3d)r^3,\dots$
Here, $a$ is the first term of the arithmetic progression, $d$ is the common difference of the arithmetic progression, and $r$ is the common ratio of the geometric progression.

To find the sum of the infinite AGP, we can use the following formula:

$S=\frac{a}{1-r}+\frac{dr}{(1-r{)}^2}$
This is the sum of an infinite arithmetic-geometric progression.

Recommended Video Based on Arithmetico Geometric Progression

Solved Example Based on Arithmetico Geometric Progression

Example 1: $1+3+7+15+31+.....$ $$ to $n$ terms
1) $2^{n+1}-n$
2) $2^{n+1}-n-2$
3) $2^n-n-2$
4) None of these

Solution
$\begin{array}{rl}& S_n=1+3+7+15+31+\dots \dots +T_n\\ & S_n=1+3+7+\dots \dots \dots \dots \dots +T_{n-1}+T_n\end{array}$
Subtracting

$\begin{array}{rl}& 0=1+2+4+8\dots (\text{ nterms })-T_n\\ & T_n=1\times \frac{(2^n-1)}{2-1}\\ & =(2^n-1)\\ & S_n=\sum _1^n{T}_n=\sum _1^n{2}^n-\sum _1^{n}1\\ & =2(2^n-1)-n\\ & =2^{n+1}-(n+2)\end{array}$
Hence, the answer is the option (2).

Example 2 : S is the sum of the first 9 terms $(x+ka)+(x^2+(k+2)a)+(x^3+(k+4)a)+(x^4+(k+6)a)+\dots$ where $a\ne 0$ and $x\ne 1$.If $S=\frac{x^{10}-x+45a(x-1)}{x-1}$ then k is
1) 3
2) 2
3) -3
4) -5

Solution
$\begin{array}{rl}& S=(x+ka)+(x^2+(k+2)a)+(x^3+(k+4)a)+(x^4+(k+6)a)+\dots \\ & =(x+x^2+x^3+---x^9)+a(k+(k+2)+(k+4)+\cdots (k+16))\end{array}$
First series is GP and second series becomes an AP after separ ating k terms .So,

$=\frac{x(x^9-1)}{(x-1)}+a[9k+72]$
Now, comparing this with

$S=\frac{x^{10}-x+45a(x-1)}{x-1}$
$\begin{array}{rl}& \therefore 9k+72=45\\ & k+8=5\\ & k=-3\end{array}$

Example 3 : Which of the following is not an AGP?
1) $1,2x,3x^2,4x^3$
2) $1,1,3/4,1/2,5/16\dots$.
3) $3a,5a^2,7a^3$
4) $1,{3.2}^2,{4.2}^3,{5.2}^4$

Solution

Options 1 and 3 are AGP
In Option 2, terms can be written as $1,2/2,3/4,4/8,5/16\dots$ so it is an AGP Option 4 is wrong, as for it to an AGP, the first term should have been 2.2 $(=4)$

Hence, the answer is the option (4).

Example 4: What is the sum of the first 10 terms of $1.2+{2.2}^2+{3.2}^3+\dots .$.
1) ${11.2}^{11}+2$
2) ${9.2}^{11}+2$
3) ${11.2}^{11}-2$
4) ${9.2}^{11}-2$

Solution
$S=1.2+{2.2}^2+{3.2}^3+\dots .10{.2}^{10}$
As the common ratio of the corresponding GP is 2 , so

$2S={1.2}^2+{2.2}^3+\dots \dots \dots \dots \dots ..10{.2}^{11}$
Subtracting these equations

$\begin{array}{rl}& -S=1(2+2^2+2^3+\dots ..+2^{10})-{10.2}^{11}\\ & S={10.2}^{11}-(2+2^2+2^3+\dots ..+2^{10})\\ & S={10.2}^{11}-2(2^{10}-1)\\ & ={10.2}^{11}-2^{11}+2={9.2}^{11}+2\end{array}$
Hence, the answer is the option (2).