Sum of N terms of an AP - Formula and Examples

An arithmetic progression is a sequence in which each term increases or decreases by a constant term or fixed number. The sum of n terms of the series helps us to find the sum of the series like fibonacci series. In real life, we sum n terms of an AP for calculation of the sum of series of a numbers.

This Story also Contains

1. Arithmetic Progression
2. General Term of an AP
3. The sum of n terms Of AP
4. Important points to remember
5. The sum of natural number
6. The sum of odd numbers
7. Solved Examples Based on Sum of n terms of AP

In this article, we will cover the concept of the Sum of n terms of Arithmetic Progression. This category falls under the broader category of Sequence and series, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Questions based on this topic have been asked frequently in JEE Mains.

Arithmetic Progression

An arithmetic progression is a sequence in which each term increases or decreases by a constant term or fixed number. This fixed number is called the common difference of an AP and is generally denoted by ‘$d$’.

Eg, 1, 4, 7, 10,.... is an AP with a common difference 3

Also, 2, 1, 0, -1,.... is an AP with a common difference of -1

If $a_1, a_2, a_3, a_4 \ldots \ldots \ldots a_{n-1}, a_n$ are in AP,

Then $d=a_2-a_1=a_3-a_2=\ldots \ldots \ldots \ldots$ = $a_n-a_{n-1}$

In AP, the first term is generally denoted by ‘$a$’

General Term of an AP

We found a formula for the general term of a sequence, we can also find a formula for the general term of an arithmetic sequence. First, write the first few terms of a sequence where the first term is ‘$a$’ and the common difference is ‘$d$’. We will then look for a pattern.

i.e. $a, a+d, a+2 d, a+3 d, \ldots \ldots \ldots$

Then the n^th term (general term) of the A.P. is $\mathrm{\mathit{a_n=a+(n-1)d}}$.

$a_1=a+(1-1) d=a$

$a_2=a+(2-1) d=a+d$

$a_3=a+(3-1) d=a+2 d$

$a_4=a+(4-1) d=a+3 d \ldots \ldots a_n=a+(n-1) d=l=$ last term

On simplification of the general term, we can see that the general term of an AP is always linear in $n$

$T_n=an+b$

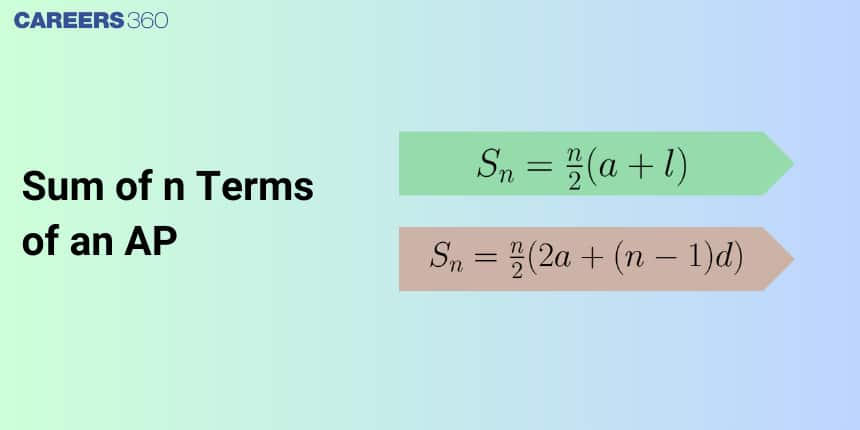
The sum of n terms Of AP

The addition of the first n terms in the arithmetic sequence equals the sum of the $n$ terms in AP. It can be expressed as follows: $n$ divided by 2 times the product of the difference between the second and first terms (also known as the common difference) and "$d$," or twice the first term ($a$), plus $(n-1)$, where $n$ is the number of terms that need to be added

The sum, S_n of $n$ terms of an AP with the first term ‘$a$’ and common difference ‘d’ is given by

$\begin{array}{l}{S_{n}=\frac{n}{2}[2 a+(n-1) d]} \\ {\text { OR }} \\ {S_{n}=\frac{n}{2}[a+l]} \\ {a \rightarrow \text { first term }} \\ {d \rightarrow \text { common difference }} \\ {n \rightarrow \text { number of terms }}\end{array}$

Proof

$\begin{aligned} & S_n=a+(a+d)+(a+2 d)+\ldots \ldots+(a+(n-2) d)+(a+(n-1) d) \ldots \ldots(i) \\ & \text { Rewrite } S_n=(a+(n-1) d)+(a+(n-2) d)+\ldots \ldots \ldots+(a-2 d)+(a-d)+a \ldots \ldots\end{aligned}$

$\begin{aligned} & 2 S_n=(2 a+(n-1) d)+(2 a+(n-1) d+\ldots \ldots \ldots \cdots \cdots \cdot \text { upto } n \text { term } \\ & S_n=\frac{n}{2}[(2 a+(n-1) d)]\end{aligned}$

Also, $a+(n-1) d=l=$ last term we can also write

$
S_n=\frac{n}{2}[a+a+(n-1) d]=\frac{n}{2}[a+l]
$

Important points to remember

1. If sum of a few terms (like 3, 4, or 5 terms) in an A.P. is given in the problem, then selecting the following terms reduces the calculation

• If we need to choose three terms in an A.P., then choose $(a-d), a,(a+d)$

[Note: Here the first term is a-d, and the common difference is $d$]

• If we need to choose four terms in an A.P., then choose $(a-3d), (a-d), (a+d), (a+3d)$

[Note: Here the first term is a-3d, and the common difference is 2 $d$ ]

• If we need to choose five terms in an A.P., then choose $(a-2d), (a-d)$, $a,(a+d),(a+2 d)$

[Note: Here the first term is a-2d, and the common difference is $d$]

2. The sum of terms equidistant from the beginning and end of an AP is constant and it equals the sum of the first and the last terms.

$\mathit{a_1+a_n=a_2+a_{n-1}=a_3+a_{n- 2}=......=a_r+a_{n-r+1}}$

The sum of natural number

The sum of the first n-natural numbers is given by

$
1+2+3+4+5+\ldots \ldots+n=\frac{\mathrm{n}(\mathrm{n}+1)}{2}
$
Use the formula, sum of $n-$ term of an AP $\mathrm{S}_{\mathrm{n}}=\frac{\mathrm{n}}{2}(\mathrm{a}+1)$; where, $\mathrm{a}=1, \mathrm{l}=\mathrm{n}$ and number of term is n

$
\begin{aligned}
& \mathrm{S}_{\mathrm{n}}=\frac{2}{\mathrm{n}}(1+\mathrm{n}) \\
& \Rightarrow \sum_{\mathrm{n}=1}^{\mathrm{n}} \mathrm{n}=\frac{\mathrm{n}(\mathrm{n}+1)}{2}
\end{aligned}
$

The sum of odd numbers

The sum of odd numbers is given by

$\begin{aligned} & 1+3+5+7+\ldots \ldots \text { upto } \mathrm{n} \text { term }=\frac{\mathrm{n}}{2}(2 \cdot 1+(\mathrm{n}-1) \cdot 2) \\ & \Rightarrow \sum(2 \mathrm{n}-1)=\mathrm{n}^2\end{aligned}$

Recommended Video Based on Sum of N Terms of an AP:

Solved Examples Based on Sum of n terms of AP

Example 1: Let $\mathrm{a_1, a_2, a_3, \ldots be \: an \: A.P. If \: a_7=3}$, the product be minimum and the sum of its first $\mathrm{n}$ terms is zero, then $\mathrm{n !-4 a_{n(n+2)}}$ is equal to : [JEE MAINS 2023]

Solution

$\begin{aligned} & \mathrm{a}_7=3 \mathrm{a}_1 \mathrm{a}_4 \text { minimum } \\ & \mathrm{a}+6 d=3 \\ & \mathrm{a}(\mathrm{a}+3 \mathrm{~d}) \rightarrow \text { minimum } \\ & \mathrm{S}_{\mathrm{n}}=0 \Rightarrow \frac{\mathrm{n}}{2}\left[n \mathrm{na}_1+(\mathrm{n}-1) \mathrm{d}\right]=0 \\ & 2 \mathrm{a}_1+(\mathrm{n}-1) \mathrm{d}=0 \end{aligned}$
Let $\mathrm{ a(a+3 d)}$ is minimum

$\mathrm{ f(d)=(3-6 d)(3-6 d+3 d) }$
$\mathrm{ f(d)=(3-6 d)(3-3 d) }$
$\mathrm{=18 d^2-27 d+9 \text { is minimum at } d=\frac{27}{2 \times 18}=\frac{9 \times 3}{2 \times 9 \times 2}=\frac{3}{4}}$

$\mathrm{So,d=\frac{3}{4}}$

$\mathrm{So,d=\frac{3}{4}}$

$\mathrm{a_{1}+6d=3}$

$\mathrm{a_{1}=3-6\frac{3}{4}=3-\frac{9}{2}=-3}$

Putting $\mathrm{a_{1}=\frac{-3}{2}\&d=\frac{3}{4}\: in (1))}$

$\mathrm{ 2\left(\frac{-3}{2}\right)+(\mathrm{n}-1)\left(\frac{3}{4}\right)=0 }$
$\mathrm{ \frac{3}{4}(\mathrm{n}-1)=3 }$
$\mathrm{ \mathrm{n}-1=4 }$
$\mathrm{ \mathrm{n}=5 }$
$\mathrm{ \mathrm{ni}-4 \mathrm{a}_{\mathrm{n}(\mathrm{n}+2)} }$
$\mathrm{ \mathrm{n}=5 \text { so } \mathrm{n} !=5 !=120 }$
$\mathrm{ \&\: \mathrm{a}_{5(7)}=\mathrm{a}_{35}=\frac{-3}{2}+(34)\left(\frac{3}{4}\right) }$
$\mathrm{ =\frac{-3}{2}+\frac{51}{2} }$
$\mathrm{ =\frac{48}{2}=24 }$
$\mathrm{ 5 !-4(24)=24 }$

Example 2: Let be an A.P. If the sum of its first four terms is 50 and the sum of its last four terms is 170, then the product of its middle two terms is [JEE MAINS 2023]

Solution

We have to find the product of its middle two terms
The following data is given to us,

$
a_1=8
$
The sum of its first four terms $=50$
The sum of its last four terms $=170$
First, find the common difference,

$\begin{aligned} & \mathrm{a}_1=8 \\ & \mathrm{~d}=\text { common difference } \\ & \frac{4}{2}[16+3 \mathrm{~d}]=50 \\ & \Rightarrow \mathrm{d}=3 \end{aligned}$

Next, we are going to find the number of terms in an AP,

$ \begin{gathered} \frac{4}{2}\left[2 a_n+3(-d)\right]=170 \\ \Rightarrow 2\left(a_1+(n-1) d\right)-3 d=85 \\ \Rightarrow 16+6(n-1)-9=85 \\ \quad n-1=13 \\ \quad n=14 \end{gathered}$

Since the number of terms is 14, the middle two terms are 7 and 8
Product of middle two terms $=\mathrm{T}_7 \times \mathrm{T}_8$
$ \begin{aligned} & =\left(\mathrm{a}_1+6 \mathrm{~d}\right)\left(\mathrm{a}_1+7 \mathrm{~d}\right) \\ & =(8+18)(8+21) \\ & =(26)(29)=754 \end{aligned}$

Hence, the required answer is 754.

Example 3: Let terms and $5,9,13,17, \ldots \text { upto } 59$ terms be two series. Then the sum of the terms common to both the series is equal to __________ [JEE MAINS 2022]

Solution

Both given series are $\mathrm{AP's}$

$3,6,9,12, \ldots \\$

$3,6,9,12, \ldots \\$

$5,9,13,17, \ldots \\$

$\mathrm{AP} \\$

$\mathrm{a^{\prime}=5, d^{\prime}=4, n^{\prime}=59}$

Common terms to $\mathrm{2AP's} . \\$ also form an $\mathrm{AP} \\$

The first term of common $\mathrm{AP,A=9} \\$

Common Difference $\mathrm{D=lcm(d,d')} \\$

$\mathrm{=\operatorname{lcm}(3,4)}\\ \\$

$\mathrm{=12}$

Let the Nth term of this common $\mathrm{AP}$ be its last term

Last term of the first $\mathrm{A P =3+(78-1) 3} \\$

$\mathrm{=234}$

Last term of second $\mathrm{A P =5+(59-1) 4} \\$

$\mathrm{=237}$

$\mathrm{\therefore \quad A+(N-1) D \leqslant 234} \\$

$\mathrm{9+(N-1) 12 \leqslant 234} \\$

$\mathrm{N-1 \leq \frac{225}{12}} \\$

$\mathrm{\Rightarrow \quad N \leqslant 19.75} \\$

$\mathrm{\therefore \quad N=19}$

$\mathrm{\text{Sum of common AP}}\\$

$\mathrm{=\frac{19}{2}[2 \cdot 9+(19-1) 12]} \\$

$\mathrm{=19[9+18(6)]} \\$

$\mathrm{=2223} \\$

Hence, the answer is $\mathrm{2223}$.

Example 4:Suppose $\mathrm{a_{1}, a_{2}, \ldots, a_{\mathrm{n}}, \ldots}$be an arithmetic progression of natural numbers. If the ratio of the sum of the first five terms to the sum of the first nine terms of the progression is $5: 17$ and $\mathrm{ 110<a_{15}<120,}$ then the sum of the first ten terms of the progression is equal to [JEE MAINS 2022]

Hence, the answer is 6.