Equation of Sphere

A Sphere is the locus of a point which moves in space in such a way that its distance from a fixed point always remains constant. The fixed point is called the centre of the sphere and the fixed distance is called the radius of the sphere. In real life, we use spheres to find the surface, volume, and diameter.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Sphere: Definition
2. Equation of Sphere
3. Derivation of Equation Of Sphere
4. Main elements of a sphere
5. Properties of a Sphere
6. Equation of sphere in diametric form
7. Equation of sphere in cylindrical coordinates
8. Equation of sphere in vector form
9. Solved Examples Based on the Equation of Sphere
10. Summary

In this article, we will cover the concept of the Equation of A Plane In The Normal Form. This topic falls under the broader category of Three Dimensional Geometry, which is a crucial chapter in Class 12 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of six questions have been asked on this topic in JEE Main from 2013 to 2023.

Sphere: Definition

A Sphere is the locus of a point which moves in space in such a way that its distance from a fixed point always remains constant. The fixed point is called the centre of the sphere and the fixed distance is called the radius of the sphere.

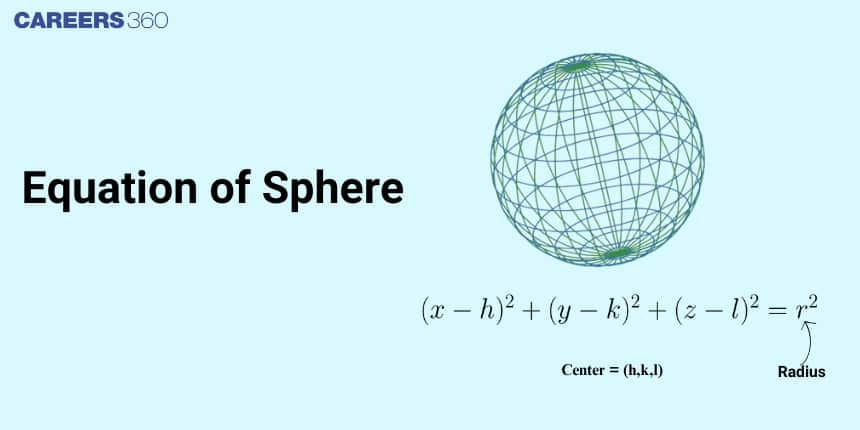
Equation of Sphere

Spheres are the $3D$ representations of circles. The equation of a sphere is similar to that of a circle but with an extra variable for the extra dimension.

If $P(x, y, z)$ is an arbitrary point on the sphere, therefore required equation of the sphere is

$
(x-a)^2+(y-b)^2+(z-c)^2=r^2
$

Derivation of Equation Of Sphere

Let $C(a, b, c)$ be the centre of the sphere with radius $r$. Let $P(x, y, z)$ be any point on the sphere,

Then,

$
\begin{aligned}
C P & =r \\
|C P| & =r
\end{aligned}
$
From the distance formula

$
\begin{array}{lc}
\Rightarrow & C P^2=r^2 \\
\Rightarrow & (x-a)^2+(y-b)^2+(z-c)^2=r^2
\end{array}
$

since, $P(x, y, z)$ is an arbitrary point on the sphere, therefore required equation of the sphere is

$
(x-a)^2+(y-b)^2+(z-c)^2=r^2
$

NOTE:

NOTE:
If the centre of the sphere is at the origin the equation of the sphere is $x^2+y^2+z^2=r^2$

The equation of the sphere can also be written as $x^2+y^2+z^2-2(a x+b y+c z)+d=0$, where $d=a^2+b^2+c^2-r^2$

Main elements of a sphere

• Radius: The length of the line segment made between the centre of the sphere to some point on its surface.

• Diameter: The length of the line segment starting from one point on the surface of the sphere and extending to the other point which is accurately opposite to it, surpassing via the centre is known as the diameter of the sphere. The length of the diameter is accurate, twice the length of the radius.

• Circumference: The length of the immense circle of the sphere is known as its circumference. The edge of the dotted circle or the cross-section of the sphere holding its centre is called its circumference.

• Volume: Similar to any other three-dimensional object, a sphere occupies some amount of space. This amount of space engaged by it is known as its volume. It is expressed in cubic units.

• Surface Area: The area covered by the surface of the sphere is called its surface area. It is measured in square units.

Properties of a Sphere

The Following Properties of a sphere are:

• A Sphere is symmetrical in every direction.
• It has neither edges nor vertices.
• A sphere is not a polyhedron since it does not contain vertices, edges, and flat faces. A polyhedron is an entity that should have a flat face.
• Air bubbles adopt the shape of a sphere since the sphere’s surface area is the least.
• The sphere would have the greatest volume among all the shapes with a similar surface area.

Equation of sphere in diametric form

The equation of the sphere when extremities of the diameter are given is called the Equation of a sphere in diametric form. So, the equation of a sphere whose extremities of diameter are $A\left(x_1, y_1, z_1\right)$ and $B\left(x_2, y_2, z_2\right)$ is given by

$
\left(x-x_1\right)\left(x-x_2\right)+\left(y-y_1\right)\left(y-y_2\right)+\left(z-z_1\right)\left(z-z_2\right)=0
$

Equation of sphere in cylindrical coordinates

In cylindrical coordinates, we have

$
X=r \cos (p), Y=r \sin (p) \text { and } Z=z
$
Here, ' $r$ ' is the radius in the $x-y$ plane and ' $p$ ' is the angle in the $x-y$ plane.
We know that the equation of sphere in the Cartesian coordinates system is $x^2+y^2+z^2=r^2$, where ' $r$ ' is the radius of the sphere.

Since $x^2+y^2=r^2$ in cylindrical coordinates, the equation of sphere in cylindrical coordinates can be written as

$
r^2+z^2=R^2
$

Equation of sphere in vector form

The general vector equation of a sphere with centre $C$ having position vector 'c' and radius is 'a'
is given as

$
(r-c)^2=a^2
$

i.e., $r^2-2 r \cdot c+\left(c^2-a^2\right)=0$

Recommended Video Based on Equation of Sphere

Solved Examples Based on the Equation of Sphere

Example 1: If the plane $2 a x-3 a y+4 a z+6=0$ passes through the midpoint of the line joining the centres of the spheres $x^2+y^2+z^2+6 x-8 y-2 z=13$ and $x^2+y^2+z^2-10 x+4 y-2 z=8$ then $a$ equals

Solution: Centre of $S_1: C_1(-3,4,1)$
Centre of $S_2: C_2(5,-2,1)$
Midpoint of $C_1 C_2$ is $(1,1,1)$
$2 a x-3 a y+4 a z+6=0$ passes through $(1,1,1)$
$2 a-3 a+4 a+6=0$
$a=-2$
Hence, the answer is -$2$

Example 2: The plane $x+2 y-z=4$ cuts the sphere $x^2+y^2+z^2-x+z-2=0$ in a circle of radius

Solution

Centre is $\left(\frac{1}{2}, 0,-\frac{1}{2}\right) \quad r=\sqrt{\frac{5}{2}}$

The perpendicular distance from the centre

$
\begin{aligned}
& \frac{\left|\frac{1}{2}+\frac{1}{2}-4\right|}{\sqrt{6}}=\sqrt{\frac{3}{2}} \\
& \text { Radius }^2=\left(\sqrt{\frac{5}{2}}\right)^2-\left(\sqrt{\frac{3}{2}}\right)^2=1
\end{aligned}
$

Hence, the answer is $1$

Example 3: If $(2,3,5)$ is one end of the diameter of the sphere $x^2+y^2+z^2-6 x-12 y-2 z+20=0$, then the coordinates of the other end of the diameter are

Solution: Coordinate of center of sphere $=(3,6,1)$
Let other end be $(x, y, z)$

$
\begin{aligned}
& \frac{x+2}{2}=3, \frac{y+3}{2}=6, \frac{z+5}{2}=1 \\
& x=4, y=9, z=-3
\end{aligned}
$
Hence, the answer is $(4,9,-3)$

Example 4: The radius of the circle in which the sphere $x^2+y^2+z^2+2 x-2 y-4 z-19=0$ is cut by the plane $x+2 y+2 z+7=0$ is

Solution:
Center of sphere $=(-1,1,2)$

$
\text { radius }=\sqrt{1+1+4+19}=5
$
Perpendicular distance $=\frac{12}{3}=4$
Hence, $r^2=5^2-4^2=3^2$

$
r=3
$
Hence, the answer is 3

Example 5: The shortest distance from the plane $12 x+4 y+3 z=327$ to the sphere $x^2+y^2+z^2+4 x-2 y-6 z=155$ is

Solution:
Center of sphere $=\mathrm{C}(-2,1,3)$ and $\mathrm{r}=13$

$
\begin{aligned}
& \text { Distance of centre from plane }=\frac{|12(-2)+4(1)+3(3)-327|}{13} \\
& =\frac{338}{13}=26
\end{aligned}
$
Hence shortest distance is $26-13=13$ units.
Hence, the answer is $13$

Summary

The equation of a sphere, $(x-h)^2+(y-k)^2+(z-I)^2=r^2$, defines a geometrically perfect three-dimensional surface where every point is equidistant from a central point $(h, k, l)$ by a fixed radius $r$. The knowledge of the sphere provides not only arithmetic but also geometrical insights. Understanding the equation of the sphere helps us to solve and analyze real-life applications.